Deck 12: Polynomial Functions of Higher Degree
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/63
Play
Full screen (f)
Deck 12: Polynomial Functions of Higher Degree
1
Determine the number of turning points of the graph of the function.
A)Number of turning points: 3
B)Number of turning points: 1
C)Number of turning points: 5
D)Number of turning points: 2
E)Number of turning points: 4
A)Number of turning points: 3
B)Number of turning points: 1
C)Number of turning points: 5
D)Number of turning points: 2
E)Number of turning points: 4
Number of turning points: 1
2
Find all the real zeros of the polynomial function and determine the multiplicity of each zero and the number of turning points of the graph of the function.
A)All Real Zeros: 0, ;Even multiplicity;number of turning points: 2
B)All Real Zeros: ;Even multiplicity;number of turning points: 1
C)All Real Zeros: 0, ;Odd multiplicity;number of turning points: 2
D)All Real Zeros: 0,1, ;Even multiplicity;number of turning points: 3
E)All Real Zeros: ;Odd multiplicity;number of turning points: 1
A)All Real Zeros: 0, ;Even multiplicity;number of turning points: 2
B)All Real Zeros: ;Even multiplicity;number of turning points: 1
C)All Real Zeros: 0, ;Odd multiplicity;number of turning points: 2
D)All Real Zeros: 0,1, ;Even multiplicity;number of turning points: 3
E)All Real Zeros: ;Odd multiplicity;number of turning points: 1
All Real Zeros: ;Even multiplicity;number of turning points: 1
3
Select from the following which is the polynomial function that has the given zeros.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)
4
Select the graph of and the transformation .
A)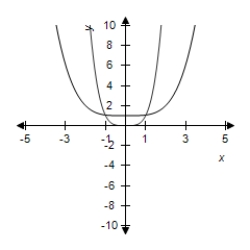
B)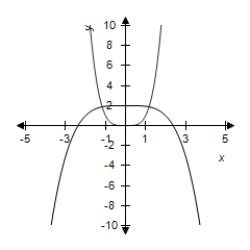
C)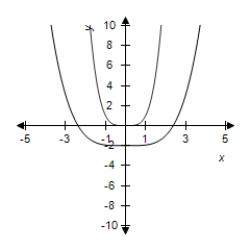
D)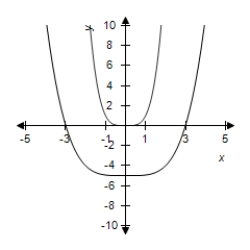
E)
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
5
Select from the following which is the polynomial function that has the given zeros.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
6
Select the graph of and the transformation .
A)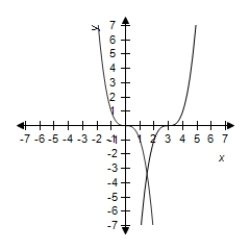
B)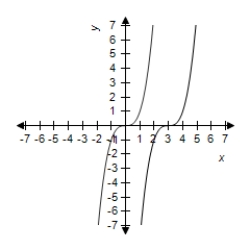
C)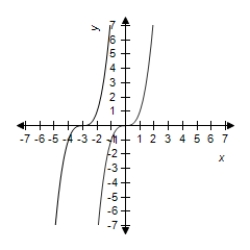
D)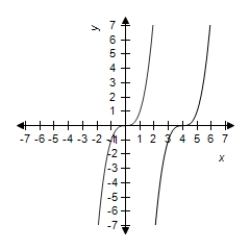
E)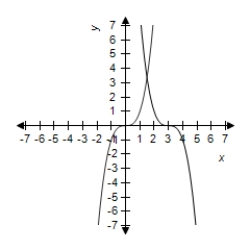
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
7
Select the correct graph of the function.
A)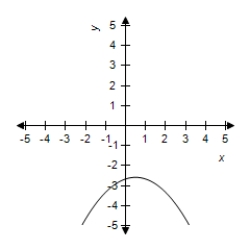
B)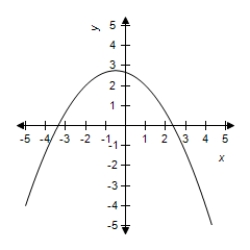
C)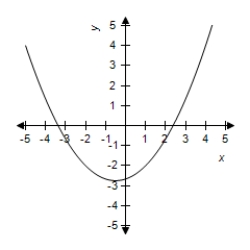
D)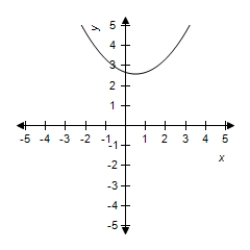
E)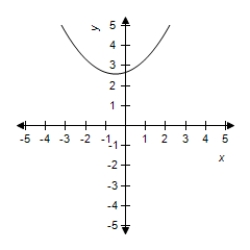
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
8
Select the graph of and the transformation .
A)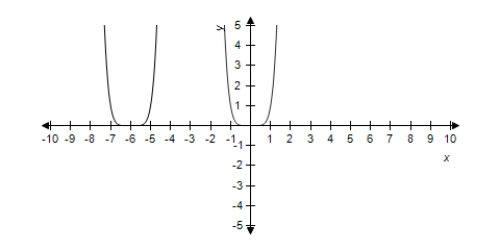
B)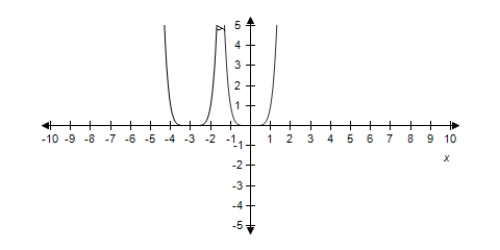
C)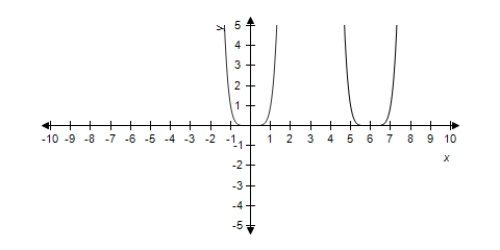
D)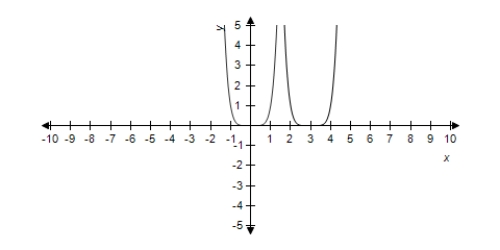
E)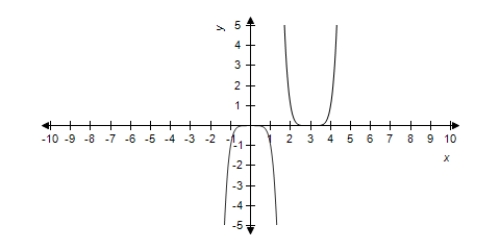
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
9
Select the correct graph of the functions f and g which shows the right-hand and left-hand behaviors of f and g appear identical. ,
A)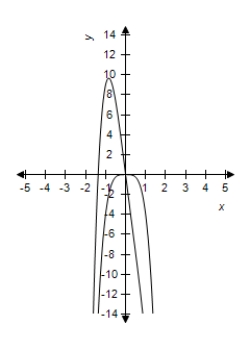
B)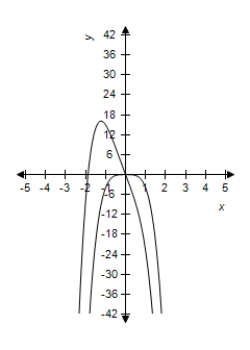
C)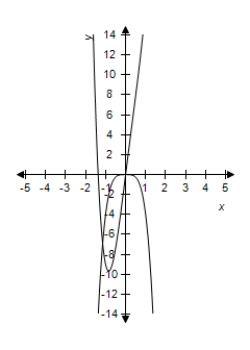
D)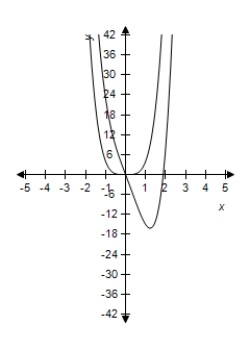
E)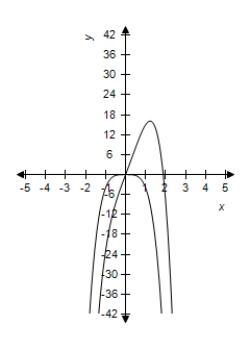
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
10
Select the correct description of right-hand and left-hand behavior of the graph of the polynomial function.
A)Rises to the left,falls to the right
B)Rises to the right,rises to the left
C)Falls to the left,rises to the right
D)Falls to the right
E)Falls to the left,falls to the right
A)Rises to the left,falls to the right
B)Rises to the right,rises to the left
C)Falls to the left,rises to the right
D)Falls to the right
E)Falls to the left,falls to the right

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
11
Select the graph of and the transformation .
A)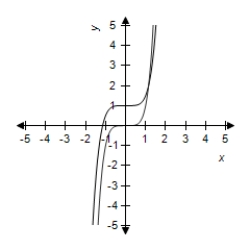
B)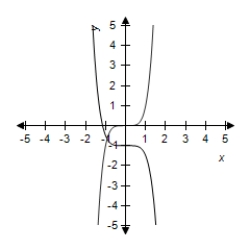
C)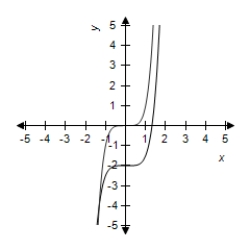
D)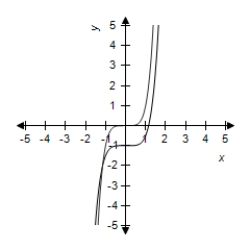
E)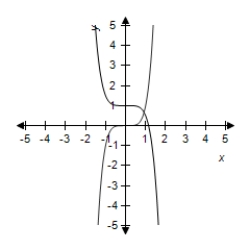
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
12
Select the correct description of right-hand and left-hand behavior of the graph of the polynomial function.
A)Falls to the left,rises to the right
B)Falls to the left,falls to the right
C)Rises to the left,rises to the right
D)Rises to the left,falls to the right
E)Falls to the left
A)Falls to the left,rises to the right
B)Falls to the left,falls to the right
C)Rises to the left,rises to the right
D)Rises to the left,falls to the right
E)Falls to the left

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
13
Select the correct graph of the functions f and g in the same viewing window.Zoom out sufficiently far to show that the right-hand and left-hand behaviors of f and g appear identical. ,
A)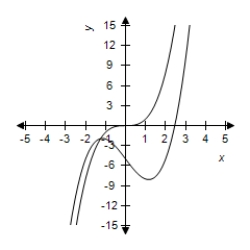
B)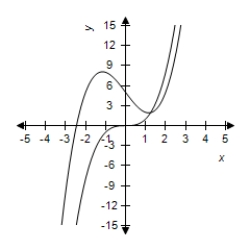
C)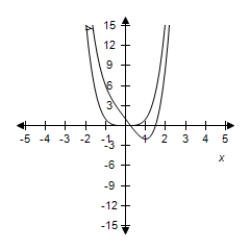
D)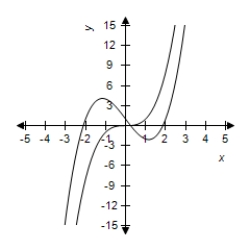
E)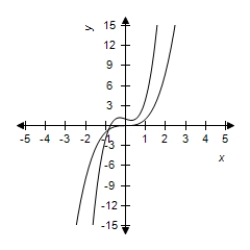
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
14
Select the correct graph of the function.
A)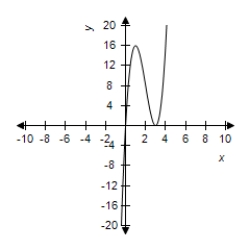
B)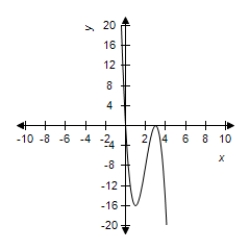
C)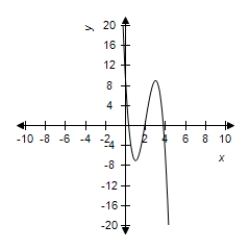
D)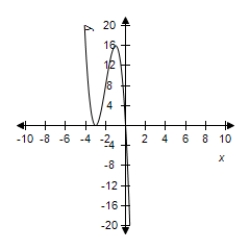
E)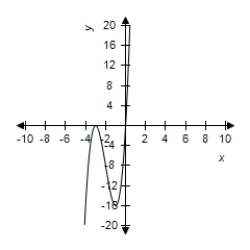
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
15
Find all the real zeros of the polynomial function.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
16
Select from the following which is the polynomial function that has the given zeros.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
17
Select the correct description of right-hand and left-hand behavior of the graph of the polynomial function.
A)Falls to the left,falls to the right
B)Rises to the left,rises to the right
C)Rises to the left,falls to the right
D)Falls to the left,rises to the right
E)Falls to the left
A)Falls to the left,falls to the right
B)Rises to the left,rises to the right
C)Rises to the left,falls to the right
D)Falls to the left,rises to the right
E)Falls to the left

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
18
Select the correct description of right-hand and left-hand behavior of the graph of the polynomial function.
A)Rises to the left,rises to the right
B)Falls to the left,rises to the right
C)Falls to the left,falls to the right
D)Rises to the left,falls to the right
E)Rises to the left
A)Rises to the left,rises to the right
B)Falls to the left,rises to the right
C)Falls to the left,falls to the right
D)Rises to the left,falls to the right
E)Rises to the left

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
19
Select the correct graph of the function.
A)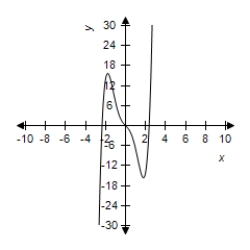
B)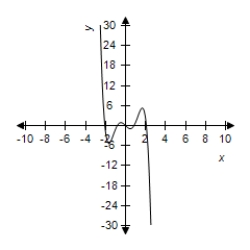
C)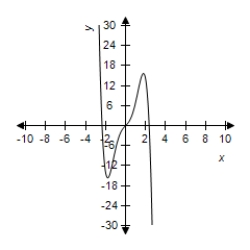
D)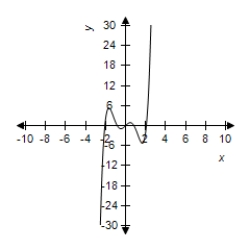
E)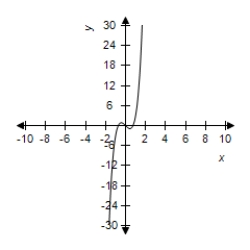
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
20
Find all the real zeros of the polynomial function.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
21
Select from the following which is the polynomial function that has the given zeros.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
22
Select the graph of the function and use the zero or root feature to approximate the real zeros of the function.
A)Zeros: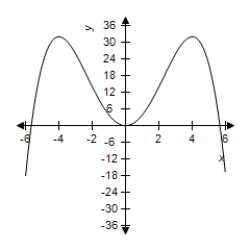
B)Zeros: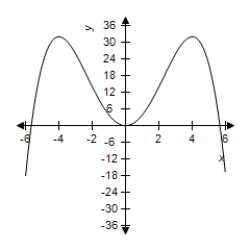
C)Zeros: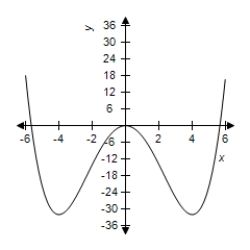
D)Zeros: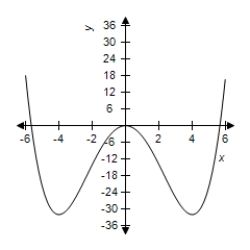
E)Zeros: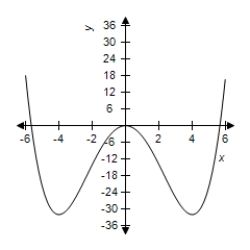
A)Zeros:

B)Zeros:

C)Zeros:

D)Zeros:

E)Zeros:


Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
23
Select from the following which is the polynomial of degree n that has the given zero(s).
Zero
Degree
A)
B)
C)
D)
E)
Zero
Degree
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
24
Select from the following which is the polynomial function that has the given zeros.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
25
Select from the following which is the polynomial of degree n that has the given zero(s).
Zeros
Degree
A)
B)
C)
D)
E)
Zeros
Degree
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
26
Select the graph of the function and determine the zeros of the polynomial.
A)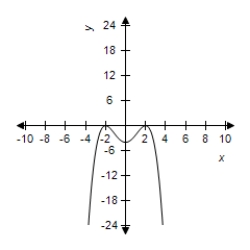
B)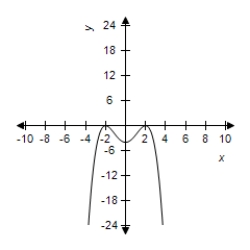
C)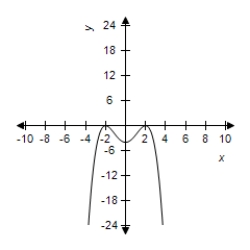
D)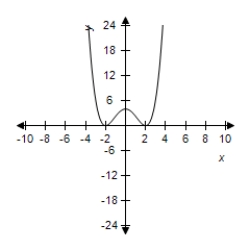
E)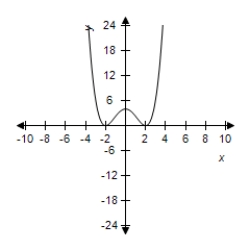
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
27
Select the graph of the function and use the zero or root feature to approximate the real zeros of the function.
A) Zeros: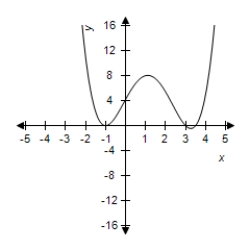
B) Zeros: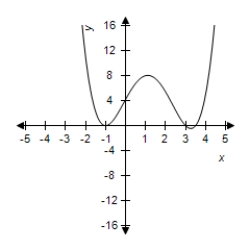
C) Zeros: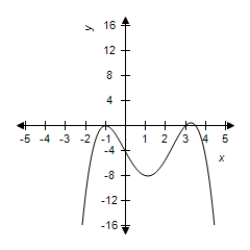
D) Zeros: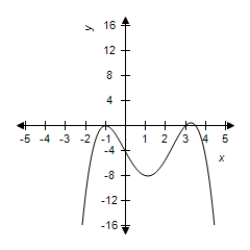
E) Zeros: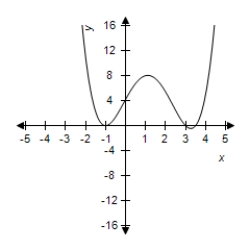
A) Zeros:

B) Zeros:

C) Zeros:

D) Zeros:

E) Zeros:


Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
28
Select from the following which is the polynomial of degree n that has the given zero(s).
Zero
Degree
A)
B)
C)
D)
E)
Zero
Degree
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
29
An open box is to be made from a square piece of material,38 inches on a side,by cutting equal squares with sides of length x from the corners and turning up the sides (see figure) 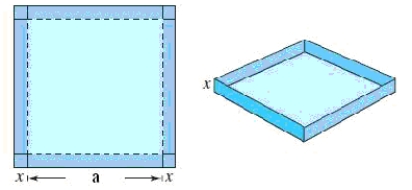 where .
where .
Determine the domain of the following function,V(x)represents the volume of the box .
A)Domain:
B)Domain:
C)Domain:
D)Domain:
E)Domain:
 where .
where .Determine the domain of the following function,V(x)represents the volume of the box .
A)Domain:
B)Domain:
C)Domain:
D)Domain:
E)Domain:

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
30
Select from the following which is the polynomial of degree n that has the given zero(s).
Zero
Degree
A)
B)
C)
D)
E)
Zero
Degree
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
31
Select the graph of the function and determine the zeros of the polynomial.
A)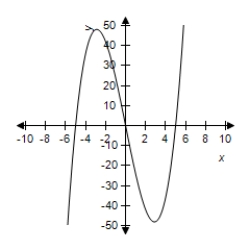
B)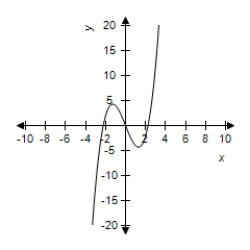
C)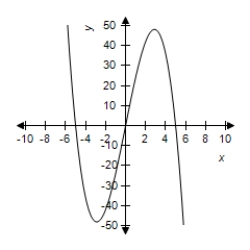
D)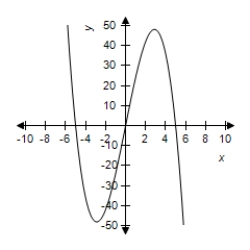
E)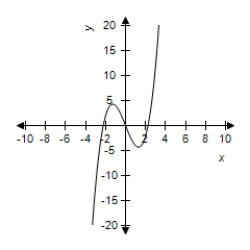
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
32
Select the graph of the function and determine the zeros of the polynomial.
A) No zeros
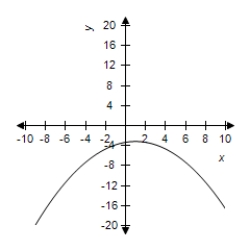
B) No zeros
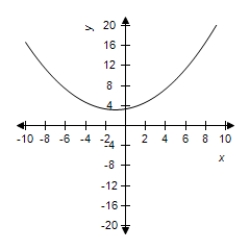
C)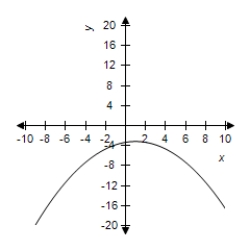
D) No zeros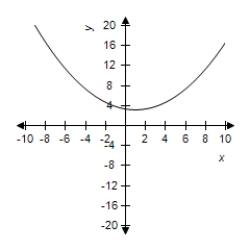
E)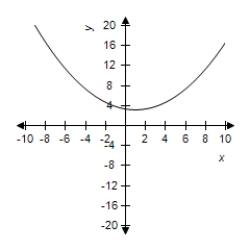
A) No zeros

B) No zeros

C)

D) No zeros

E)


Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
33
Match the equation with its graph.
A)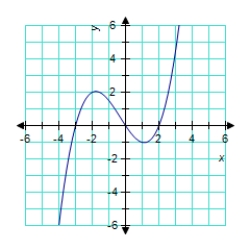
B)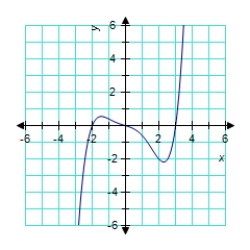
C)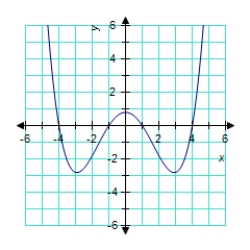
D)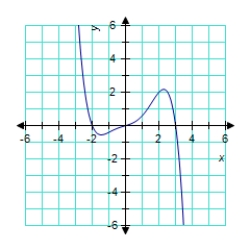
E)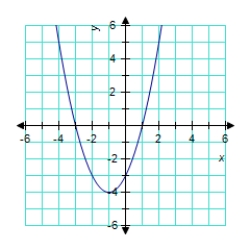
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
34
Select the graph of the function and use the zero or root feature to approximate the real zeros of the function.
A)Zeros: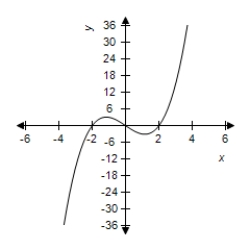
B)Zeros: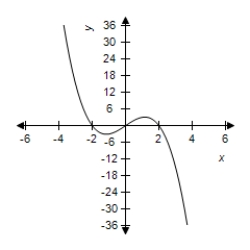
C)Zeros: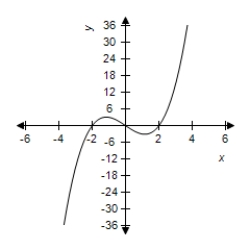
D)Zeros: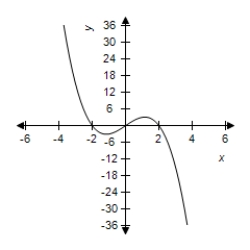
E)Zeros: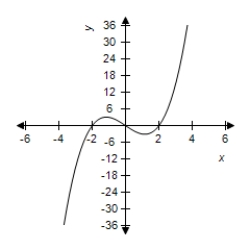
A)Zeros:

B)Zeros:

C)Zeros:

D)Zeros:

E)Zeros:


Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
35
Select the graph of the function and determine the zeros of the polynomial.
A) 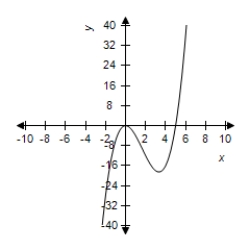
B)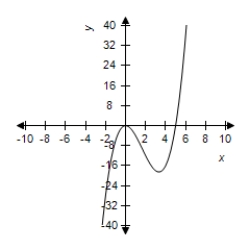
C)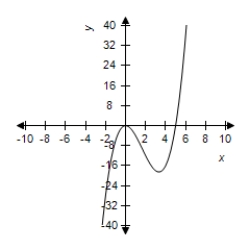
D)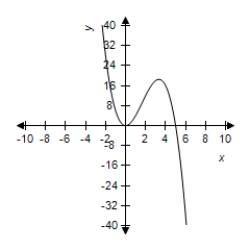
E)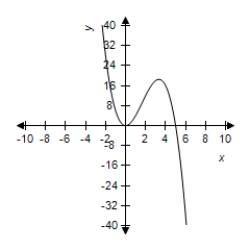
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
36
Describe the right-hand and the left-hand behavior of the graph of .
A)Because the degree is even and the leading coefficient is negative,the graph falls to the left and rises to the right.
B)Because the degree is even and the leading coefficient is negative,the graph rises to the left and falls to the right.
C)Because the degree is even and the leading coefficient is negative,the graph falls to the left and falls to the right.
D)Because the degree is odd and the leading coefficient is negative,the graph rises to the left and rises to the right.
E)Because the degree is even and the leading coefficient is negative,the graph rises to the left and rises to the right.
A)Because the degree is even and the leading coefficient is negative,the graph falls to the left and rises to the right.
B)Because the degree is even and the leading coefficient is negative,the graph rises to the left and falls to the right.
C)Because the degree is even and the leading coefficient is negative,the graph falls to the left and falls to the right.
D)Because the degree is odd and the leading coefficient is negative,the graph rises to the left and rises to the right.
E)Because the degree is even and the leading coefficient is negative,the graph rises to the left and rises to the right.

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
37
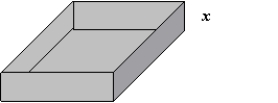
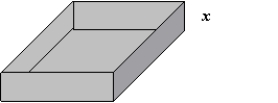
A roofing contractor is fabricating gutters from -inch aluminum sheeting.The contractor plans to use an aluminum siding folding press to create the gutter by creasing equal lengths for the sidewalls (see figure). 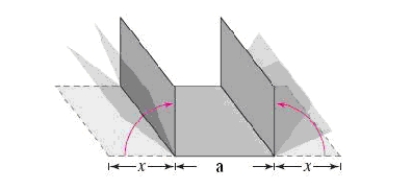 where Let x represent the height of the sidewall of the gutter.Write a function A that represents the
where Let x represent the height of the sidewall of the gutter.Write a function A that represents the
Cross-sectional area of the gutter.
A)
B)
C)
D)
E)
 where Let x represent the height of the sidewall of the gutter.Write a function A that represents the
where Let x represent the height of the sidewall of the gutter.Write a function A that represents theCross-sectional area of the gutter.
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
38
Sketch the graph of the function by finding the zeros of the polynomial,
A) 0,2,3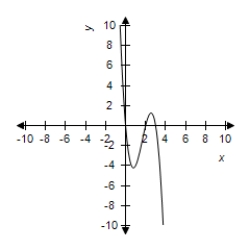
B) 0,2,-3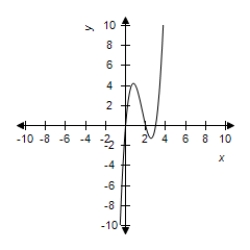
C) 0,-2,3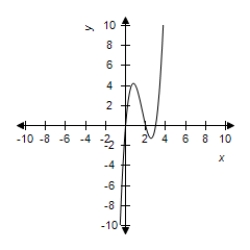
D) 0,2,3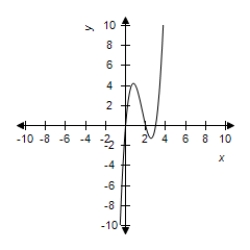
E) 0,-2,-3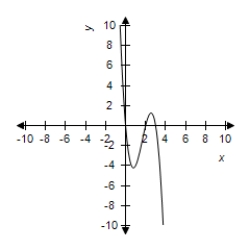
A) 0,2,3

B) 0,2,-3

C) 0,-2,3

D) 0,2,3

E) 0,-2,-3


Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
39
Select the graph of the function and determine the zeros of the polynomial.
A)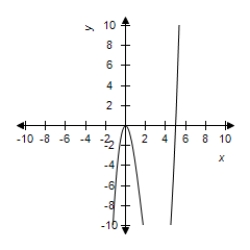
B)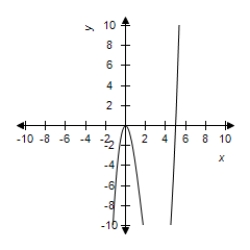
C)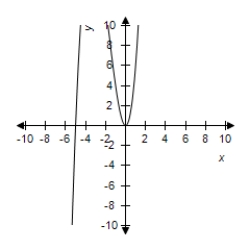
D)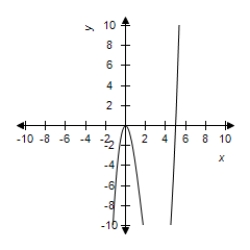
E)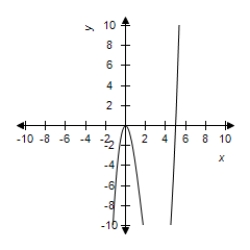
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
40
The growth of a red oak tree is approximated by the function
Where G is the height of the tree (in feet)and t is its age (in years).
Select the correct graph of the function.
A) 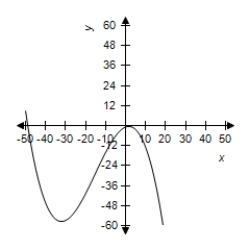
B)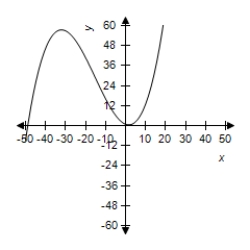
C)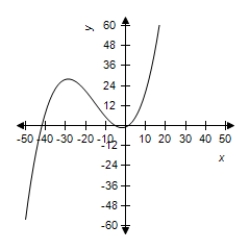
D)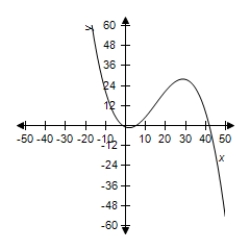
E)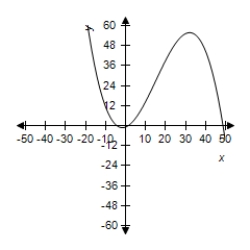
Where G is the height of the tree (in feet)and t is its age (in years).
Select the correct graph of the function.
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
41
Find a polynomial with the given zeros.
A)
B)
C)
D)
E)none of these
A)
B)
C)
D)
E)none of these

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
42
Using a graphing utility,graph and approximate the zeros and their multiplicity.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
43
An open box is to be made from a square piece of cardboard,22 inches on a side,by cutting equal squares with sides of length x from the corners and turning up the sides (see figure below).If the volume of the box is represented by ,determine the domain of . 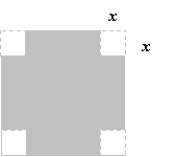
A)
B)
C)
D)
E)


A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
44
Find a polynomial with the given zeros.
A)
B)
C)
D)
E)none of these
A)
B)
C)
D)
E)none of these

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
45
Graph the polynomial function.
A)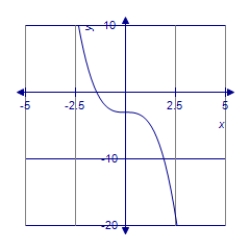
B)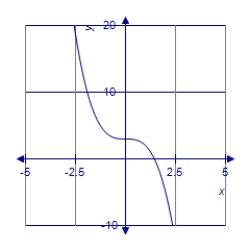
C)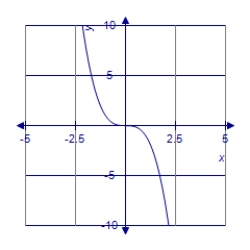
D)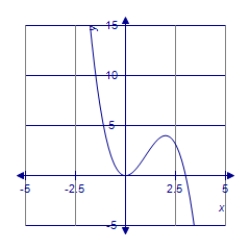
A)

B)

C)

D)


Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
46
Well whether the function is even or odd.If it is neither,so indicate.
A)even
B)odd
C)neither
A)even
B)odd
C)neither

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
47
Find all real zeros of the polynomial and determine the multiplicity of each.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
48
Find all real zeros of the polynomial and determine the multiplicity of each.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
49
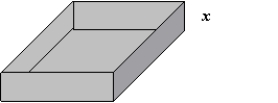
An open box is to be made from a square piece of cardboard,33 inches on a side,by cutting equal squares with sides of length x from the corners and turning up the sides (see figure below).Determine the function,V,in terms of x,that represents the volume of the box. 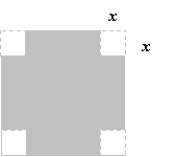
A)
B)
C)
D)
E)


A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
50
Using a graphing utility,graph and approximate the zeros and their multiplicity.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
51
Find all real zeros of the polynomial and determine the multiplicity of each.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
52
Find all real zeros of the polynomial and determine the multiplicity of each.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
53
Graph the polynomial function.
A)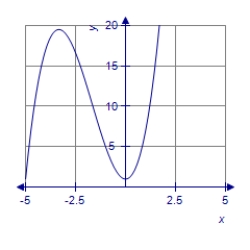
B)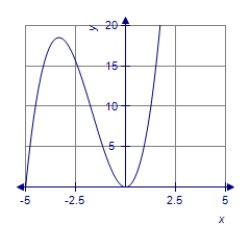
C)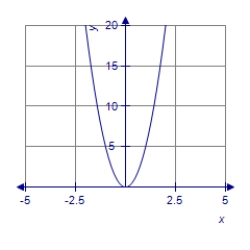
D)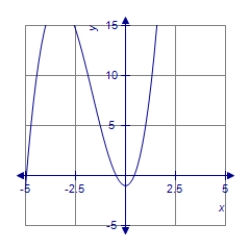
A)

B)

C)

D)


Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
54
Find a polynomial with the given zeros.
A)
B)
C)
D)
E)none of these
A)
B)
C)
D)
E)none of these

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
55
Find a polynomial with the given zeros.
A)
B)
C)
D)
E)none of these
A)
B)
C)
D)
E)none of these

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
56
The graph of the function g(x)is a translation of the graph of f (x)= x 3.Graph the function g(x)= (x - 1)3.
A)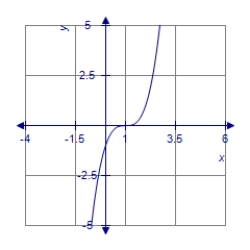
B)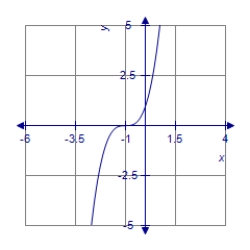
C)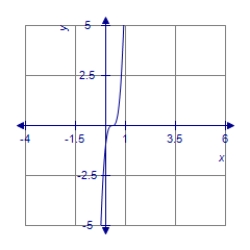
D)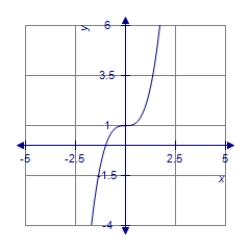
A)

B)

C)

D)


Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
57
The graph of the function g(x)is a translation of the graph of f (x)= x 3.Graph the function g(x)= (x - 3)3 - 1.
A)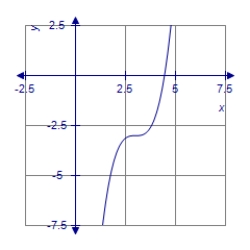
B)
C)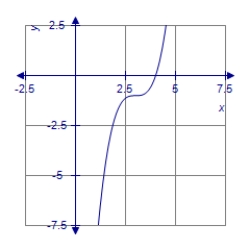
D)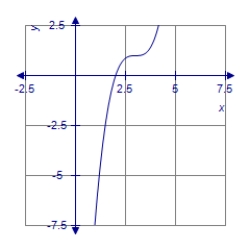
A)

B)

C)

D)


Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
58
Describe the right-hand and the left-hand behavior of the graph of .
A)Because the degree is odd and the leading and the second coefficients are positive,the graph falls to the left and rises to the right.
B)Because the degree is odd,the leading and the second coefficient are positive,the graph rises to the left and rises to the right.
C)Because the degree is odd,and the leading coefficient is positive,the graph falls to the left and falls to the right.
D)Because the degree is odd,and the leading coefficient is positive,the graph rises to the left and falls to the right.
E)Because the degree is even and the leading coefficient is positive,the graph rises to the left and rises to the right.
A)Because the degree is odd and the leading and the second coefficients are positive,the graph falls to the left and rises to the right.
B)Because the degree is odd,the leading and the second coefficient are positive,the graph rises to the left and rises to the right.
C)Because the degree is odd,and the leading coefficient is positive,the graph falls to the left and falls to the right.
D)Because the degree is odd,and the leading coefficient is positive,the graph rises to the left and falls to the right.
E)Because the degree is even and the leading coefficient is positive,the graph rises to the left and rises to the right.

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
59
Find all real zeros of the polynomial and determine the multiplicity of each.
A)
B)
C)
D)
A)
B)
C)
D)

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
60
Describe the right-hand and the left-hand behavior of the graph of .
A)Because the degree is odd and the leading coefficient is negative,the graph rises to the left and falls to the right.
B)Because the degree is odd and the leading coefficient is negative,the graph falls to the left and rises to the right.
C)Because the degree is odd and the leading coefficient is positive,the graph falls to the left and falls to the right.
D)Because the degree is odd and the leading coefficient is positive,the graph rises to the left and rises to the right.
E)Because the degree is even and the leading coefficient is negative,the graph rises to the left and falls to the right.
A)Because the degree is odd and the leading coefficient is negative,the graph rises to the left and falls to the right.
B)Because the degree is odd and the leading coefficient is negative,the graph falls to the left and rises to the right.
C)Because the degree is odd and the leading coefficient is positive,the graph falls to the left and falls to the right.
D)Because the degree is odd and the leading coefficient is positive,the graph rises to the left and rises to the right.
E)Because the degree is even and the leading coefficient is negative,the graph rises to the left and falls to the right.

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
61
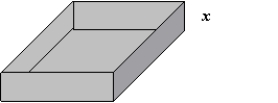
An open box is to be made from a square piece of cardboard,24 inches on a side,by cutting equal squares with sides of length x from the corners and turning up the sides (see figure below).If the volume of the box is represented by ,determine the domain of . 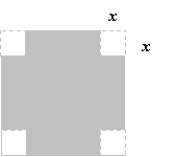
A)
B)
C)
D)
E)


A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
62
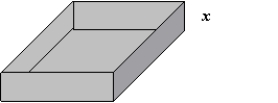
An open box is to be made from a square piece of cardboard,28 inches on a side,by cutting equal squares with sides of length x from the corners and turning up the sides (see figure below).Determine the function,V,in terms of x,that represents the volume of the box. 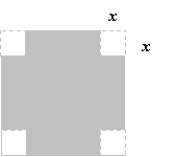
A)
B)
C)
D)
E)


A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
63
An open box is to be made from a square piece of cardboard, on a side,by cutting equal squares with sides of length x from the corners and turning up the sides (see figure below).After determining the function V,in terms of x,that represents the volume of the box,use a graphing utility to estimate the dimensions that will maximize its volume. 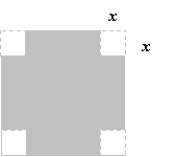
A)
B)
C)
D)
E)


A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck



