Deck 22: Exponential and Logarithmic Models
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/56
Play
Full screen (f)
Deck 22: Exponential and Logarithmic Models
1
An initial investment of $4000 grows at an annual interest rate of 5% compounded continuously.How long will it take to double the investment?
A)1 year
B)14.40 years
C)13.86 years
D)14.86 years
E)13.40 years
A)1 year
B)14.40 years
C)13.86 years
D)14.86 years
E)13.40 years
13.86 years
2
Select the correct graph for the given function
A)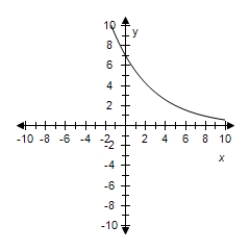
B)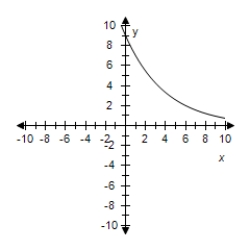
C)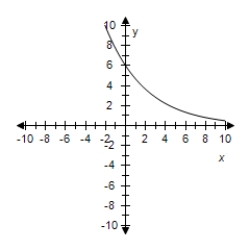
D)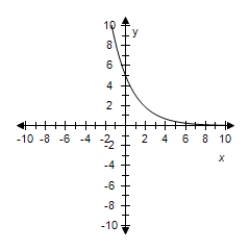
E)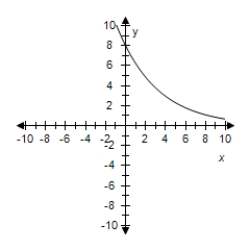
A)

B)

C)

D)

E)



3
An initial investment of $5000 doubles in value in 6.3 years.Assuming continuous compounding,what was the interest rate? Round to the nearest tenth of a percent.
A)11.0%
B)4.8%
C)5.5%
D)6.3%
E)100%
A)11.0%
B)4.8%
C)5.5%
D)6.3%
E)100%
11.0%
4
Complete the table for a savings account in which interest is compounded continuously.
(Round the answer up to two decimal places. )
A)Time to double: Amount after 10 years:
B)Time to double: Amount after 10 years:
C)Time to double: Amount after 10 years:
D)Time to double: Amount after 10 years:
E)Time to double: Amount after 10 years:
(Round the answer up to two decimal places. )
A)Time to double: Amount after 10 years:
B)Time to double: Amount after 10 years:
C)Time to double: Amount after 10 years:
D)Time to double: Amount after 10 years:
E)Time to double: Amount after 10 years:

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
5
Select the correct graph for the given function
A)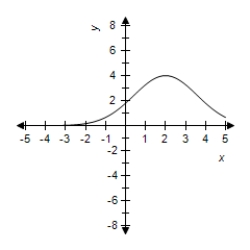
B)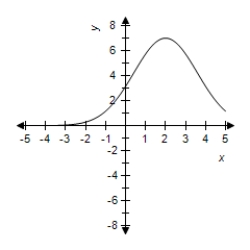
C)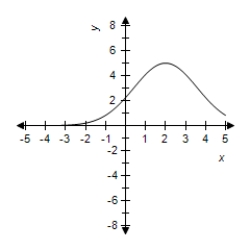
D)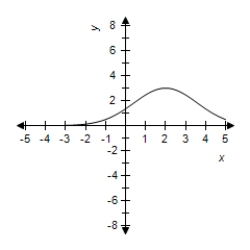
E)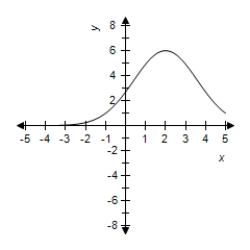
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
6
Complete the table for a savings account in which interest is compounded continuously.
(Round the answer up to two decimal places. )
A)Annual rate: Amount after 10 years:
B)Annual rate: Amount after 10 years:
C)Annual rate: Amount after 10 years:
D)Annual rate: Amount after 10 years:
E)Annual rate: Amount after 10 years:
(Round the answer up to two decimal places. )
A)Annual rate: Amount after 10 years:
B)Annual rate: Amount after 10 years:
C)Annual rate: Amount after 10 years:
D)Annual rate: Amount after 10 years:
E)Annual rate: Amount after 10 years:

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
7
Complete the table for a savings account in which interest is compounded continuously. (Round the answer up to two decimal places. )
A)Annual rate: Time to double: 10
B)Annual rate: Time to double: 15 yr
C)Annual rate: Time to double: 10
D)Annual rate: Time to double: 14 yr
E)Annual rate: Time to double: 10
A)Annual rate: Time to double: 10
B)Annual rate: Time to double: 15 yr
C)Annual rate: Time to double: 10
D)Annual rate: Time to double: 14 yr
E)Annual rate: Time to double: 10

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
8
Complete the table for a savings account in which interest is compounded continuously. (Round the answer up to two decimal places. )
A)Annual rate: Time to double: 14 yr
B)Annual rate: Time to double: 11 yr
C)Annual rate: Time to double: 11 yr
D)Annual rate: Time to double: 11 yr
E)Annual rate: Time to double: 15 yr
A)Annual rate: Time to double: 14 yr
B)Annual rate: Time to double: 11 yr
C)Annual rate: Time to double: 11 yr
D)Annual rate: Time to double: 11 yr
E)Annual rate: Time to double: 15 yr

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
9
Which investment option will pay the most interest?
A)10.2% compounded quarterly
B)10.4% compounded semiannually
C)10.0% compounded continuously
D)These investments all pay the same amount of interest.
E)10.6% compounded annually
A)10.2% compounded quarterly
B)10.4% compounded semiannually
C)10.0% compounded continuously
D)These investments all pay the same amount of interest.
E)10.6% compounded annually

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
10
Complete the table for a savings account in which interest is compounded continuously. (Round the answer up to two decimal places. )
A)Initial investment: Time to double: 13 yr
B)Initial investment: Time to double: 10 yr
C)Initial investment: Time to double: 12 yr
D)Initial investment: Time to double: 12 yr
E)Initial investment: Time to double: 12 yr
A)Initial investment: Time to double: 13 yr
B)Initial investment: Time to double: 10 yr
C)Initial investment: Time to double: 12 yr
D)Initial investment: Time to double: 12 yr
E)Initial investment: Time to double: 12 yr

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
11
Select the correct graph for the given function
A)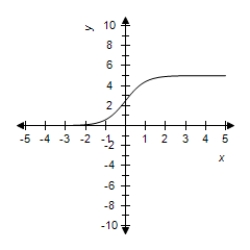
B) 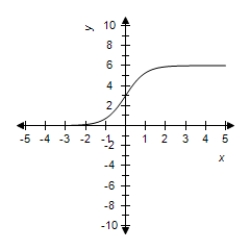
C)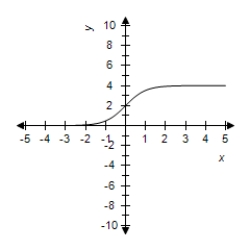
D)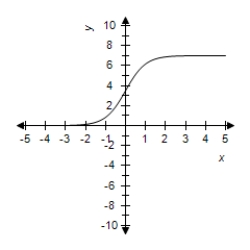
E)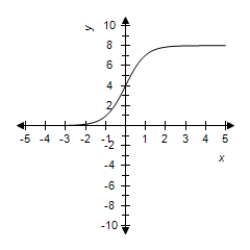
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
12
Select a scatter plot of the given data.
R t
A)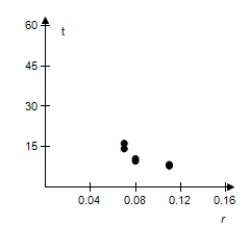
B)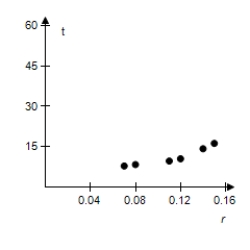
C)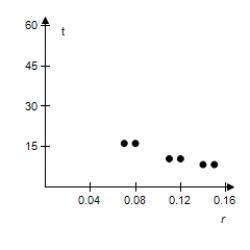
D)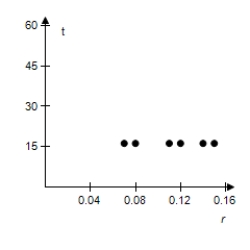
E)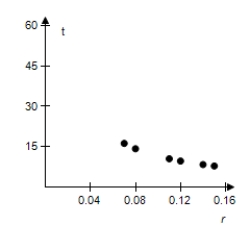
R t
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
13
Complete the table for the time t (in years)necessary for P dollars to triple if interest is compounded continuously at rate r.
(Round the answer up to two decimal places. )
A)
B)
C)
D)
E)
(Round the answer up to two decimal places. )
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
14
Select the correct graph for the given function
A)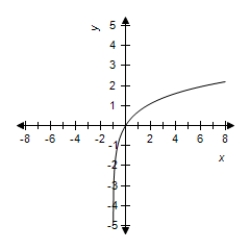
B)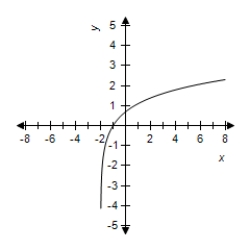
C)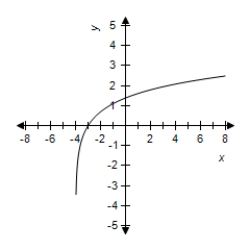
D)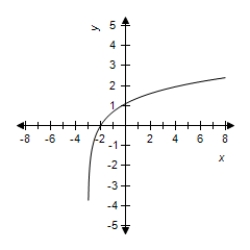
E)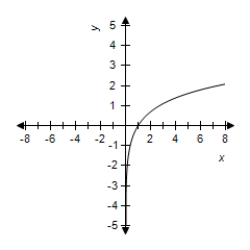
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
15
Complete the table for a savings account in which interest is compounded continuously.
(Round the answer up to two decimal places. )
A)Annual rate: Amount after 10 years:
B)Annual rate: Amount after 10 years:
C)Annual rate: Amount after 10 years:
D)Annual rate: Amount after 10 years:
E)Annual rate: Amount after 10 years:
(Round the answer up to two decimal places. )
A)Annual rate: Amount after 10 years:
B)Annual rate: Amount after 10 years:
C)Annual rate: Amount after 10 years:
D)Annual rate: Amount after 10 years:
E)Annual rate: Amount after 10 years:

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
16
Select the correct graph for the given function
A) 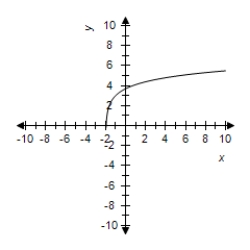
B)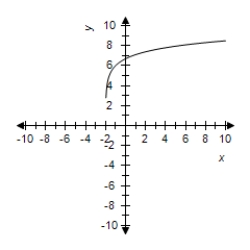
C)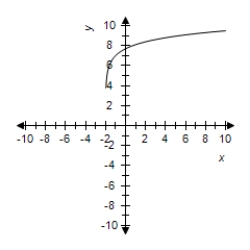
D)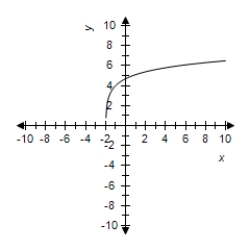
E)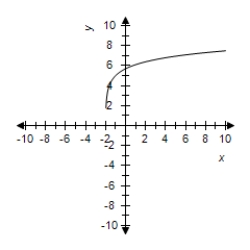
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
17
Complete the table for a savings account in which interest is compounded continuously.
(Round the answer up to two decimal places. )
A)Time to double: Amount after 10 years:
B)Time to double: Amount after 10 years:
C)Time to double: Amount after 10 years:
D)Time to double: Amount after 10 years:
E)Time to double: Amount after 10 years:
(Round the answer up to two decimal places. )
A)Time to double: Amount after 10 years:
B)Time to double: Amount after 10 years:
C)Time to double: Amount after 10 years:
D)Time to double: Amount after 10 years:
E)Time to double: Amount after 10 years:

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
18
Determine the principal that must be invested at rate r,compounded monthly,so that will be available for retirement in t years.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
19
Complete the table for a savings account in which interest is compounded continuously.
A)Initial investment: Time to double: 10 yr
B)Initial investment: Time to double: 10 yr
C)Initial investment: Time to double: 10 yr
D)Initial investment: Time to double: 10 yr
E)Initial investment: Time to double: 10 yr
A)Initial investment: Time to double: 10 yr
B)Initial investment: Time to double: 10 yr
C)Initial investment: Time to double: 10 yr
D)Initial investment: Time to double: 10 yr
E)Initial investment: Time to double: 10 yr

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
20
Select the correct graph for the given function
A)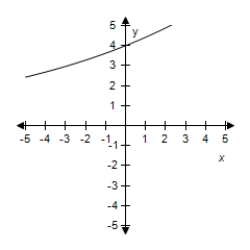
B)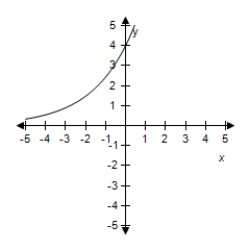
C)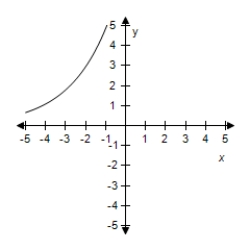
D)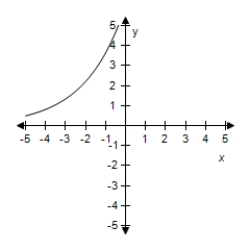
E)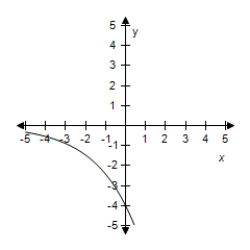
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
21
If $1 is invested in an account over a 10-year period,the amount in the account,where t represents the time in years,is given by depending on whether the account pays simple interest at or continuous compound interest at 7%.Graph each function on the same set of axes.Which grows at a higher rate?
A)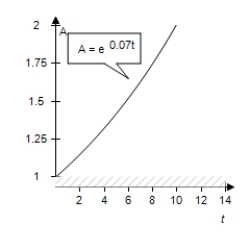
B)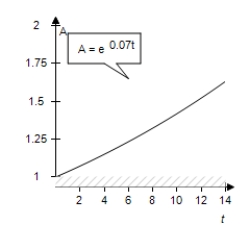
C)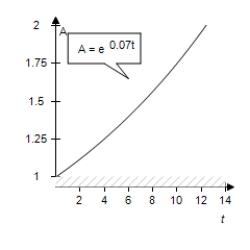
D)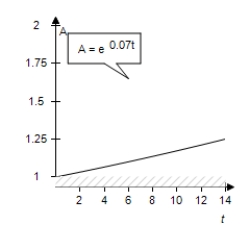
E)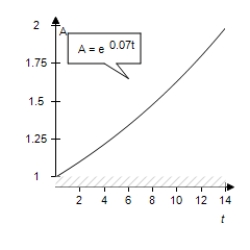
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
22
Complete the table for the radioactive isotope.
A)Initial Quantity:
B)Initial Quantity:
C)Initial Quantity:
D)Initial Quantity:
E)Initial Quantity:
A)Initial Quantity:
B)Initial Quantity:
C)Initial Quantity:
D)Initial Quantity:
E)Initial Quantity:

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
23
Find the exponential model that fits the points shown in the table.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
24
Сomplete the table for the radioactive isotope.
A)Initial Quantity:
B)Initial Quantity:
C)Initial Quantity:
D)Initial Quantity:
E)Initial Quantity:
A)Initial Quantity:
B)Initial Quantity:
C)Initial Quantity:
D)Initial Quantity:
E)Initial Quantity:

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
25
Find the exponential model that fits the points shown in the graph. 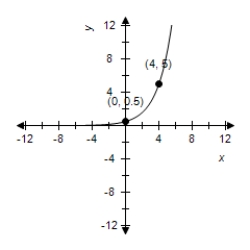
A)
B)
C)
D)
E)

A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
26
The value V (in millions of dollars)of a famous painting can be modeled by where t represents the year,with t = 0 corresponding to 2000.In 2008,the same painting was sold for $65 million.Predict the value of the painting in 2018.(Round your answer to two decimal places. )
A)$674.61 million
B)$874.61 million
C)$474.61 million
D)$774.61 million
E)$574.61 million
A)$674.61 million
B)$874.61 million
C)$474.61 million
D)$774.61 million
E)$574.61 million

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
27
The population P of a culture of bacteria is described by the equation ,where t is the time,in hours,relative to the time at which the population was 1300.What was the population at hours?
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
28
Complete the table for the radioactive isotope.Round your answer to two decimal places.
A)Amount after 1000 years:
B)Amount after 1000 years:
C)Amount after 1000 years:
D)Amount after 1000 years:
E)Amount after 1000 years:
A)Amount after 1000 years:
B)Amount after 1000 years:
C)Amount after 1000 years:
D)Amount after 1000 years:
E)Amount after 1000 years:

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
29
Complete the table for the radioactive isotope.
A)Initial Quantity:
B)Initial Quantity:
C)Initial Quantity:
D)Initial Quantity:
E)Initial Quantity:
A)Initial Quantity:
B)Initial Quantity:
C)Initial Quantity:
D)Initial Quantity:
E)Initial Quantity:

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
30
The population P of a bacteria culture is modeled by ,where t is the time in hours.If the population of the culture was 5800 after 40 hours,how long does it take for the population to double? Round to the nearest tenth of an hour.
A)54.8 hours
B)8.9 hours
C)79.9 hours
D)81.7 hours
E)56.6 hours
A)54.8 hours
B)8.9 hours
C)79.9 hours
D)81.7 hours
E)56.6 hours

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
31
The number y of hits a new search-engine website receives each month can be modeled by where t represents the number of months the website has been operating.In the website's third month,there were 10,000 hits.Find the value of k,and use this value to predict the number of hits the website will receive after 22 months.
A) ;About 184,894,691,979 hits
B) ;About 2,921,047 hits
C) ;About 22,832,533,585 hits
D) ;About 57,970,933 hits
E) ;About 1,150,488,266 hits
A) ;About 184,894,691,979 hits
B) ;About 2,921,047 hits
C) ;About 22,832,533,585 hits
D) ;About 57,970,933 hits
E) ;About 1,150,488,266 hits

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
32
The populations P (in thousands)of Horry County,South Carolina from 1970 through 2007 can be modeled by where t represents the year,with corresponding to 1970.Use the model to complete the table.Round your answer to two decimal places.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
33
Find the exponential model that fits the points shown in the graph. 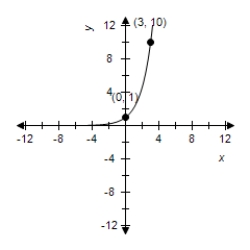
A)
B)
C)
D)
E)

A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
34
Complete the table for the radioactive isotope.Round your answer to two decimal places.
A)Amount after 1000 years:
B)Amount after 1000 years:
C)Amount after 1000 years:
D)Amount after 1000 years:
E)Amount after 1000 years:
A)Amount after 1000 years:
B)Amount after 1000 years:
C)Amount after 1000 years:
D)Amount after 1000 years:
E)Amount after 1000 years:

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
35
Find the exponential model that fits the points shown in the table.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
36
Select a scatter plot of the given data. r
4%
6%
8%
10%
12%
14%
T
28)01
18)85
14)27
11)53
9)69
8)38
A)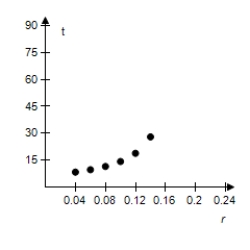
B)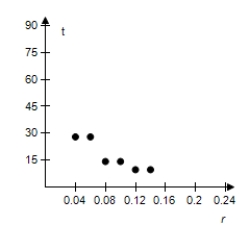
C)
D)
E)
4%
6%
8%
10%
12%
14%
T
28)01
18)85
14)27
11)53
9)69
8)38
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
37
The population P (in thousands)of Reno,Nevada from 2000 through 2007 can be modeled by where t represents the year,with corresponding to 2000.In 2005,the population of Reno was about 395,000.According to the model,during what year will the population reach 486,000.00?
A)2013
B)2005
C)2021
D)2017
E)2009
A)2013
B)2005
C)2021
D)2017
E)2009

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
38
Complete the table for the radioactive isotope.Round your answer to two decimal places.
A)Amount after 1000 years:
B)Amount after 1000 years:
C)Amount after 1000 years:
D)Amount after 1000 years:
E)Amount after 1000 years:
A)Amount after 1000 years:
B)Amount after 1000 years:
C)Amount after 1000 years:
D)Amount after 1000 years:
E)Amount after 1000 years:

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
39
The population P (in thousands)of Orlando,Florida from 2000 through 2007 can be modeled by where t represents the year,with corresponding to 2000.In 2006,the population of Orlando,Florida was about 1,883,000.00.Find the value of k.
A)k = 0.03183
B)k = 0.02863
C)k = 0.02163
D)k = 0.03453
E)k = 0.03063
A)k = 0.03183
B)k = 0.02863
C)k = 0.02163
D)k = 0.03453
E)k = 0.03063

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
40
Complete the table for the time t (in years)necessary for P dollars to triple if interest is compounded annually at rate r.Round your answer to two decimal places.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
41
An initial investment of $2000 grows at an annual interest rate of 4% compounded continuously.How long will it take to double the investment?
A)17.33 years
B)18.33 years
C)18.00 years
D)17.00 years
E)1 year
A)17.33 years
B)18.33 years
C)18.00 years
D)17.00 years
E)1 year

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
42
The chemical acidity of a solution is measured in units of pH: ,where is the hydrogen ion concentration in the solution.What is if the ?
A)3.800
B)
C)
D)
E)
A)3.800
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
43
The population P of a culture of bacteria is described by the equation 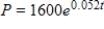 ,where t is the time,in hours,relative to the time at which the population was 1600.
,where t is the time,in hours,relative to the time at which the population was 1600.
(a)What was the population at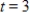 hours? Show your work.
hours? Show your work.
(b)After how many hours will the population reach 10000.00? Round to the nearest tenth of an hour.Show your work.
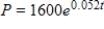 ,where t is the time,in hours,relative to the time at which the population was 1600.
,where t is the time,in hours,relative to the time at which the population was 1600.(a)What was the population at
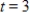 hours? Show your work.
hours? Show your work.(b)After how many hours will the population reach 10000.00? Round to the nearest tenth of an hour.Show your work.

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
44
A population growing at an annual rate r will triple in a time t given by the formula .If the growth rate remains constant and equals 9% per year,how long will it take the population of the town to triple?
A)2.2 years
B)5.3 years
C)6.6 years
D)1 years
E)12.2 years
A)2.2 years
B)5.3 years
C)6.6 years
D)1 years
E)12.2 years

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
45
The chemical acidity of a solution is measured in units of pH: ,where is the hydrogen ion concentration in the solution.If a sample of rain has a pH of 3.3,how many times higher is its than pure water's,which has a pH of 7?
A)
B)
C)
D)
E)7
A)
B)
C)
D)
E)7

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
46
Find the intensity I of an earthquake measuring R on the Richter scale .
Costa Rica in 2009,
A)
B)
C)
D)
E)
Costa Rica in 2009,
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
47
The chemical acidity of a solution is measured in units of pH: ,where is the hydrogen ion concentration in the solution.If a sample of rain has a pH of 3.4,how many times higher is its than pure water's,which has a pH of 7?
A)
B)
C)7
D)
E)
A)
B)
C)7
D)
E)

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
48
Tritium,a radioactive isotope of hydrogen,has a half-life of 12.4 years.Of an initial sample of 33 grams,how much will remain after 69 years?
A)0 grams
B)29.1351 grams
C)10.9074 grams
D)8.2500 grams
E)0.6973 grams
A)0 grams
B)29.1351 grams
C)10.9074 grams
D)8.2500 grams
E)0.6973 grams

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
49
Carbon dating presumes that,as long as a plant or animal is alive,the proportion of its carbon that is 14C is constant.The amount of 14C in an object made from harvested plants,like paper,will decline exponentially according to the equation ,where A represents the amount of 14C in the object,Ao represents the amount of 14C in living organisms,and t is the time in years since the plant was harvested.If an archeological artifact has 30% as much 14C as a living organism,how old would you predict it to be? Round to the nearest year.
A)28,040 years
B)5715 years
C)9926 years
D)14,758 years
E)100 years
A)28,040 years
B)5715 years
C)9926 years
D)14,758 years
E)100 years

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
50
A cell site is a site where electronic communications equipment is placed in a cellular network for the use of mobile phones.The numbers of cell sites from 1985 through 2008 can be modeled by where t represents the year,with corresponding to 1985.Use the model to find the numbers of cell sites in the year 2007 .
A)211,071
B)209,071
C)208,071
D)207,071
E)210,071
A)211,071
B)209,071
C)208,071
D)207,071
E)210,071

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
51
Find the magnitude R of an earthquake of intensity .
A)7.43
B)6.43
C)8.43
D)10.43
E)9.43
A)7.43
B)6.43
C)8.43
D)10.43
E)9.43

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
52
Find the magnitude R of an earthquake of intensity .
A)3.20
B)5.20
C)4.20
D)2.20
E)6.20
A)3.20
B)5.20
C)4.20
D)2.20
E)6.20

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
53
Carbon dating presumes that,as long as a plant or animal is alive,the proportion of its carbon that is 14C is constant.The amount of 14C in an object made from harvested plants,like paper,will decline exponentially according to the equation ,where A represents the amount of 14C in the object,Ao represents the amount of 14C in living organisms,and t is the time in years since the plant was harvested.If an archeological artifact has 35% as much 14C as a living organism,how old would you predict it to be? Round to the nearest year.
A)5715 years
B)29,310 years
C)93 years
D)8655 years
E)15,427 years
A)5715 years
B)29,310 years
C)93 years
D)8655 years
E)15,427 years

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
54
Find the intensity I of an earthquake measuring R on the Richter scale .
Southern Sumatra,Indonesia in 2007,
A)
B)
C)
D)
E)
Southern Sumatra,Indonesia in 2007,
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
55
The populations P (in thousands)of Pittsburgh,Pennsylvania from 2000 through 2007 can be modeled by where t represents the year,with corresponding to 2000.Use the model to find the numbers of cell sites in the year 2009.
A)2,326,853.00
B)2,327,853.00
C)2,329,853.00
D)2,328,853.00
E)2,330,853.00
A)2,326,853.00
B)2,327,853.00
C)2,329,853.00
D)2,328,853.00
E)2,330,853.00

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck
56
The chemical acidity of a solution is measured in units of pH: ,where is the hydrogen ion concentration in the solution.What is if the ?
A)
B)
C)
D)
E)6.800
A)
B)
C)
D)
E)6.800

Unlock Deck
Unlock for access to all 56 flashcards in this deck.
Unlock Deck
k this deck



