Deck 24: Trigonometric Functions The Unit Circle
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/50
Play
Full screen (f)
Deck 24: Trigonometric Functions The Unit Circle
1
Evaluate (if possible)the sine,cosine,and tangent of the real number.
A) corresponds to the point .
B) corresponds to the point .
C) corresponds to the point .
D) corresponds to the point .
E)Not possible
A) corresponds to the point .
B) corresponds to the point .
C) corresponds to the point .
D) corresponds to the point .
E)Not possible
corresponds to the point .
2
Find the point (x,y)on the unit circle that corresponds to the real number t.
A) corresponds to the point .
B) corresponds to the point .
C) corresponds to the point .
D) corresponds to the point .
E) corresponds to the point .
A) corresponds to the point .
B) corresponds to the point .
C) corresponds to the point .
D) corresponds to the point .
E) corresponds to the point .
corresponds to the point .
3
Evaluate (if possible)the sine,cosine,and tangent of the real number.
A) corresponds to the point .
B) corresponds to the point .
C) corresponds to the point .
D) corresponds to the point .
E)Not possible
A) corresponds to the point .
B) corresponds to the point .
C) corresponds to the point .
D) corresponds to the point .
E)Not possible
corresponds to the point .
4
Find the exact values of the three trigonometric functions sine,cosine,tangent of the real number t. 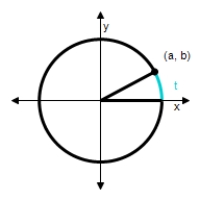 a = ,b =
a = ,b =
A)sin t = cos t =
Tan t =
B)sin t = cos t =
Tan t =
C)sin t = cos t =
Tan t =
D)sin t = cos t =
Tan t =
E)sin t = cos t =
Tan t =
 a = ,b =
a = ,b = A)sin t = cos t =
Tan t =
B)sin t = cos t =
Tan t =
C)sin t = cos t =
Tan t =
D)sin t = cos t =
Tan t =
E)sin t = cos t =
Tan t =

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
5
Find the point (x,y)on the unit circle that corresponds to the real number t.(Round your answer to one decimal place. )
A) corresponds to the point .
B) corresponds to the point .
C) corresponds to the point .
D) corresponds to the point .
E) corresponds to the point .
A) corresponds to the point .
B) corresponds to the point .
C) corresponds to the point .
D) corresponds to the point .
E) corresponds to the point .

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
6
Find the exact values of the three trigonometric functions sine,cosine,tangent of the real number t. 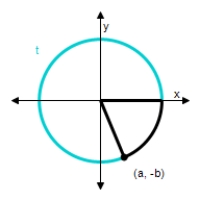
A)
B)
C)
D)
E)

A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
7
Evaluate (if possible)the sine,cosec,and tangent of the real number.
A) corresponds to the point .
B) corresponds to the point .
C) corresponds to the point .
D) corresponds to the point .
E)Not possible
A) corresponds to the point .
B) corresponds to the point .
C) corresponds to the point .
D) corresponds to the point .
E)Not possible

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
8
Evaluate (if possible)the sine,cosine,and tangent of the real number.
A) corresponds to the point .
B) corresponds to the point .
C) corresponds to the point .
D) corresponds to the point .
E)Not possible
A) corresponds to the point .
B) corresponds to the point .
C) corresponds to the point .
D) corresponds to the point .
E)Not possible

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
9
Find the point (x,y)on the unit circle that corresponds to the real number t.
A) corresponds to the point .
B) corresponds to the point .
C) corresponds to the point .
D) corresponds to the point .
E) corresponds to the point .
A) corresponds to the point .
B) corresponds to the point .
C) corresponds to the point .
D) corresponds to the point .
E) corresponds to the point .

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
10
Evaluate (if possible)the sine,cosine,and tangent of the real number.
A) corresponds to the point .
B) corresponds to the point .
C) corresponds to the point .
D) corresponds to the point .
E)Not possible
A) corresponds to the point .
B) corresponds to the point .
C) corresponds to the point .
D) corresponds to the point .
E)Not possible

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
11
Evaluate the trigonometric function using its period as an aid.
Cos 8π
A)cos 8π = -8
B)cos 8π = 0
C)cos 8π = 8
D)cos 8π = ∞
E)cos 8π = 1
Cos 8π
A)cos 8π = -8
B)cos 8π = 0
C)cos 8π = 8
D)cos 8π = ∞
E)cos 8π = 1

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
12
Evaluate the trigonometric function using its period as an aid.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
13
Find the exact values of the three trigonometric functions sine,cosine,tangent of the real number t. 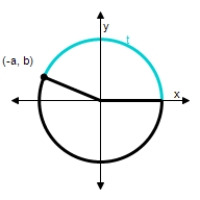
A)
B)
C)
D)
E)

A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
14
Find the exact values of the three trigonometric functions sine,cosine,tangent of the real number t. 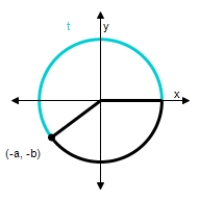
A)
B)
C)
D)
E)

A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
15
Evaluate (if possible)the sine,cosine,and tangent of the real number.
A) corresponds to the point .
B) corresponds to the point .
C) corresponds to the point .
D) corresponds to the point .
E)Not possible
A) corresponds to the point .
B) corresponds to the point .
C) corresponds to the point .
D) corresponds to the point .
E)Not possible

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
16
Evaluate the trigonometric function using its period as an aid.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
17
Find the point (x,y)on the unit circle that corresponds to the real number t.
A) corresponds to the point .
B) corresponds to the point .
C) corresponds to the point .
D) corresponds to the point .
E) corresponds to the point .
A) corresponds to the point .
B) corresponds to the point .
C) corresponds to the point .
D) corresponds to the point .
E) corresponds to the point .

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
18
Evaluate the trigonometric function using its period as an aid.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
19
Evaluate (if possible)the sine,cosine,and tangent of the real number.
A) corresponds to the point .
B) corresponds to the point .
C) corresponds to the point .
D) corresponds to the point .
E)Not possible
A) corresponds to the point .
B) corresponds to the point .
C) corresponds to the point .
D) corresponds to the point .
E)Not possible

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
20
Find the point (x,y)on the unit circle that corresponds to the real number t.
A) corresponds to the point .
B) corresponds to the point .
C) corresponds to the point .
D) corresponds to the point .
E) corresponds to the point .
A) corresponds to the point .
B) corresponds to the point .
C) corresponds to the point .
D) corresponds to the point .
E) corresponds to the point .

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
21
Find the value of given trigonometric function.Round your answer to four decimal places.
A) - 0.5000
B) 0.6000
C) 0.4000
D) 0.7000
E) 0.5000
A) - 0.5000
B) 0.6000
C) 0.4000
D) 0.7000
E) 0.5000

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
22
Use the value of the trigonometric function to find .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
23
Use the value of the trigonometric function to find .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
24
Use the value of the trigonometric function to find .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
25
Find the value of given trigonometric function.Round your answer to four decimal places.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
26
Use the value of the trigonometric function to find .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
27
Use the value of the trigonometric function to find .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
28
Evaluate the trigonometric function using its period as an aid.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
29
Find the value of given trigonometric function.Round your answer to four decimal places..
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
30
Use the value of the trigonometric function to find .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
31
Use the value of the trigonometric function to find .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
32
Use the value of the trigonometric function to find the .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
33
Find the value of given trigonometric function.Round your answer to four decimal places.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
34
Use the value of the trigonometric function to find .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
35
Use the value of the trigonometric function to find .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
36
Find the value of given trigonometric function.Round your answer to four decimal places.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
37
Find the value of given trigonometric function.Round your answer to four decimal places.
A) 0.3142
B) 0.6142
C) - 0.4142
D) 0.4142
E) 0.5142
A) 0.3142
B) 0.6142
C) - 0.4142
D) 0.4142
E) 0.5142

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
38
Use the value of the trigonometric function to find .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
39
Use the value of the trigonometric function to find .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
40
Evaluate the trigonometric function using its period as an aid.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
41
Find the value of given trigonometric function.Round your answer to four decimal places.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
42
Find the value of given trigonometric function.Round your answer to four decimal places.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
43
The displacement from equilibrium of an oscillating weight suspended by a spring and subject to the damping effect of friction is given by ,where y is the displacement (in feet)and t is the time (in seconds).Complete the following table.(Round your answer to four decimal places. )
A)
B)
C)
A)
B)
C)

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
44
Find the value of given trigonometric function.Round your answer to four decimal places.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
45
The displacement from equilibrium of an oscillating weight suspended by a spring is given by ,where y is the displacement (in feet)and t is the time (in seconds).Find the displacements when .(Round your answers to two decimal places. )
A) foot
B) foot
C) foot
D) foot
E) foot
A) foot
B) foot
C) foot
D) foot
E) foot

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
46
Find the point on the unit circle that corresponds to the real number .Use your results to evaluate .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
47
The displacement from equilibrium of an oscillating weight suspended by a spring is given by ,where y is the displacement (in feet)and t is the time (in seconds).Find the displacements when .
A) foot
B) foot
C) foot
D) foot
E) foot
A) foot
B) foot
C) foot
D) foot
E) foot

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
48
Find the value of given trigonometric function.Round your answer to four decimal places.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
49
Find the point on the unit circle that corresponds to the real number .Use your results to evaluate .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck
50
The displacement from equilibrium of an oscillating weight suspended by a spring is given by ,where y is the displacement in centimeters and t is the time in seconds.Find the displacement when ,rounding answer to four decimal places.
A)-3.7018 cm
B)-6.4350 cm
C)23.8825 cm
D)2.9298 cm
E)-1.6362 cm
A)-3.7018 cm
B)-6.4350 cm
C)23.8825 cm
D)2.9298 cm
E)-1.6362 cm

Unlock Deck
Unlock for access to all 50 flashcards in this deck.
Unlock Deck
k this deck



