Deck 9: The Time Value of Money
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/100
Play
Full screen (f)
Deck 9: The Time Value of Money
1
An amount of money to be received in the future is worth less today than the stated amount.
True
2
If a single amount were put on deposit at a given interest rate and allowed to grow, its future value could be determined by reference to the future value of $1 table.
True
3
The future value is the same concept as the way money grows in a bank account.
True
4
The interest factor for the present value of a single sum is equal to (1 + i)/i.

Unlock Deck
Unlock for access to all 100 flashcards in this deck.
Unlock Deck
k this deck
5
As the interest rate increases, the interest factor (IF) for the present value of $1 increases.

Unlock Deck
Unlock for access to all 100 flashcards in this deck.
Unlock Deck
k this deck
6
Cash flow decisions that ignore the time value of money will probably not be as accurate as those decisions that do rely on the time value of money.

Unlock Deck
Unlock for access to all 100 flashcards in this deck.
Unlock Deck
k this deck
7
The time value of money concept is fundamental to the analysis of cash inflow and outflow decisions covering periods of over one year.

Unlock Deck
Unlock for access to all 100 flashcards in this deck.
Unlock Deck
k this deck
8
The interest factor for the future value of a single sum is equal to (1 + n)i.

Unlock Deck
Unlock for access to all 100 flashcards in this deck.
Unlock Deck
k this deck
9
The interest factor for a future value (FVIF) is equal to (1 + i)n.

Unlock Deck
Unlock for access to all 100 flashcards in this deck.
Unlock Deck
k this deck
10
To determine the current worth of 4 annual payments of $1,000 at 4%, one would refer to a table for the present value of $1.

Unlock Deck
Unlock for access to all 100 flashcards in this deck.
Unlock Deck
k this deck
11
The interest factor for the present value of a single amount is the inverse of the future value interest factor.

Unlock Deck
Unlock for access to all 100 flashcards in this deck.
Unlock Deck
k this deck
12
In determining the future value of an annuity, the final payment is not compounded at all.

Unlock Deck
Unlock for access to all 100 flashcards in this deck.
Unlock Deck
k this deck
13
The time value of money is not a useful concept in determining the value of a bond or in capital investment decisions.

Unlock Deck
Unlock for access to all 100 flashcards in this deck.
Unlock Deck
k this deck
14
Compounding refers to the growth process that turns $1 today into a greater value several periods in the future.

Unlock Deck
Unlock for access to all 100 flashcards in this deck.
Unlock Deck
k this deck
15
Discounting refers to the growth process that turns $1 today into a greater value several periods in the future.

Unlock Deck
Unlock for access to all 100 flashcards in this deck.
Unlock Deck
k this deck
16
In determining the interest factor (IF) for the present value of $1, one could use the reciprocal of the IF for the future value of $1 at the same rate and time period.

Unlock Deck
Unlock for access to all 100 flashcards in this deck.
Unlock Deck
k this deck
17
The future value of an annuity assumes that the payments are received at the end of the year and that the last payment does not compound.

Unlock Deck
Unlock for access to all 100 flashcards in this deck.
Unlock Deck
k this deck
18
Higher interest rates (discount rates) reduce the present value of amounts to be received in the future.

Unlock Deck
Unlock for access to all 100 flashcards in this deck.
Unlock Deck
k this deck
19
The present value of a positive future inflow can become negative as discount rates become higher and higher.

Unlock Deck
Unlock for access to all 100 flashcards in this deck.
Unlock Deck
k this deck
20
The formula PV = FV(1 + n)i will determine the present value of $1.

Unlock Deck
Unlock for access to all 100 flashcards in this deck.
Unlock Deck
k this deck
21
Calculation of the yield of an investment provides the total return over multiple years.

Unlock Deck
Unlock for access to all 100 flashcards in this deck.
Unlock Deck
k this deck
22
Using semi-annual compounding rather than annual compounding will increase the future value of an annuity.

Unlock Deck
Unlock for access to all 100 flashcards in this deck.
Unlock Deck
k this deck
23
The time value of money concept becomes less critical as the prime rate increases.

Unlock Deck
Unlock for access to all 100 flashcards in this deck.
Unlock Deck
k this deck
24
The interest factor for the future value of an annuity is simply the sum of the interest factors for the future value using the same number of periods.

Unlock Deck
Unlock for access to all 100 flashcards in this deck.
Unlock Deck
k this deck
25
The farther into the future any given amount is received, the larger its present value.

Unlock Deck
Unlock for access to all 100 flashcards in this deck.
Unlock Deck
k this deck
26
The present value of an annuity table provides a short-cut for calculating the future value of a steady stream of payments, denoted as A. The same value can be calculated directly from the following equation:
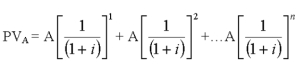
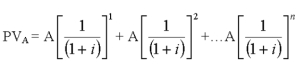

Unlock Deck
Unlock for access to all 100 flashcards in this deck.
Unlock Deck
k this deck
27
In paying off a mortgage loan, the amount of the periodic payment that goes toward the reduction of principal increases over the life of the mortgage.

Unlock Deck
Unlock for access to all 100 flashcards in this deck.
Unlock Deck
k this deck
28
Under what conditions must a distinction be made between money to be received today and money to be received in the future?
A) A period of recession.
B) When idle money can earn a positive return.
C) When there is no risk of nonpayment in the future.
D) When current interest rates are different from expected future rates.
A) A period of recession.
B) When idle money can earn a positive return.
C) When there is no risk of nonpayment in the future.
D) When current interest rates are different from expected future rates.

Unlock Deck
Unlock for access to all 100 flashcards in this deck.
Unlock Deck
k this deck
29
If an individual's cost of capital were 6%, he/she would prefer to receive $110 at the end of one year rather than $100 right now. 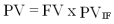
(App. B: 6%, 1 period)
= $110 x .0.943 = $104
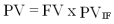
(App. B: 6%, 1 period)
= $110 x .0.943 = $104

Unlock Deck
Unlock for access to all 100 flashcards in this deck.
Unlock Deck
k this deck
30
When adjusting for semi-annual compounding of an annuity, the adjustments include multiplying the periods and annuity by 2.

Unlock Deck
Unlock for access to all 100 flashcards in this deck.
Unlock Deck
k this deck
31
In evaluating capital investment projects, current outlays must be judged against the current value of future benefits.

Unlock Deck
Unlock for access to all 100 flashcards in this deck.
Unlock Deck
k this deck
32
As the compounding rate becomes lower and lower, the future value of inflows approaches
A) 0
B) the present value of the inflows
C) infinity
D) need more information
A) 0
B) the present value of the inflows
C) infinity
D) need more information

Unlock Deck
Unlock for access to all 100 flashcards in this deck.
Unlock Deck
k this deck
33
When the inflation rate is zero, the present value of $1 is identical to the future value of $1.

Unlock Deck
Unlock for access to all 100 flashcards in this deck.
Unlock Deck
k this deck
34
The amount of annual payments necessary to accumulate a desired total can be found by reference to the present value of an annuity table.

Unlock Deck
Unlock for access to all 100 flashcards in this deck.
Unlock Deck
k this deck
35
Discounted at 6%, $1000 received three years from now is worth less than $800 received today. 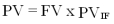
(App. B: 3 periods, 6%)
= $1,000 x .840 = $840
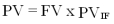
(App. B: 3 periods, 6%)
= $1,000 x .840 = $840

Unlock Deck
Unlock for access to all 100 flashcards in this deck.
Unlock Deck
k this deck
36
The amount of annual payments necessary to repay a mortgage loan can be found by reference to the present value of an annuity table.

Unlock Deck
Unlock for access to all 100 flashcards in this deck.
Unlock Deck
k this deck
37
Discounted at 10%, $1000 received at the end of each year for three years is worth less than $2,700 received today.
PVA = A PVIFA (App. D: 3 periods, 10%)
= $1,000 .2.487 = $2,487
PVA = A PVIFA (App. D: 3 periods, 10%)
= $1,000 .2.487 = $2,487

Unlock Deck
Unlock for access to all 100 flashcards in this deck.
Unlock Deck
k this deck
38
An annuity is a series of consecutive payments of equal amount.

Unlock Deck
Unlock for access to all 100 flashcards in this deck.
Unlock Deck
k this deck
39
The future value of an annuity table provides a short-cut for calculating the future value of a steady stream of payments, denoted as A. The same value can be calculated directly from the following equation:
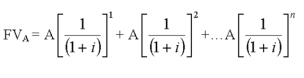
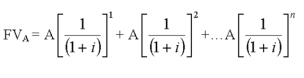

Unlock Deck
Unlock for access to all 100 flashcards in this deck.
Unlock Deck
k this deck
40
Pension fund retirement accounts use the present value of an annuity to calculate the ending value upon retirement.

Unlock Deck
Unlock for access to all 100 flashcards in this deck.
Unlock Deck
k this deck
41
As the discount rate becomes higher and higher, the present value of inflows approaches
A) 0
B) minus infinity
C) plus infinity
D) need more information
A) 0
B) minus infinity
C) plus infinity
D) need more information

Unlock Deck
Unlock for access to all 100 flashcards in this deck.
Unlock Deck
k this deck
42
To find the yield on investments which require the payment of a single amount initially, and which then return a single amount some time in the future, the correct table to use is
A) the present value of $1
B) the future value of $1
C) present value of an annuity of $1
D) (a) and (b) above.
A) the present value of $1
B) the future value of $1
C) present value of an annuity of $1
D) (a) and (b) above.

Unlock Deck
Unlock for access to all 100 flashcards in this deck.
Unlock Deck
k this deck
43
To save for her newborn son's college education, Lea Wilson will invest $1,000 at the beginning of each year for the next 18 years. The interest rate is 12 percent. What is the future value?
A) $7,690.
B) $34,931.
C) $63,440.
D) $62,440.
A) $7,690.
B) $34,931.
C) $63,440.
D) $62,440.

Unlock Deck
Unlock for access to all 100 flashcards in this deck.
Unlock Deck
k this deck
44
If you were to put $1,000 in the bank at 6% interest each year for the next ten years, which table would you use to find the ending balance in your account?
A) Present value of $1
B) Future value of $1
C) Present value of an annuity of $1
D) Future value of an annuity of $1
A) Present value of $1
B) Future value of $1
C) Present value of an annuity of $1
D) Future value of an annuity of $1

Unlock Deck
Unlock for access to all 100 flashcards in this deck.
Unlock Deck
k this deck
45
Ambrin Corp. expects to receive $2,000 per year for 10 years and $3,500 per year for the next 10 years. What is the present value of this 20 year cash flow? Use an 11% discount rate.
A) $19,034
B) $27,870
C) $32,389
D) none of these
A) $19,034
B) $27,870
C) $32,389
D) none of these

Unlock Deck
Unlock for access to all 100 flashcards in this deck.
Unlock Deck
k this deck
46
Mr. Blochirt is creating a college investment fund for his daughter. He will put in $1,000 per year for the next 15 years and expects to earn a 6% annual rate of return. How much money will his daughter have when she starts college?
A) $11,250
B) $12,263
C) $24,003
D) $23,276
A) $11,250
B) $12,263
C) $24,003
D) $23,276

Unlock Deck
Unlock for access to all 100 flashcards in this deck.
Unlock Deck
k this deck
47
As the time period until receipt increases, the present value of an amount at a fixed interest rate
A) decreases.
B) remains the same.
C) increases.
D) Not enough information to tell.
A) decreases.
B) remains the same.
C) increases.
D) Not enough information to tell.

Unlock Deck
Unlock for access to all 100 flashcards in this deck.
Unlock Deck
k this deck
48
Sharon Smith will receive $1 million in 50 years. The discount rate is 14%. As an alternative, she can receive $1,000 today. Which should she choose?
A) the $1 million dollars in 50 years.
B) $2,000 today.
C) she should be indifferent.
D) need more information.
A) the $1 million dollars in 50 years.
B) $2,000 today.
C) she should be indifferent.
D) need more information.

Unlock Deck
Unlock for access to all 100 flashcards in this deck.
Unlock Deck
k this deck
49
An annuity may be defined as
A) a payment at a fixed interest rate.
B) a series of payments of unequal amount.
C) a series of yearly payments.
D) a series of consecutive payments of equal amounts.
A) a payment at a fixed interest rate.
B) a series of payments of unequal amount.
C) a series of yearly payments.
D) a series of consecutive payments of equal amounts.

Unlock Deck
Unlock for access to all 100 flashcards in this deck.
Unlock Deck
k this deck
50
Pedro Gonzalez will invest $5,000 at the beginning of each year for the next 9 years. The interest rate is 8 percent. What is the future value?
A) $58,471.
B) $62,440.
C) $67,435.
D) $72,435.
A) $58,471.
B) $62,440.
C) $67,435.
D) $72,435.

Unlock Deck
Unlock for access to all 100 flashcards in this deck.
Unlock Deck
k this deck
51
Mr. Nailor invests $5,000 in a money market account at his local bank. He receives annual interest of 8% for 7 years. How much return will his investment earn during this time period?
A) $2,915
B) $3,570
C) $6,254
D) $8,570
A) $2,915
B) $3,570
C) $6,254
D) $8,570

Unlock Deck
Unlock for access to all 100 flashcards in this deck.
Unlock Deck
k this deck
52
You are to receive $12,000 at the end of 5 years. The available yield on investments is 6%. Which table would you use to determine the value of that sum today?
A) Present value of an annuity of $1
B) Future value of an annuity
C) Present value of $1
D) Future value of $1
A) Present value of an annuity of $1
B) Future value of an annuity
C) Present value of $1
D) Future value of $1

Unlock Deck
Unlock for access to all 100 flashcards in this deck.
Unlock Deck
k this deck
53
The concept of time value of money is important to financial decision making because
A) it emphasizes earning a return on invested capital.
B) it recognizes that earning a return makes $1 worth more today than $1 received in the future.
C) it can be applied to future cash flows in order to compare different streams of income.
D) all of these
A) it emphasizes earning a return on invested capital.
B) it recognizes that earning a return makes $1 worth more today than $1 received in the future.
C) it can be applied to future cash flows in order to compare different streams of income.
D) all of these

Unlock Deck
Unlock for access to all 100 flashcards in this deck.
Unlock Deck
k this deck
54
Ali Shah sets aside 2,000 each year for 5 years. He then withdraws the funds on an equal annual basis for the next 4 years. If Ali wishes to determine the amount of the annuity to be withdrawn each year, he should use the following two tables in this order:
A) present value of an annuity of $1; future value of an annuity of $1
B) future value of an annuity of $1; present value of an annuity of $1
C) future value of an annuity of $1; present value of a $1
D) future value of an annuity of $1; future value of a $1
A) present value of an annuity of $1; future value of an annuity of $1
B) future value of an annuity of $1; present value of an annuity of $1
C) future value of an annuity of $1; present value of a $1
D) future value of an annuity of $1; future value of a $1

Unlock Deck
Unlock for access to all 100 flashcards in this deck.
Unlock Deck
k this deck
55
If you invest $10,000 at 10% interest, how much will you have in 10 years?
A) $13,860
B) $25,940
C) $3,860
D) $80,712
A) $13,860
B) $25,940
C) $3,860
D) $80,712

Unlock Deck
Unlock for access to all 100 flashcards in this deck.
Unlock Deck
k this deck
56
As the interest rate increases, the present value of an amount to be received at the end of a fixed period
A) increases.
B) decreases.
C) remains the same.
D) Not enough information to tell.
A) increases.
B) decreases.
C) remains the same.
D) Not enough information to tell.

Unlock Deck
Unlock for access to all 100 flashcards in this deck.
Unlock Deck
k this deck
57
In determining the future value of a single amount, one measures
A) the future value of periodic payments at a given interest rate.
B) the present value of an amount discounted at a given interest rate.
C) the future value of an amount allowed to grow at a given interest rate.
D) the present value of periodic payments at a given interest rate.
A) the future value of periodic payments at a given interest rate.
B) the present value of an amount discounted at a given interest rate.
C) the future value of an amount allowed to grow at a given interest rate.
D) the present value of periodic payments at a given interest rate.

Unlock Deck
Unlock for access to all 100 flashcards in this deck.
Unlock Deck
k this deck
58
Lou Lewis borrows $10,000 to be repaid over 10 years at 9 percent. Repayment of principal in the first year is:
A) $1,558
B) $658
C) $742
D) $885
A) $1,558
B) $658
C) $742
D) $885

Unlock Deck
Unlock for access to all 100 flashcards in this deck.
Unlock Deck
k this deck
59
The IF for the future value of an annuity is 4.641 at 10% for 4 years. If we wish to accumulate $8,000 by the end of 4 years, how much should the annual payments be?
A) $2,500
B) $2,000
C) $1,724
D) none of these
A) $2,500
B) $2,000
C) $1,724
D) none of these

Unlock Deck
Unlock for access to all 100 flashcards in this deck.
Unlock Deck
k this deck
60
How much must you invest at 8% interest in order to see your investment grow to $8,000 in 10 years?
A) $3,070
B) $3,704
C) $3,105
D) none of these
A) $3,070
B) $3,704
C) $3,105
D) none of these

Unlock Deck
Unlock for access to all 100 flashcards in this deck.
Unlock Deck
k this deck
61
Mr. Fish wants to build a house in 8 years. He estimates that the total cost will be $150,000. If he can put aside $10,000 at the end of each year, what rate of return must he earn in order to have the amount needed?
A) Between 17% and 18%
B) Between 15% and 16%
C) 12%
D) None of these
A) Between 17% and 18%
B) Between 15% and 16%
C) 12%
D) None of these

Unlock Deck
Unlock for access to all 100 flashcards in this deck.
Unlock Deck
k this deck
62
Mr. Darden is selling his house for $200,000. He bought it for $164,000 ten years ago. What is the annual return on his investment?
A) 2%
B) Between 3% and 5%
C) 10%
D) None of these
A) 2%
B) Between 3% and 5%
C) 10%
D) None of these

Unlock Deck
Unlock for access to all 100 flashcards in this deck.
Unlock Deck
k this deck
63
A home buyer signed a 20-year, 8% mortgage for $72,500. Given the following information, how much should the annual loan payments be?
Present value of $1 PVIF= .215
Future value of $1 FVIF= 4.661
Present value of annuity PVIFA= 9.818
Future value of annuity FVIFA= 45.762
A) $1,584
B) $7,384
C) $15,555
D) $15,588
Present value of $1 PVIF= .215
Future value of $1 FVIF= 4.661
Present value of annuity PVIFA= 9.818
Future value of annuity FVIFA= 45.762
A) $1,584
B) $7,384
C) $15,555
D) $15,588

Unlock Deck
Unlock for access to all 100 flashcards in this deck.
Unlock Deck
k this deck
64
Increasing the number of periods will increase all of the following except
A) the present value of an annuity.
B) the present value of $1.
C) the future value of $1.
D) the future value of an annuity.
A) the present value of an annuity.
B) the present value of $1.
C) the future value of $1.
D) the future value of an annuity.

Unlock Deck
Unlock for access to all 100 flashcards in this deck.
Unlock Deck
k this deck
65
Mike Carlson will receive $12,000 a year from the end of the third year to the end of the 12thyear (10 payments). The discount rate is 10%. The present value today of this deferred annuity is:
A) $61, 450
B) $42,185
C) $55,379
D) $60,909
A) $61, 450
B) $42,185
C) $55,379
D) $60,909

Unlock Deck
Unlock for access to all 100 flashcards in this deck.
Unlock Deck
k this deck
66
Mr. Smith has just invested $10,000 for his son (age 7). The money will be used for his son's education 15 years from now. He calculates that he will need $100,000 for his son's education by the time the boy goes to school. What rate of return will Dr. Stein need to achieve this goal?
A) between 9% and 10%
B) between 16% and 17%
C) between 10% and 11%
D) between 15% and 16%
A) between 9% and 10%
B) between 16% and 17%
C) between 10% and 11%
D) between 15% and 16%

Unlock Deck
Unlock for access to all 100 flashcards in this deck.
Unlock Deck
k this deck
67
You will deposit $2,000 today. It will grow for 6 years at 10% interest compounded semiannually. You will then withdraw the funds annually over the next 4 years. The annual interest rate is 8%. Your annual withdrawal will be:
A) $2,340
B) $4,332
C) $797
D) $1,085
A) $2,340
B) $4,332
C) $797
D) $1,085

Unlock Deck
Unlock for access to all 100 flashcards in this deck.
Unlock Deck
k this deck
68
The shorter the length of time between a present value and its corresponding future value,
A) the lower the present value, relative to the future value.
B) the higher the present value, relative to the future value.
C) the higher the interest rate used in the present-valuation.
D) none of these.
A) the lower the present value, relative to the future value.
B) the higher the present value, relative to the future value.
C) the higher the interest rate used in the present-valuation.
D) none of these.

Unlock Deck
Unlock for access to all 100 flashcards in this deck.
Unlock Deck
k this deck
69
John Doeber borrowed $150,000 to buy a house. His loan cost was 6% and he promised to repay the loan in 15 equal annual payments. What is the principal outstanding after the first loan payment?
A) $143,555
B) $134,560
C) $141,200
D) None of these
A) $143,555
B) $134,560
C) $141,200
D) None of these

Unlock Deck
Unlock for access to all 100 flashcards in this deck.
Unlock Deck
k this deck
70
Dr. J. wants to buy a Dell computer which will cost $3,000 three years from today. He would like to set aside an equal amount at the end of each year in order to accumulate the amount needed. He can earn 8% annual return. How much should he set aside?
A) $879
B) $627
C) $924
D) $1,243
A) $879
B) $627
C) $924
D) $1,243

Unlock Deck
Unlock for access to all 100 flashcards in this deck.
Unlock Deck
k this deck
71
Football player Walter Johnson signs a contract calling for payments of $250,000 per year, to begin 10 years from now. To find the present value of this contract, which table or tables should you use?
A) The future value of $1
B) The future value of an annuity of $1 and the future value of $1
C) The present value of an annuity of $1 and the present value of $1
D) None of these
A) The future value of $1
B) The future value of an annuity of $1 and the future value of $1
C) The present value of an annuity of $1 and the present value of $1
D) None of these

Unlock Deck
Unlock for access to all 100 flashcards in this deck.
Unlock Deck
k this deck
72
The future value of a $500 investment today at 10 percent annual interest compounded semiannually for 5 years is
A) $805
B) $814
C) $750
D) $923
A) $805
B) $814
C) $750
D) $923

Unlock Deck
Unlock for access to all 100 flashcards in this deck.
Unlock Deck
k this deck
73
A dollar today is worth more than a dollar to be received in the future because
A) risk of nonpayment in the future.
B) the dollar can be invested today and earn interest.
C) inflation will reduce purchasing power of a future dollar.
D) None of these.
A) risk of nonpayment in the future.
B) the dollar can be invested today and earn interest.
C) inflation will reduce purchasing power of a future dollar.
D) None of these.

Unlock Deck
Unlock for access to all 100 flashcards in this deck.
Unlock Deck
k this deck
74
The higher the rate used in determining the future value of a $1 annuity,
A) the smaller the future value at the end of the period.
B) the greater the future value at the end of a period.
C) the greater the present value at the beginning of a period.
D) none of these - the interest has no effect on the future value of an annuity.
A) the smaller the future value at the end of the period.
B) the greater the future value at the end of a period.
C) the greater the present value at the beginning of a period.
D) none of these - the interest has no effect on the future value of an annuity.

Unlock Deck
Unlock for access to all 100 flashcards in this deck.
Unlock Deck
k this deck
75
John Doeber borrowed $150,000 to buy a house. His loan cost was 6% and he promised to repay the loan in 15 equal annual payments. How much are the annual payments?
A) $3,633
B) $9,250
C) $13,113
D) $15,445
A) $3,633
B) $9,250
C) $13,113
D) $15,445

Unlock Deck
Unlock for access to all 100 flashcards in this deck.
Unlock Deck
k this deck
76
A retirement plan guarantees to pay to you or your estate a fixed amount for 20 years. At the time of retirement you will have $73,425 to your credit in the plan. The plan anticipates earning 9% interest. Given the following information, how much will your annual benefits be?
Present value of $1 PVIF= .178
Future value of $1 FVIF= 5.604
Present value of annuity PVIFA= 9.129
Future value of annuity FVIFA= 51.16
A) $1,435
B) $13,070
C) $8,043
D) $13,102
Present value of $1 PVIF= .178
Future value of $1 FVIF= 5.604
Present value of annuity PVIFA= 9.129
Future value of annuity FVIFA= 51.16
A) $1,435
B) $13,070
C) $8,043
D) $13,102

Unlock Deck
Unlock for access to all 100 flashcards in this deck.
Unlock Deck
k this deck
77
Joe Nautilus has $210,000 and wants to retire. What return must his money earn so he may receive annual benefits of $30,000 for the next 10 years.
A) 12%
B) Between 12% and 13%
C) About 7%
D) Greater than 15%
A) 12%
B) Between 12% and 13%
C) About 7%
D) Greater than 15%

Unlock Deck
Unlock for access to all 100 flashcards in this deck.
Unlock Deck
k this deck
78
After 10 years, 100 shares of stock originally purchased for $500 was sold for $900. What was the yield on the investment? Choose the closest answer.
A) 19%
B) 2.5%
C) 8.5%
D) 6%
A) 19%
B) 2.5%
C) 8.5%
D) 6%

Unlock Deck
Unlock for access to all 100 flashcards in this deck.
Unlock Deck
k this deck
79
Carol Thomas will pay out $6,000 at the end of the year 2, $8,000 at the end of year 3, and receive $10,000 at the end of year 4. With an interest rate of 13 percent, what is the net value of the payments vs. receipts in today's dollars?
A) $7,326.
B) $10,242.
C) $16,372.
D) $4,112.
A) $7,326.
B) $10,242.
C) $16,372.
D) $4,112.

Unlock Deck
Unlock for access to all 100 flashcards in this deck.
Unlock Deck
k this deck
80
Babe Ruth Jr. has agreed to play for the Cleveland Indians for $3 million per year for the next 10 years. What table would you use to calculate the value of this contract in today's dollars?
A) Present value of an annuity
B) Present value of a single amount
C) Future value of an annuity
D) None of these
A) Present value of an annuity
B) Present value of a single amount
C) Future value of an annuity
D) None of these

Unlock Deck
Unlock for access to all 100 flashcards in this deck.
Unlock Deck
k this deck



