Deck 4: Exponential and Logarithmic Functions
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/96
Play
Full screen (f)
Deck 4: Exponential and Logarithmic Functions
1
Graph using a table of values and integer inputs between -3 and 3. Indicate whether the function is increasing or decreasing.
y =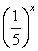
y =
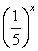
decreasing 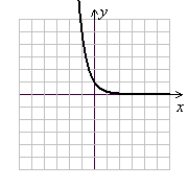 (Gridlines are spaced one unit apart.)
(Gridlines are spaced one unit apart.)
 (Gridlines are spaced one unit apart.)
(Gridlines are spaced one unit apart.) 2
Use the following to answer questions :
Use a calculator (as needed) to evaluate the function as indicated. Round values to the thousandths place if necessary.
P(t) = 1700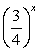
t = 8.5
Use a calculator (as needed) to evaluate the function as indicated. Round values to the thousandths place if necessary.
P(t) = 1700
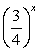
t = 8.5
0.008
3
Use the following to answer questions :
Use a calculator (as needed) to evaluate the function as indicated. Round values to the thousandths place if necessary.
P(t) = 1700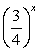
t = 7
A) 99.473
B) 103.724
C) 107.618
D) 111.831
Use a calculator (as needed) to evaluate the function as indicated. Round values to the thousandths place if necessary.
P(t) = 1700
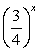
t = 7
A) 99.473
B) 103.724
C) 107.618
D) 111.831
99.473
4
Determine a likely candidate for the inverse function by reasoning and test points.
f(x) = x + 3
f(x) = x + 3

Unlock Deck
Unlock for access to all 96 flashcards in this deck.
Unlock Deck
k this deck
5
Compute 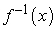 . f(x) =
. f(x) = 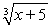
A) f -1(x) = x3 - 5
B) f -1(x) = x3 + 5
C) f -1(x) = (x + 5)3
D) f -1(x) =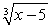
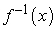 . f(x) =
. f(x) = 
A) f -1(x) = x3 - 5
B) f -1(x) = x3 + 5
C) f -1(x) = (x + 5)3
D) f -1(x) =
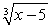

Unlock Deck
Unlock for access to all 96 flashcards in this deck.
Unlock Deck
k this deck
6
Graph using a table of values and integer inputs between -3 and 3. y = 5x
A)
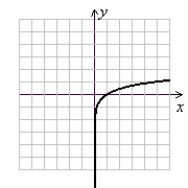
(Gridlines are spaced one unit apart.)
B)
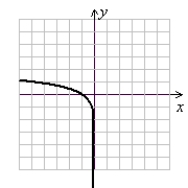
(Gridlines are spaced one unit apart.)
C)
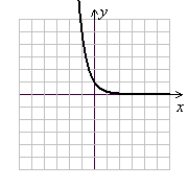
(Gridlines are spaced one unit apart.)
D)
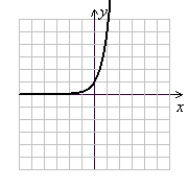
(Gridlines are spaced one unit apart.)
A)

(Gridlines are spaced one unit apart.)
B)

(Gridlines are spaced one unit apart.)
C)

(Gridlines are spaced one unit apart.)
D)

(Gridlines are spaced one unit apart.)

Unlock Deck
Unlock for access to all 96 flashcards in this deck.
Unlock Deck
k this deck
7
Determine whether the function is one-to-one. If not, state why.
{(3, 4), (1, 7), (8, 3), (-1, 4), (2, 5), (6, -1)}
{(3, 4), (1, 7), (8, 3), (-1, 4), (2, 5), (6, -1)}

Unlock Deck
Unlock for access to all 96 flashcards in this deck.
Unlock Deck
k this deck
8
Use the following to answer questions :
Use a calculator (as needed) to evaluate the function as indicated. Round values to the thousandths place if necessary.
P(t) = 5800 · 16x
t =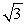
A) 548,013.289
B) 548,025.391
C) 548,036.523
D) 548,048.647
Use a calculator (as needed) to evaluate the function as indicated. Round values to the thousandths place if necessary.
P(t) = 5800 · 16x
t =
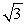
A) 548,013.289
B) 548,025.391
C) 548,036.523
D) 548,048.647

Unlock Deck
Unlock for access to all 96 flashcards in this deck.
Unlock Deck
k this deck
9
Prove (using compositions) that g(x) = f -1(x).
f(x) = -3x + 1, g(x) =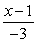
f(x) = -3x + 1, g(x) =
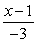

Unlock Deck
Unlock for access to all 96 flashcards in this deck.
Unlock Deck
k this deck
10
Compute 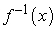 . f(x) = x3 + 8
. f(x) = x3 + 8
A)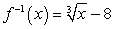 , x ≥ 0
, x ≥ 0
B)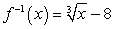
C)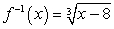 , x ≥ 8
, x ≥ 8
D)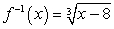
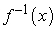 . f(x) = x3 + 8
. f(x) = x3 + 8A)
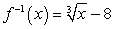 , x ≥ 0
, x ≥ 0B)
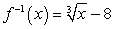
C)
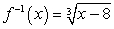 , x ≥ 8
, x ≥ 8D)
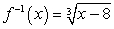

Unlock Deck
Unlock for access to all 96 flashcards in this deck.
Unlock Deck
k this deck
11
Use the following to answer questions :
Use a calculator (as needed) to evaluate the function as indicated. Round values to the thousandths place if necessary.
P(t) = 5800 · 16x
t =
Use a calculator (as needed) to evaluate the function as indicated. Round values to the thousandths place if necessary.
P(t) = 5800 · 16x
t =
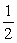

Unlock Deck
Unlock for access to all 96 flashcards in this deck.
Unlock Deck
k this deck
12
Determine whether the graph is that of a one-to-one function. If not, give an example of how the definition of one-to-oneness is violated. 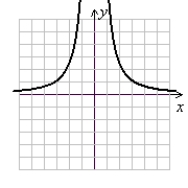 (Gridlines are spaced one unit apart.)
(Gridlines are spaced one unit apart.)
 (Gridlines are spaced one unit apart.)
(Gridlines are spaced one unit apart.)
Unlock Deck
Unlock for access to all 96 flashcards in this deck.
Unlock Deck
k this deck
13
Plot f(x) and its inverse f -1(x) on the same grid and "dash-in" the line y = x.
f(x) = 2x + 3; f -1(x) =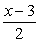
f(x) = 2x + 3; f -1(x) =
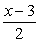

Unlock Deck
Unlock for access to all 96 flashcards in this deck.
Unlock Deck
k this deck
14
Determine whether the function is one-to-one. If not, state why.
{(-2, 5), (-4, 8), (3, 4), (-6, 7), (-3, 6), (1, 0)}
{(-2, 5), (-4, 8), (3, 4), (-6, 7), (-3, 6), (1, 0)}

Unlock Deck
Unlock for access to all 96 flashcards in this deck.
Unlock Deck
k this deck
15
Determine if the function is one-to-one by noting the functions family to which it belongs and mentally picturing the shape of its graph. f(x) = 4x - 10
A) one-to-one
B) not one-to-one
A) one-to-one
B) not one-to-one

Unlock Deck
Unlock for access to all 96 flashcards in this deck.
Unlock Deck
k this deck
16
Determine if the function is one-to-one by noting the functions family to which it belongs and mentally picturing the shape of its graph. f(x) = 8x2 + 5
A) one-to-one
B) not one-to-one
A) one-to-one
B) not one-to-one

Unlock Deck
Unlock for access to all 96 flashcards in this deck.
Unlock Deck
k this deck
17
Determine if the function is one-to-one by noting the functions family to which it belongs and mentally picturing the shape of its graph. f(x) = -|x + 7|-5
A) one-to-one
B) not one-to-one
A) one-to-one
B) not one-to-one

Unlock Deck
Unlock for access to all 96 flashcards in this deck.
Unlock Deck
k this deck
18
Find the inverse function of the one-to-one function given.
{(-1, 1), (-3, 4), (4, 0), (-5, 3), (-2, 2)}
{(-1, 1), (-3, 4), (4, 0), (-5, 3), (-2, 2)}

Unlock Deck
Unlock for access to all 96 flashcards in this deck.
Unlock Deck
k this deck
19
Plot f(x) and its inverse f -1(x) on the same grid and "dash-in" the line y = x.
f(x) = (x + 3)2, x ≥ -3; f -1(x) =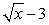
f(x) = (x + 3)2, x ≥ -3; f -1(x) =
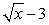

Unlock Deck
Unlock for access to all 96 flashcards in this deck.
Unlock Deck
k this deck
20
Compute 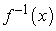 . f(x) = 2x - 6
. f(x) = 2x - 6
A)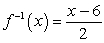
B)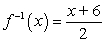
C)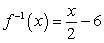
D)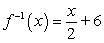
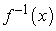 . f(x) = 2x - 6
. f(x) = 2x - 6A)
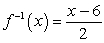
B)
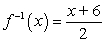
C)
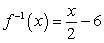
D)
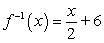

Unlock Deck
Unlock for access to all 96 flashcards in this deck.
Unlock Deck
k this deck
21
Graph by the following function by translating the basic function y = bx, sketching the asymptote, and strategically plotting a few points to round out the graph.
y =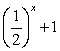
y =
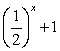

Unlock Deck
Unlock for access to all 96 flashcards in this deck.
Unlock Deck
k this deck
22
Graph by the following function by translating the basic function y = bx, sketching the asymptote, and strategically plotting a few points to round out the graph. y = 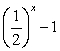
A)
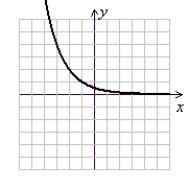
(Gridlines are spaced one unit apart.)
B)
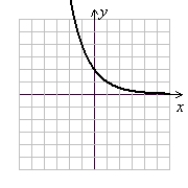
(Gridlines are spaced one unit apart.)
C)
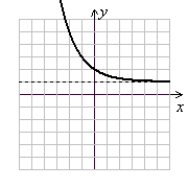
(Gridlines are spaced one unit apart.)
D)
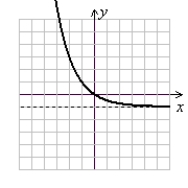
(Gridlines are spaced one unit apart.)
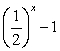
A)

(Gridlines are spaced one unit apart.)
B)

(Gridlines are spaced one unit apart.)
C)

(Gridlines are spaced one unit apart.)
D)

(Gridlines are spaced one unit apart.)

Unlock Deck
Unlock for access to all 96 flashcards in this deck.
Unlock Deck
k this deck
23
Graph by the following function by translating the basic function y = bx, sketching the asymptote, and strategically plotting a few points to round out the graph. Clearly state what shifts are applied.
y = 2x + 1 - 2
y = 2x + 1 - 2

Unlock Deck
Unlock for access to all 96 flashcards in this deck.
Unlock Deck
k this deck
24
Graph by the following function by translating the basic function y = bx, sketching the asymptote, and strategically plotting a few points to round out the graph. y = 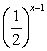
A)
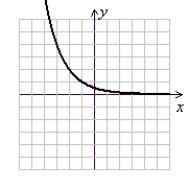
(Gridlines are spaced one unit apart.)
B)
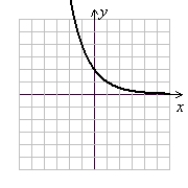
(Gridlines are spaced one unit apart.)
C)
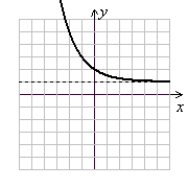
(Gridlines are spaced one unit apart.)
D)
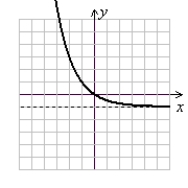
(Gridlines are spaced one unit apart.)
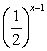
A)

(Gridlines are spaced one unit apart.)
B)

(Gridlines are spaced one unit apart.)
C)

(Gridlines are spaced one unit apart.)
D)

(Gridlines are spaced one unit apart.)

Unlock Deck
Unlock for access to all 96 flashcards in this deck.
Unlock Deck
k this deck
25
Graph by the following function by translating the basic function y = bx, sketching the asymptote, and strategically plotting a few points to round out the graph. y = 2x - 1
A)
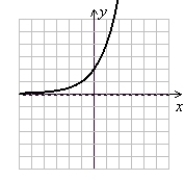
(Gridlines are spaced one unit apart.)
B)
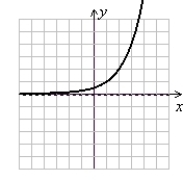
(Gridlines are spaced one unit apart.)
C)
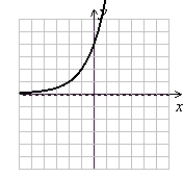
(Gridlines are spaced one unit apart.)
D)
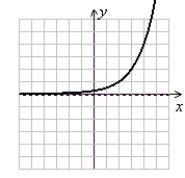
(Gridlines are spaced one unit apart.)
A)

(Gridlines are spaced one unit apart.)
B)

(Gridlines are spaced one unit apart.)
C)

(Gridlines are spaced one unit apart.)
D)

(Gridlines are spaced one unit apart.)

Unlock Deck
Unlock for access to all 96 flashcards in this deck.
Unlock Deck
k this deck
26
Write the equation in exponential form.
2 = log 5 25
2 = log 5 25

Unlock Deck
Unlock for access to all 96 flashcards in this deck.
Unlock Deck
k this deck
27
Solve the exponential equation. 27x + 2 = 3
A)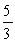
B)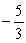
C)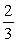
D)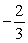
A)
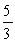
B)
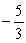
C)
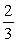
D)
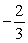

Unlock Deck
Unlock for access to all 96 flashcards in this deck.
Unlock Deck
k this deck
28
Determine the value of the expression without using a calculator. log 3 9
A)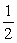
B)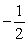
C) 2
D) -2
A)
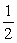
B)
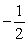
C) 2
D) -2

Unlock Deck
Unlock for access to all 96 flashcards in this deck.
Unlock Deck
k this deck
29
Graph by the following function by translating the basic function y = bx, sketching the asymptote, and strategically plotting a few points to round out the graph.
y =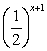
y =
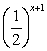

Unlock Deck
Unlock for access to all 96 flashcards in this deck.
Unlock Deck
k this deck
30
Determine the value of x by writing the equation in exponential form. log x
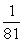 = -4
= -4
A) 3
B) -3
C)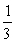
D)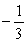
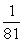 = -4
= -4A) 3
B) -3
C)
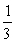
D)
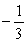

Unlock Deck
Unlock for access to all 96 flashcards in this deck.
Unlock Deck
k this deck
31
$200 is invested in an account paying 7.5% compounded annually. How much will the account be worth after 8 years? [Round to the nearest cent. Use the compound interest formula ![<strong>$200 is invested in an account paying 7.5% compounded annually. How much will the account be worth after 8 years? [Round to the nearest cent. Use the compound interest formula .]</strong> A) \approx $351.54 B) \approx $353.38 C) \approx $356.70 D) \approx $361.98](https://d2lvgg3v3hfg70.cloudfront.net/TB3307/11ea7f29_4fe0_cae6_9ecd_d502e6a1eb9a_TB3307_00.jpg) .]
.]
A) $351.54
B) $353.38
C) $356.70
D) $361.98
![<strong>$200 is invested in an account paying 7.5% compounded annually. How much will the account be worth after 8 years? [Round to the nearest cent. Use the compound interest formula .]</strong> A) \approx $351.54 B) \approx $353.38 C) \approx $356.70 D) \approx $361.98](https://d2lvgg3v3hfg70.cloudfront.net/TB3307/11ea7f29_4fe0_cae6_9ecd_d502e6a1eb9a_TB3307_00.jpg) .]
.]A) $351.54
B) $353.38
C) $356.70
D) $361.98

Unlock Deck
Unlock for access to all 96 flashcards in this deck.
Unlock Deck
k this deck
32
Determine the value without using a calculator. log 3
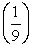
A)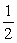
B)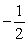
C) 2
D) -2
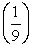
A)
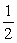
B)
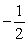
C) 2
D) -2

Unlock Deck
Unlock for access to all 96 flashcards in this deck.
Unlock Deck
k this deck
33
Solve the exponential equation.
100x - 3 = 1000x
100x - 3 = 1000x

Unlock Deck
Unlock for access to all 96 flashcards in this deck.
Unlock Deck
k this deck
34
Graph by the following function by translating the basic function y = bx, sketching the asymptote, and strategically plotting a few points to round out the graph. Clearly state what shifts are applied.
y = 4-x
y = 4-x

Unlock Deck
Unlock for access to all 96 flashcards in this deck.
Unlock Deck
k this deck
35
Graph by the following function by translating the basic function y = bx, sketching the asymptote, and strategically plotting a few points to round out the graph. Clearly state what shifts are applied.
y = 2x + 2
y = 2x + 2

Unlock Deck
Unlock for access to all 96 flashcards in this deck.
Unlock Deck
k this deck
36
Determine the value without using a calculator.
log 17 17
log 17 17

Unlock Deck
Unlock for access to all 96 flashcards in this deck.
Unlock Deck
k this deck
37
Solve the exponential equation. 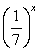 = 343
= 343
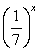 = 343
= 343
Unlock Deck
Unlock for access to all 96 flashcards in this deck.
Unlock Deck
k this deck
38
Solve the exponential equation and check your answer by substituting into the original equation.
2x = 8
2x = 8

Unlock Deck
Unlock for access to all 96 flashcards in this deck.
Unlock Deck
k this deck
39
Write the equation in logarithmic form. 24 = 16
A) 16 = log 2 4
B) 4 = log 2 16
C) 2 = log 4 16
D) 16 = log 4 2
A) 16 = log 2 4
B) 4 = log 2 16
C) 2 = log 4 16
D) 16 = log 4 2

Unlock Deck
Unlock for access to all 96 flashcards in this deck.
Unlock Deck
k this deck
40
Determine the value of x by writing the equation in exponential form.
log 2 x = 5
log 2 x = 5

Unlock Deck
Unlock for access to all 96 flashcards in this deck.
Unlock Deck
k this deck
41
Graph the exponential function. f(x) = e-x - 2 + 1
A)
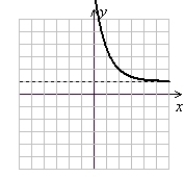
(Gridlines are spaced one unit apart.)
B)
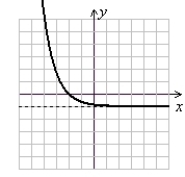
(Gridlines are spaced one unit apart.)
C)
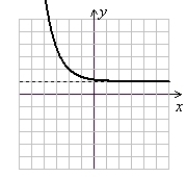
(Gridlines are spaced one unit apart.)
D)
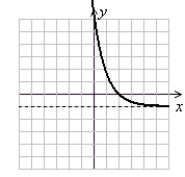
(Gridlines are spaced one unit apart.)
A)

(Gridlines are spaced one unit apart.)
B)

(Gridlines are spaced one unit apart.)
C)

(Gridlines are spaced one unit apart.)
D)

(Gridlines are spaced one unit apart.)

Unlock Deck
Unlock for access to all 96 flashcards in this deck.
Unlock Deck
k this deck
42
Solve the equation by writing it in logarithmic form. Answer in exact form. 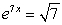
A) x =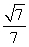
B) x =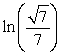
C) x =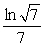
D) x =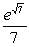
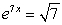
A) x =
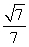
B) x =
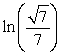
C) x =
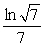
D) x =
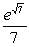

Unlock Deck
Unlock for access to all 96 flashcards in this deck.
Unlock Deck
k this deck
43
Determine the domain of the function. ![<strong>Determine the domain of the function. </strong> A) x \in (-4, 2) B) x \in [-4, 2) C) x \in (-?, -4) \cup (2, ?) D) x \in (-?, -4] \cup (2, ?)](https://d2lvgg3v3hfg70.cloudfront.net/TB3307/11ea7f29_4fe2_0374_9ecd_6d4659b78c68_TB3307_00.jpg)
A) x (-4, 2)
B) x [-4, 2)
C) x (-?, -4) (2, ?)
D) x (-?, -4] (2, ?)
![<strong>Determine the domain of the function. </strong> A) x \in (-4, 2) B) x \in [-4, 2) C) x \in (-?, -4) \cup (2, ?) D) x \in (-?, -4] \cup (2, ?)](https://d2lvgg3v3hfg70.cloudfront.net/TB3307/11ea7f29_4fe2_0374_9ecd_6d4659b78c68_TB3307_00.jpg)
A) x (-4, 2)
B) x [-4, 2)
C) x (-?, -4) (2, ?)
D) x (-?, -4] (2, ?)

Unlock Deck
Unlock for access to all 96 flashcards in this deck.
Unlock Deck
k this deck
44
Evaluate the expression using the change-of-base formula and either base 10 or base e. Answer in approximate form using 8 decimal places. log 3 27
A) 2.84767879
B) 2.98768768
C) 3.00000000
D) 3.32345613
A) 2.84767879
B) 2.98768768
C) 3.00000000
D) 3.32345613

Unlock Deck
Unlock for access to all 96 flashcards in this deck.
Unlock Deck
k this deck
45
Use a calculator to evaluate ln 401, rounded to six decimal places.
A) 4.992827
B) 5.993961
C) 7.117417
D) 8.184048
A) 4.992827
B) 5.993961
C) 7.117417
D) 8.184048

Unlock Deck
Unlock for access to all 96 flashcards in this deck.
Unlock Deck
k this deck
46
Use the following to answer questions :
The magnitude of an earthquake is given by the equation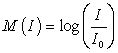 where I is the actual intensity of the earthquake and I0 is the reference intensity (the smallest earth movement that can be recorded on a seismograph).
where I is the actual intensity of the earthquake and I0 is the reference intensity (the smallest earth movement that can be recorded on a seismograph).
Find the intensity I of the earthquake given M(I) = 4.5. Round your answer to the nearest tenth.
A) ≈ 31,632.9I0
B) ≈ 31,622.8I0
C) ≈ 31,617.6I0
D) ≈ 31,610.5I0
The magnitude of an earthquake is given by the equation
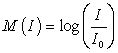 where I is the actual intensity of the earthquake and I0 is the reference intensity (the smallest earth movement that can be recorded on a seismograph).
where I is the actual intensity of the earthquake and I0 is the reference intensity (the smallest earth movement that can be recorded on a seismograph).Find the intensity I of the earthquake given M(I) = 4.5. Round your answer to the nearest tenth.
A) ≈ 31,632.9I0
B) ≈ 31,622.8I0
C) ≈ 31,617.6I0
D) ≈ 31,610.5I0

Unlock Deck
Unlock for access to all 96 flashcards in this deck.
Unlock Deck
k this deck
47
Graph the logarithmic function.
f(x) = -ln(x + 1)
f(x) = -ln(x + 1)

Unlock Deck
Unlock for access to all 96 flashcards in this deck.
Unlock Deck
k this deck
48
Solve the equation by writing it in exponential form. Answer in exact form and approximate form using a calculator (round to thousandths).
ln x = 1.587
ln x = 1.587

Unlock Deck
Unlock for access to all 96 flashcards in this deck.
Unlock Deck
k this deck
49
Use a calculator to find an approximate value, rounded to four decimal places. log 0.078
A) -1.7313
B) -1.4066
C) -1.1079
D) -0.1667
A) -1.7313
B) -1.4066
C) -1.1079
D) -0.1667

Unlock Deck
Unlock for access to all 96 flashcards in this deck.
Unlock Deck
k this deck
50
Graph the function using transformations of y = log b x and strategically plotting a few points.
f(x) = -log 3(x + 2)
f(x) = -log 3(x + 2)

Unlock Deck
Unlock for access to all 96 flashcards in this deck.
Unlock Deck
k this deck
51
Use the following to answer questions :
The magnitude of an earthquake is given by the equation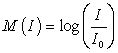 where I is the actual intensity of the earthquake and I0 is the reference intensity (the smallest earth movement that can be recorded on a seismograph).
where I is the actual intensity of the earthquake and I0 is the reference intensity (the smallest earth movement that can be recorded on a seismograph).
-Find the value of M(I) when I = 81,500I0. Round your answer to the nearest tenth.
A) 4.6
B) 4.7
C) 4.8
D) 4.9
The magnitude of an earthquake is given by the equation
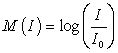 where I is the actual intensity of the earthquake and I0 is the reference intensity (the smallest earth movement that can be recorded on a seismograph).
where I is the actual intensity of the earthquake and I0 is the reference intensity (the smallest earth movement that can be recorded on a seismograph).-Find the value of M(I) when I = 81,500I0. Round your answer to the nearest tenth.
A) 4.6
B) 4.7
C) 4.8
D) 4.9

Unlock Deck
Unlock for access to all 96 flashcards in this deck.
Unlock Deck
k this deck
52
Solve the equation by writing it in exponential form. Answer in decimal form to the thousandths place. ln e6x = -32.4
A) x = 0.027
B) x = 1.686
C) x = -0.644
D) x = -5.400
A) x = 0.027
B) x = 1.686
C) x = -0.644
D) x = -5.400

Unlock Deck
Unlock for access to all 96 flashcards in this deck.
Unlock Deck
k this deck
53
Determine the domain of the function. 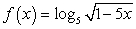

Unlock Deck
Unlock for access to all 96 flashcards in this deck.
Unlock Deck
k this deck
54
Graph the logarithmic function. f(x) = ln(x - 2) + 3
A)
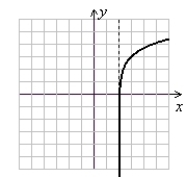
(Gridlines are spaced one unit apart.)
B)
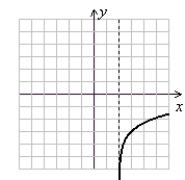
(Gridlines are spaced one unit apart.)
C)
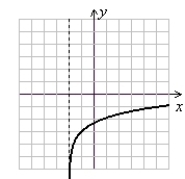
(Gridlines are spaced one unit apart.)
D)
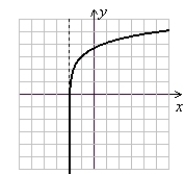
(Gridlines are spaced one unit apart.)
A)

(Gridlines are spaced one unit apart.)
B)

(Gridlines are spaced one unit apart.)
C)

(Gridlines are spaced one unit apart.)
D)

(Gridlines are spaced one unit apart.)

Unlock Deck
Unlock for access to all 96 flashcards in this deck.
Unlock Deck
k this deck
55
Graph the function using transformations of y = log b x and strategically plotting a few points.
f(x) = log 3 x + 2
f(x) = log 3 x + 2

Unlock Deck
Unlock for access to all 96 flashcards in this deck.
Unlock Deck
k this deck
56
Determine the value of x by writing the equation in exponential form. log 16 32 = x
A)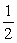
B)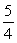
C) 2
D)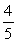
A)
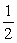
B)
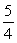
C) 2
D)
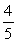

Unlock Deck
Unlock for access to all 96 flashcards in this deck.
Unlock Deck
k this deck
57
Use a calculator to find an approximate value, rounded to four decimal places. log 732
A) 2.7411
B) 2.8645
C) 3.2966
D) 3.8057
A) 2.7411
B) 2.8645
C) 3.2966
D) 3.8057

Unlock Deck
Unlock for access to all 96 flashcards in this deck.
Unlock Deck
k this deck
58
The time required for a population to triple is given by 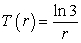 , where r represents the growth rate (expressed as a decimal) and T(r) gives the years required. How long would it take a population to triple if the growth rate were 3.9%? Round your answer to the nearest tenth.
, where r represents the growth rate (expressed as a decimal) and T(r) gives the years required. How long would it take a population to triple if the growth rate were 3.9%? Round your answer to the nearest tenth.
A). 28.2 years
B). 28.3 years
C). 28.4 years
D). 28.5 years
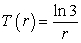 , where r represents the growth rate (expressed as a decimal) and T(r) gives the years required. How long would it take a population to triple if the growth rate were 3.9%? Round your answer to the nearest tenth.
, where r represents the growth rate (expressed as a decimal) and T(r) gives the years required. How long would it take a population to triple if the growth rate were 3.9%? Round your answer to the nearest tenth.A). 28.2 years
B). 28.3 years
C). 28.4 years
D). 28.5 years

Unlock Deck
Unlock for access to all 96 flashcards in this deck.
Unlock Deck
k this deck
59
Graph the exponential function.
f(x) = ex + 2 - 3
f(x) = ex + 2 - 3

Unlock Deck
Unlock for access to all 96 flashcards in this deck.
Unlock Deck
k this deck
60
Use a calculator to evaluate e3.4, rounded to six decimal places.
A) 26.962966
B) 27.055977
C) 28.840644
D) 29.964100
A) 26.962966
B) 27.055977
C) 28.840644
D) 29.964100

Unlock Deck
Unlock for access to all 96 flashcards in this deck.
Unlock Deck
k this deck
61
Use the properties of logarithms to write the expression as a sum or difference of simple logarithmic terms. 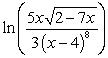
A)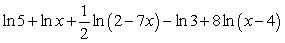
B)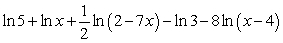
C)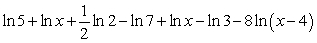
D)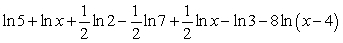
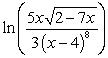
A)
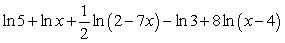
B)
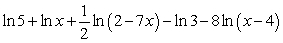
C)
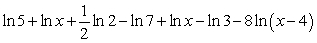
D)
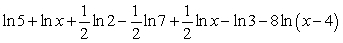

Unlock Deck
Unlock for access to all 96 flashcards in this deck.
Unlock Deck
k this deck
62
Use the properties of logarithms to write the expression as a sum or difference of simple logarithmic terms. 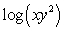
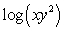

Unlock Deck
Unlock for access to all 96 flashcards in this deck.
Unlock Deck
k this deck
63
Solve using the method of your choice. Answer in approximate form rounded to four decimal places. 10x = 29
A) x 1.4624
B) x 2.9000
C) x 3.3673
D) x 0.3367
A) x 1.4624
B) x 2.9000
C) x 3.3673
D) x 0.3367

Unlock Deck
Unlock for access to all 96 flashcards in this deck.
Unlock Deck
k this deck
64
Solve using any appropriate method. Answer in exact form. ln(x + 1) + ln 8 = 6
A) x = e6 - 9
B) x = ln 6 - 9
C) x =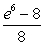
D) x =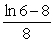
A) x = e6 - 9
B) x = ln 6 - 9
C) x =
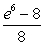
D) x =
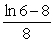

Unlock Deck
Unlock for access to all 96 flashcards in this deck.
Unlock Deck
k this deck
65
Solve using any appropriate method. Clearly identify any extraneous roots. If there are no solutions, so state. log(x + 20) - log x = log(x + 2)
A) x = -5, 4
B) x = 4, -5 is extraneous
C) x = 5, 4 is extraneous
D) No solution
A) x = -5, 4
B) x = 4, -5 is extraneous
C) x = 5, 4 is extraneous
D) No solution

Unlock Deck
Unlock for access to all 96 flashcards in this deck.
Unlock Deck
k this deck
66
Use the properties of logarithms to write the expression as a single term. ln(x + 3) - ln(x + 1)
A)![<strong>Use the properties of logarithms to write the expression as a single term. ln(x + 3) - ln(x + 1)</strong> A) B) ln(3) C) ln[(x + 3)(x + 1)] D) ln(2)](https://d2lvgg3v3hfg70.cloudfront.net/TB3307/11ea7f29_4fe4_9b9e_9ecd_4f61ea42f08f_TB3307_11.jpg)
B) ln(3)
C) ln[(x + 3)(x + 1)]
D) ln(2)
A)
![<strong>Use the properties of logarithms to write the expression as a single term. ln(x + 3) - ln(x + 1)</strong> A) B) ln(3) C) ln[(x + 3)(x + 1)] D) ln(2)](https://d2lvgg3v3hfg70.cloudfront.net/TB3307/11ea7f29_4fe4_9b9e_9ecd_4f61ea42f08f_TB3307_11.jpg)
B) ln(3)
C) ln[(x + 3)(x + 1)]
D) ln(2)

Unlock Deck
Unlock for access to all 96 flashcards in this deck.
Unlock Deck
k this deck
67
Write the equation in the simplified form bx = k (exponential term = constant). Do not solve. 4x (47x - 1) = 16
A) 46x - 1 = 16
B) 48x - 1 = 16
C)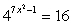
D)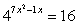
A) 46x - 1 = 16
B) 48x - 1 = 16
C)
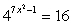
D)
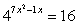

Unlock Deck
Unlock for access to all 96 flashcards in this deck.
Unlock Deck
k this deck
68
Solve using the method of your choice. Answer in exact form. 3x = 23x - 8
A) x =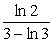
B) x =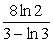
C) x =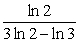
D) x =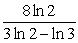
A) x =
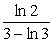
B) x =
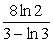
C) x =
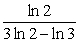
D) x =
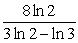

Unlock Deck
Unlock for access to all 96 flashcards in this deck.
Unlock Deck
k this deck
69
Solve using any appropriate method. Clearly identify any extraneous roots. If there are no solutions, so state. log(-x - 2) = log(3x) - log x
A) x = 0, -5
B) x = 0, 5
C) x = 5
D) No solution
A) x = 0, -5
B) x = 0, 5
C) x = 5
D) No solution

Unlock Deck
Unlock for access to all 96 flashcards in this deck.
Unlock Deck
k this deck
70
Solve the equation using the uniqueness property of logarithms.
log(10x + 10) = log 20
log(10x + 10) = log 20

Unlock Deck
Unlock for access to all 96 flashcards in this deck.
Unlock Deck
k this deck
71
Solve by converting to exponential form. log(3x - 5) = 2
A) x =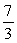
B) x =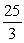
C) x = 35
D) x = 34
A) x =
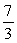
B) x =
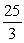
C) x = 35
D) x = 34

Unlock Deck
Unlock for access to all 96 flashcards in this deck.
Unlock Deck
k this deck
72
Write the equation in the simplified form bx = k (exponential term = constant). Do not solve.
1 - 4e0.2x = -35
1 - 4e0.2x = -35

Unlock Deck
Unlock for access to all 96 flashcards in this deck.
Unlock Deck
k this deck
73
Solve the equation in two ways: by equating bases and using the uniqueness properties and by applying a base-10 or base-e logarithm and using the power property of logarithms. 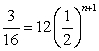
A) n = 4
B) n = 5
C) n = 6
D) n = 7
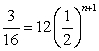
A) n = 4
B) n = 5
C) n = 6
D) n = 7

Unlock Deck
Unlock for access to all 96 flashcards in this deck.
Unlock Deck
k this deck
74
Write the equation in the simplified form log b x = k (logarithmic term = constant). Do not solve. ln(x - 3) - ln x = -ln(5x)
A)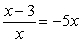
B) ln(5x3 - 15x2) = 0
C)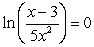
D) ln(5x - 15) = 0
A)
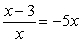
B) ln(5x3 - 15x2) = 0
C)
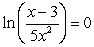
D) ln(5x - 15) = 0

Unlock Deck
Unlock for access to all 96 flashcards in this deck.
Unlock Deck
k this deck
75
Use the properties of logarithms to write the expression as a single term.
ln(x + 7) + ln(7x)
ln(x + 7) + ln(7x)

Unlock Deck
Unlock for access to all 96 flashcards in this deck.
Unlock Deck
k this deck
76
Solve using the method of your choice. Answer in exact form and approximate form rounded to four decimal places.
8x + 3 = 4602
8x + 3 = 4602

Unlock Deck
Unlock for access to all 96 flashcards in this deck.
Unlock Deck
k this deck
77
Use the power property of logarithms to rewrite the term as a product of a constant and a logarithmic term.
ln74x - 3
ln74x - 3

Unlock Deck
Unlock for access to all 96 flashcards in this deck.
Unlock Deck
k this deck
78
Write the equation in the simplified form log b x = k (logarithmic term = constant). Do not solve.
5log 3 x + log 3(x + 8) = -3
5log 3 x + log 3(x + 8) = -3

Unlock Deck
Unlock for access to all 96 flashcards in this deck.
Unlock Deck
k this deck
79
Solve the equation in two ways: by equating bases and using the uniqueness properties and by applying a base-10 or base-e logarithm and using the power property of logarithms.
3x = 27
3x = 27

Unlock Deck
Unlock for access to all 96 flashcards in this deck.
Unlock Deck
k this deck
80
Compute the average rate of change of y = ln x over the interval [2, 2.0001]. Round your answer to the nearest hundredth
A) 0.49
B) 0.50
C) 0.62
D) 0.81
A) 0.49
B) 0.50
C) 0.62
D) 0.81

Unlock Deck
Unlock for access to all 96 flashcards in this deck.
Unlock Deck
k this deck



