Deck 4: Rational, Power, and Root Functions
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/48
Play
Full screen (f)
Deck 4: Rational, Power, and Root Functions
1
(a) Sketch the graph of .
(b) Explain how the graph in part (a) is obtained from the graph of .
(c) Use a graphing calculator to obtain an accurate depiction of the graph in part (a).
(b) Explain how the graph in part (a) is obtained from the graph of .
(c) Use a graphing calculator to obtain an accurate depiction of the graph in part (a).
(a)
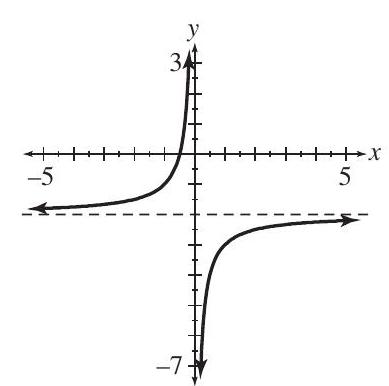
(b) The graph of is reflected across the -axis and shifted 2 units downward.
(c)
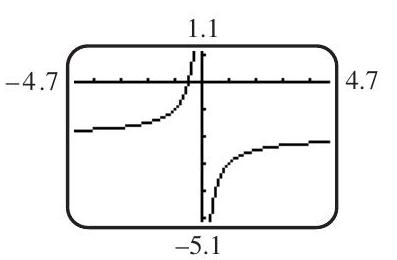

(b) The graph of is reflected across the -axis and shifted 2 units downward.
(c)

2
(a) Sketch the graph of .
(b) Explain how the graph in part (a) is obtained from the graph of .
(c) Use a graphing calculator to obtain an accurate depiction of the graph in part (a).
(b) Explain how the graph in part (a) is obtained from the graph of .
(c) Use a graphing calculator to obtain an accurate depiction of the graph in part (a).
(a)
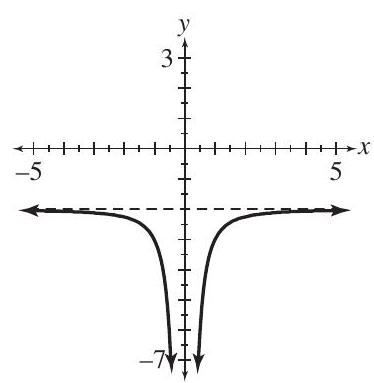
(b) The graph of is reflected across the -axis and shifted 2 units downward.
(c)
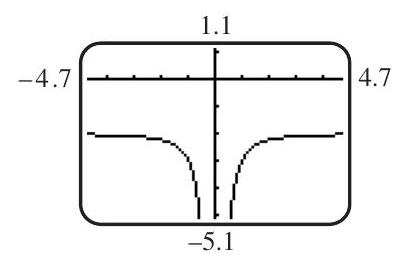

(b) The graph of is reflected across the -axis and shifted 2 units downward.
(c)

3
Consider the rational function defined by . Determine the answers to (a) - (e) analytically:
(a) Equations of the vertical asymptotes
(b) Equation of the horizontal asymptote
(c) -intercept
(d) -intercepts, if any
(e) Coordinates of the point where the graph of intersects its horizontal asymptote. Now sketch a comprehensive graph of .
(a) Equations of the vertical asymptotes
(b) Equation of the horizontal asymptote
(c) -intercept
(d) -intercepts, if any
(e) Coordinates of the point where the graph of intersects its horizontal asymptote. Now sketch a comprehensive graph of .
(a)
(b)
(c) 2
(d)
(e)
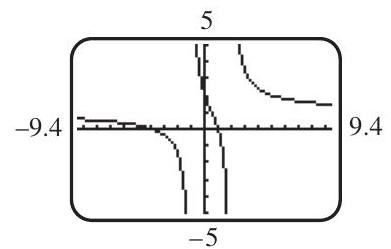
(b)
(c) 2
(d)
(e)

4
Find the equation of the oblique asymptote of the graph of the rational function defined by . Then graph the function and its asymptote using a graphing calculator to illustrate an accurate comprehensive graph.

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
5
Consider the rational function defined by .
(a) For what value of does the graph exhibit a "hole"?
(b) Graph the function and show the "hole" in the graph.
(a) For what value of does the graph exhibit a "hole"?
(b) Graph the function and show the "hole" in the graph.

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
6
(a) Solve the following rational equation analytically: .
(b) Use the results of part (a) and a graph to find the solution set of .
(b) Use the results of part (a) and a graph to find the solution set of .

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
7
The parking attendants working at the exit of a parking ramp can process at most 15 cars per minute. If cars arrive randomly at an average rate of vehicles per minute, then the average wait in minutes for a car to exit the ramp is approximated by , where .
(a) Evaluate , and . Interpret the results.
(b) Graph using the window by . Identify the vertical asymptote. What happens to as approaches 15 ?
(c) Find when the wait is 2 minutes.
(a) Evaluate , and . Interpret the results.
(b) Graph using the window by . Identify the vertical asymptote. What happens to as approaches 15 ?
(c) Find when the wait is 2 minutes.

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
8
The length and cross-sectional area of a wire determine the resistance that the wire gives to the flow of electricity. The resistance of a wire varies directly as the length of the wire and inversely as the cross-sectional area. A wire with a length of and a cross-sectional area of has a resistance of . If another wire has a length of and a cross-sectional area of , determine the resistance of the wire.

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
9
A manufacturer needs to construct a box with a lid for a special product. The only stipulations are that the volume of the box should be 1500 cubic centimeters and that the box should have a square base. The cost for producing such a box has been determined to be represented by the function , where is the cost of the box in dollars and is the length of a side of the base in centimeters. Use the graph of to determine the side length that will minimize the cost of the box, and determine what this cost will be.
(Hint: Use the window by .)
(Hint: Use the window by .)

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
10
Graph the function in the standard viewing window. Then do each of the following:
(a) Determine the domain analytically.
(b) Use the graph to find the range.
(c) Fill in the blank with either increases or decreases: The function over its entire domain.
(d) Solve the equation graphically.
(e) Solve the inequality graphically.
(a) Determine the domain analytically.
(b) Use the graph to find the range.
(c) Fill in the blank with either increases or decreases: The function over its entire domain.
(d) Solve the equation graphically.
(e) Solve the inequality graphically.

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
11
(a) Solve the equation analytically. Support the solution(s) with a graph.
(b) Use the graph to find the solution set of .
(c) Use the graph to find the solution set of .
(b) Use the graph to find the solution set of .
(c) Use the graph to find the solution set of .

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
12
A sidewalk in a public park is to be constructed from a restroom at point to the street at point , and another sidewalk is to be constructed from point to a swimming pool at point . Distances are shown in the figure provided. Find the distance which will minimize the combined length of the two sidewalks. What is the total length of the sidewalk to be constructed?
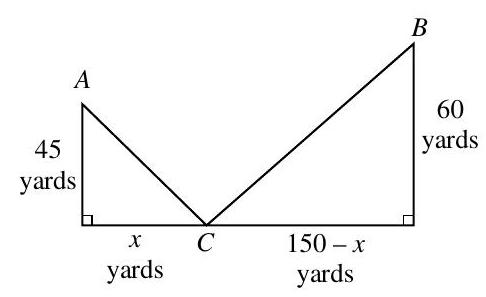


Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
13
(a) Sketch the graph of .
(b) Explain how the graph in part (a) is obtained from the graph of .
(c) Use a graphing calculator to obtain an accurate depiction of the graph in part (a).
(b) Explain how the graph in part (a) is obtained from the graph of .
(c) Use a graphing calculator to obtain an accurate depiction of the graph in part (a).

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
14
(a) Sketch the graph of
(b) Explain how the graph in part (a) is obtained from the graph of .
(c) Use a graphing calculator to obtain an accurate depiction of the graph in part (a).
(b) Explain how the graph in part (a) is obtained from the graph of .
(c) Use a graphing calculator to obtain an accurate depiction of the graph in part (a).

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
15
Consider the rational function defined by . Determine the answers to (a) - (e) analytically:
(a) Equations of the vertical asymptotes
(b) Equation of the horizontal asymptote
(c) -intercept
(d) -intercepts, if any
(e) Coordinates of the point where the graph of intersects its horizontal asymptote. Now sketch a comprehensive graph of .
(a) Equations of the vertical asymptotes
(b) Equation of the horizontal asymptote
(c) -intercept
(d) -intercepts, if any
(e) Coordinates of the point where the graph of intersects its horizontal asymptote. Now sketch a comprehensive graph of .

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
16
Find the equation of the oblique asymptote of the graph of the rational function defined by . Then graph the function and its asymptote using a graphing calculator to illustrate an accurate comprehensive graph.

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
17
Consider the rational function defined by .
(a) For what value of does the graph exhibit a "hole"?
(b) Graph the function and show the "hole" in the graph.
(a) For what value of does the graph exhibit a "hole"?
(b) Graph the function and show the "hole" in the graph.

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
18
(a) Solve the following rational equation analytically: .
(b) Use the results of part (a) and a graph to find the solution set of .
(b) Use the results of part (a) and a graph to find the solution set of .

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
19
The concession stand at a sporting event can fill at most 12 orders per minute. If people arrive randomly at an average rate of people per minute, then the average wait in minutes before an order is filled is approximated by where .
(a) Evaluate , and . Interpret the results.
(b) Graph using the window by . Identify the vertical asymptote. What happens to as approaches 12 ?
(c) Find when the wait is 4 minutes.
(a) Evaluate , and . Interpret the results.
(b) Graph using the window by . Identify the vertical asymptote. What happens to as approaches 12 ?
(c) Find when the wait is 4 minutes.

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
20
A building requires a beam 44 meters long, .32 meter wide, and .12 meter high. The maximum load of a horizontal beam that is supported at both ends varies directly as the width and the square of the height and inversely as the length between supports. If a beam of the same material 38 meter long, .25 meter wide, and .11 meter high can support a maximum load of 94.33 kilograms, what is the maximum load the beam in the building will support?

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
21
A manufacturer needs to construct a box with a lid for a special product. The only stipulations are that the volume of the box should be 3000 cubic centimeters and that the box should have a square base. The cost for producing such a box has been determined to be represented by the function , where is the cost of the box in dollars and is the length of a side of the base in centimeters. Use the graph of to determine the side length that will minimize the cost of the box, and determine what this cost will be.
(Hint: Use the window by .)
(Hint: Use the window by .)

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
22
Graph the function in the standard viewing window. Then do each of the following:
(a) Determine the domain analytically.
(b) Use the graph to find the range.
(c) Fill in the blank with either increases or decreases: The function over its entire domain.
(d) Solve the equation graphically.
(e) Solve the inequality graphically.
(a) Determine the domain analytically.
(b) Use the graph to find the range.
(c) Fill in the blank with either increases or decreases: The function over its entire domain.
(d) Solve the equation graphically.
(e) Solve the inequality graphically.

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
23
(a) Solve the equation analytically. Support the solution(s) with a graph.
(b) Use the graph to find the solution set of .
(c) Use the graph to find the solution set of .
(b) Use the graph to find the solution set of .
(c) Use the graph to find the solution set of .

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
24
Two buildings are situated on level ground, 65 feet apart, as shown in the figure. The building on the left is 20 feet high and the other building is 40 feet high. An expensive decorative banner is to be strung from the top edge of one building to the top edge of the other, pulled tightly so that it touches the ground at point somewhere between the two buildings. Let represent the distance from to the base of the building on the left. Find the value of that will minimize the length of the banner. How long is this banner?
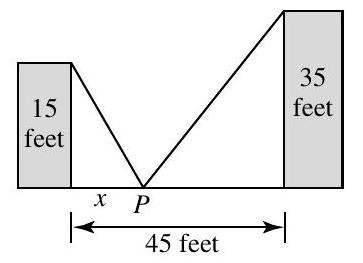


Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
25
(a) Sketch the graph of .
(b) Explain how the graph in part (a) is obtained from the graph of .
(c) Use a graphing calculator to obtain an accurate depiction of the graph in part (a).
(b) Explain how the graph in part (a) is obtained from the graph of .
(c) Use a graphing calculator to obtain an accurate depiction of the graph in part (a).

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
26
(a) Sketch the graph of .
(b) Explain how the graph in part (a) is obtained from the graph of .
(c) Use a graphing calculator to obtain an accurate depiction of the graph in part (a).
(b) Explain how the graph in part (a) is obtained from the graph of .
(c) Use a graphing calculator to obtain an accurate depiction of the graph in part (a).

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
27
Consider the rational function defined by . Determine the answers to (a) - (e) analytically:
(a) Equations of the vertical asymptotes
(b) Equation of the horizontal asymptote
(c) -intercept
(d) -intercepts, if any
(e) Coordinates of the point where the graph of intersects its horizontal asymptote. Now sketch a comprehensive graph of .
(a) Equations of the vertical asymptotes
(b) Equation of the horizontal asymptote
(c) -intercept
(d) -intercepts, if any
(e) Coordinates of the point where the graph of intersects its horizontal asymptote. Now sketch a comprehensive graph of .

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
28
Find the equation of the oblique asymptote of the graph of the rational function defined by . Then graph the function and its asymptote using a graphing calculator to illustrate an accurate comprehensive graph.

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
29
Consider the rational function defined by .
(a) For what value of does the graph exhibit a "hole"?
(b) Graph the function and show the "hole" in the graph.
(a) For what value of does the graph exhibit a "hole"?
(b) Graph the function and show the "hole" in the graph.

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
30
(a) Solve the following rational equation analytically: .
(b) Use the results of part (a) and a graph to find the solution set of .
(b) Use the results of part (a) and a graph to find the solution set of .

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
31
The parking attendants working at the exit of a parking ramp can process at most 22 cars per minute. If cars arrive randomly at an average rate of vehicles per minute, then the average wait in minutes for a car to exit the ramp is approximated by , where .
(a) Evaluate , and . Interpret the results.
(b) Graph using the window by . Identify the vertical asymptote. What happens to as approaches 22?
(c) Find when the wait is 5 minutes.
(a) Evaluate , and . Interpret the results.
(b) Graph using the window by . Identify the vertical asymptote. What happens to as approaches 22?
(c) Find when the wait is 5 minutes.

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
32
The length and cross-sectional area of a wire determine the resistance that the wire gives to the flow of electricity. The resistance of a wire varies directly as the length of the wire and inversely as the cross-sectional area. A wire with a length of and a cross-sectional area of has a resistance of . If another wire has a length of and a cross-sectional area of , determine the resistance of the wire.

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
33
A manufacturer needs to construct a box with a lid for a special product. The only stipulations are that the volume of the box should be 2515 cubic centimeters and that the box should have a square base. The cost for producing such a box has been determined to be represented by the function , where is the cost of the box in dollars and is the length of a side of the base in centimeters. Use the graph of to determine the side length that will minimize the cost of the box, and determine what this cost will be.
(Hint: Use the window by .)
(Hint: Use the window by .)

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
34
Graph the function in the standard viewing window. Then do each of the following:
(a) Determine the domain analytically.
(b) Use the graph to find the range.
(c) Fill in the blank with either increases or decreases: The function over its entire domain.
(d) Solve the equation graphically.
(e) Solve the inequality graphically.
(a) Determine the domain analytically.
(b) Use the graph to find the range.
(c) Fill in the blank with either increases or decreases: The function over its entire domain.
(d) Solve the equation graphically.
(e) Solve the inequality graphically.

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
35
(a) Solve the equation analytically. Support the solution(s) with a graph.
(b) Use the graph to find the solution set of .
(c) Use the graph to find the solution set of .
(b) Use the graph to find the solution set of .
(c) Use the graph to find the solution set of .

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
36
Two buildings are situated on level ground, 65 feet apart, as shown in the figure. The building on the left is 30 feet high and the other building is 45 feet high. An expensive decorative banner is to be strung from the top edge of one building to the top edge of the other, pulled tightly so that it touches the ground at point somewhere between the two buildings. Let represent the distance from to the base of the building on the left. Find the value of that will minimize the length of the banner. How long is this banner?
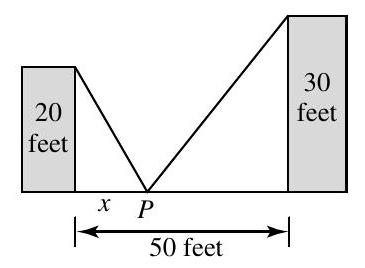


Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
37
(a) Sketch the graph of .
(b) Explain how the graph in part (a) is obtained from the graph of .
(c) Use a graphing calculator to obtain an accurate depiction of the graph in part (a).
(b) Explain how the graph in part (a) is obtained from the graph of .
(c) Use a graphing calculator to obtain an accurate depiction of the graph in part (a).

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
38
(a) Sketch the graph of .
(b) Explain how the graph in part (a) is obtained from the graph of .
(c) Use a graphing calculator to obtain an accurate depiction of the graph in part (a).
(b) Explain how the graph in part (a) is obtained from the graph of .
(c) Use a graphing calculator to obtain an accurate depiction of the graph in part (a).

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
39
Consider the rational function defined by . Determine the answers to (a) - (e) analytically:
(a) Equations of the vertical asymptotes
(b) Equation of the horizontal asymptote
(c) -intercept
(d) -intercepts, if any
(e) Coordinates of the point where the graph of intersects its horizontal asymptote. Now sketch a comprehensive graph of .
(a) Equations of the vertical asymptotes
(b) Equation of the horizontal asymptote
(c) -intercept
(d) -intercepts, if any
(e) Coordinates of the point where the graph of intersects its horizontal asymptote. Now sketch a comprehensive graph of .

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
40
Find the equation of the oblique asymptote of the graph of the rational function defined by . Then graph the function and its asymptote using a graphing calculator to illustrate an accurate comprehensive graph.

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
41
Consider the rational function defined by .
(a) For what value of does the graph exhibit a "hole"?
(b) Graph the function and show the "hole" in the graph.
(a) For what value of does the graph exhibit a "hole"?
(b) Graph the function and show the "hole" in the graph.

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
42
(a) Solve the following rational equation analytically: .
(b) Use the results of part (a) and a graph to find the solution set of .
(b) Use the results of part (a) and a graph to find the solution set of .

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
43
The concession stand at a sporting event can fill at most 9 orders per minute. If people arrive randomly at an average rate of people per minute, then the average wait in minutes before an order is filled is approximated by , where .
(a) Evaluate , and . Interpret the results.
(b) Graph using the window by . Identify the vertical asymptote. What happens to as approaches 9 ?
(c) Find when the wait is 2 minutes.
(a) Evaluate , and . Interpret the results.
(b) Graph using the window by . Identify the vertical asymptote. What happens to as approaches 9 ?
(c) Find when the wait is 2 minutes.

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
44
A building requires a beam 52 meters long, .28 meter wide, and .11 meter high. The maximum load of a horizontal beam that is supported at both ends varies directly as the width and the square of the height and inversely as the length between supports. If a beam of the same material 46 meters long, .30 meter wide, and .08 meter high can support a maximum load of 49.46 kilograms, what is the maximum load the beam in the building will support?

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
45
A manufacturer needs to construct a box with a lid for a special product. The only stipulations are that the volume of the box should be 2000 cubic centimeters and that the box should have a square base. The cost for producing such a box has been determined to be represented by the function , where is the cost of the box in dollars and is the length of a side of the base in centimeters. Use the graph of to determine the side length that will minimize the cost of the box, and determine what this cost will be.
(Hint: Use the window by .)
(Hint: Use the window by .)

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
46
Graph the function in the standard viewing window. Then do each of the following:
(a) Determine the domain analytically.
(b) Use the graph to find the range.
(c) Fill in the blank with either increases or decreases: The function over its entire domain.
(d) Solve the equation graphically.
(e) Solve the inequality graphically.
(a) Determine the domain analytically.
(b) Use the graph to find the range.
(c) Fill in the blank with either increases or decreases: The function over its entire domain.
(d) Solve the equation graphically.
(e) Solve the inequality graphically.

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
47
(a) Solve the equation analytically. Support the solution(s) with a graph.
(b) Use the graph to find the solution set of .
(c) Use the graph to find the solution set of .
(b) Use the graph to find the solution set of .
(c) Use the graph to find the solution set of .

Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck
48
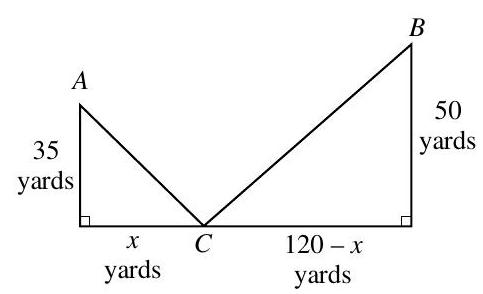
A sidewalk in a public park is to be constructed from a restroom at point to the street at point , and another sidewalk is to be constructed from point to a swimming pool at point . Distances are shown in the figure provided. Find the distance which will minimize the combined length of the two sidewalks. What is the total length of the sidewalk to be constructed?


Unlock Deck
Unlock for access to all 48 flashcards in this deck.
Unlock Deck
k this deck



