Deck 3: Polynomial Functions

Question 1

Perform each operation with complex numbers. Give answers in $a+b i$ form.
(a) $(2+6 i)-(1-3 i)$
(b) $\frac{7+4 i}{1+2 i}$
(c) Simplify $i^{39}$
(d) $-2 i(5-i)^{3}$

Answer 1

(a) $1+9 i$
(b) $3-2 i$
(c) $-i$
(d) $-148-220 i$

Question 2

For the quadratic function $P(x)=-3 x^{2}-6 x+9$ do each of the following.
(a) Find the vertex using an analytic method.
(b) Give a comprehensive graph and use a calculator to support your result in part (a).
(c) Find the zeros of $P$ and support your result using a graph for one zero and a table for the other.
(d) Find the $y$ -intercept analytically.
(e) State the domain and range of $P$ .
(f) Give the interval over which the function is increasing, and the interval over which it is decreasing.

Answer 2

(a) $(-1,12)$
(b)

(c) $-3 ; 1$
(d) 9
(e) domain: $(-\infty, \infty)$ ; range: $(-\infty, 12]$
(f) increasing: $(-\infty,-1]$ ; decreasing: $[-1, \infty)$

Question 3

(a) Solve the quadratic equation $2 x^{2}+5 x-2=0$ analytically. Give the solutions in exact form.
(b) Graph $P(x)=2 x^{2}+5 x-2$ with a calculator. Use your results in part (a) along with this graph to give the solution set of each inequality. Express endpoints of the intervals in exact form.
(i) $P(x)>0$
(ii) $P(x) \leq 0$

Answer 3

(a) $\left\{\frac{-5 \pm 1 \overline{41}}{4}\right\}$
(b)
(i) $\left(-\infty, \frac{-5-\sqrt{41}}{4}\right) \cup\left(\frac{-5+\sqrt{41}}{4}, \infty\right)$
(ii) $\left[\frac{-5-\sqrt{41}}{4}, \frac{-5+\sqrt{41}}{4}\right]$

Question 4

The width of a rectangular box is 3 times its height, and its length is 2 more than its height. Find the dimensions of the box if its volume is 288 cubic inches.

Question 5

The table gives the high school dropout rate (as a percent of enrollment) in the United States for the years 2002 to 2008. (Source: United States Census Bureau, 2008)

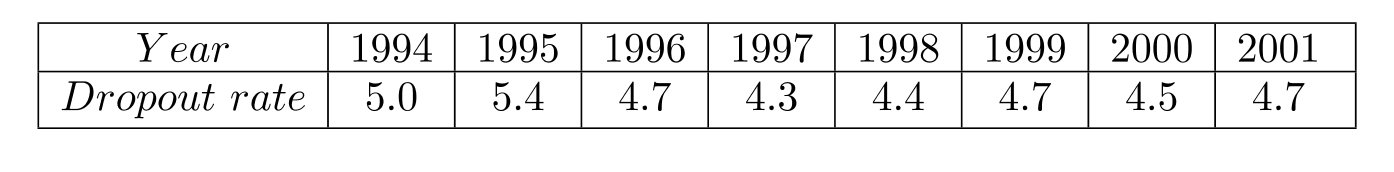
(a) Plot the data letting $x=2$ represent 2002, $x=3$ represent 2003, and so on.
(b) Find a function of the form $f(x)=a(x-h)^{2}+k$ that models this data. Let $(4,4.4)$ represent the vertex, and use (7,3.3) to determine the value of $a$ .
(c) Use the statistical capability of a graphing calculator to find the best-fitting quadratic function, $g$ , for this data. Graph both functions $g$ and $f$ from part (b) in the same window as the data points. Which function is the better fit?

Question 6

(a) Given that $f(x)=x^{6}+x^{5}-10 x^{4}+7 x^{3}-x^{2}+10 x-8$ has 1 as a zero of multiplicity 2,2 as a single zero, and -4 as a single zero, find all other zeros of $f$ .
(b) Use the information from part (a) to sketch the graph of $f$ by hand. Give an end behavior diagram.

Question 7

Perform the following for the function defined by $f(x)=4 x^{4}-45 x^{2}-49$ .
(a) Find all zeros analytically.
(b) Find a comprehensive graph of $f$ , and support the real zeros found in part (a).
(c) Discuss the symmetry of the graph of $f$ .
(d) Use the graph and the results from part (a) to find the solution set of each of the following inequalities.
(i) $f(x) \geq 0$
(ii) $f(x)<0$

Question 8

Perform the following for the function defined by $f(x)=3 x^{4}-11 x^{3}+4 x^{2}+13 x-5$ .
(a) List all possible rational zeros.
(b) Find all rational zeros.
(c) Use the intermediate value theorem to show that there must be a zero between 0 and 1.
(d) Use Descartes' rule of signs to determine the possible number of positive zeros and negative zeros.
(e) Use the boundedness theorem to show that there is no zero less than -2 and no zero greater than 4 .

Question 9

(a) Use only a graphical method to find the real solutions of $x^{5}+3 x^{4}-5 x^{3}+2 x^{2}-7 x+3=0$ .
(b) Based on your answer in part (a), how many nonreal (complex) solutions does the equation have?

Question 10

Divide.
(a) $\frac{6 x^{4}-9 x^{2}}{3 x}$
(b) $\frac{x^{3}-3 x^{2}+5 x-6}{x-1}$

Question 11

Perform each operation with complex numbers. Give answers in $a+b i$ form.
(a) $(-2+4 i)-(3-7 i)$
(b) $\frac{9-7 i}{1-3 i}$
(c) Simplify $i^{57}$
(d) $5 i(3-i)^{2}$

Question 12

For the quadratic function $P(x)=-2 x^{2}-4 x+6$ do each of the following.
(a) Find the vertex using an analytic method.
(b) Give a comprehensive graph and use a calculator to support your result in part (a).
(c) Find the zeros of $P$ and support your result using a graph for one zero and a table for the other.
(d) Find the $y$ -intercept analytically.
(e) State the domain and range of $P$
(f) Give the interval over which the function is increasing, and the interval over which it is decreasing.

Question 13

(a) Solve the quadratic equation $4 x^{2}+2 x-1=0$ analytically. Give the solutions in exact form.
(b) Graph $P(x)=4 x^{2}+2 x-1$ with a calculator. Use your results in part (a) along with this graph to give the solution set of each inequality. Express endpoints of the intervals in exact form.
(i) $P(x)>0$
(ii) $P(x) \leq 0$

Question 14

The length of a rectangular box is 2 times its height, and its width is 5 more than its height. Find the dimensions of the box if its volume is 792 cubic inches.

Question 15

The table gives the number of females who earned bachelor's degrees in the United States (in thousands) for the years 1997 to 2006. (Source: Statistical Abstract of the United States, 2006)

(a) Plot the data letting $x=7$ represent $1997, x=8$ represent 1998 , and so on.
(b) Find a function of the form $f(x)=a(x-h)^{2}+k$ that models this data. Let $(7,652)$ represent the vertex, and use $(16,855)$ to determine the value of $a$ .
(c) Use the statistical capability of a graphing calculator to find the best-fitting quadratic function, $g$ , for this data. Graph both functions $g$ and $f$ from part (b) in the same window as the data points.
Which function is the better fit?

Question 16

(a) Given that $f(x)=x^{6}-2 x^{5}-13 x^{4}+70 x^{3}-160 x^{2}+184 x-80$ has 2 as a zero of multiplicity 2,1 as a single zero, and -5 as a single zero, find all other zeros of $f$ .
(b) Use the information from part (a) to sketch the graph of $f$ by hand. Give an end behavior diagram.

Question 17

Perform the following for the function defined by $f(x)=4 x^{4}+7 x^{2}-36$ .
(a) Find all zeros analytically.
(b) Find a comprehensive graph of $f$ , and support the real zeros found in part (a).
(c) Discuss the symmetry of the graph of $f$ .
(d) Use the graph and the results from part (a) to find the solution set of each of the following inequalities.
(i) $f(x) \geq 0$
(ii) $f(x)<0$

Question 18

Perform the following for the function defined by $f(x)=3 x^{4}-8 x^{3}-6 x^{2}+17 x+6$ .
(a) List all possible rational zeros.
(b) Find all rational zeros.
(c) Use the intermediate value theorem to show that there must be a zero between -2 and -1 .
(d) Use Descartes' rule of signs to determine the possible number of positive zeros and negative zeros.
(e) Use the boundedness theorem to show that there is no zero less than -2 and no zero greater than 4 .

Question 19

(a) Use only a graphical method to find the real solutions of $x^{5}-3 x^{4}-5 x^{3}+6 x^{2}+2 x+3=0$ .
(b) Based on your answer in part (a), how many nonreal (complex) solutions does the equation have?

Question 20

Divide.
(a) $\frac{x^{3}-3 x^{2}+2}{x-1}$
(b) $\frac{x^{4}-2 x^{3}+2 x^{2}-3}{x^{2}+3}$

Question 21

Perform each operation with complex numbers. Give answers in $a+b i$ form.
(a) $(8-6 i)-(-7+3 i)$
(b) $\frac{5+14 i}{2+3 i}$
(c) Simplify $i^{76}$
(d) $2 i(3-i)^{2}$

Question 22

For the quadratic function $P(x)=-3 x^{2}+6 x+9$ do each of the following.
(a) Find the vertex using an analytic method.
(b) Give a comprehensive graph and use a calculator to support your result in part (a).
(c) Find the zeros of $P$ and support your result using a graph for one zero and a table for the other.
(d) Find the $y$ -intercept analytically.
(e) State the domain and range of $P$ .
(f) Give the interval over which the function is increasing, and the interval over which it is decreasing.

Question 23

(a) Solve the quadratic equation $3 x^{2}+4 x-3=0$ analytically. Give the solutions in exact form.
(b) Graph $P(x)=3 x^{2}+4 x-3$ with a calculator. Use your results in part (a) along with this graph to give the solution set of each inequality. Express endpoints of the intervals in exact form.
(i) $P(x)>0$
(ii) $P(x) \leq 0$

Question 24

The length of a rectangular box is 10 times its width, and its height is 7 more than its width. Find the dimensions of the box if its volume is 360 cubic inches.

Question 25

The table gives the number of females who earned doctoral degrees in the United States (in thousands) for the years 1997 to 2006. (Source: Statistical Abstract of the United States, 2006)

(a) Plot the data letting $x=7$ represent 1997, $x=8$ represent 1998, and so on.
(b) Find a function of the form $f(x)=a(x-h)^{2}+k$ that models this data. Let $(7,19)$ represent the vertex, and use $(16,27)$ to determine the value of $a$ .
(c) Use the statistical capability of a graphing calculator to find the best-fitting quadratic function, $g$ , for this data. Graph both functions $g$ and $f$ from part (b) in the same window as the data points.
Which function is the better fit?

Question 26

(a) Given that $f(x)=x^{6}-9 x^{5}+28 x^{4}-34 x^{3}+40 x-32$ has 2 as a zero of multiplicity 2,4 as a single zero, and -1 as a single zero, find all other zeros of $f$ .
(b) Use the information from part (a) to sketch the graph of $f$ by hand. Give an end behavior diagram.

Question 27

Perform the following for the function defined by $f(x)=4 x^{4}-5 x^{2}-9$ .
(a) Find all zeros analytically.
(b) Find a comprehensive graph of $f$ , and support the real zeros found in part (a).
(c) Discuss the symmetry of the graph of $f$ .
(d) Use the graph and the results from part (a) to find the solution set of each of the following inequalities.
(i) $f(x) \geq 0$
(ii) $f(x)<0$

Question 28

Perform the following for the function defined by $f(x)=2 x^{4}+x^{3}-13 x^{2}-5 x+15$ .
(a) List all possible rational zeros.
(b) Find all rational zeros.
(c) Use the intermediate value theorem to show that there must be a zero between 2 and 3.
(d) Use Descartes' rule of signs to determine the possible number of positive zeros and negative zeros.
(e) Use the boundedness theorem to show that there is no zero less than -3 and no zero greater than 3 .

Question 29

(a) Use only a graphical method to find the real solutions of $x^{5}-9 x^{4}+4 x^{3}-3 x^{2}+x+2=0$ .
(b) Based on your answer in part (a), how many nonreal (complex) solutions does the equation have?

Question 30

Divide.
(a) $\frac{x^{3}+3 x^{2}-2 x+2}{x+2}$
(b) $\frac{x^{4}-x^{3}+4 x^{2}-3 x+3}{x^{2}+3}$

Question 31

Perform each operation with complex numbers. Give answers in $a+b i$ form.
(a) $(-3-7 i)-(-2-3 i)$
(b) $\frac{10+11 i}{1-4 i}$
(c) Simplify $i^{106}$
(d) $6 i(5-i)^{2}$

Question 32

For the quadratic function $P(x)=-2 x^{2}+4 x+6$ do each of the following.
(a) Find the vertex using an analytic method.
(b) Give a comprehensive graph and use a calculator to support your result in part (a).
(c) Find the zeros of $P$ and support your result using a graph for one zero and a table for the other.
(d) Find the $y$ -intercept analytically.
(e) State the domain and range of $P$ .
(f) Give the interval over which the function is increasing, and the interval over which it is decreasing.

Question 33

(a) Solve the quadratic equation $2 x^{2}+5 x-1=0$ analytically. Give the solutions in exact form.
(b) Graph $P(x)=2 x^{2}+5 x-1$ with a calculator. Use your results in part (a) along with this graph to give the solution set of each inequality. Express endpoints of the intervals in exact form.
(i) $P(x)<0$
(ii) $P(x) \geq 0$

Question 34

The width of a rectangular box is 4 times its height, and its length is 10 more than its height. Find the dimensions of the box if its volume is 192 cubic inches.

Question 35

The table gives the average price (in dollars) for a gallon of regular unleaded gasoline as measured in September of the years 2003 to 2009. (Source: The Bureau of Labor Statistics, 2009)

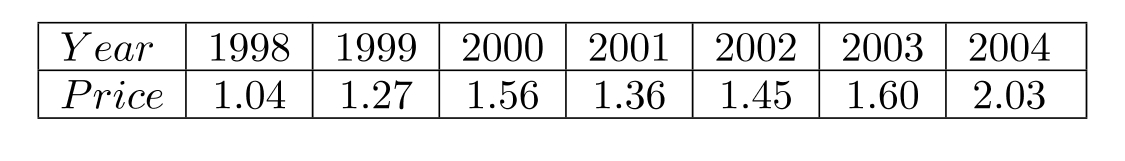
(a) Plot the data letting $x=3$ represent 2003, $x=4$ represent 2004, and so on.
(b) Find a function of the form $f(x)=a(x-h)^{2}+k$ that models this data. Let $(8,3.70)$ represent the vertex, and use $(3,1.73)$ to determine the value of $a$ .
(c) Use the statistical capability of a graphing calculator to find the best-fitting quadratic function, $g$ , for this data. Graph both functions $g$ and $f$ from part (b) in the same window as the data points. Which function is the better fit?

Question 36

(a) Given that $f(x)=x^{6}-5 x^{5}+3 x^{4}+23 x^{3}-112 x^{2}+162 x-72$ has 1 as a zero of multiplicity $2,-3$ as a single zero, and 4 as a single zero, find all other zeros of $f$ .
(b) Use the information from part (a) to sketch the graph of $f$ by hand. Give an end behavior diagram.

Question 37

Perform the following for the function defined by $f(x)=4 x^{4}+15 x^{2}-4$ .
(a) Find all zeros analytically.
(b) Find a comprehensive graph of $f$ , and support the real zeros found in part (a).
(c) Discuss the symmetry of the graph of $f$ .
(d) Use the graph and the results from part (a) to find the solution set of each of the following inequalities.
(i) $f(x) \geq 0$
(ii) $f(x)<0$

Question 38

Perform the following for the function defined by $f(x)=2 x^{4}+11 x^{3}+11 x^{2}-18 x-20$ .
(a) List all possible rational zeros.
(b) Find all rational zeros.
(c) Use the intermediate value theorem to show that there must be a zero between 1 and 2.
(d) Use Descartes' rule of signs to determine the possible number of positive zeros and negative zeros.
(e) Use the boundedness theorem to show that there is no zero less than -6 and no zero greater than 2 .

Question 39

(a) Use only a graphical method to find the real solutions of $x^{5}-2 x^{4}+2 x^{3}-5 x^{2}+3 x-3=0$ .
(b) Based on your answer in part (a), how many nonreal (complex) solutions does the equation have?

Question 40

Divide.
(a) $\frac{x^{3}+2 x+3}{x-2}$
(b) $\frac{2 x^{4}+2 x^{3}-3 x^{2}+3 x-9}{2 x^{2}+3}$