Deck 11: Sequences, Series, and the Binomial Theorem
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/98
Play
Full screen (f)
Deck 11: Sequences, Series, and the Binomial Theorem
1
Find and for .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)
2
Write the first five terms of the sequence . Assume that n begins with 1.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)
3
Write an expression for the n th term of the sequence Assume that n begins with 1.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)
4
Write the first five terms of the sequence . Assume that n begins with 1.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 98 flashcards in this deck.
Unlock Deck
k this deck
5
Write the first five terms of the sequence . Assume that n begins with 1.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 98 flashcards in this deck.
Unlock Deck
k this deck
6
Write an expression for the n th term of the sequence Assume that n begins with 1.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 98 flashcards in this deck.
Unlock Deck
k this deck
7
Simplify the expression .
A)
B)
C)
D) 990
E) 720
A)
B)
C)
D) 990
E) 720

Unlock Deck
Unlock for access to all 98 flashcards in this deck.
Unlock Deck
k this deck
8
Write an expression for the n th term of the sequence Assume that n begins with 1.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 98 flashcards in this deck.
Unlock Deck
k this deck
9
Write the first five terms of the sequence . Assume that n begins with 1.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 98 flashcards in this deck.
Unlock Deck
k this deck
10
Write an expression for the n th term of the sequence Assume that n begins with 1.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 98 flashcards in this deck.
Unlock Deck
k this deck
11
Write an expression for the n th term of the sequence Assume that n begins with 1.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 98 flashcards in this deck.
Unlock Deck
k this deck
12
Write the first five terms of the sequence . Assume that n begins with 1.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 98 flashcards in this deck.
Unlock Deck
k this deck
13
Write an expression for the n th term of the sequence Assume that n begins with 1.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 98 flashcards in this deck.
Unlock Deck
k this deck
14
Find the partial sum .
A) 104
B) 140
C) 102
D) 142
E) 120
A) 104
B) 140
C) 102
D) 142
E) 120

Unlock Deck
Unlock for access to all 98 flashcards in this deck.
Unlock Deck
k this deck
15
Find of the sequence .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 98 flashcards in this deck.
Unlock Deck
k this deck
16
Write the first five terms of the sequence . Assume that n begins with 1.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 98 flashcards in this deck.
Unlock Deck
k this deck
17
Write an expression for the n th term of the sequence Assume that n begins with 1.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 98 flashcards in this deck.
Unlock Deck
k this deck
18
Match the sequence with the graph of its first 10 terms.
A)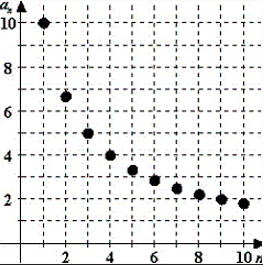
B)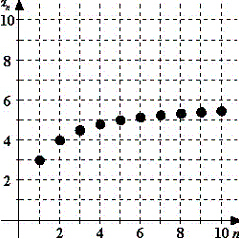
C)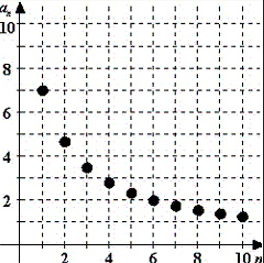
D)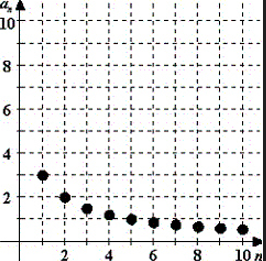
E)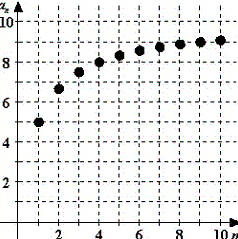
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 98 flashcards in this deck.
Unlock Deck
k this deck
19
Write the first five terms of the sequence . Assume that n begins with 1.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 98 flashcards in this deck.
Unlock Deck
k this deck
20
Write the first five terms of the sequence . Assume that n begins with 1.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 98 flashcards in this deck.
Unlock Deck
k this deck
21
Find a formula for the n th term of the arithmetic sequence.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 98 flashcards in this deck.
Unlock Deck
k this deck
22
Find the common difference of the arithmetic sequence
A) 22
B) 11
C) -22
D) 34
E) -11
A) 22
B) 11
C) -22
D) 34
E) -11

Unlock Deck
Unlock for access to all 98 flashcards in this deck.
Unlock Deck
k this deck
23
Write the sum using sigma notation. Begin with .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 98 flashcards in this deck.
Unlock Deck
k this deck
24
Write the sum using sigma notation. Begin with .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 98 flashcards in this deck.
Unlock Deck
k this deck
25
Find the partial sum of .
A) 930
B) 705
C) 255
D) 240
E) 2280
A) 930
B) 705
C) 255
D) 240
E) 2280

Unlock Deck
Unlock for access to all 98 flashcards in this deck.
Unlock Deck
k this deck
26
Find a formula for the n th term of the arithmetic sequence.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 98 flashcards in this deck.
Unlock Deck
k this deck
27
Find a formula for the n th term of the arithmetic sequence.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 98 flashcards in this deck.
Unlock Deck
k this deck
28
Write the first five terms of the arithmetic sequence defined recursively.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 98 flashcards in this deck.
Unlock Deck
k this deck
29
Find a formula for the n th term of the arithmetic sequence.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 98 flashcards in this deck.
Unlock Deck
k this deck
30
Find the partial sum
A)
B)
C)
D)
E) .
A)
B)
C)
D)
E) .

Unlock Deck
Unlock for access to all 98 flashcards in this deck.
Unlock Deck
k this deck
31
Determine whether the sequence is arithmetic. If so, find the common difference.
A)arithmetic; 14
B)arithmetic; 2
C)arithmetic;7
D)arithmetic; 28
E)not arithmetic
A)arithmetic; 14
B)arithmetic; 2
C)arithmetic;7
D)arithmetic; 28
E)not arithmetic

Unlock Deck
Unlock for access to all 98 flashcards in this deck.
Unlock Deck
k this deck
32
Write the sum using sigma notation. Begin with .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 98 flashcards in this deck.
Unlock Deck
k this deck
33
Determine whether the sequence is arithmetic. If so, find the common difference.
A)
B)
C) 2
5
D)
E)The sequence is not arithmetic.
A)
B)
C) 2
5
D)
E)The sequence is not arithmetic.

Unlock Deck
Unlock for access to all 98 flashcards in this deck.
Unlock Deck
k this deck
34
Find the partial sum .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 98 flashcards in this deck.
Unlock Deck
k this deck
35
Find a formula for the n th term of the arithmetic sequence.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 98 flashcards in this deck.
Unlock Deck
k this deck
36
A deposit of is made in an account that earns interest compounded yearly. The balance in the account after N years is given by , Find the balance in this account after years by computing . Round your answer to the nearest cent.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 98 flashcards in this deck.
Unlock Deck
k this deck
37
Write the first five terms of the arithmetic sequence defined recursively.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 98 flashcards in this deck.
Unlock Deck
k this deck
38
Find the common difference of the arithmetic sequence with the n th term .
A) -5
B) -6
C) 6
D) -1
E) 5
A) -5
B) -6
C) 6
D) -1
E) 5

Unlock Deck
Unlock for access to all 98 flashcards in this deck.
Unlock Deck
k this deck
39
Find the common difference of the arithmetic sequence
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 98 flashcards in this deck.
Unlock Deck
k this deck
40
Determine whether the sequence is arithmetic. If so, find the common difference.
A) 8
B) 4
C)
D)
E)The sequence is not arithmetic.
A) 8
B) 4
C)
D)
E)The sequence is not arithmetic.

Unlock Deck
Unlock for access to all 98 flashcards in this deck.
Unlock Deck
k this deck
41
Find the common ratio of the geometric sequence.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 98 flashcards in this deck.
Unlock Deck
k this deck
42
Find the common ratio of the geometric sequence.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 98 flashcards in this deck.
Unlock Deck
k this deck
43
Write the first five terms of the geometric sequence, given and .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 98 flashcards in this deck.
Unlock Deck
k this deck
44
Find a formula for the n th term of the geometric sequence. Assume that n begins with 1.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 98 flashcards in this deck.
Unlock Deck
k this deck
45
Find the sum of the first 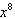 positive integers.
positive integers.
A)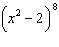
B)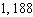
C)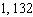
D)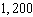
E)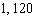
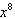 positive integers.
positive integers.A)
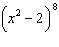
B)
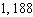
C)
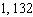
D)
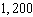
E)
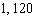

Unlock Deck
Unlock for access to all 98 flashcards in this deck.
Unlock Deck
k this deck
46
Find the sum of the first 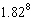 positive even integers.
positive even integers.
A)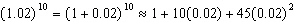
B)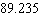
C)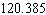
D)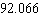
E)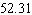
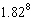 positive even integers.
positive even integers.A)
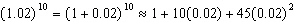
B)
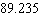
C)
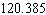
D)
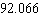
E)
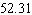

Unlock Deck
Unlock for access to all 98 flashcards in this deck.
Unlock Deck
k this deck
47
A jogger decides to take a jog of miles. The first two miles take the jogger minutes and minutes respectively to complete each mile. The jogger estimates that this pattern will continue for miles. Estimate the time required to jog miles.
A) minutes
B) minutes
C) minutes
D) minutes
E) minutes
A) minutes
B) minutes
C) minutes
D) minutes
E) minutes

Unlock Deck
Unlock for access to all 98 flashcards in this deck.
Unlock Deck
k this deck
48
Determine whether the sequence is geometric. If so, find the common ratio.
A)9
B)15
C)
D)6
E)not geometric
A)9
B)15
C)
D)6
E)not geometric

Unlock Deck
Unlock for access to all 98 flashcards in this deck.
Unlock Deck
k this deck
49
Determine whether the sequence is geometric. If so, find the common ratio.
A)-4
B)4
C)
D)
E)not geometric
A)-4
B)4
C)
D)
E)not geometric

Unlock Deck
Unlock for access to all 98 flashcards in this deck.
Unlock Deck
k this deck
50
Find the common ratio of the geometric sequence.
A) 1
B)-11
C) -1
D)
E)11
A) 1
B)-11
C) -1
D)
E)11

Unlock Deck
Unlock for access to all 98 flashcards in this deck.
Unlock Deck
k this deck
51
Find a formula for the n th term of the geometric sequence. Assume that n begins with 1.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 98 flashcards in this deck.
Unlock Deck
k this deck
52
Find the n th partial sum of the arithmetic sequence.
A) 390
B) 438
C) 426
D) 354
E) 402
A) 390
B) 438
C) 426
D) 354
E) 402

Unlock Deck
Unlock for access to all 98 flashcards in this deck.
Unlock Deck
k this deck
53
Find the partial sum of .
A) -13.75
B) 0.25
C) 2.5
D) 1.5
E) 3
A) -13.75
B) 0.25
C) 2.5
D) 1.5
E) 3

Unlock Deck
Unlock for access to all 98 flashcards in this deck.
Unlock Deck
k this deck
54
Find the n th partial sum of the arithmetic sequence.
A) 440
B) 790
C) 405
D) 395
E) 880
A) 440
B) 790
C) 405
D) 395
E) 880

Unlock Deck
Unlock for access to all 98 flashcards in this deck.
Unlock Deck
k this deck
55
Determine whether the sequence is geometric. If so, find the common ratio.
A)
B) -
C)
D) -
E)not geometric
A)
B) -
C)
D) -
E)not geometric

Unlock Deck
Unlock for access to all 98 flashcards in this deck.
Unlock Deck
k this deck
56
A museum only allows patrons to enter at fifteen minute intervals. An observer notices that every weekday at 2:00 there are people waiting at the entrance and at 3:00 there are people waiting. In addition, there are people waiting at the entrance at all 15-minute intervals that are not on the hour. This pattern continues through 6:00. How many people wait to enter the museum in the interval from 2:00 to 6:00?
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 98 flashcards in this deck.
Unlock Deck
k this deck
57
In section 112 of Hammer Stadium there are rows of seats: in the first row, seats in the second row, seats in the third row, and so on. What is the cost of each ticket if the total cost of all the seats in section 112 is ? Round the answer to two decimal places.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 98 flashcards in this deck.
Unlock Deck
k this deck
58
Find the n th partial sum of the arithmetic sequence.
A) 52.65
B) 92.30
C) 105.30
D) 46.15
E) 49.40
A) 52.65
B) 92.30
C) 105.30
D) 46.15
E) 49.40

Unlock Deck
Unlock for access to all 98 flashcards in this deck.
Unlock Deck
k this deck
59
In your new job as an accountant you are told that your starting salary will be with an increase of at the end of each of the first years. How much will you be paid through the end of your first 5 years of employment with the company?
A)$
B)$
C)$
D)$
E)$
A)$
B)$
C)$
D)$
E)$

Unlock Deck
Unlock for access to all 98 flashcards in this deck.
Unlock Deck
k this deck
60
Find the n th partial sum of the arithmetic sequence.
A) 1926
B) 2304
C) -198
D) 958.5
E) 1152
A) 1926
B) 2304
C) -198
D) 958.5
E) 1152

Unlock Deck
Unlock for access to all 98 flashcards in this deck.
Unlock Deck
k this deck
61
Find the n th partial sum of the geometric sequence.
A)1,820
B)5,465
C)200
D)5,514
E)5,808
A)1,820
B)5,465
C)200
D)5,514
E)5,808

Unlock Deck
Unlock for access to all 98 flashcards in this deck.
Unlock Deck
k this deck
62
Find the balance of an increasing annuity in which a principal of $300 is invested each month for 33 years, compounded monthly at a rate of 8%.
A)$42,454.33
B)$583,960.90
C)$63,895.28
D)$123,835.77
E)$11,406.08
A)$42,454.33
B)$583,960.90
C)$63,895.28
D)$123,835.77
E)$11,406.08

Unlock Deck
Unlock for access to all 98 flashcards in this deck.
Unlock Deck
k this deck
63
Find the partial sum.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 98 flashcards in this deck.
Unlock Deck
k this deck
64
Evaluate the binomial coefficient .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 98 flashcards in this deck.
Unlock Deck
k this deck
65
Find the partial sum.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 98 flashcards in this deck.
Unlock Deck
k this deck
66
A ball is dropped from a height of 10 feet. Each time it drops h feet, it rebounds 0.79 h feet. Find the total distance traveled by the ball. Round your answer to two decimal places.
A)75.24
B)37.62
C)47.62
D)85.24
E)22.66
A)75.24
B)37.62
C)47.62
D)85.24
E)22.66

Unlock Deck
Unlock for access to all 98 flashcards in this deck.
Unlock Deck
k this deck
67
Find the n th partial sum of the geometric sequence. Round your answer to 2 decimal places.
A)330.57
B)173.76
C)752.71
D)232.82
E)296.70
A)330.57
B)173.76
C)752.71
D)232.82
E)296.70

Unlock Deck
Unlock for access to all 98 flashcards in this deck.
Unlock Deck
k this deck
68
Find a formula for the n th term of the geometric sequence. Assume that n begins with 1.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 98 flashcards in this deck.
Unlock Deck
k this deck
69
You accept a job as a manager that pays a salary of $34,000 the first year. During the next 39 years, you receive a 4% raise each year. What would your total salary be over the 40 year period? Round answer to the nearest cent.
A)$3,740,420.98
B)$3,230,867.53
C)$3,394,102.24
D)$2,922,991.43
E)$2,638,342.67
A)$3,740,420.98
B)$3,230,867.53
C)$3,394,102.24
D)$2,922,991.43
E)$2,638,342.67

Unlock Deck
Unlock for access to all 98 flashcards in this deck.
Unlock Deck
k this deck
70
Find the sum.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 98 flashcards in this deck.
Unlock Deck
k this deck
71
A company buys a machine for $400,000. During the next 8 years, the machine depreciates at the rate of 25% per year. That is, at the end of each year, the depreciated value is 75% of what it was at the beginning of the year. Find the depreciated value of the machine at the end of the 8 full years. Round answer to the nearest cent.
A)24.41
B)30,033.87
C)6.10
D)53,393.55
E)40,045.17
A)24.41
B)30,033.87
C)6.10
D)53,393.55
E)40,045.17

Unlock Deck
Unlock for access to all 98 flashcards in this deck.
Unlock Deck
k this deck
72
Find the balance of an increasing annuity in which a principal of $20 is invested each month for 40 years, compounded monthly at a rate of 8%.
A)$939.44
B)$11,878.94
C)$5,744.14
D)$70,305.62
E)$3,703.31
A)$939.44
B)$11,878.94
C)$5,744.14
D)$70,305.62
E)$3,703.31

Unlock Deck
Unlock for access to all 98 flashcards in this deck.
Unlock Deck
k this deck
73
Find the n th partial sum of the geometric sequence.
A)3326
B)3277
C)-819
D)3620
E)-51
A)3326
B)3277
C)-819
D)3620
E)-51

Unlock Deck
Unlock for access to all 98 flashcards in this deck.
Unlock Deck
k this deck
74
Find the n th partial sum of the geometric sequence.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 98 flashcards in this deck.
Unlock Deck
k this deck
75
A city of 700,000 people is growing at the rate of 2% per year. That is, at the end of each year, the population is 1.02 times the population at the beginning of the year. Estimate the population years 19 from now. Round to the nearest integer.
A)1,243,091
B)1,019,768
C)1,103,829
D)1,082,186
E)1,194,821
A)1,243,091
B)1,019,768
C)1,103,829
D)1,082,186
E)1,194,821

Unlock Deck
Unlock for access to all 98 flashcards in this deck.
Unlock Deck
k this deck
76
Find the sum.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 98 flashcards in this deck.
Unlock Deck
k this deck
77
Evaluate the binomial coefficient .
A)1
B)
C)
D)
E)
A)1
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 98 flashcards in this deck.
Unlock Deck
k this deck
78
Find of the geometric sequence, given and
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 98 flashcards in this deck.
Unlock Deck
k this deck
79
Evaluate the binomial coefficient .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 98 flashcards in this deck.
Unlock Deck
k this deck
80
Find the sum.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 98 flashcards in this deck.
Unlock Deck
k this deck



