Deck 5: Formulas, Functions, Linear Equations, and Inequalities in Two Variables
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/63
Play
Full screen (f)
Deck 5: Formulas, Functions, Linear Equations, and Inequalities in Two Variables
1
Find the slope of the line containing the given points.
A) -3
B) 3
C)
D)
E) Undefined
A) -3
B) 3
C)
D)
E) Undefined
3
2
Name the set of inputs (domain) and the set of outputs (range). The capital of the state is a function of the name of the state.
A) {capital}; {name of the state}
B) {name of the state}; {capital}
C) {name of governor}; {capital}
D) {area of state}; {capital}
E) {name of state}; {area of state}
A) {capital}; {name of the state}
B) {name of the state}; {capital}
C) {name of governor}; {capital}
D) {area of state}; {capital}
E) {name of state}; {area of state}
{name of the state}; {capital}
3
Which set of ordered pairs is a function? For each function, name the set of inputs (domain) and the set of outputs (range). a. (7, 9), (7, 11), (7, 13)
b. (7, 9), (9, 11), (11, 13)
A) b is a function. Domain is {7, 9, 11}, range is {9, 11, 13}.
B) a is a function. Domain is {7}, range is {9, 11, 13}.
C) a is a function. Domain is {7}, range is {9, 11, 13}.
B is a function. Domain is {7, 9, 11}, range is {9, 11, 13}.
D) b is a function. Domain is {7}, range is {9, 11, 13}.
A is a function. Domain is {7, 9, 11}, range is {9, 11, 13}.
E) a and b are not functions.
b. (7, 9), (9, 11), (11, 13)
A) b is a function. Domain is {7, 9, 11}, range is {9, 11, 13}.
B) a is a function. Domain is {7}, range is {9, 11, 13}.
C) a is a function. Domain is {7}, range is {9, 11, 13}.
B is a function. Domain is {7, 9, 11}, range is {9, 11, 13}.
D) b is a function. Domain is {7}, range is {9, 11, 13}.
A is a function. Domain is {7, 9, 11}, range is {9, 11, 13}.
E) a and b are not functions.
b is a function. Domain is {7, 9, 11}, range is {9, 11, 13}.
4
Define variables and write the sentence in function notation. The circumference of a circle is a function of the diameter. Circumference is times diameter.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
5
Find and .
A) ,
B) ,
C) ,
D) ,
E) ,
A) ,
B) ,
C) ,
D) ,
E) ,

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
6
Use the slope formula to find the slope. (Hint: To learn the formula, write the complete formula each time you use it.)
A) 0
B)
C)
D)
E) undefined
A) 0
B)
C)
D)
E) undefined

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
7
Given the function , find
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
8
Name the set of inputs (domain) and the set of outputs (range). The cost of a long distance telephone call is a function of how long the parties talk.
A) {distance}; {units of time}
B) {cost of call}; {units of time}
C) {units of time}; {cost of call}
D) {units of time}; {distance}
E) {distance}; {cost of call}
A) {distance}; {units of time}
B) {cost of call}; {units of time}
C) {units of time}; {cost of call}
D) {units of time}; {distance}
E) {distance}; {cost of call}

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
9
Find the slope of the line containing the given points.
A) 4
B)
C) 0
D) 2
E)
A) 4
B)
C) 0
D) 2
E)

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
10
Define variables and write the sentence in function notation. An hourly worker earns $16 per hour. The amount earned is a function of the number of hours worked.
A) A = amount earned ($); x = number of hours worked;
B) A = amount earned ($); x = number of hours worked;
C) A = amount earned ($); x = number of hours worked;
D) A = amount earned ($); x = number of hours worked;
E) A = amount earned ($); x = number of hours worked;
A) A = amount earned ($); x = number of hours worked;
B) A = amount earned ($); x = number of hours worked;
C) A = amount earned ($); x = number of hours worked;
D) A = amount earned ($); x = number of hours worked;
E) A = amount earned ($); x = number of hours worked;

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
11
Use the slope formula to find the slope. (Hint: To learn the formula, write the complete formula each you use it.)
A) -4
B) -7
C) 0
D) 2
E) undefined
A) -4
B) -7
C) 0
D) 2
E) undefined

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
12
Use the slope formula to find slope.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
13
Given the function f(x) = 2x2 - 1, find 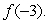
A) 17
B) 19
C) -13
D) 21
E) 20
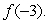
A) 17
B) 19
C) -13
D) 21
E) 20

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
14
Find the slope of the line containing the given points.
A)
B)
C)
D) 0
E) undefined
A)
B)
C)
D) 0
E) undefined

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
15
Use the slope formula to find the slope. (Hint: To learn the formula, write the complete formula each time you use it.)
A) 9
B) 0
C) 10
D) 8
E) undefined
A) 9
B) 0
C) 10
D) 8
E) undefined

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
16
Given the function , find
A) 74
B) 22
C) 40
D) -20
E) 14
A) 74
B) 22
C) 40
D) -20
E) 14

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
17
Which set of ordered pairs is a function? For each function, name the set of inputs (domain) and the set of outputs (range). a. (9, 99), (9, 999), (9, 9999)
b. (-4, 8), (-5, 8), (-6, 8)
A) a is a function. Domain is {9}, range is {99, 999, 9999}.
B) b is a function. Domain is {-4, -5, -6}, range is {8}.
C) a is a function. Domain is {9}, range is {99, 999, 9999}.
B is a function. Domain is {-4, -5, -6}, range is {8}.
D) b is a function. Domain is {9}, range is {99, 999, 9999}.
A is a function. Domain is {-4, -5, -6}, range is {8}.
E) a and b are not functions.
b. (-4, 8), (-5, 8), (-6, 8)
A) a is a function. Domain is {9}, range is {99, 999, 9999}.
B) b is a function. Domain is {-4, -5, -6}, range is {8}.
C) a is a function. Domain is {9}, range is {99, 999, 9999}.
B is a function. Domain is {-4, -5, -6}, range is {8}.
D) b is a function. Domain is {9}, range is {99, 999, 9999}.
A is a function. Domain is {-4, -5, -6}, range is {8}.
E) a and b are not functions.

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
18
Use the slope formula to find the slope. (Hint: To learn the formula, write the complete formula each time you use it.)
A) 0
B) 2
C) -1
D) -2
E) -3
A) 0
B) 2
C) -1
D) -2
E) -3

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
19
Is the set of ordered pairs a function? For each function, name the set of inputs (domain) and the set of outputs (range). (Dou, Ron), (Dou, Ian), (Dou, Vernon)
A) Function. Domain is {Dou}, range is {Ron, Ian, Vernon}.
B) Function. Domain is {Dou}, range is {Ron}.
C) Function. Domain is {Dou}, range is {Ron, Ian}.
D) Function. Domain is {Ron, Ian, Vernon}, range is {Dou}.
E) Not a function
A) Function. Domain is {Dou}, range is {Ron, Ian, Vernon}.
B) Function. Domain is {Dou}, range is {Ron}.
C) Function. Domain is {Dou}, range is {Ron, Ian}.
D) Function. Domain is {Ron, Ian, Vernon}, range is {Dou}.
E) Not a function

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
20
Find and .
A) ,
B) ,
C) ,
D) ,
E) ,
A) ,
B) ,
C) ,
D) ,
E) ,

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
21
Find the equation of the line that passes through the points (1, -2) and (4, 7).
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
22
Find the slope and y-intercept of the line
A) (0, 5)
B) (5, 0)
C) (0, 3)
D) (0, -5)
E) (0, -3)
A) (0, 5)
B) (5, 0)
C) (0, 3)
D) (0, -5)
E) (0, -3)

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
23
Write an equation for the given data. slope =
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
24
Arrange slopes from flattest to steepest.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
25
Find the slope and y-intercept of the line
A) (0, 5)
B) (5, 0)
C) (0, -3)
D) (0, 3)
E) (0, -3)
A) (0, 5)
B) (5, 0)
C) (0, -3)
D) (0, 3)
E) (0, -3)

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
26
Use the slope formula to find the slope. and
A)
B) b
C) 0
D) a
E) undefined
A)
B) b
C) 0
D) a
E) undefined

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
27
Find x if f(x) = 0.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
28
If output values get smaller as input values get larger, then a straight line drawn from the table will :
A) have an undefined slope.
B) have a zero slope.
C) have a positive slope.
D) have a negative slope.
E) fail the vertical-line test.
A) have an undefined slope.
B) have a zero slope.
C) have a positive slope.
D) have a negative slope.
E) fail the vertical-line test.

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
29
Use the slope formula to find the slope. and
A)
B)
C)
D) 0
E) undefined
A)
B)
C)
D) 0
E) undefined

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
30
Find the x- and y-intercepts of the line 2x + 3y = 12 .
A) (4, 0), (0, 6)
B) (-6, 0), (0, 4)
C) (6, 0), (0, 4)
D) (4, 0), (0, -6)
E) (6, 0), (0, -4)
A) (4, 0), (0, 6)
B) (-6, 0), (0, 4)
C) (6, 0), (0, 4)
D) (4, 0), (0, -6)
E) (6, 0), (0, -4)

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
31
Find the equation of the line that passes through the points (0, 1) and (4, -11).
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
32
Arrange slopes from flattest to steepest.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
33
Write an equation for the given data.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
34
The output is the total cost of a shirt, where x is the price of the shirt and a sales tax of 9% of the price is added. Write an equation describing the output (y) as a function of the input (x).
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
35
Find the slope and the vertical-axis intercept for the equation. Assume that the output variable is on the left.
A) The slope is -385 and the vertical-axis intercept is 55.
B) The slope is 385 and the vertical-axis intercept is 55.
C) The slope is 55 and the vertical-axis intercept is 385.
D) The slope is 55 and the vertical-axis intercept is -385.
E) The slope is -55 and the vertical-axis intercept is 385.
A) The slope is -385 and the vertical-axis intercept is 55.
B) The slope is 385 and the vertical-axis intercept is 55.
C) The slope is 55 and the vertical-axis intercept is 385.
D) The slope is 55 and the vertical-axis intercept is -385.
E) The slope is -55 and the vertical-axis intercept is 385.

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
36
Use the slope formula to find the slope. and
A)
B)
C)
D) 0
E) undefined
A)
B)
C)
D) 0
E) undefined

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
37
The output is the total cost of a taxi ride of x miles. The taxi driver charges a $5 fee plus $2 for each mile traveled. Write an equation describing the output (y) as a function of the input (x).
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
38
Find the slope and y-intercept for given equation.
A) ,
B) ,
C) ,
D) ,
E) ,
A) ,
B) ,
C) ,
D) ,
E) ,

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
39
Find the x- and y-intercepts of the line
A) (-5, 0), (0, 7)
B) (0, -5), (7, 0)
C) (-3, 0), (0, 6)
D) (-5, 0), (0, -7)
E) (-3, 0), (0, -6)
A) (-5, 0), (0, 7)
B) (0, -5), (7, 0)
C) (-3, 0), (0, 6)
D) (-5, 0), (0, -7)
E) (-3, 0), (0, -6)

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
40
Find the slope and the vertical-axis intercept for the equation. Assume that the output variable is on the left.
A) The slope is 80 and the vertical-axis intercept is 0.15.
B) The slope is 0.15 and the vertical-axis intercept is 95.
C) The slope is 0.25 and the vertical-axis intercept is 95.
D) The slope is 0.15 and the vertical-axis intercept is 80.
E) The slope is 90 and the vertical-axis intercept is 0.15.
A) The slope is 80 and the vertical-axis intercept is 0.15.
B) The slope is 0.15 and the vertical-axis intercept is 95.
C) The slope is 0.25 and the vertical-axis intercept is 95.
D) The slope is 0.15 and the vertical-axis intercept is 80.
E) The slope is 90 and the vertical-axis intercept is 0.15.

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
41
Identify the steps needed to change the first inequality into the second. to
A) Subtract 3x from both sides.
Divide both sides by -4 and reverse inequality sign.
B) Subtract 3x from both sides.
Divide both sides by 4 and reverse inequality sign.
C) Add 4y to both sides.
Divide both sides by -3 and reverse inequality sign.
D) Add 4y to both sides.
Divide both sides by 3 and reverse inequality sign.
E) Add 4y to both sides.
Divide both sides by 3.
A) Subtract 3x from both sides.
Divide both sides by -4 and reverse inequality sign.
B) Subtract 3x from both sides.
Divide both sides by 4 and reverse inequality sign.
C) Add 4y to both sides.
Divide both sides by -3 and reverse inequality sign.
D) Add 4y to both sides.
Divide both sides by 3 and reverse inequality sign.
E) Add 4y to both sides.
Divide both sides by 3.

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
42
Alberto earns a weekly salary of $500 plus 20% of his sales volume. Write an equation describing the total weekly earnings for x dollars in sales.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
43
Find the equation of the line that contains the point (-9, -6) and has slope .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
44
Which ordered pair is a solution to the inequality?
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
45
Which ordered pair is a solution to the inequality?
A) (12, 1)
B) (-1, -11)
C) (31, 3)
D) (2, -4)
E) (3, -7)
A) (12, 1)
B) (-1, -11)
C) (31, 3)
D) (2, -4)
E) (3, -7)

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
46
Identify the steps needed to change the first inequality into the second.
A) Subtract x from both sides
Divide both sides by 2
B) Subtract x from both sides
Divide both sides by 2 and reverse the inequality sign
C) Add x to both sides
Divide both sides by 2 and reverse the inequality sign
D) Add x to both sides
Divide both sides by 2
E) Multiply both sides by
A) Subtract x from both sides
Divide both sides by 2
B) Subtract x from both sides
Divide both sides by 2 and reverse the inequality sign
C) Add x to both sides
Divide both sides by 2 and reverse the inequality sign
D) Add x to both sides
Divide both sides by 2
E) Multiply both sides by

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
47
Find the equation of the line that contains the point (-27, 13) and has slope .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
48
Find the equation of the line that contains the point (6, -26) and has slope -5.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
49
Which ordered pair is a solution to the inequality?
A) (-3, 1)
B) (3, -1)
C) (6, -1)
D) (-12, 2)
E) (-15, 3)
A) (-3, 1)
B) (3, -1)
C) (6, -1)
D) (-12, 2)
E) (-15, 3)

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
50
Which ordered pair is a solution to the inequality?
A) (4, 5)
B) (-4, 3)
C) (0, 5)
D) (7, 6)
E) (4, 0)
A) (4, 5)
B) (-4, 3)
C) (0, 5)
D) (7, 6)
E) (4, 0)

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
51
Which ordered pair is a solution to the inequality?
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
52
Write the equation for the problem below using Yolanda's $400 monthly expense account is set up through an automatic teller machine (ATM). She withdraws funds, using the $35 Fast Cash Option. The equation describes the amount that remains in her account after she makes x withdrawals during the month.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
53
Four equations are shown. a.
b.
c.
d. Which lines are parallel?
A) c and b
B) c and d
C) c and a
D) d and a
E) a and b
b.
c.
d. Which lines are parallel?
A) c and b
B) c and d
C) c and a
D) d and a
E) a and b

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
54
In the problem below, state whether the described change gives a parallel line or a steeper line and write the new equation. The total monthly cost, in dollars, of a loan is C = 0.01x + 3, where x is the number of dollars borrowed and 3 is the current service fee. The service fee rises by 9 dollars.
A) steeper; C = 9.01x + 3
B) parallel; C = 0.01x + 9
C) steeper; C = 0.01x + 12
D) parallel; C = 9.01x + 3
E) parallel; C = 0.01x + 12
A) steeper; C = 9.01x + 3
B) parallel; C = 0.01x + 9
C) steeper; C = 0.01x + 12
D) parallel; C = 9.01x + 3
E) parallel; C = 0.01x + 12

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
55
Which ordered pair is a solution to the inequality?
A)
B)
C)
D) .
E)
A)
B)
C)
D) .
E)

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
56
Find the equation of the line that passes through the points (0, 0) and (-5, 4).
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
57
Find the equation of the line that contains the point (-1, 3) and has slope -2.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
58
In the problem below, state whether the described change gives a parallel line or a steeper line and write the new equation. The total cost, in dollars, of gasoline is C = 2.30g, where g is in gallons. The gasoline rises in price by $0.80 per gallon.
A) parallel;
B) steeper;
C) parallel; C = 3.20g + 0.80
D) steeper; C = 3.10g
E) parallel; C = 3.10g
A) parallel;
B) steeper;
C) parallel; C = 3.20g + 0.80
D) steeper; C = 3.10g
E) parallel; C = 3.10g

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
59
Find the equation of the line that passes through the points (0, 3) and (-5, 6).
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
60
Which ordered pair is a solution to the inequality?
A) (-2, -2)
B) (3, 5)
C) (5, -3)
D) (-4, 4)
E) (-1, 0)
A) (-2, -2)
B) (3, 5)
C) (5, -3)
D) (-4, 4)
E) (-1, 0)

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
61
A dieter limits a snack to 75 calories. What are three possible combinations of small carrots at 25 calories each and medium celery stalks at 3 calories each.
A) The first combination: 1 carrot and 19 celery stalks.
The second combination: 2 carrots and 8 celery stalks.
The third combination: 3 carrots and 0 celery stalks.
B) The first combination: 1 carrot and 16 celery stalks.
The second combination: 2 carrots and 10 celery stalks.
The third combination: 3 carrots and 0 celery stalks.
C) The first combination: 1 carrot and 16 celery stalks.
The second combination: 2 carrots and 8 celery stalks.
The third combination: 3 carrots and 2 celery stalks.
D) The first combination: 1 carrot and 19 celery stalks.
The second combination: 2 carrots and 10 celery stalks.
The third combination: 3 carrots and 2 celery stalks.
E) The first combination: 1 carrot and 16 celery stalks.
The second combination: 2 carrots and 8 celery stalks.
The third combination: 3 carrots and 0 celery stalks.
A) The first combination: 1 carrot and 19 celery stalks.
The second combination: 2 carrots and 8 celery stalks.
The third combination: 3 carrots and 0 celery stalks.
B) The first combination: 1 carrot and 16 celery stalks.
The second combination: 2 carrots and 10 celery stalks.
The third combination: 3 carrots and 0 celery stalks.
C) The first combination: 1 carrot and 16 celery stalks.
The second combination: 2 carrots and 8 celery stalks.
The third combination: 3 carrots and 2 celery stalks.
D) The first combination: 1 carrot and 19 celery stalks.
The second combination: 2 carrots and 10 celery stalks.
The third combination: 3 carrots and 2 celery stalks.
E) The first combination: 1 carrot and 16 celery stalks.
The second combination: 2 carrots and 8 celery stalks.
The third combination: 3 carrots and 0 celery stalks.

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
62
Which ordered pair is a solution to the inequality?
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck
63
To meet expenses, a local theater group has a ticket sales goal of $2800. Regular tickets sell for $16 and student/senior tickets sell for $14. Let x = number of regular tickets sold and y = number of student/senior tickets sold. Write an inequality describing possible combinations of ticket sales that would meet, or surpass, the goal.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 63 flashcards in this deck.
Unlock Deck
k this deck



