Deck 10: Managing Engineering Design
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/16
Play
Full screen (f)
Deck 10: Managing Engineering Design
1
What are the reasons for dividing the systems engineering/new product development process into phases or stages?
Systems engineering and new product development should be divided into multiple phases to emphasize the efforts being directed to complete the project into a particular area. The division of the phases is created so that there is logical flow between the phases.
It also helps in efficient utilization of available resources and completion of project within time.
Thus, division of project into stages provides a logical continuum of tasks that need to be completed with least possible iterations.
It also helps in efficient utilization of available resources and completion of project within time.
Thus, division of project into stages provides a logical continuum of tasks that need to be completed with least possible iterations.
2
Given three components, each with a reliability of 0.9, calculate the reliability of a total system in which the three are arranged in ( a ) three in series, ( b ) three in parallel, and ( c ), ( d ) two different series/parallel designs each using a total of only three components.
a) If the three components are arranged in series, calculate reliability of the entire system using following formula:
 Where,
Where,
R n represents the reliability of the n th component in the system.
In this case, the system has three components each with reliability of 0.9, therefore, total reliability of the system
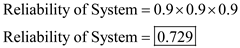 Thus, the system is 72.9% reliable.
Thus, the system is 72.9% reliable.
b) If three components are arranged in parallel, calculate reliability of the entire system using following formula:
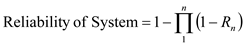 Where,
Where,
R n represents reliability of n th component in the system.
In this case, the system has three components each with reliability of 0.9, therefore, the total reliability of the system is calculated as follows:
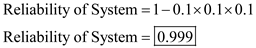 Thus, the system is 99.9% reliable.
Thus, the system is 99.9% reliable.
c) If two components are used in parallel and one component in series to the pair in parallel, calculate total reliability of the system in following manner:
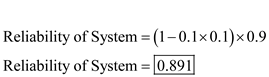 Thus, the system is 89.1% reliable.
Thus, the system is 89.1% reliable.
d) If two components are placed in series, and that pair is placed in parallel with another component, calculate total reliability of the system in following manner:
 Thus, the system is 98.1% reliable.
Thus, the system is 98.1% reliable.
 Where,
Where,R n represents the reliability of the n th component in the system.
In this case, the system has three components each with reliability of 0.9, therefore, total reliability of the system
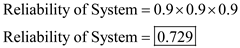 Thus, the system is 72.9% reliable.
Thus, the system is 72.9% reliable. b) If three components are arranged in parallel, calculate reliability of the entire system using following formula:
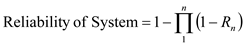 Where,
Where,R n represents reliability of n th component in the system.
In this case, the system has three components each with reliability of 0.9, therefore, the total reliability of the system is calculated as follows:
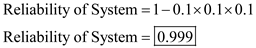 Thus, the system is 99.9% reliable.
Thus, the system is 99.9% reliable. c) If two components are used in parallel and one component in series to the pair in parallel, calculate total reliability of the system in following manner:
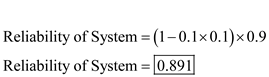 Thus, the system is 89.1% reliable.
Thus, the system is 89.1% reliable. d) If two components are placed in series, and that pair is placed in parallel with another component, calculate total reliability of the system in following manner:
 Thus, the system is 98.1% reliable.
Thus, the system is 98.1% reliable. 3
How does the design work done in the technical feasibility stage of new product development differ from that done in the later design stages?
The design work done in the technical feasibility stage is not in detail of how the product will be manufactured or of the particular features of the product, instead focuses on determining whether the project can be completed within time and resources or not.
However, the work done in new product development stage will delve more into the details and specific features of the product etc.
However, the work done in new product development stage will delve more into the details and specific features of the product etc.
4
A car contains both hydraulic brakes (reliability 0.95) and mechanical brakes (reliability 0.98). What is the probability of stopping ( a ) rapidly at high speed, assuming both systems must work, and ( b ) at low speed, assuming either system will stop the car?

Unlock Deck
Unlock for access to all 16 flashcards in this deck.
Unlock Deck
k this deck
5
What are the principal reasons that a configuration control system is necessary?

Unlock Deck
Unlock for access to all 16 flashcards in this deck.
Unlock Deck
k this deck
6
An engineered system consists of one each of three components X, Y, and Z with reliabilities R X, R Y, and R Z of 0.94, 0.80, and 0.95. ( a ) What is the system reliability, assuming that one component of each type must work? ( b ) If required system reliability is 0.85, show how you can meet this goal by replacing one of these components with two of that same component in parallel.

Unlock Deck
Unlock for access to all 16 flashcards in this deck.
Unlock Deck
k this deck
7
For an engineering design or project management system you are familiar with, describe the drawing release and design review processes.

Unlock Deck
Unlock for access to all 16 flashcards in this deck.
Unlock Deck
k this deck
8
For a component to which the bathtub curve model of reliability applies, describe the provisions you would make to assure a low hazard rate in use of the component.

Unlock Deck
Unlock for access to all 16 flashcards in this deck.
Unlock Deck
k this deck
9
Summarize the history of gradually increasing liability of industry for damage caused by their products.

Unlock Deck
Unlock for access to all 16 flashcards in this deck.
Unlock Deck
k this deck
10
An engineered system has a hazard rate of 0.01 failure per hour. ( a ) What is its MTBF? If the same system has an MTBM of 60 hours, an MDT of 20 hours, and an MTTR of six hours, what are its ( b ) inherent availability and ( c ) operational availability?

Unlock Deck
Unlock for access to all 16 flashcards in this deck.
Unlock Deck
k this deck
11
What are potential solutions to the liability crisis that haunts American enterprises and institutions today?

Unlock Deck
Unlock for access to all 16 flashcards in this deck.
Unlock Deck
k this deck
12
Select a product line in a specific industry, and list actions that can be taken to reduce product liability.

Unlock Deck
Unlock for access to all 16 flashcards in this deck.
Unlock Deck
k this deck
13
Identify a company and product (aside from televisions and automobiles), and tell how good or poor reliability has significantly affected company success. How has reliability changed on the product selected?

Unlock Deck
Unlock for access to all 16 flashcards in this deck.
Unlock Deck
k this deck
14
Describe some mechanisms a designer can use to improve maintainability.

Unlock Deck
Unlock for access to all 16 flashcards in this deck.
Unlock Deck
k this deck
15
Give an example of a consumer product with which you are familiar that, through recent redesign, seems to be a greater value (a better ratio of utility to apparent cost).

Unlock Deck
Unlock for access to all 16 flashcards in this deck.
Unlock Deck
k this deck
16
Discuss how the management functions of planning, organizing, leading, and controlling relate to the engineering design process.

Unlock Deck
Unlock for access to all 16 flashcards in this deck.
Unlock Deck
k this deck



