Deck 3: Linear and Exponential Change: Comparing Growth Rates
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/72
Play
Full screen (f)
Deck 3: Linear and Exponential Change: Comparing Growth Rates
1
The graph of a linear function is a:
A)curve.
B)jagged line.
C)straight line.
D)dotted line.
A)curve.
B)jagged line.
C)straight line.
D)dotted line.
C
2
Suppose you are at the base of a hill, and you see a sign that reads "Elevation 4000 feet." The road you are on goes straight up the hill to the top, where you see another sign that reads "Elevation 4500 feet." If the top of the hill is 5 horizontal miles from the base, what is the growth rate in your elevation with respect to horizontal distance as you drive up the road?
A)150 feet per mile
B)100 feet per mile
C)200 feet per mile
D)500 feet per mile
A)150 feet per mile
B)100 feet per mile
C)200 feet per mile
D)500 feet per mile
B
3
If we drop a rock near the surface of Earth, its velocity increases by 32 feet per second for each second the rock falls. If v is the velocity in feet per second and t is the time in seconds:
A)v = 32t
B)t = 32v
C)v = t + 32
D)t = v + 32
A)v = 32t
B)t = 32v
C)v = t + 32
D)t = v + 32
A
4
On rural highways, the average speed S (in miles per hour) is related to the amount of curvature C (in degrees) of the road. Suppose that on a straight road (C = 0), the average speed is 47.5 miles per hour and that this decreases by 0.65 mph for each additional degree of curvature. What is the average speed if the curvature is 15 degrees?
A)42.35 mph
B)37.75 mph
C)57.15 mph
D)38.35 mph
A)42.35 mph
B)37.75 mph
C)57.15 mph
D)38.35 mph

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
5
Suppose that the cost of purchasing CDs from a music club is a flat membership fee of $25 plus $10 for each CD purchased. If C is the cost in dollars and n is the number of CDs bought, then the amount of money you pay would be:
A)C = 25n + 10
B)C = 10n + 25
C)C = 25n - 10
D)C = 10 - 25n
A)C = 25n + 10
B)C = 10n + 25
C)C = 25n - 10
D)C = 10 - 25n

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
6
In the linear function y = mx + b, b is the:
A)growth rate.
B)slope.
C)initial value.
D)balance.
A)growth rate.
B)slope.
C)initial value.
D)balance.

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
7
A function is called linear if it has a(n) _____ growth rate.
A)increasing
B)decreasing
C)varied
D)constant
A)increasing
B)decreasing
C)varied
D)constant

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
8
The speed of sound at 0 degrees Fahrenheit is 1052.3 feet per second. For every degree of rise in temperature (t), the speed of sound (S) increases by 1.1 feet per second. Find the relation between the S and t.
A)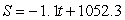
B)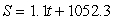
C)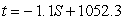
D)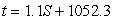
A)
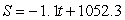
B)
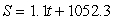
C)
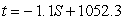
D)
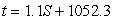

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
9
The following table shows average life expectancy in years at birth in the given year:
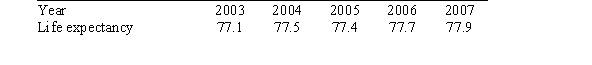 If t denotes the time in years since 2003 and E is the life expectancy in years, then the trend line for this data is given by E = 0.18t + 77.16. If this trend line persisted through 2012, what would be the average life expectancy in years of a child born in 2012?
If t denotes the time in years since 2003 and E is the life expectancy in years, then the trend line for this data is given by E = 0.18t + 77.16. If this trend line persisted through 2012, what would be the average life expectancy in years of a child born in 2012?
A)78.8 years
B)79.0 years
C)78.6 years
D)79.2 years
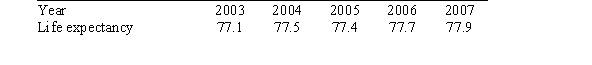 If t denotes the time in years since 2003 and E is the life expectancy in years, then the trend line for this data is given by E = 0.18t + 77.16. If this trend line persisted through 2012, what would be the average life expectancy in years of a child born in 2012?
If t denotes the time in years since 2003 and E is the life expectancy in years, then the trend line for this data is given by E = 0.18t + 77.16. If this trend line persisted through 2012, what would be the average life expectancy in years of a child born in 2012? A)78.8 years
B)79.0 years
C)78.6 years
D)79.2 years

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
10
This table shows the total number of patients diagnosed with the flu since an outbreak started:  Find the slope of the linear function giving the number of diagnosed flu cases per day.
Find the slope of the linear function giving the number of diagnosed flu cases per day.
A)0.7
B)0.9
C)1.2
D)1.4
 Find the slope of the linear function giving the number of diagnosed flu cases per day.
Find the slope of the linear function giving the number of diagnosed flu cases per day.A)0.7
B)0.9
C)1.2
D)1.4

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
11
For the linear function y = mx + b, each 1-unit increase in x corresponds to a change of _____ unit(s) in y.
A)1
B)2
C)m
D)b
A)1
B)2
C)m
D)b

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
12
Suppose that a music club charges $12 per CD but offers a 5% discount on orders larger than 10 CDs. The cost per order is a linear function of the number of CDs ordered.

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
13
This table shows the total number of patients diagnosed with the flu since an outbreak started:  Find the formula for the linear function giving the number of diagnosed flu cases in terms of time if F is the number of flu patients diagnosed and d is time in days.
Find the formula for the linear function giving the number of diagnosed flu cases in terms of time if F is the number of flu patients diagnosed and d is time in days.
A)F = 21d + 0.7
B)F = 0.7d + 21
C)F = 1.4d + 21
D)F = 2.1d + 0.7
 Find the formula for the linear function giving the number of diagnosed flu cases in terms of time if F is the number of flu patients diagnosed and d is time in days.
Find the formula for the linear function giving the number of diagnosed flu cases in terms of time if F is the number of flu patients diagnosed and d is time in days.A)F = 21d + 0.7
B)F = 0.7d + 21
C)F = 1.4d + 21
D)F = 2.1d + 0.7

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
14
Given a set of data points, the _____ line comes as close as possible to fitting those data.
A)interpretation
B)regression
C)stabilizing
D)growth
A)interpretation
B)regression
C)stabilizing
D)growth

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
15
On rural highways, the average speed S (in miles per hour) is related to the amount of curvature C (in degrees) of the road. Suppose that on a straight road (C = 0), the average speed is 47.5 miles per hour and this decreases by 0.65 mph for each additional degree of curvature. Find the formula expressing S as a linear function of C.
A)S = 0.65C + 47.5
B)S = 47.5C + 0.65
C)S = 47.5C - 0.65
D)S = -0.65C + 47.5
A)S = 0.65C + 47.5
B)S = 47.5C + 0.65
C)S = 47.5C - 0.65
D)S = -0.65C + 47.5


Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
16
A new laptop computer selling for $899 in January fell in price to $719 in six months. Assuming the relationship of price to time is linear, determine the decrease in price over each month.
A)$45
B)$40
C)$35
D)$30
A)$45
B)$40
C)$35
D)$30

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
17
A(n) _____ function is a function that changes at a constant percentage rate.
A)logarithmic
B)linear
C)exponential
D)increasing
A)logarithmic
B)linear
C)exponential
D)increasing

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
18
A salesman earns a base salary of $1500 a month plus 4% of his monthly sales. In that case:
A)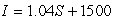
B)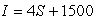
C)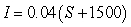
D)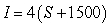
A)
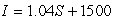
B)
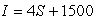
C)
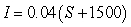
D)
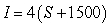

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
19
The speed of sound at 0 degrees Fahrenheit is 1052.3 feet per second. For every degree of rise in temperature, the speed of sound increases by 1.1 feet per second. If the speed of sound is 1131.5 feet per second, then the temperature is _____ degrees Fahrenheit.
A)72
B)77
C)82
D)87
A)72
B)77
C)82
D)87

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
20
The speed of sound at 0 degrees Fahrenheit is 1052.3 feet per second. For every degree of rise in temperature, the speed of sound increases by 1.1 feet per second. At 67 degrees the speed of sound is _____ feet per second.
A)73.7
B)1119.3
C)1053.4
D)1126.0
A)73.7
B)1119.3
C)1053.4
D)1126.0

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
21
If the decibel of a sound is doubled, then the relative intensity will be:
A)doubled.
B)squared.
C)increased by a factor of 10.
D)increased by a factor of 100.
A)doubled.
B)squared.
C)increased by a factor of 10.
D)increased by a factor of 100.

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
22
Suppose the number of Internet domain hosts grew according to the rule Next year's number = 1.47 × Current number. If the number of domain hosts initially was 8.4 million, find an exponential function that gives the number of hosts, H, in terms of time, t
A)H = 1.47 × (8.4)t
B)H = 1.47 + (8.4)t
C)H = 8.4 + (1.47)t
D)H = 8.4 × (1.47)t
A)H = 1.47 × (8.4)t
B)H = 1.47 + (8.4)t
C)H = 8.4 + (1.47)t
D)H = 8.4 × (1.47)t

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
23
The _____ of a radioactive substance is the time it takes for half of the substance to decay.
A)doubling time
B)half-life
C)shelf-life
D)halving time
A)doubling time
B)half-life
C)shelf-life
D)halving time

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
24
The formula for an exponential function y of t is:
A)y = Initial value × Base
B)y = Initial value × (Base)t
C)y = Base × (Initial value)t
D)y = Initial value + (Base)t
A)y = Initial value × Base
B)y = Initial value × (Base)t
C)y = Base × (Initial value)t
D)y = Initial value + (Base)t

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
25
An ordinary sheet of paper is about a tenth of a millimeter thick. Find an exponential formula that gives the thickness, T, in millimeters after n folds.
A)T = 0.1 × 2n
B)T = 0.1 × 4n
C)T = 0.2 × (0.5)n
D)T = 0.1 × (0.5)n
A)T = 0.1 × 2n
B)T = 0.1 × 4n
C)T = 0.2 × (0.5)n
D)T = 0.1 × (0.5)n

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
26
Actinium-225 has a half-life of 10 days. Suppose we have an initial amount of 100 grams of actinium-225. How much would be present after 30 days?
A)50 grams
B)33.3 grams
C)25 grams
D)12.5 grams
A)50 grams
B)33.3 grams
C)25 grams
D)12.5 grams

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
27
If a population grows exponentially as time increases, then time _____ as population increases.
A)grows exponentially
B)grows logarithmically
C)decays exponentially
D)decays logarithmically
A)grows exponentially
B)grows logarithmically
C)decays exponentially
D)decays logarithmically

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
28
From 1929 to the early 1930s, the prices of consumer goods actually decreased. Economists call this phenomenon deflation. The rate of deflation during this period was around 7% per year. Suppose this rate of deflation persisted over 20 years. What would be the cost after 20 years of an item that cost $500 initially?
A)$23.25
B)$61.66
C)$271.90
D)$117.12
A)$23.25
B)$61.66
C)$271.90
D)$117.12

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
29
The _____ of an earthquake is the logarithm of relative intensity.
A)magnitude
B)scale
C)Richter value
D)degree
A)magnitude
B)scale
C)Richter value
D)degree

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
30
Suppose that in January there is a magnitude 4.5 earthquake hitting the East Coast of the United States. Six months later, a magnitude 6.5 earthquake hits the West Coast. How many times more intense was the West Coast quake than the East Coast quake?
A)2
B)10
C)100
D)1000
A)2
B)10
C)100
D)1000

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
31
The probability P (as a decimal) that no tsunami with waves over 15 feet high will strike a beach community over a period of t years is given by the formula P = 0.93t. What is the percentage decrease of the probability for each 1-year increase in the time interval?
A)3%
B)5%
C)7%
D)9%
A)3%
B)5%
C)7%
D)9%

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
32
The following table shows the number of certain objects for given time . Which of the following best describes as a function of ? 



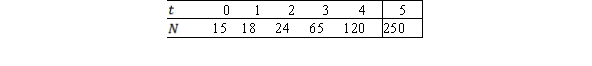
A)linear
B)exponential
C)logarithmic
D)constant





A)linear
B)exponential
C)logarithmic
D)constant

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
33
The probability P (as a decimal) that a tsunami with waves over 15 feet high will NOT strike a beach community over a period of t years is given by the formula P = 0.93t. What is the probability that someone living in the beach community for 20 years will NOT encounter a tsunami with waves 15 feet or higher?
A)23.4%
B)18.6%
C)33.7%
D)14.0%
A)23.4%
B)18.6%
C)33.7%
D)14.0%

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
34
An investment grows according to the rule: Next month's balance = 1.003 × Current balance. Find the percentage increase for one year.
A)0.003%
B)1.81%
C)0.3%
D)12.036%
A)0.003%
B)1.81%
C)0.3%
D)12.036%

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
35
The rate of inflation measures the percentage increase in the price of consumer goods. The rate of inflation from the year 2005 to 2007 was 5%. Suppose that this rate persisted through 2015. What would be the cost in 2015 of an item that costs $100 in 2005?
A)$127.63
B)$135.89
C)$149.63
D)$162.89
A)$127.63
B)$135.89
C)$149.63
D)$162.89

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
36
The number of blogs (or weblogs) grew rapidly for several years. According to one report, since early 2004 the number of blogs has doubled in size every six months. What is the percent increase from March 2004 to March 2006?
A)1500%
B)1600%
C)3100%
D)3200%
A)1500%
B)1600%
C)3100%
D)3200%

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
37
Initially, a population is 750, and it grows by 3% each year. Find a formula for the population, P, at any time, t.
A)P = 1.03 × (750)t
B)P = 0.03 × (750)t
C)P = 750 × (1.03)t
D)P = 750 × (0.03)t
A)P = 1.03 × (750)t
B)P = 0.03 × (750)t
C)P = 750 × (1.03)t
D)P = 750 × (0.03)t

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
38
Suppose Mark's salary grows by $2500 each year and Sarah's salary grows by 2.5% each year. Which one has a salary that grows exponentially?
A)Mark
B)Sarah
C)Both
D)Neither
A)Mark
B)Sarah
C)Both
D)Neither

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
39
An exponential function y of t is characterized by the following property: When t increases by 1, to find the new value of y, we multiply the current value by _____.
A)t + 1
B)t - 1
C)the initial value
D)the base
A)t + 1
B)t - 1
C)the initial value
D)the base

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
40
For traffic that flows on a highway, the headway is the average time between vehicles. On four-lane highways, the probability P (as a decimal) that the headway is at least t seconds when there are 500 vehicles per hour traveling one way is given by P = 0.87t. Under these circumstances, what is the probability that the headway is at least 15 seconds?
A)13.05%
B)12.38%
C)11.45%
D)10.55%
A)13.05%
B)12.38%
C)11.45%
D)10.55%

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
41
The acidity of a solution is determined by the concentration H of hydrogen ions. The formula is . The accompanying exponential formula is H = 0.1pH. Lower pH values indicate a more acidic solution. Normal rain has a pH of 5.6. Suppose acid rain has a pH of 4.3. How many times as acidic as normal rain is this? 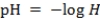
A)2
B)5
C)10
D)20
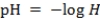
A)2
B)5
C)10
D)20

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
42
In an area prone to earthquakes there is a 6.9 magnitude quake. Two years before there was a 6.6 magnitude quake. The relative intensity of the 6.9 quake was about twice that of the 6.6 quake.

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
43
Solve the equation . 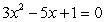
A)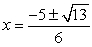
B)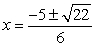
C)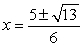
D)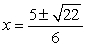
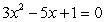
A)
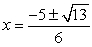
B)
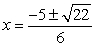
C)
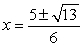
D)
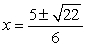

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
44
Which of the following is a quadratic function?
A)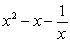
B)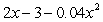
C)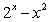
D)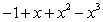
A)
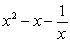
B)
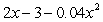
C)
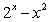
D)
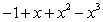

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
45
Which of the following statements is NOT correct for the function ? 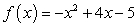
A)The function is a quadratic function.
B)The graph of is open downward.
C)The vertex of the graph of is .
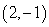
D)The function has 2 distinct real zeros.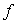
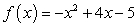
A)The function is a quadratic function.
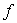
B)The graph of is open downward.
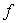
C)The vertex of the graph of is .
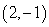
D)The function has 2 distinct real zeros.
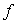

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
46
How many times more intense is a 6.0 magnitude earthquake than a 3.0 magnitude earthquake?
A)2
B)3
C)100
D)1000
A)2
B)3
C)100
D)1000

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
47
The graph of a quadratic function has a special shape known as a:
A)line.
B)parabola.
C)hyperbola.
D)ellipse.
A)line.
B)parabola.
C)hyperbola.
D)ellipse.

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
48
Suppose that inflation is 3% per year. This means the cost of an item increases by 3% each year. Suppose a jacket cost $150 in 2011. How long will it take for the jacket to cost $200 dollars?
A)5 years
B)10 years
C)15 years
D)20 years
A)5 years
B)10 years
C)15 years
D)20 years

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
49
Radium-226 is subject to radioactive decay, and each year the amount present is reduced by 4.2%. The amount of radium-226 is an exponential function of time in years. What is the base of this exponential function?
A)0.042
B)0.238
C)0.958
D)4.2
A)0.042
B)0.238
C)0.958
D)4.2

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
50
What is the number of distinct real solutions for the equation ? 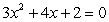
A)0
B)1
C)2
D)3
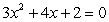
A)0
B)1
C)2
D)3

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
51
Suppose you make an investment of $2000 that you are not allowed to cash in for five years. Unfortunately, the value of the investment decreases by 15% per year. How long will it be before your investment decreases to half its original value?
A)2.5 years
B)3.6 years
C)4.1 years
D)4.3 years
A)2.5 years
B)3.6 years
C)4.1 years
D)4.3 years

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
52
A speaker is playing music at 75 decibels. A second speaker playing the same music at the same decibel reading is placed beside the first. What is the decibel reading of the pair of speakers?
A)150 decibels
B)180 decibels
C)98 decibels
D)78 decibels
A)150 decibels
B)180 decibels
C)98 decibels
D)78 decibels

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
53
The loudness of sound decreases as the distance from the source increases. Doubling the distance from a sound multiplies the relative intensity of the sound by one-fourth. When the sound of a speaker is recorded as 80 decibels at 10 feet, what is the decibel reading if four identical speakers are added at 20 feet?
A)83 decibels
B)84 decibels
C)92 decibels
D)93 decibels
A)83 decibels
B)84 decibels
C)92 decibels
D)93 decibels

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
54
An increase of 1 on the pH scale causes acidity to be multiplied by a factor of 0.1, while a decrease of 1 on the pH scale causes acidity to be multiplied by a factor of 10. If pure water has a pH of 7, how many times as acidic as pure water is a solution measuring a pH of 3?
A)10
B)100
C)1000
D)10,000
A)10
B)100
C)1000
D)10,000

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
55
The loudness of sound decreases as the distance from the source increases. Doubling the distance from a sound multiplies the relative intensity of the sound by one-fourth. If the sound of a speaker is recorded at 80 decibels at 4 feet, what is the decibel reading at 8 feet?
A)20 decibels
B)46 decibels
C)68 decibels
D)74 decibels
A)20 decibels
B)46 decibels
C)68 decibels
D)74 decibels

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
56
An investment is growing by 9% each year. How long does it take the investment to double in value?
A)7 years
B)8 years
C)10 years
D)11 years
A)7 years
B)8 years
C)10 years
D)11 years

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
57
Suppose that inflation is 3% per year. This means the cost of an item increases by 3% each year. Suppose a jacket cost $150 in 2011. Find a formula that gives the cost of the jacket C in dollars after t years.
A)C = $150 + 1.03t
B)C = $150 × 1.03t
C)C = $150 × 1.3t
D)C = $150 + 1.3t
A)C = $150 + 1.03t
B)C = $150 × 1.03t
C)C = $150 × 1.3t
D)C = $150 + 1.3t

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
58
Factor the equation . 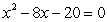
A)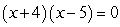
B)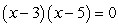
C)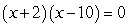
D)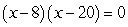
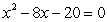
A)
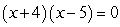
B)
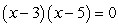
C)
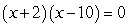
D)
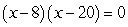

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
59
If the per capita growth rate of the world population continues to be what it was in the year 2000, the world population t years after July 1, 2000, will be 6.085 × 1.0121t billion. According to this formula, when will the world population reach 9 billion?
A)41.3 years after July 1, 2000
B)32.5 years after July 1, 2000
C)22.7 years after July 1, 2000
D)6.8 years after July 1, 2000
A)41.3 years after July 1, 2000
B)32.5 years after July 1, 2000
C)22.7 years after July 1, 2000
D)6.8 years after July 1, 2000

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
60
What is the product of two solutions of the equation ? 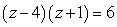
A)-10
B)24
C)-24
D)10
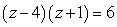
A)-10
B)24
C)-24
D)10

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
61
A small business has the following revenue and cost functions for a product: and . If the break-even point lies where revenue equals cost, how many break-even points are there? 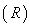
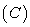
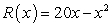
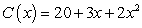
A)0
B)1
C)2
D)3
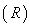
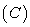
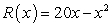
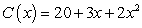
A)0
B)1
C)2
D)3

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
62
A ball is hit upward with an initial velocity of 128 feet per second. After seconds, its height is given by the function . How many seconds does the ball take to reach its maximum height? 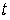
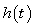
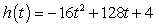
A)4.0 seconds
B)4.5 seconds
C)5.0 seconds
D)5.5 seconds
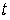
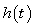
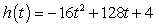
A)4.0 seconds
B)4.5 seconds
C)5.0 seconds
D)5.5 seconds

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
63
A ball is hit upward with an initial velocity of 128 feet per second. After seconds, its height is given by the function . What is the maximum height in feet of the ball? 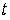
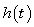
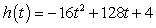
A)256 feet
B)260 feet
C)264 feet
D)268 feet
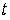
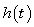
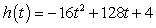
A)256 feet
B)260 feet
C)264 feet
D)268 feet

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
64
What value of makes a minimum? 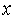
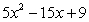
A)1.5
B)2
C)3
D)3.5
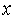
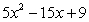
A)1.5
B)2
C)3
D)3.5

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
65
Suppose that a rifle bullet follows a parabolic path given by , where both and are measured in feet. How far downrange will the bullet hit the ground? 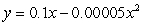
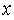
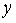
A)2000 feet
B)2200 feet
C)2500 feet
D)3000 feet
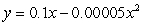
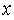
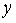
A)2000 feet
B)2200 feet
C)2500 feet
D)3000 feet

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
66
What is the maximum value of the function ? 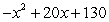
A)200
B)230
C)250
D)280
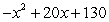
A)200
B)230
C)250
D)280

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
67
Cutting 7-inch-long squares from a square piece of cardboard and folding up the edges, you want to make an open box (without lid) with a square bottom, a height of 7 inches, and a volume of 252 cubic inches. What should be the dimension of the cardboard?
A)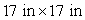
B)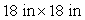
C)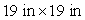
D)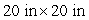
A)
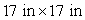
B)
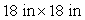
C)
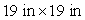
D)
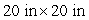

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
68
A walkway of a park has the shape of a right triangle with three side lengths . Find the perimeter of the right triangle. 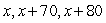
A)240
B)270
C)285
D)300
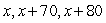
A)240
B)270
C)285
D)300

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
69
You have a 400-foot roll of fencing to enclose a playground. If you want to construct a rectangular playground, what is the largest possible area of the playground?
A)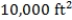
B)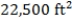
C)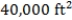
D)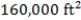
A)
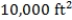
B)
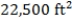
C)
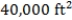
D)
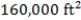

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
70
You have a 600-foot roll of fencing to enclose two separate rectangular playgrounds for different age groups. If the two playgrounds are identical and adjacent, and form a larger rectangular region, what is the largest possible area of the playgrounds in total?
A)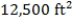
B)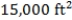
C)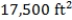
D)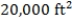
A)
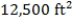
B)
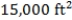
C)
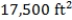
D)
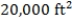

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
71
A rectangular playground has the dimensions 20 yards by 30 yards. You want to double the space by adding the same amount to both the length and width. What is the perimeter of the expanded playground?
A)140 yards
B)160 yards
C)180 yards
D)120 yards
A)140 yards
B)160 yards
C)180 yards
D)120 yards

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck
72
For a certain product X, the profit is modeled as , where equals the number of units sold. How many units sold will produce the maximum profit? 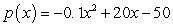
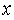
A)20 units
B)50 units
C)100 units
D)200 units
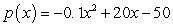
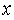
A)20 units
B)50 units
C)100 units
D)200 units

Unlock Deck
Unlock for access to all 72 flashcards in this deck.
Unlock Deck
k this deck



