Deck 16: Data Preparation for Analysis
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/15
Play
Full screen (f)
Deck 16: Data Preparation for Analysis
1
How is sample size determined based on anticipated cross-tab analyses
Cross tab analyses: A method used for understanding the link between two or more nominal or categorical variables.
In cross tab analyses grouping of two variables in one point is called a cell. The sample size is determined by defining the minimum number of respondent in each cell. The minimum number of cases falling into a cell is generally taken to be 5 but if the cross tab is central to the purpose of study, then by general rule of thumb 100 or more cases are needed in each cell.
Different purpose of studies requires different sample size. But the stages in the research process are very much related with sample size, and a decision with respect to one stage can affect all the other stages. Therefore, decision with respect to method of analysis can have an important force on the size of the sample that should be selected.
In cross tab analyses grouping of two variables in one point is called a cell. The sample size is determined by defining the minimum number of respondent in each cell. The minimum number of cases falling into a cell is generally taken to be 5 but if the cross tab is central to the purpose of study, then by general rule of thumb 100 or more cases are needed in each cell.
Different purpose of studies requires different sample size. But the stages in the research process are very much related with sample size, and a decision with respect to one stage can affect all the other stages. Therefore, decision with respect to method of analysis can have an important force on the size of the sample that should be selected.
2
A survey was being designed by the marketing research department of a medium-sized manufacturer of household appliances. The general aim was to assess customer satisfaction with the company's dishwashers. As part of this general objective, management wanted to measure the average maintenance expenditure per year per household, the average number of malfunctions or breakdowns per year, and the number of times a dishwasher is cleaned within a year. Management wished to be 95% confident in the results. Furthermore, the magnitude of the error was not to exceed +/-$4 for maintenance expenditures, +/-1 for malfunctions, and +/-4 for cleanings. The research department noted that while some households would spend nothing on maintenance expenditures per year, others might spend as much as $120. Also, while some dish-washers would experience no breakdowns within a year, the maximum expected would be no more than three. Finally, while some dishwashers might not be cleaned at all during the year, others might be cleaned as frequently as once a month.
a. How large a sample would you recommend if each of the three variables were considered separately Show all your calculations.
b. What size sample would you recommend overall given that management felt that the expenditure on repairs was most important and the number of cleanings least impor-tant to know accurately
a. How large a sample would you recommend if each of the three variables were considered separately Show all your calculations.
b. What size sample would you recommend overall given that management felt that the expenditure on repairs was most important and the number of cleanings least impor-tant to know accurately
Precision: The Precision is equal to scale of error in the estimation of a population characteristic.Confidence: The confidence interval is equal to the level to which one can sense that an estimate approximates to the true value. The formula for calculating the necessary sample size for estimating the population mean is as follows:
 Here,
Here,
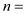 Required sample size
Required sample size
 Z-score corresponding to the anticipated degree of confidence
Z-score corresponding to the anticipated degree of confidence
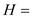 Half precision
Half precision
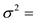 Variance of the characteristic in the population
Variance of the characteristic in the population
Consider the provide details to solve the subparts. According to the provide details, management wants 95% confident in the result. Also the precision of the error was not to exceed
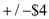 for maintenance expenditure,
for maintenance expenditure,
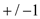 for break down and
for break down and
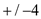 for cleanings.a.
for cleanings.a.
Let us calculate sample size for each variable, first of all consider maintenance expenditure. The variation range for maintenance expenditures is varies between
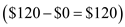 and the estimated standard deviation would be:
and the estimated standard deviation would be:
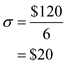 The Z-score corresponding to the anticipated degree of confidence 95% is
The Z-score corresponding to the anticipated degree of confidence 95% is
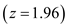 . With preferred precision of
. With preferred precision of
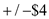 and 95% confident the calculation of sample size is as follows:
and 95% confident the calculation of sample size is as follows:
 Therefore, the sample size is equal to 96 for preferred precision and given variable.Consider break down variable. The variation range for breakdown yearly is varies between
Therefore, the sample size is equal to 96 for preferred precision and given variable.Consider break down variable. The variation range for breakdown yearly is varies between
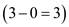 and the estimated standard deviation would be:
and the estimated standard deviation would be:
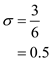 The Z-score corresponding to the anticipated degree of confidence 95% is
The Z-score corresponding to the anticipated degree of confidence 95% is
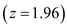 . With preferred precision of
. With preferred precision of
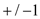 and 95% confident the calculation of sample size is as follows:
and 95% confident the calculation of sample size is as follows:
 Therefore, the sample size is approximately equal to 1 for preferred precision and given variable.Consider cleaning variable. Some dishwashers not cleaned throughout the year or some cleaned regularly as once a month. The variation range for cleanings is varies between
Therefore, the sample size is approximately equal to 1 for preferred precision and given variable.Consider cleaning variable. Some dishwashers not cleaned throughout the year or some cleaned regularly as once a month. The variation range for cleanings is varies between
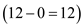 and the estimated standard deviation would be:
and the estimated standard deviation would be:
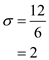 The Z-score corresponding to the anticipated degree of confidence 95% is
The Z-score corresponding to the anticipated degree of confidence 95% is
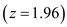 . With preferred precision of
. With preferred precision of
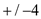 and 95% confident the calculation of sample size is as follows:
and 95% confident the calculation of sample size is as follows:
 Therefore, the sample size is approximately equal to 1 for preferred precision and given variable.b.
Therefore, the sample size is approximately equal to 1 for preferred precision and given variable.b.
If the management considers that the expenditure on repairs was most important and the number of cleanings slightest important variable, then management will select the sample size for the most important variable. Hence the management will select the recommended sample size as
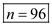 .
.
 Here,
Here,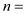 Required sample size
Required sample size Z-score corresponding to the anticipated degree of confidence
Z-score corresponding to the anticipated degree of confidence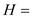 Half precision
Half precision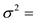 Variance of the characteristic in the population
Variance of the characteristic in the populationConsider the provide details to solve the subparts. According to the provide details, management wants 95% confident in the result. Also the precision of the error was not to exceed
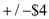 for maintenance expenditure,
for maintenance expenditure, 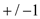 for break down and
for break down and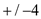 for cleanings.a.
for cleanings.a. Let us calculate sample size for each variable, first of all consider maintenance expenditure. The variation range for maintenance expenditures is varies between
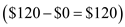 and the estimated standard deviation would be:
and the estimated standard deviation would be: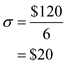 The Z-score corresponding to the anticipated degree of confidence 95% is
The Z-score corresponding to the anticipated degree of confidence 95% is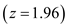 . With preferred precision of
. With preferred precision of 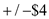 and 95% confident the calculation of sample size is as follows:
and 95% confident the calculation of sample size is as follows: Therefore, the sample size is equal to 96 for preferred precision and given variable.Consider break down variable. The variation range for breakdown yearly is varies between
Therefore, the sample size is equal to 96 for preferred precision and given variable.Consider break down variable. The variation range for breakdown yearly is varies between 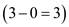 and the estimated standard deviation would be:
and the estimated standard deviation would be: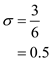 The Z-score corresponding to the anticipated degree of confidence 95% is
The Z-score corresponding to the anticipated degree of confidence 95% is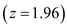 . With preferred precision of
. With preferred precision of 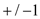 and 95% confident the calculation of sample size is as follows:
and 95% confident the calculation of sample size is as follows: Therefore, the sample size is approximately equal to 1 for preferred precision and given variable.Consider cleaning variable. Some dishwashers not cleaned throughout the year or some cleaned regularly as once a month. The variation range for cleanings is varies between
Therefore, the sample size is approximately equal to 1 for preferred precision and given variable.Consider cleaning variable. Some dishwashers not cleaned throughout the year or some cleaned regularly as once a month. The variation range for cleanings is varies between 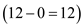 and the estimated standard deviation would be:
and the estimated standard deviation would be: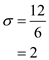 The Z-score corresponding to the anticipated degree of confidence 95% is
The Z-score corresponding to the anticipated degree of confidence 95% is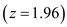 . With preferred precision of
. With preferred precision of 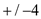 and 95% confident the calculation of sample size is as follows:
and 95% confident the calculation of sample size is as follows: Therefore, the sample size is approximately equal to 1 for preferred precision and given variable.b.
Therefore, the sample size is approximately equal to 1 for preferred precision and given variable.b. If the management considers that the expenditure on repairs was most important and the number of cleanings slightest important variable, then management will select the sample size for the most important variable. Hence the management will select the recommended sample size as
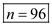 .
. 3
In determining sample size, what factors must an analyst consider
Sample size is the total number of observations in the sample that is used to make analysis. For example: if researcher is doing research on 100 men to notice their average height then the sample size can be considered as 100. Generally, the sample size can be denoted by n.There are some factors that researcher will keep in mind while determining the sample size for any particular study. Those factors are written below:
1. Selection of sample size will depends on the accuracy of the estimate. Hence, the first factor is the precision of estimate.2. The second factor, that the researcher will notice is the degree of confidence. It tells whether the population parameter will lie within the precision interval or not.3. The third factor is the variation in parameter of interest. It will notice how much variation is on the parameter of interest or the variable that is needed to study.The above points are very important to determine sample size for getting appropriate results in the study performed by any researcher because these are the base steps to plan about a sample size.
1. Selection of sample size will depends on the accuracy of the estimate. Hence, the first factor is the precision of estimate.2. The second factor, that the researcher will notice is the degree of confidence. It tells whether the population parameter will lie within the precision interval or not.3. The third factor is the variation in parameter of interest. It will notice how much variation is on the parameter of interest or the variable that is needed to study.The above points are very important to determine sample size for getting appropriate results in the study performed by any researcher because these are the base steps to plan about a sample size.
4
The management of a major dairy wanted to determine the average ounces of milk consumed per resident in the state of Montana. Past trends indicated that the variation fill milk consumption(s) was four ounces. A 95% confidence level is required, and the error -1 is not to exceed +/-12 ounces.
a. What sample size would you recommend Show your calculations.
b. Management wanted to double the level of precision and increase the level of confidence to 99%. What sample size would you recommend Show your calculations. Comment on your results.
a. What sample size would you recommend Show your calculations.
b. Management wanted to double the level of precision and increase the level of confidence to 99%. What sample size would you recommend Show your calculations. Comment on your results.

Unlock Deck
Unlock for access to all 15 flashcards in this deck.
Unlock Deck
k this deck
5
What is the difference between degree of confidence and degree of precision

Unlock Deck
Unlock for access to all 15 flashcards in this deck.
Unlock Deck
k this deck
6
The manager of a local recreational center wanted to determine the average amount each customer spent on traveling to and from the center. On the basis of the findings, the manager was planning on raising the entrance fee. The manager noted that customers living near the center would spend almost nothing on traveling. On die other hand, customers living at die other side of town had to travel about 15 miles and spent about 30 cents (U.S.) per mile on average. The manager wanted to be 95% confident of die findings and did not want the error to exceed +/-10 cents. What sample size should the manager use to determine the average travel expenditure Show your calculations.

Unlock Deck
Unlock for access to all 15 flashcards in this deck.
Unlock Deck
k this deck
7
How do you determine the sample size necessary to estimate a population mean with some desired degree of precision and confidence

Unlock Deck
Unlock for access to all 15 flashcards in this deck.
Unlock Deck
k this deck
8
A large manufacturer of chemicals recently came under severe criticism from various environmentalists for its disposal of industrial effluent and waste. In response, management launched a campaign to counter the bad publicity it was receiving. A study of the effectiveness of the campaign indicated that about 20% of the residents of the city were aware of the campaign and the company's position. In conducting the study, a sample of 400 was used and a 95% confidence level was specified. Three months later, it was believed that 30% of the residents were aware of the campaign. However, management decided to do another survey and specified a 99% confidence level and a margin error of +/-2 percentage points. What sample size would you recommend for this study Show all your calculations.

Unlock Deck
Unlock for access to all 15 flashcards in this deck.
Unlock Deck
k this deck
9
What effect would relaxing the precision with which a population mean is estimated have on sample size Decreasing the degree of confidence from 95 to 90%

Unlock Deck
Unlock for access to all 15 flashcards in this deck.
Unlock Deck
k this deck
10
Score-It, Inc. is a large manufacturer of video games for game systems. The marketing research department is designing a survey to determine attitudes toward the products. Additionally, the percentage of households: owning game systems and the average usage rate per week are to be determined. The department wants to be 95% confident of the results and does not want the error to exceed +/-3 percentage points for video game ownership and +/-1 hour for average usage rate. Previous reports indicate that about 70% of the households own game systems, and the average usage rate is 20 hours with a standard deviation of 10 hours.
a. What sample size would you recommend, assuming only the percentage of households owning video games is to be determined Show all your calculations.
b. What sample size would you recommend, assuming only the average usage rate per week is to be determined Show all your calculations.
c. What sample size would you recommend, assuming both the above variables are to be determined Why
a. What sample size would you recommend, assuming only the percentage of households owning video games is to be determined Show all your calculations.
b. What sample size would you recommend, assuming only the average usage rate per week is to be determined Show all your calculations.
c. What sample size would you recommend, assuming both the above variables are to be determined Why

Unlock Deck
Unlock for access to all 15 flashcards in this deck.
Unlock Deck
k this deck
11
Suppose that you wanted to estimate a population proportion within +/-3 percentage points at the 95% level of confidence. How would you proceed, and what quantities would you need to estimate

Unlock Deck
Unlock for access to all 15 flashcards in this deck.
Unlock Deck
k this deck
12
The local gas and electric company in a city in the northeast United States recently started a campaign to encourage people to reduce unnecessary use of gas and electricity. To assess the effectiveness of the campaign, management wanted to do a survey to deter-mine the proportion of people that had adopted the recommended energy-saving measures. What sample size would you recommend if the error was not to exceed +/-0.025 percentage point and the confidence level was to be 90% Show your calculations.

Unlock Deck
Unlock for access to all 15 flashcards in this deck.
Unlock Deck
k this deck
13
Where can you go for the estimates of population variance needed for calculating sample size for a mean

Unlock Deck
Unlock for access to all 15 flashcards in this deck.
Unlock Deck
k this deck
14
If you had absolutely no idea about a population proportion but wanted to use the most conservative position possible, what would your estimate be Why

Unlock Deck
Unlock for access to all 15 flashcards in this deck.
Unlock Deck
k this deck
15
What is the correct procedure for choosing sample size when making multiple estimates

Unlock Deck
Unlock for access to all 15 flashcards in this deck.
Unlock Deck
k this deck



