Deck 1: The Art of Problem Solving
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/190
Play
Full screen (f)
Deck 1: The Art of Problem Solving
1
Determine the most probable next term in the sequence.
5, 7, 9, 11, 13
A)14
B)17
C)18
D)15
5, 7, 9, 11, 13
A)14
B)17
C)18
D)15
D
2
Decide whether the argument is an example of inductive or deductive reasoning.
His last four at bats were strikeouts. Therefore, the next will be a strikeout.
A)Deductive
B)Inductive
His last four at bats were strikeouts. Therefore, the next will be a strikeout.
A)Deductive
B)Inductive
B
3
Determine the most probable next term in the sequence.
8, 13, 20, 29, 40, 53
A)66
B)64
C)68
D)70
8, 13, 20, 29, 40, 53
A)66
B)64
C)68
D)70
C
4
Determine the most probable next term in the sequence.



Unlock Deck
Unlock for access to all 190 flashcards in this deck.
Unlock Deck
k this deck
5
Use inductive reasoning to predict the next equation.
76 - 74 + 72 - 70 = 77 - 75 + 73 - 71 86 - 84 + 82 - 80 = 87 - 85 + 83 - 81
A)96 + 94 - 92 - 90 = 97 + 95 - 93 + 91
B)96 - 94 - 92 + 90 = 97 - 95 + 93 - 91
C)96 + 94 - 92 + 90 = 97 + 95 - 93 + 91
D)96 - 94 + 92 - 90 = 97 - 95 + 93 - 91
76 - 74 + 72 - 70 = 77 - 75 + 73 - 71 86 - 84 + 82 - 80 = 87 - 85 + 83 - 81
A)96 + 94 - 92 - 90 = 97 + 95 - 93 + 91
B)96 - 94 - 92 + 90 = 97 - 95 + 93 - 91
C)96 + 94 - 92 + 90 = 97 + 95 - 93 + 91
D)96 - 94 + 92 - 90 = 97 - 95 + 93 - 91

Unlock Deck
Unlock for access to all 190 flashcards in this deck.
Unlock Deck
k this deck
6
Decide whether the argument is an example of inductive or deductive reasoning.
Practice makes perfect. I have practiced. Therefore, I'll be perfect.
A)Inductive
B)Deductive
Practice makes perfect. I have practiced. Therefore, I'll be perfect.
A)Inductive
B)Deductive

Unlock Deck
Unlock for access to all 190 flashcards in this deck.
Unlock Deck
k this deck
7


Unlock Deck
Unlock for access to all 190 flashcards in this deck.
Unlock Deck
k this deck
8
Determine the most probable next term in the sequence.
6, -24, 96, -384, 1536
A)-2688
B)6144
C)-6144
D)2688
6, -24, 96, -384, 1536
A)-2688
B)6144
C)-6144
D)2688

Unlock Deck
Unlock for access to all 190 flashcards in this deck.
Unlock Deck
k this deck
9
Determine the most probable next term in the sequence.
2, 5, 4, 10, 8, 20
A)40
B)12
C)30
D)16
2, 5, 4, 10, 8, 20
A)40
B)12
C)30
D)16

Unlock Deck
Unlock for access to all 190 flashcards in this deck.
Unlock Deck
k this deck
10


Unlock Deck
Unlock for access to all 190 flashcards in this deck.
Unlock Deck
k this deck
11
Determine the most probable next term in the sequence.
34, 28, 22, 16, 10
A)4
B)0
C)2
D)6
34, 28, 22, 16, 10
A)4
B)0
C)2
D)6

Unlock Deck
Unlock for access to all 190 flashcards in this deck.
Unlock Deck
k this deck
12
Decide whether the argument is an example of inductive or deductive reasoning.
All U.S. Presidents have come from the original 48 states. Therefore, no U.S. President can be from Alaska.
A)Deductive
B)Inductive
All U.S. Presidents have come from the original 48 states. Therefore, no U.S. President can be from Alaska.
A)Deductive
B)Inductive

Unlock Deck
Unlock for access to all 190 flashcards in this deck.
Unlock Deck
k this deck
13
Decide whether the argument is an example of inductive or deductive reasoning.
Every coach knows his sport well. John Madden is a football coach. Therefore John Madden knows football well.
A)Deductive
B)Inductive
Every coach knows his sport well. John Madden is a football coach. Therefore John Madden knows football well.
A)Deductive
B)Inductive

Unlock Deck
Unlock for access to all 190 flashcards in this deck.
Unlock Deck
k this deck
14
Determine the most probable next term in the sequence.
0005, 0050, 0500, 5000, 5005
A)5006
B)5050
C)5500
D)5000
0005, 0050, 0500, 5000, 5005
A)5006
B)5050
C)5500
D)5000

Unlock Deck
Unlock for access to all 190 flashcards in this deck.
Unlock Deck
k this deck
15
Use inductive reasoning to predict the next equation.
10 × 12 = 11 × 13 - 23 12 × 14 = 13 × 15 - 27
A)14 × 16 = 17 × 27 - 31
B)14 × 16 = 15 × 17 + 29
C)14 × 16 = 15 × 17 - 31
D)14 × 16 = 15 × 17 - 29
10 × 12 = 11 × 13 - 23 12 × 14 = 13 × 15 - 27
A)14 × 16 = 17 × 27 - 31
B)14 × 16 = 15 × 17 + 29
C)14 × 16 = 15 × 17 - 31
D)14 × 16 = 15 × 17 - 29

Unlock Deck
Unlock for access to all 190 flashcards in this deck.
Unlock Deck
k this deck
16
Decide whether the argument is an example of inductive or deductive reasoning.
5 + 31 = 36, 17 + 5 = 22, 17 + 37 = 54. Therefore, the sum of two prime numbers is even.
A)Inductive
B)Deductive
5 + 31 = 36, 17 + 5 = 22, 17 + 37 = 54. Therefore, the sum of two prime numbers is even.
A)Inductive
B)Deductive

Unlock Deck
Unlock for access to all 190 flashcards in this deck.
Unlock Deck
k this deck
17
Determine the most probable next term in the sequence.
486, 162, 54, 18, 6
486, 162, 54, 18, 6


Unlock Deck
Unlock for access to all 190 flashcards in this deck.
Unlock Deck
k this deck
18
Decide whether the argument is an example of inductive or deductive reasoning.
Fresh fruit costs more in winter. This is January. Therefore these fresh strawberries will cost more.
A)Deductive
B)Inductive
Fresh fruit costs more in winter. This is January. Therefore these fresh strawberries will cost more.
A)Deductive
B)Inductive

Unlock Deck
Unlock for access to all 190 flashcards in this deck.
Unlock Deck
k this deck
19
Determine the most probable next term in the sequence.



Unlock Deck
Unlock for access to all 190 flashcards in this deck.
Unlock Deck
k this deck
20
Determine the most probable next term in the sequence.
1, 4, 1, 4, 4, 1, 4, 4, 4, 1, 4, 4, 4, 4, 1, 4, 4, 4, 4, 4
1, 4, 1, 4, 4, 1, 4, 4, 4, 1, 4, 4, 4, 4, 1, 4, 4, 4, 4, 4


Unlock Deck
Unlock for access to all 190 flashcards in this deck.
Unlock Deck
k this deck
21
Use the method of Gauss to find the sum.
4 + 8 + 12 + . . . + 500
A)31,500
B)31,250
C)3906.25
D)250,000
4 + 8 + 12 + . . . + 500
A)31,500
B)31,250
C)3906.25
D)250,000

Unlock Deck
Unlock for access to all 190 flashcards in this deck.
Unlock Deck
k this deck
22
Use the method of Gauss to find the sum.
1 + 2 + 3 + . . . + 375
A)35,156.25
B)141,376
C)70,312.5
D)70,500
1 + 2 + 3 + . . . + 375
A)35,156.25
B)141,376
C)70,312.5
D)70,500

Unlock Deck
Unlock for access to all 190 flashcards in this deck.
Unlock Deck
k this deck
23
Use the method of Gauss to find the sum.
1 + 2 + 3 + . . . + 650
A)105,625
B)211,575
C)422,500
D)211,250
1 + 2 + 3 + . . . + 650
A)105,625
B)211,575
C)422,500
D)211,250

Unlock Deck
Unlock for access to all 190 flashcards in this deck.
Unlock Deck
k this deck
24
Use the method of Gauss to find the sum.
2 + 4 + 6 + . . . + 400
A)161,604
B)40,200
C)40,000
D)10,000
2 + 4 + 6 + . . . + 400
A)161,604
B)40,200
C)40,000
D)10,000

Unlock Deck
Unlock for access to all 190 flashcards in this deck.
Unlock Deck
k this deck
25
Find a pattern and use it to solve the problem.
Find the next term: 1, 1, 2, 3, 5, 8, 13
A)16
B)21
C)15
D)18
Find the next term: 1, 1, 2, 3, 5, 8, 13
A)16
B)21
C)15
D)18

Unlock Deck
Unlock for access to all 190 flashcards in this deck.
Unlock Deck
k this deck
26
Use inductive reasoning to predict the next equation.
37,037 × 3 = 111,111 37,037 × 6 = 222,222
37,037 × 9 = 333,333
37,037 × 12 = 444,444
A)37,037 × 15 = 555,555
B)37,037 × 18 = 666,666
C)111,111 × 15 = 1,666,665
D)37,037 × 13 = 481,481
37,037 × 3 = 111,111 37,037 × 6 = 222,222
37,037 × 9 = 333,333
37,037 × 12 = 444,444
A)37,037 × 15 = 555,555
B)37,037 × 18 = 666,666
C)111,111 × 15 = 1,666,665
D)37,037 × 13 = 481,481

Unlock Deck
Unlock for access to all 190 flashcards in this deck.
Unlock Deck
k this deck
27
Use the method of Gauss to find the sum.
1 + 2 + 3 + . . . + 1000
A)500,500
B)500,000
C)250,000
D)1,002,001
1 + 2 + 3 + . . . + 1000
A)500,500
B)500,000
C)250,000
D)1,002,001

Unlock Deck
Unlock for access to all 190 flashcards in this deck.
Unlock Deck
k this deck
28
Find a pattern and use it to solve the problem.
Find the next term: T, F, S, E, T, T, F
A)S
B)F
C)T
D)E
Find the next term: T, F, S, E, T, T, F
A)S
B)F
C)T
D)E

Unlock Deck
Unlock for access to all 190 flashcards in this deck.
Unlock Deck
k this deck
29
Use inductive reasoning to predict the next equation.
(4 × 1)x (2 × 1)= 8 (4 × 10)x (2 × 2)= 160
(4 × 100)x (2 × 3)= 2400
A)(4 × 1000)x (2 × 4)= 36,000
B)(4 × 1000)x (2 × 4)= 3200
C)(4 × 1000)x (2 × 4)= 28,000
D)(4 × 1000)x (2 × 4)= 32,000
(4 × 1)x (2 × 1)= 8 (4 × 10)x (2 × 2)= 160
(4 × 100)x (2 × 3)= 2400
A)(4 × 1000)x (2 × 4)= 36,000
B)(4 × 1000)x (2 × 4)= 3200
C)(4 × 1000)x (2 × 4)= 28,000
D)(4 × 1000)x (2 × 4)= 32,000

Unlock Deck
Unlock for access to all 190 flashcards in this deck.
Unlock Deck
k this deck
30
Use inductive reasoning to predict the next equation.
9 × 10 = 11 × 12 - (9 + 10 + 11 + 12) 10 × 11 = 12 × 13 - (10 + 11 + 12 + 13)
A)12 × 13 = 14 × 15 - (11 + 10 + 9 + 8)
B)12 × 13 = 14 × 15 - (12 + 13 + 14 + 15)
C)11 × 12 = 13 × 14 - (9 + 10 + 11 + 12 + 13 + 14)
D)11 × 12 = 13 × 14 - (11 + 12 + 13 + 14)
9 × 10 = 11 × 12 - (9 + 10 + 11 + 12) 10 × 11 = 12 × 13 - (10 + 11 + 12 + 13)
A)12 × 13 = 14 × 15 - (11 + 10 + 9 + 8)
B)12 × 13 = 14 × 15 - (12 + 13 + 14 + 15)
C)11 × 12 = 13 × 14 - (9 + 10 + 11 + 12 + 13 + 14)
D)11 × 12 = 13 × 14 - (11 + 12 + 13 + 14)

Unlock Deck
Unlock for access to all 190 flashcards in this deck.
Unlock Deck
k this deck
31
Use inductive reasoning to predict the next equation.
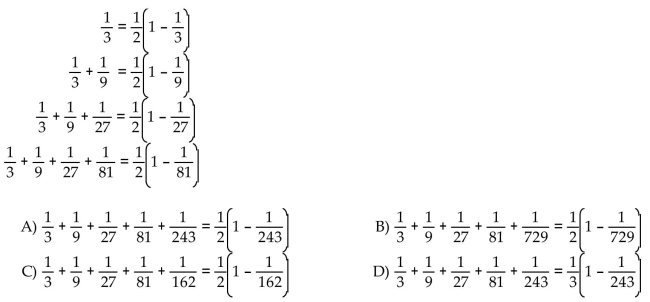
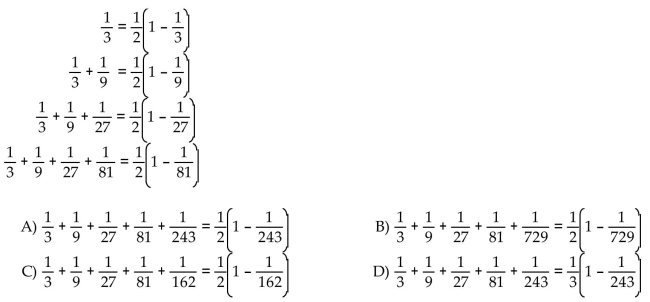

Unlock Deck
Unlock for access to all 190 flashcards in this deck.
Unlock Deck
k this deck
32
Use inductive reasoning to predict the next equation.
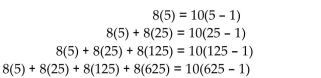

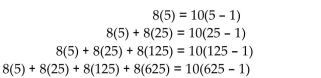


Unlock Deck
Unlock for access to all 190 flashcards in this deck.
Unlock Deck
k this deck
33
Find a pattern and use it to solve the problem.
Find the next term: 5, 10, 15, 25
A)30
B)35
C)50
D)55
Find the next term: 5, 10, 15, 25
A)30
B)35
C)50
D)55

Unlock Deck
Unlock for access to all 190 flashcards in this deck.
Unlock Deck
k this deck
34
How many triangles are in the next figure? 


Unlock Deck
Unlock for access to all 190 flashcards in this deck.
Unlock Deck
k this deck
35
Find a pattern and use it to solve the problem.
Find the next term: S, S, M, T, W
A)S
B)T
C)F
D)M
Find the next term: S, S, M, T, W
A)S
B)T
C)F
D)M

Unlock Deck
Unlock for access to all 190 flashcards in this deck.
Unlock Deck
k this deck
36
Use inductive reasoning to predict the next equation.
(1 × 9)- 7 = 2 (21 × 9)- 7 = 182
(321 × 9)- 7 = 2882
A)(4321 × 9)- 7 = 3881
B)(4321 × 9)- 7 = 28,882
C)(432 × 9)- 7 = 38,882
D)(4321 × 9)- 7 = 38,882
(1 × 9)- 7 = 2 (21 × 9)- 7 = 182
(321 × 9)- 7 = 2882
A)(4321 × 9)- 7 = 3881
B)(4321 × 9)- 7 = 28,882
C)(432 × 9)- 7 = 38,882
D)(4321 × 9)- 7 = 38,882

Unlock Deck
Unlock for access to all 190 flashcards in this deck.
Unlock Deck
k this deck
37
Find a pattern and use it to solve the problem.
Find the next term: 21, 4, 17, 6, 11, 8
A)0
B)19
C)3
D)7
Find the next term: 21, 4, 17, 6, 11, 8
A)0
B)19
C)3
D)7

Unlock Deck
Unlock for access to all 190 flashcards in this deck.
Unlock Deck
k this deck
38
How many line segments are used in the next figure? 


Unlock Deck
Unlock for access to all 190 flashcards in this deck.
Unlock Deck
k this deck
39
Use inductive reasoning to predict the next equation.
9 × 9 = 81 99 × 99 = 9801
999 × 999 = 998,001
A)9999 × 9999 = 999,001
B)999 × 9999 = 99,980,001
C)9999 × 9999 = 1,000,001
D)9999 × 9999 = 99,980,001
9 × 9 = 81 99 × 99 = 9801
999 × 999 = 998,001
A)9999 × 9999 = 999,001
B)999 × 9999 = 99,980,001
C)9999 × 9999 = 1,000,001
D)9999 × 9999 = 99,980,001

Unlock Deck
Unlock for access to all 190 flashcards in this deck.
Unlock Deck
k this deck
40
Find a pattern and use it to solve the problem.
Find the next term: 2, 3, 5, 7, 11, 13
A)17
B)16
C)19
D)15
Find the next term: 2, 3, 5, 7, 11, 13
A)17
B)16
C)19
D)15

Unlock Deck
Unlock for access to all 190 flashcards in this deck.
Unlock Deck
k this deck
41
Determine if the sequence is an arithmetic sequence, a geometric sequence, or neither. If it is either arithmetic or
geometric, give the next term in the sequence.
10, 13, 16, 19, 22, . . .
A)neither
B)arithmetic; 25
C)geometric; 25
geometric, give the next term in the sequence.
10, 13, 16, 19, 22, . . .
A)neither
B)arithmetic; 25
C)geometric; 25

Unlock Deck
Unlock for access to all 190 flashcards in this deck.
Unlock Deck
k this deck
42
Use the method of successive differences to determine the next term in the sequence.
4, 18, 90, 264, 605, 1199, . . .
A)2143
B)2132
C)2174
D)2153
4, 18, 90, 264, 605, 1199, . . .
A)2143
B)2132
C)2174
D)2153

Unlock Deck
Unlock for access to all 190 flashcards in this deck.
Unlock Deck
k this deck
43
Determine what the next equation would be, and verify that it is indeed a true statement.
11 × 11 = 121 101 × 101 = 10,201
A)1001 × 1001 = 101,101
B)1001 × 101 = 1,002,001
C)1001 × 1001 = 100,200
D)1001 × 1001 = 1,002,001
11 × 11 = 121 101 × 101 = 10,201
A)1001 × 1001 = 101,101
B)1001 × 101 = 1,002,001
C)1001 × 1001 = 100,200
D)1001 × 1001 = 1,002,001

Unlock Deck
Unlock for access to all 190 flashcards in this deck.
Unlock Deck
k this deck
44
Determine if the sequence is an arithmetic sequence, a geometric sequence, or neither. If it is either arithmetic or
geometric, give the next term in the sequence.
224, 112, 56, 28, 14, . . .
A)neither
B)geometric; 7
C)arithmetic; 7
geometric, give the next term in the sequence.
224, 112, 56, 28, 14, . . .
A)neither
B)geometric; 7
C)arithmetic; 7

Unlock Deck
Unlock for access to all 190 flashcards in this deck.
Unlock Deck
k this deck
45
Determine if the sequence is an arithmetic sequence, a geometric sequence, or neither. If it is either arithmetic or
geometric, give the next term in the sequence.
6, 12, 18, 30, 48, . . .
A)neither
B)geometric; 78
C)arithmetic; 78
geometric, give the next term in the sequence.
6, 12, 18, 30, 48, . . .
A)neither
B)geometric; 78
C)arithmetic; 78

Unlock Deck
Unlock for access to all 190 flashcards in this deck.
Unlock Deck
k this deck
46
Draw the next figure in the pattern. 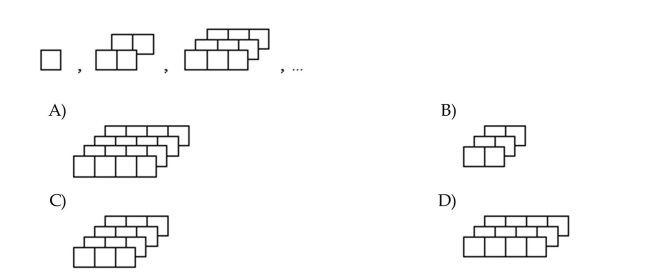


Unlock Deck
Unlock for access to all 190 flashcards in this deck.
Unlock Deck
k this deck
47
Determine what the next equation would be, and verify that it is indeed a true statement.
40 - 9 = 31 400 - 89 = 311
4000 - 789 = 3211
A)400,000 - 6,789 = 33,211
B)40,000 - 6,789 = 393,211
C)4000 - 6,789 = 33,211
D)40,000 - 6,789 = 33,211
40 - 9 = 31 400 - 89 = 311
4000 - 789 = 3211
A)400,000 - 6,789 = 33,211
B)40,000 - 6,789 = 393,211
C)4000 - 6,789 = 33,211
D)40,000 - 6,789 = 33,211

Unlock Deck
Unlock for access to all 190 flashcards in this deck.
Unlock Deck
k this deck
48
Determine if the sequence is an arithmetic sequence, a geometric sequence, or neither. If it is either arithmetic or
geometric, give the next term in the sequence.
7, 13, 13, 19, 19, . . .
A)arithmetic; 25
B)geometric; 25
C)neither
geometric, give the next term in the sequence.
7, 13, 13, 19, 19, . . .
A)arithmetic; 25
B)geometric; 25
C)neither

Unlock Deck
Unlock for access to all 190 flashcards in this deck.
Unlock Deck
k this deck
49
Draw the next figure in the pattern. 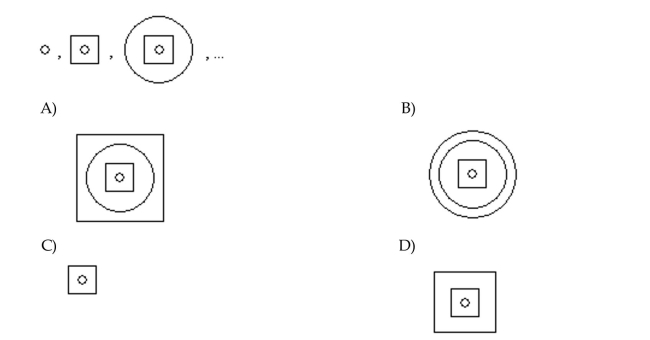


Unlock Deck
Unlock for access to all 190 flashcards in this deck.
Unlock Deck
k this deck
50
Determine if the sequence is an arithmetic sequence, a geometric sequence, or neither. If it is either arithmetic or
geometric, give the next term in the sequence.
2, 5, 10, 17, 26, . . .
A)arithmetic; 37
B)neither
C)geometric; 37
geometric, give the next term in the sequence.
2, 5, 10, 17, 26, . . .
A)arithmetic; 37
B)neither
C)geometric; 37

Unlock Deck
Unlock for access to all 190 flashcards in this deck.
Unlock Deck
k this deck
51
Determine if the sequence is an arithmetic sequence, a geometric sequence, or neither. If it is either arithmetic or
geometric, give the next term in the sequence.
4, 12, 36, 108, 324, . . .
A)arithmetic; 972
B)geometric; 972
C)neither
geometric, give the next term in the sequence.
4, 12, 36, 108, 324, . . .
A)arithmetic; 972
B)geometric; 972
C)neither

Unlock Deck
Unlock for access to all 190 flashcards in this deck.
Unlock Deck
k this deck
52
Determine what the next equation would be, and verify that it is indeed a true statement.
15 + 51 = 66 16 + 61 = 77
A)17 + 71 = 102
B)71 + 17 = 88
C)17 + 71 = 88
D)55 + 33 = 88
15 + 51 = 66 16 + 61 = 77
A)17 + 71 = 102
B)71 + 17 = 88
C)17 + 71 = 88
D)55 + 33 = 88

Unlock Deck
Unlock for access to all 190 flashcards in this deck.
Unlock Deck
k this deck
53
Use the method of successive differences to determine the next term in the sequence.
8, 25, 79, 170, 298, . . .
A)499
B)426
C)500
D)463
8, 25, 79, 170, 298, . . .
A)499
B)426
C)500
D)463

Unlock Deck
Unlock for access to all 190 flashcards in this deck.
Unlock Deck
k this deck
54
Use the method of successive differences to determine the next term in the sequence.
20, 31, 45, 62, 82, . . .
A)107
B)102
C)108
D)105
20, 31, 45, 62, 82, . . .
A)107
B)102
C)108
D)105

Unlock Deck
Unlock for access to all 190 flashcards in this deck.
Unlock Deck
k this deck
55
Determine if the sequence is an arithmetic sequence, a geometric sequence, or neither. If it is either arithmetic or
geometric, give the next term in the sequence.
0, 5, 10, 20, 30, . . .
A)geometric; 40
B)arithmetic; 40
C)neither
geometric, give the next term in the sequence.
0, 5, 10, 20, 30, . . .
A)geometric; 40
B)arithmetic; 40
C)neither

Unlock Deck
Unlock for access to all 190 flashcards in this deck.
Unlock Deck
k this deck
56
Use the method of successive differences to determine the next term in the sequence.
8, 17, 35, 64, 106, 163, . . .
A)239
B)246
C)237
D)235
8, 17, 35, 64, 106, 163, . . .
A)239
B)246
C)237
D)235

Unlock Deck
Unlock for access to all 190 flashcards in this deck.
Unlock Deck
k this deck
57
Determine what the next equation would be, and verify that it is indeed a true statement.
(6 × 1)× (2 × 1)= 12 (6 × 10)× (2 × 2)= 240
(6 × 100)× (2 × 3)= 3600
A)(6 × 1000)× (2 × 4)= 42,000
B)(6 × 1000)× (2 × 4)= 4800
C)(6 × 1000)× (2 × 4)= 54,000
D)(6 × 1000)× (2 × 4)= 48,000
(6 × 1)× (2 × 1)= 12 (6 × 10)× (2 × 2)= 240
(6 × 100)× (2 × 3)= 3600
A)(6 × 1000)× (2 × 4)= 42,000
B)(6 × 1000)× (2 × 4)= 4800
C)(6 × 1000)× (2 × 4)= 54,000
D)(6 × 1000)× (2 × 4)= 48,000

Unlock Deck
Unlock for access to all 190 flashcards in this deck.
Unlock Deck
k this deck
58
Determine what the next equation would be, and verify that it is indeed a true statement.
32 + 10 = 42 43 + 21 = 64
54 + 32 = 86
A)56 + 43 = 99
B)64 + 53 = 117
C)65 + 43 = 108
D)65 + 32 = 97
32 + 10 = 42 43 + 21 = 64
54 + 32 = 86
A)56 + 43 = 99
B)64 + 53 = 117
C)65 + 43 = 108
D)65 + 32 = 97

Unlock Deck
Unlock for access to all 190 flashcards in this deck.
Unlock Deck
k this deck
59
Determine if the sequence is an arithmetic sequence, a geometric sequence, or neither. If it is either arithmetic or
geometric, give the next term in the sequence.
9, 29, 49, 69, 89, . . .
A)geometric; 109
B)neither
C)arithmetic; 109
geometric, give the next term in the sequence.
9, 29, 49, 69, 89, . . .
A)geometric; 109
B)neither
C)arithmetic; 109

Unlock Deck
Unlock for access to all 190 flashcards in this deck.
Unlock Deck
k this deck
60
Determine what the next equation would be, and verify that it is indeed a true statement.
(1 × 9)- 8 = 1 (21 × 9)- 8 = 81
(321 × 9)- 8 = 2881
A)(4,321 × 9)- 8 = 38,881
B)(432 × 9)- 8 = 38,881
C)(4,321 × 9)- 8 = 28,881
D)(4,321 × 9)- 8 = 3880
(1 × 9)- 8 = 1 (21 × 9)- 8 = 81
(321 × 9)- 8 = 2881
A)(4,321 × 9)- 8 = 38,881
B)(432 × 9)- 8 = 38,881
C)(4,321 × 9)- 8 = 28,881
D)(4,321 × 9)- 8 = 3880

Unlock Deck
Unlock for access to all 190 flashcards in this deck.
Unlock Deck
k this deck
61
Use the indicated formula to find the sum.
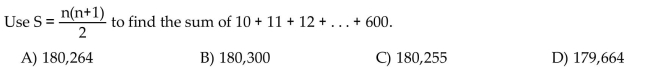
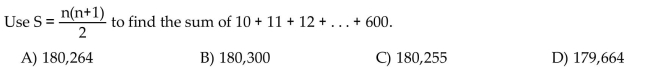

Unlock Deck
Unlock for access to all 190 flashcards in this deck.
Unlock Deck
k this deck
62
Solve the problem using inductive reasoning.
How many line segments are determined by joining dots on the last two circles?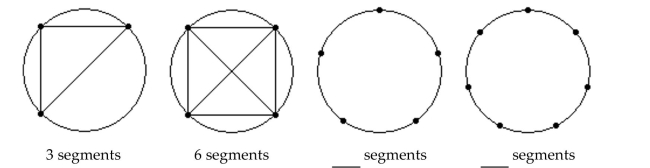
How many line segments are determined by joining dots on the last two circles?


Unlock Deck
Unlock for access to all 190 flashcards in this deck.
Unlock Deck
k this deck
63
Use the indicated formula to find the sum.
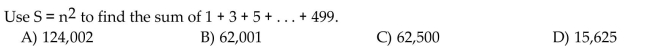
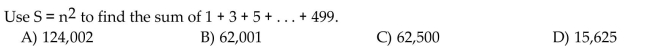

Unlock Deck
Unlock for access to all 190 flashcards in this deck.
Unlock Deck
k this deck
64
Use the indicated formula to find the sum.
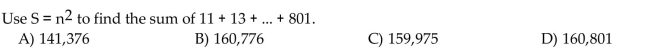
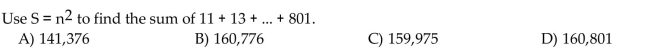

Unlock Deck
Unlock for access to all 190 flashcards in this deck.
Unlock Deck
k this deck
65
Use the appropriate formula to find the indicated figurate number.
the 14th hexagonal number
A)
the 14th hexagonal number
A)


Unlock Deck
Unlock for access to all 190 flashcards in this deck.
Unlock Deck
k this deck
66
Determine what the next equation would be, and verify that it is indeed a true statement.
2 × 4 = 3 × 5 - 7 4 × 6 = 5 × 7 - 11
A)6 × 8 = 7 × 9 - 15
B)6 × 8 = 7 × 9 - 13
C)6 × 8 = 7 × 9 + 13
D)6 × 8 = 9 × 11 - 15
2 × 4 = 3 × 5 - 7 4 × 6 = 5 × 7 - 11
A)6 × 8 = 7 × 9 - 15
B)6 × 8 = 7 × 9 - 13
C)6 × 8 = 7 × 9 + 13
D)6 × 8 = 9 × 11 - 15

Unlock Deck
Unlock for access to all 190 flashcards in this deck.
Unlock Deck
k this deck
67
Use the appropriate formula to find the indicated figurate number.
the 8th square number
A)
the 8th square number
A)


Unlock Deck
Unlock for access to all 190 flashcards in this deck.
Unlock Deck
k this deck
68
Use the indicated formula to find the sum.
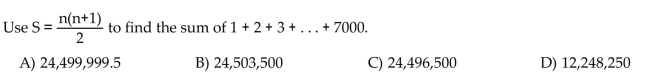
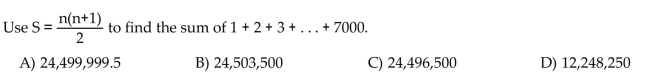

Unlock Deck
Unlock for access to all 190 flashcards in this deck.
Unlock Deck
k this deck
69
Use the indicated formula to find the sum.
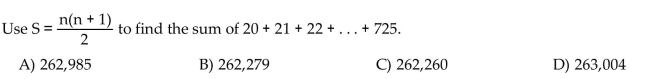
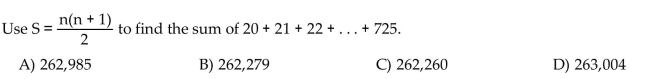

Unlock Deck
Unlock for access to all 190 flashcards in this deck.
Unlock Deck
k this deck
70
Solve the problem using inductive reasoning.
Find the number of games played in a round robin tournament for the given numbers of
teams. In a round robin tournament every team plays every other team once.
Find the number of games played in a round robin tournament for the given numbers of
teams. In a round robin tournament every team plays every other team once.


Unlock Deck
Unlock for access to all 190 flashcards in this deck.
Unlock Deck
k this deck
71
Use the appropriate formula to find the indicated figurate number.
the 8th heptagonal number
A)
the 8th heptagonal number
A)


Unlock Deck
Unlock for access to all 190 flashcards in this deck.
Unlock Deck
k this deck
72
Use the indicated formula to find the sum.
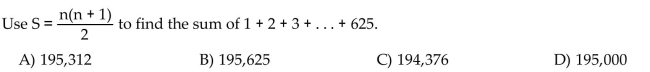
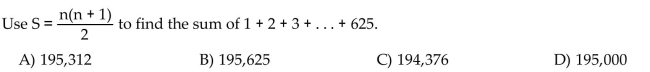

Unlock Deck
Unlock for access to all 190 flashcards in this deck.
Unlock Deck
k this deck
73
Solve the problem using inductive reasoning.
Write the next three "square" figurate numbers.
Write the next three "square" figurate numbers.


Unlock Deck
Unlock for access to all 190 flashcards in this deck.
Unlock Deck
k this deck
74
Use the appropriate formula to find the indicated figurate number.
the 6th pentagonal number
A)
the 6th pentagonal number
A)


Unlock Deck
Unlock for access to all 190 flashcards in this deck.
Unlock Deck
k this deck
75
Use the appropriate formula to find the indicated figurate number.
the 15th octagonal number
A)
the 15th octagonal number
A)


Unlock Deck
Unlock for access to all 190 flashcards in this deck.
Unlock Deck
k this deck
76
Determine what the next equation would be, and verify that it is indeed a true statement.
7 × 8 = 9 × 10 - (7 + 8 + 9 + 10) 8 × 9 = 10 × 11 - (8 + 9 + 10 + 11)
A)10 × 11 = 12 × 13 - (10 + 11 + 12 + 13)
B)9 × 10 = 11 × 12 - (9 + 10 + 11 + 12)
C)9 × 10 = 11 × 12 - (7 + 8 + 9 + 10 + 11 + 12)
D)10 × 11 = 12 × 13 - (9 + 8 + 7 + 6)
7 × 8 = 9 × 10 - (7 + 8 + 9 + 10) 8 × 9 = 10 × 11 - (8 + 9 + 10 + 11)
A)10 × 11 = 12 × 13 - (10 + 11 + 12 + 13)
B)9 × 10 = 11 × 12 - (9 + 10 + 11 + 12)
C)9 × 10 = 11 × 12 - (7 + 8 + 9 + 10 + 11 + 12)
D)10 × 11 = 12 × 13 - (9 + 8 + 7 + 6)

Unlock Deck
Unlock for access to all 190 flashcards in this deck.
Unlock Deck
k this deck
77
Use the indicated formula to find the sum.
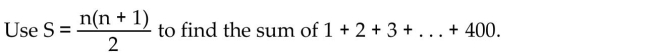
A)8020
B)80,200
C)79,800
D)80,000
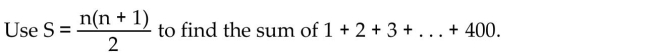
A)8020
B)80,200
C)79,800
D)80,000

Unlock Deck
Unlock for access to all 190 flashcards in this deck.
Unlock Deck
k this deck
78
Determine what the next equation would be, and verify that it is indeed a true statement.
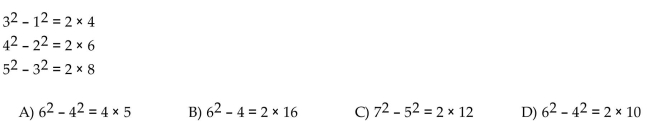
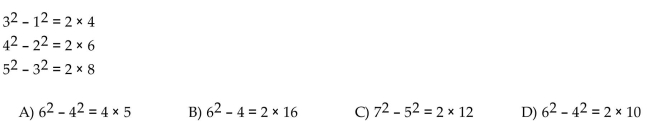

Unlock Deck
Unlock for access to all 190 flashcards in this deck.
Unlock Deck
k this deck
79
Use the appropriate formula to find the indicated figurate number.
the 6th triangular number
A)
the 6th triangular number
A)


Unlock Deck
Unlock for access to all 190 flashcards in this deck.
Unlock Deck
k this deck
80
Use the indicated formula to find the sum.
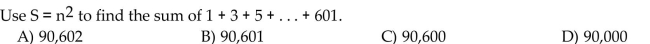
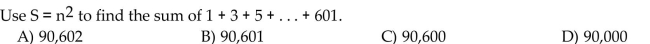

Unlock Deck
Unlock for access to all 190 flashcards in this deck.
Unlock Deck
k this deck



