Deck 5: Eigenvalues and Eigenvectors
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/20
Play
Full screen (f)
Deck 5: Eigenvalues and Eigenvectors
1
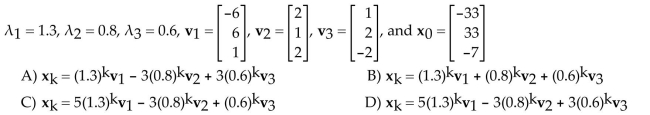
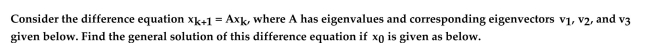
C
2
Solve the initial value problem.
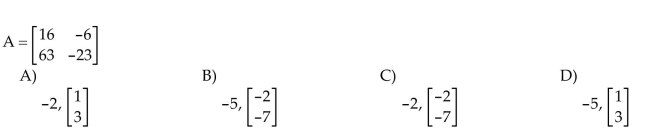
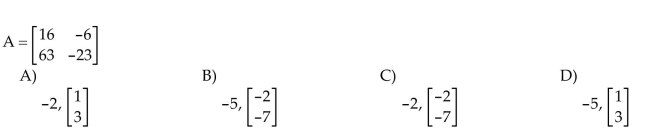
A
3
For the given matrix A, find a basis for the corresponding eigenspace for the given eigenvalue.
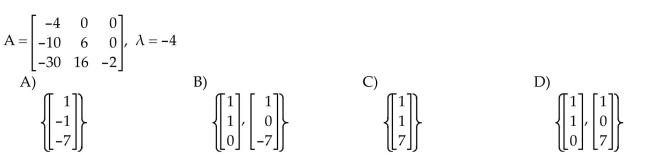

C
4
The characteristic polynomial of a 5 × 5 matrix is given below. Find the eigenvalues and their multiplicities.



Unlock Deck
Unlock for access to all 20 flashcards in this deck.
Unlock Deck
k this deck
5
Diagonalize the matrix A, if possible. That is, find an invertible matrix P and a diagonal matrix D such that
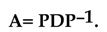
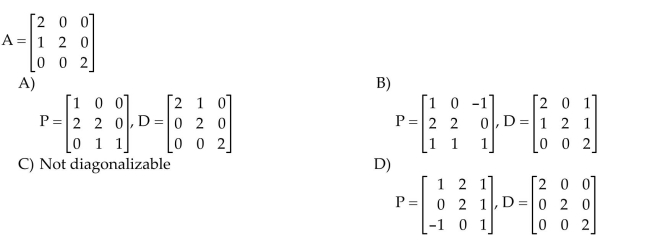
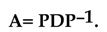
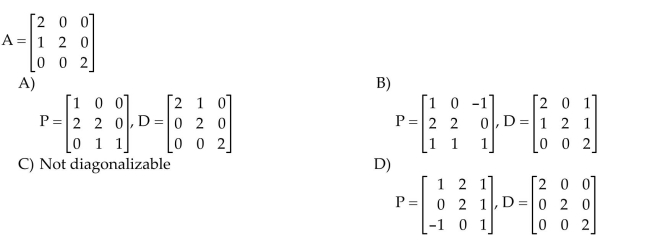

Unlock Deck
Unlock for access to all 20 flashcards in this deck.
Unlock Deck
k this deck
6
Find the eigenvalues of the given matrix.
 )6
)6
 )6
)6
Unlock Deck
Unlock for access to all 20 flashcards in this deck.
Unlock Deck
k this deck
7
Find the characteristic equation of the given matrix.
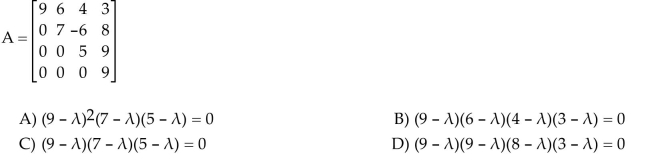
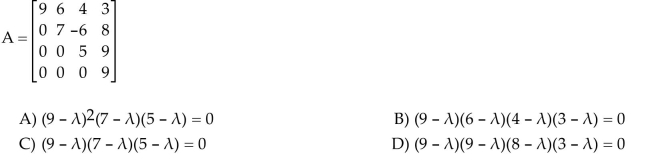

Unlock Deck
Unlock for access to all 20 flashcards in this deck.
Unlock Deck
k this deck
8
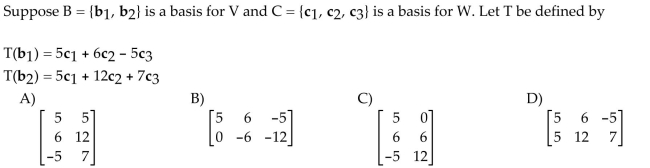

Unlock Deck
Unlock for access to all 20 flashcards in this deck.
Unlock Deck
k this deck
9
Find the characteristic equation of the given matrix.
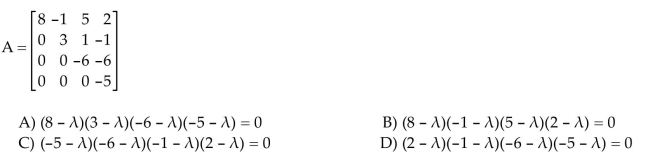
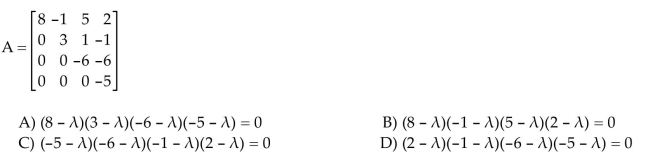

Unlock Deck
Unlock for access to all 20 flashcards in this deck.
Unlock Deck
k this deck
10
The characteristic polynomial of a 5 × 5 matrix is given below. Find the eigenvalues and their multiplicities.
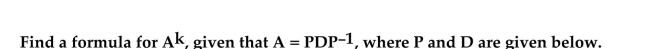
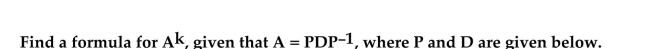

Unlock Deck
Unlock for access to all 20 flashcards in this deck.
Unlock Deck
k this deck
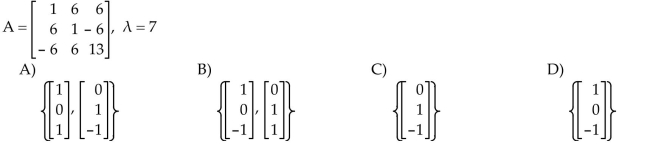
11
For the given matrix A, find a basis for the corresponding eigenspace for the given eigenvalue.


Unlock Deck
Unlock for access to all 20 flashcards in this deck.
Unlock Deck
k this deck
12
The characteristic polynomial of a 5 × 5 matrix is given below. Find the eigenvalues and their multiplicities.



Unlock Deck
Unlock for access to all 20 flashcards in this deck.
Unlock Deck
k this deck
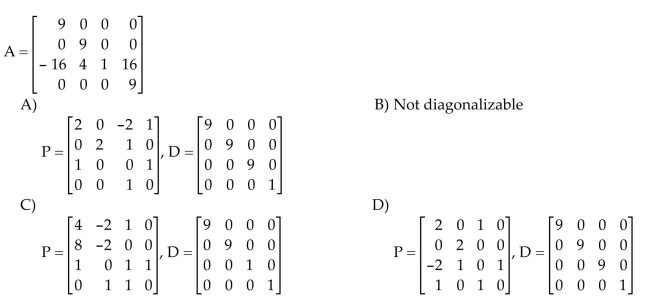
13
Diagonalize the matrix A, if possible. That is, find an invertible matrix P and a diagonal matrix D such that
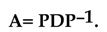
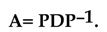


Unlock Deck
Unlock for access to all 20 flashcards in this deck.
Unlock Deck
k this deck
14
Diagonalize the matrix A, if possible. That is, find an invertible matrix P and a diagonal matrix D such that
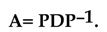
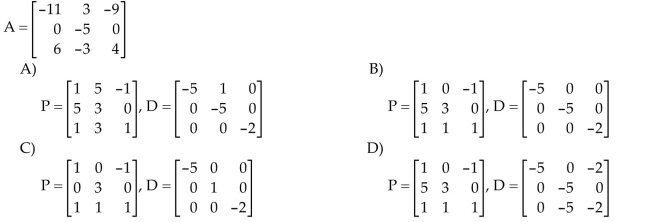
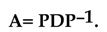
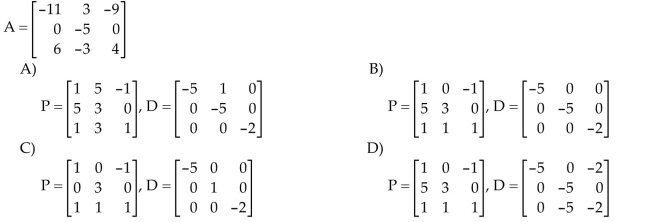

Unlock Deck
Unlock for access to all 20 flashcards in this deck.
Unlock Deck
k this deck
15
Use the inverse power method to determine the smallest eigenvalue of the matrix A.
Assume that the eigenvalues are roughly 1.3, 2.1, and 15.
Assume that the eigenvalues are roughly 1.3, 2.1, and 15.


Unlock Deck
Unlock for access to all 20 flashcards in this deck.
Unlock Deck
k this deck
16
Find the eigenvalues of the given matrix.



Unlock Deck
Unlock for access to all 20 flashcards in this deck.
Unlock Deck
k this deck
17
For the given matrix and eigenvalue, find an eigenvector corresponding to the eigenvalue.
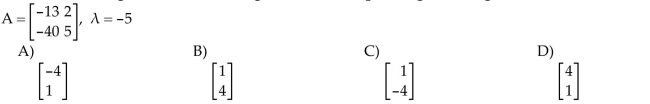
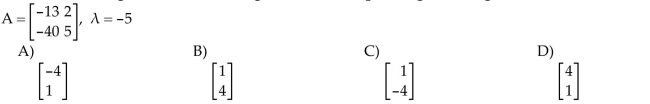

Unlock Deck
Unlock for access to all 20 flashcards in this deck.
Unlock Deck
k this deck
18
For the given matrix and eigenvalue, find an eigenvector corresponding to the eigenvalue.



Unlock Deck
Unlock for access to all 20 flashcards in this deck.
Unlock Deck
k this deck
19
Diagonalize the matrix A, if possible. That is, find an invertible matrix P and a diagonal matrix D such that
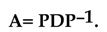
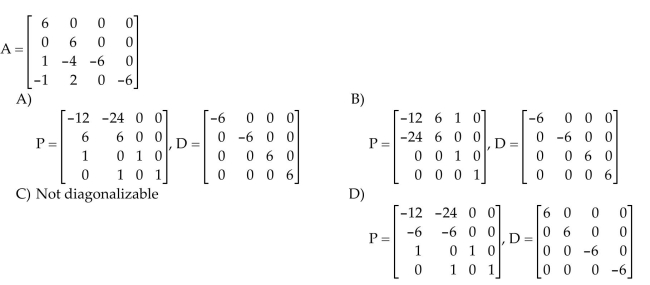
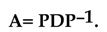

Unlock Deck
Unlock for access to all 20 flashcards in this deck.
Unlock Deck
k this deck
20
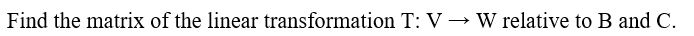
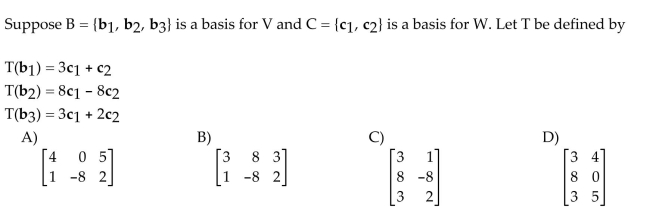

Unlock Deck
Unlock for access to all 20 flashcards in this deck.
Unlock Deck
k this deck



