Deck 10: Topics In Analytic Geometry
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/556
Play
Full screen (f)
Deck 10: Topics In Analytic Geometry
1
Find a polar equation of the conic with its focus at the pole.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)
2
Select the polar equation of the conic for e = 1.0 and identify the conic for the following equation.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)
3
Find a polar equation of the conic with its focus at the pole.
Conics Eccentricity Directrix
Ellipse
A)
B)
C)
D)
E)
Conics Eccentricity Directrix
Ellipse
A)
B)
C)
D)
E)
4
Find a polar equation of the conic with its focus at the pole.
Conics Eccentricity Directrix
Ellipse
A)
B)
C)
D)
E)
Conics Eccentricity Directrix
Ellipse
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 556 flashcards in this deck.
Unlock Deck
k this deck
5
Identify the conic and select its correct graph.
A) Ellipse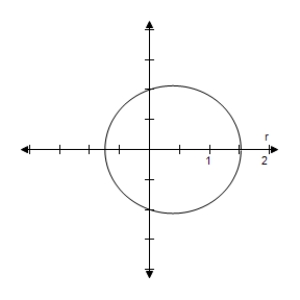
B) Ellipse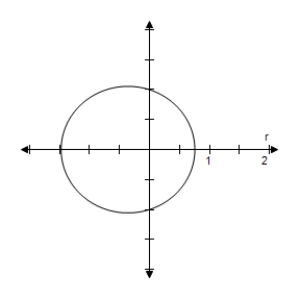
C) Ellipse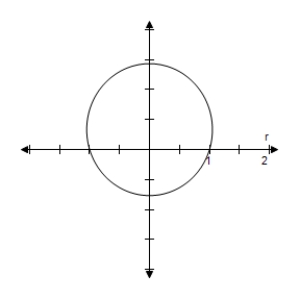
D) Ellipse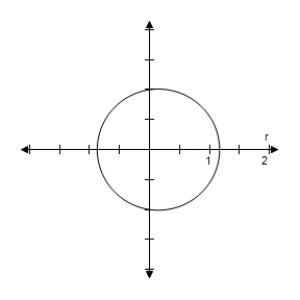
E) Ellipse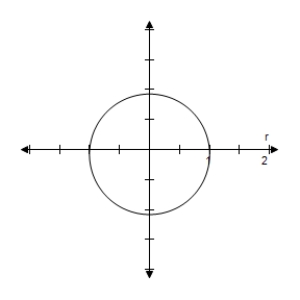
A) Ellipse

B) Ellipse

C) Ellipse

D) Ellipse

E) Ellipse


Unlock Deck
Unlock for access to all 556 flashcards in this deck.
Unlock Deck
k this deck
6
Find a polar equation of the conic with its focus at the pole.
Conics Eccentricity Directrix
Ellipse
A)
B)
C)
D)
E)
Conics Eccentricity Directrix
Ellipse
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 556 flashcards in this deck.
Unlock Deck
k this deck
7
Identify the conic and select its correct graph.
A) Hyperbola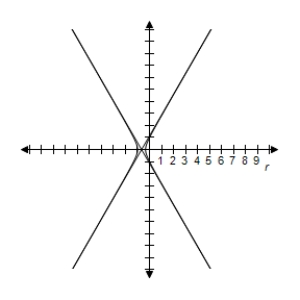
B) Hyperbola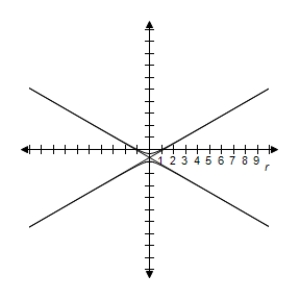
C) Hyperbola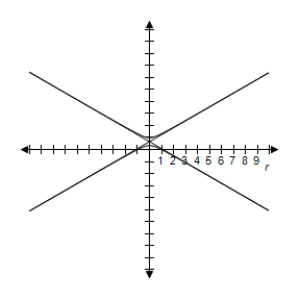
D) Hyperbola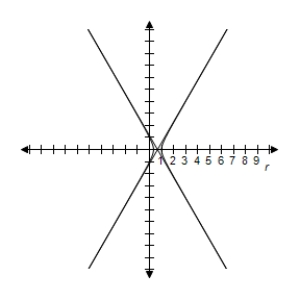
E) Hyperbola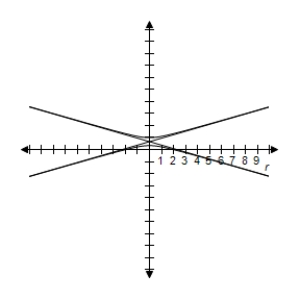
A) Hyperbola

B) Hyperbola

C) Hyperbola

D) Hyperbola

E) Hyperbola


Unlock Deck
Unlock for access to all 556 flashcards in this deck.
Unlock Deck
k this deck
8
Identify the conic and select its correct graph.
A) Parabola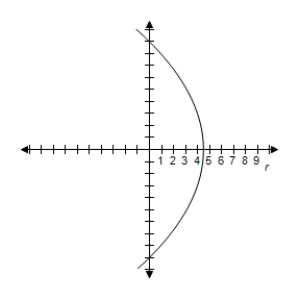
B) Parabola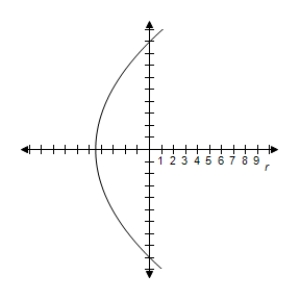
C) Hyperbola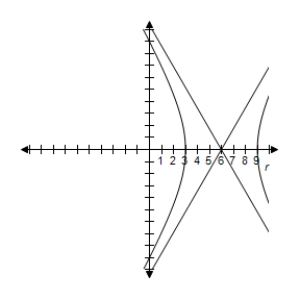
D) Parabola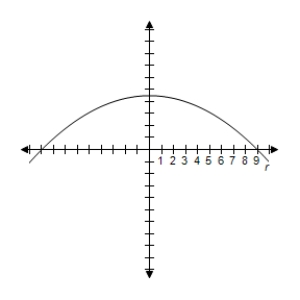
E) Parabola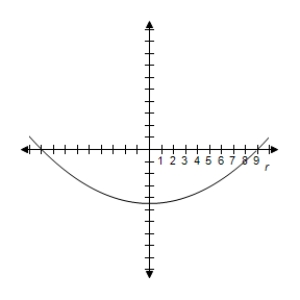
A) Parabola

B) Parabola

C) Hyperbola

D) Parabola

E) Parabola


Unlock Deck
Unlock for access to all 556 flashcards in this deck.
Unlock Deck
k this deck
9
Identify the conic and select its correct graph.
A) Parabola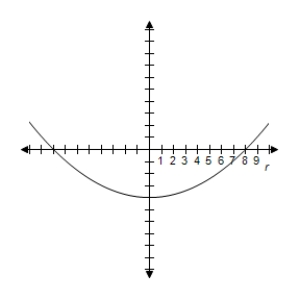
B) Parabola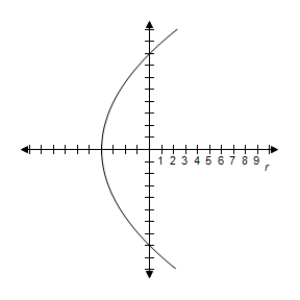
C) Hyperbola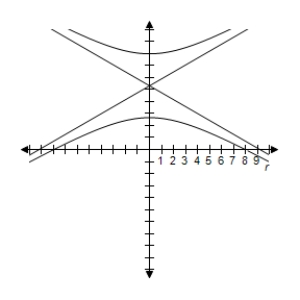
D) Parabola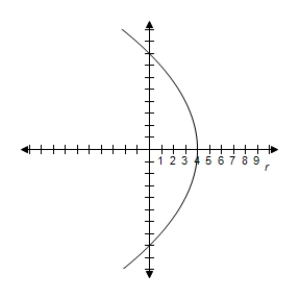
E) Parabola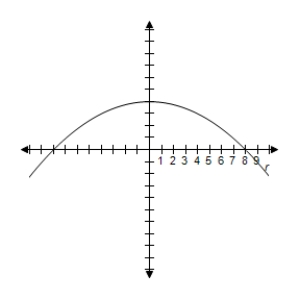
A) Parabola

B) Parabola

C) Hyperbola

D) Parabola

E) Parabola


Unlock Deck
Unlock for access to all 556 flashcards in this deck.
Unlock Deck
k this deck
10
Select the polar equation of the conic for e = 1.0 and identify the conic for the following equation.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 556 flashcards in this deck.
Unlock Deck
k this deck
11
Identify the conic and select its correct graph.
A) Parabola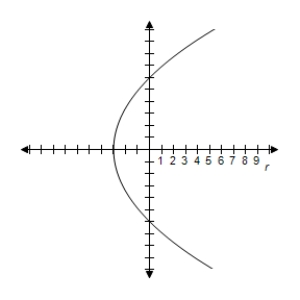
B) Parabola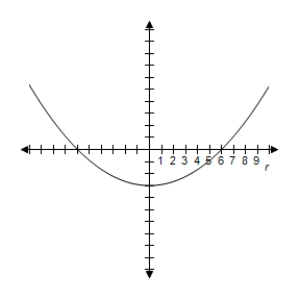
C) Hyperbola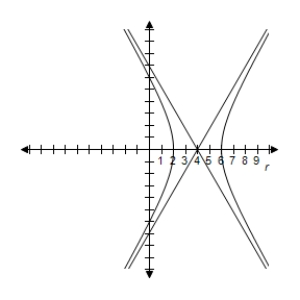
D) Parabola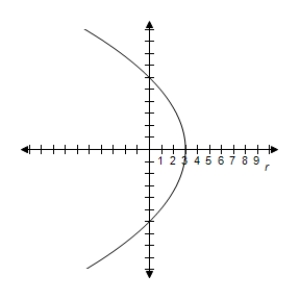
E) Parabola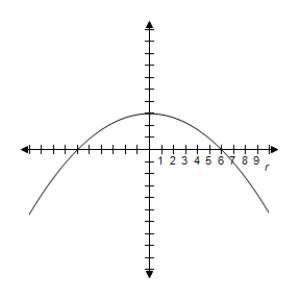
A) Parabola

B) Parabola

C) Hyperbola

D) Parabola

E) Parabola


Unlock Deck
Unlock for access to all 556 flashcards in this deck.
Unlock Deck
k this deck
12
Find a polar equation of the conic with its focus at the pole.
Conics Eccentricity Directrix
Ellipse
A)
B)
C)
D)
E)
Conics Eccentricity Directrix
Ellipse
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 556 flashcards in this deck.
Unlock Deck
k this deck
13
Find a polar equation of the conic with its focus at the pole.
Conics Eccentricity Directrix
Ellipse
A)
B)
C)
D)
E)
Conics Eccentricity Directrix
Ellipse
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 556 flashcards in this deck.
Unlock Deck
k this deck
14
Identify the conic and select its correct graph.
A) Ellipse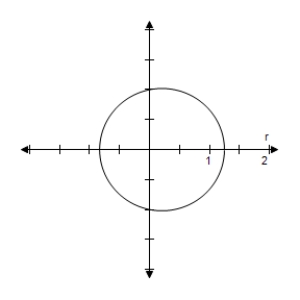
B) Ellipse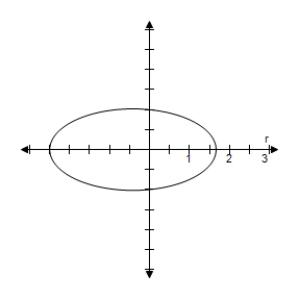
C) Ellipse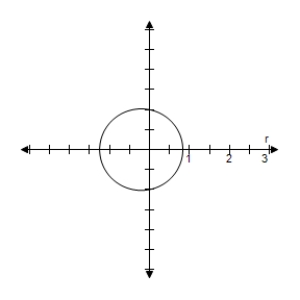
D) Ellipse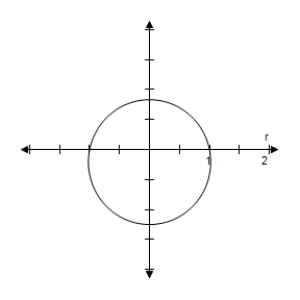
E) Ellipse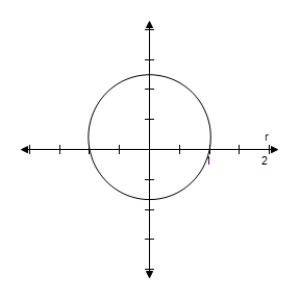
A) Ellipse

B) Ellipse

C) Ellipse

D) Ellipse

E) Ellipse


Unlock Deck
Unlock for access to all 556 flashcards in this deck.
Unlock Deck
k this deck
15
Identify the conic and select its correct graph.
A) Ellipse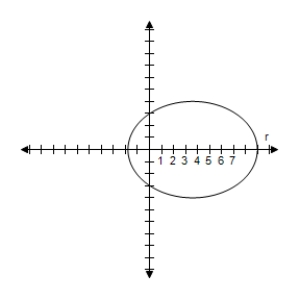
B) Ellipse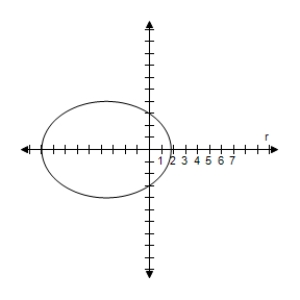
C) Ellipse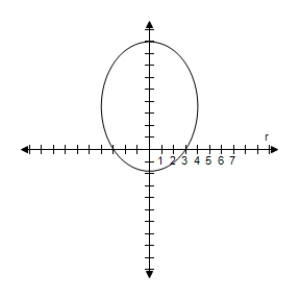
D) Ellipse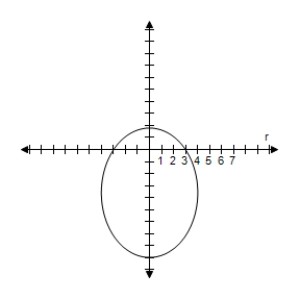
E) Ellipse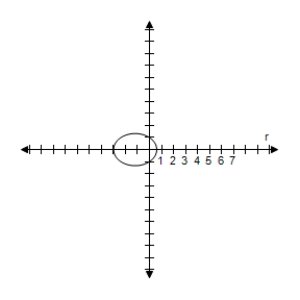
A) Ellipse

B) Ellipse

C) Ellipse

D) Ellipse

E) Ellipse


Unlock Deck
Unlock for access to all 556 flashcards in this deck.
Unlock Deck
k this deck
16
Identify the conic and select its correct graph.
A) Hyperbola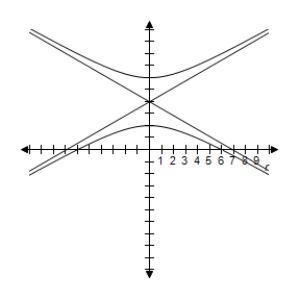
B) Parabola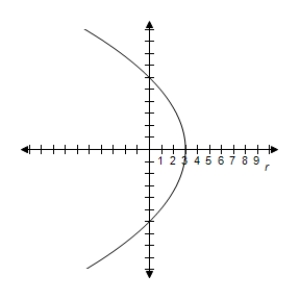
C) Parabola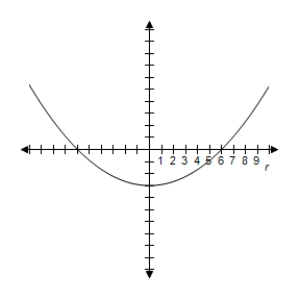
D) Parabola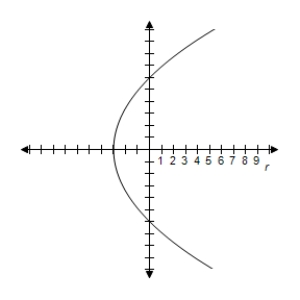
E) Parabola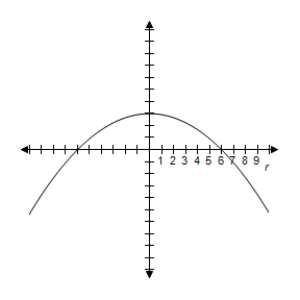
A) Hyperbola

B) Parabola

C) Parabola

D) Parabola

E) Parabola


Unlock Deck
Unlock for access to all 556 flashcards in this deck.
Unlock Deck
k this deck
17
Select the polar equation of the conic for e = 1.0 and identify the conic for the following equation.
A)
B)
C)
D)
E) .
A)
B)
C)
D)
E) .

Unlock Deck
Unlock for access to all 556 flashcards in this deck.
Unlock Deck
k this deck
18
Identify the conic and select its correct graph.
A) Hyperbola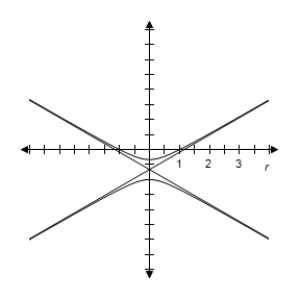
B) Hyperbola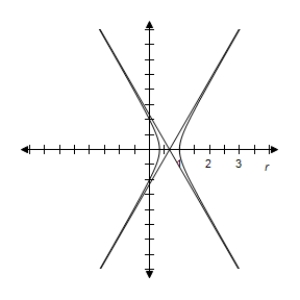
C) Hyperbola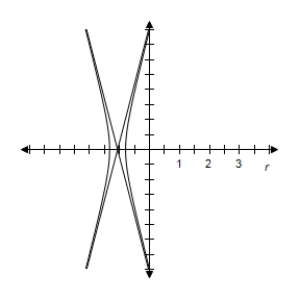
D) Hyperbola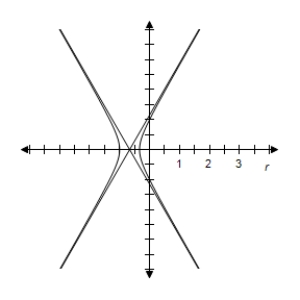
E) Hyperbola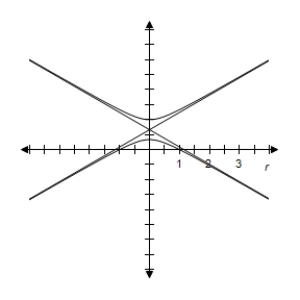
A) Hyperbola

B) Hyperbola

C) Hyperbola

D) Hyperbola

E) Hyperbola


Unlock Deck
Unlock for access to all 556 flashcards in this deck.
Unlock Deck
k this deck
19
Select the polar equation of the conic for e = 0.75 and identify the conic for the following equation.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 556 flashcards in this deck.
Unlock Deck
k this deck
20
Identify the conic and select its correct graph.
A) Hyperbola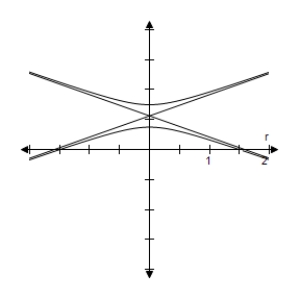
B) Hyperbola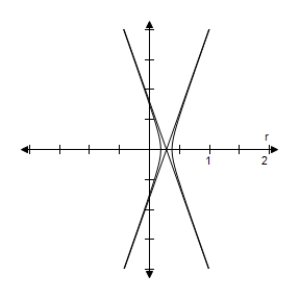
C) Hyperbola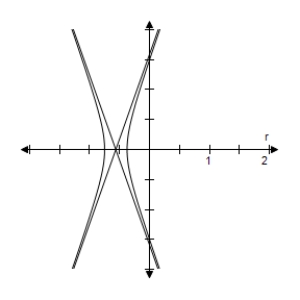
D) Hyperbola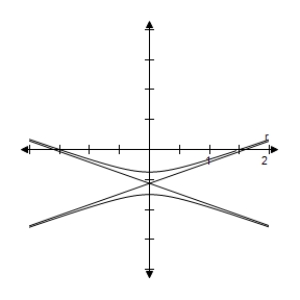
E) Hyperbola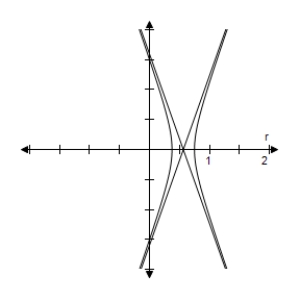
A) Hyperbola

B) Hyperbola

C) Hyperbola

D) Hyperbola

E) Hyperbola


Unlock Deck
Unlock for access to all 556 flashcards in this deck.
Unlock Deck
k this deck
21
Find a polar equation of the conic with its focus at the pole.
Conics Vertex or vertices
Parabola
A)
B)
C)
D)
E)
Conics Vertex or vertices
Parabola
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 556 flashcards in this deck.
Unlock Deck
k this deck
22
Select the polar equation of graph. 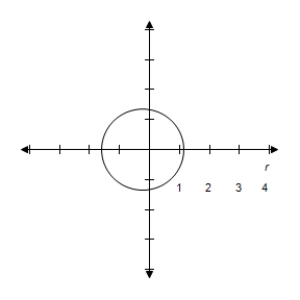
A)
B)
C)
D)
E)

A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 556 flashcards in this deck.
Unlock Deck
k this deck
23
Select the polar equation of graph. 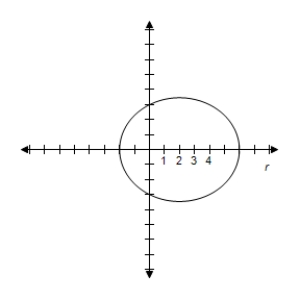
A)
B)
C)
D)
E)

A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 556 flashcards in this deck.
Unlock Deck
k this deck
24
Select correct graph to graph rotated conic.
A)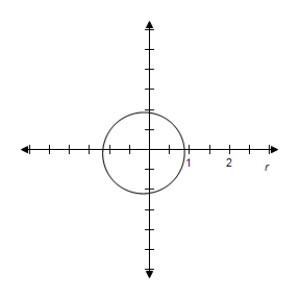
B)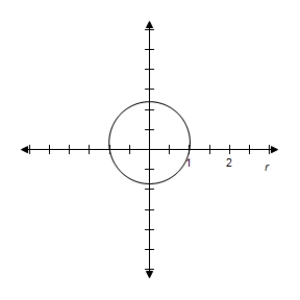
C)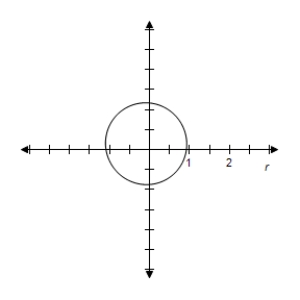
D)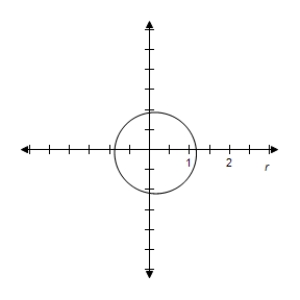
E)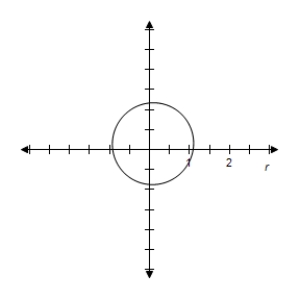
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 556 flashcards in this deck.
Unlock Deck
k this deck
25
Find a polar equation of the conic with its focus at the pole.
Conics Vertex or vertices
Parabola
A)
B)
C)
D)
E)
Conics Vertex or vertices
Parabola
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 556 flashcards in this deck.
Unlock Deck
k this deck
26
Identify the conic and select its correct graph.
A) Hyperbola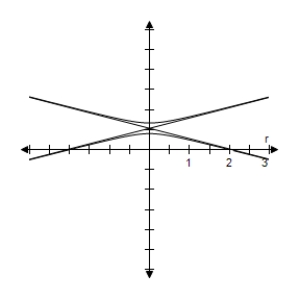
B) Hyperbola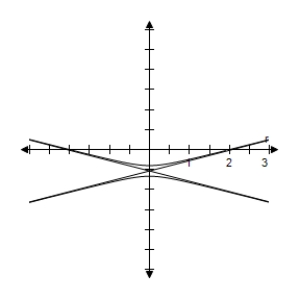
C) Hyperbola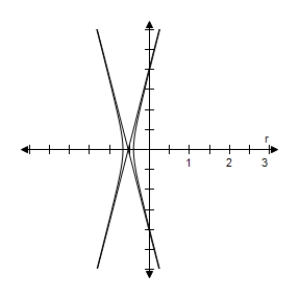
D) Hyperbola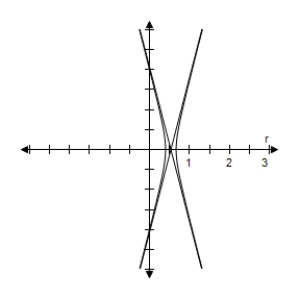
E) Hyperbola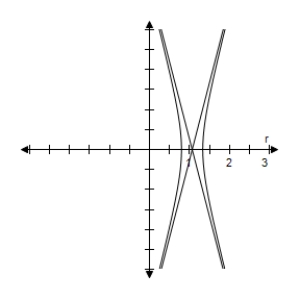
A) Hyperbola

B) Hyperbola

C) Hyperbola

D) Hyperbola

E) Hyperbola


Unlock Deck
Unlock for access to all 556 flashcards in this deck.
Unlock Deck
k this deck
27
Select the polar equation with graph. 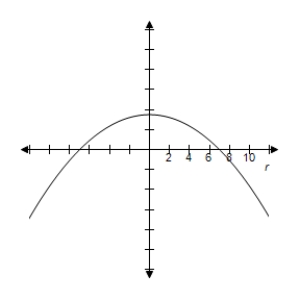
A)
B)
C)
D)
E)

A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 556 flashcards in this deck.
Unlock Deck
k this deck
28
Identify the conic and select its correct graph.
A) Hyperbola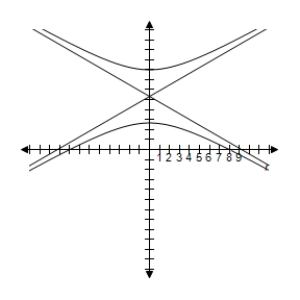
B) Ellipse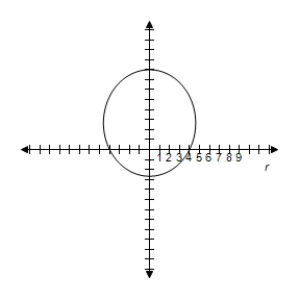
C) Hyperbola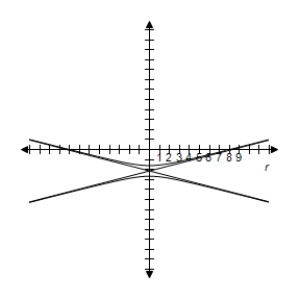
D) Ellipse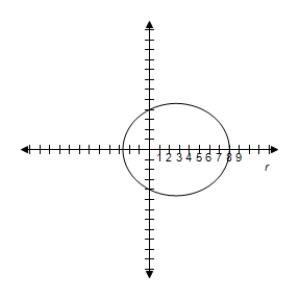
E) Hyperbola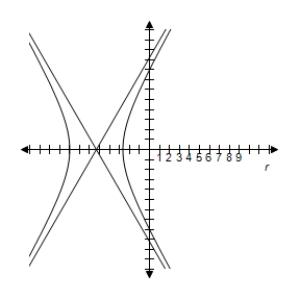
A) Hyperbola

B) Ellipse

C) Hyperbola

D) Ellipse

E) Hyperbola


Unlock Deck
Unlock for access to all 556 flashcards in this deck.
Unlock Deck
k this deck
29
Find a polar equation of the conic with its focus at the pole.
Conics Vertex or vertices
Parabola
A)
B)
C)
D)
E)
Conics Vertex or vertices
Parabola
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 556 flashcards in this deck.
Unlock Deck
k this deck
30
Select correct graph to graph rotated conic.
A)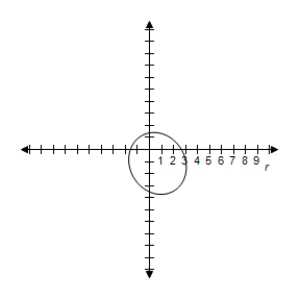
B)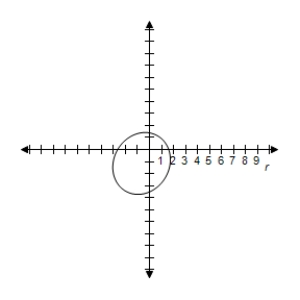
C)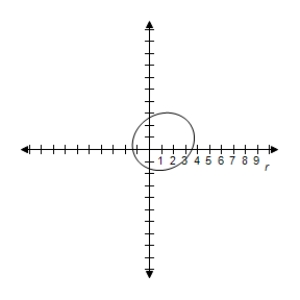
D)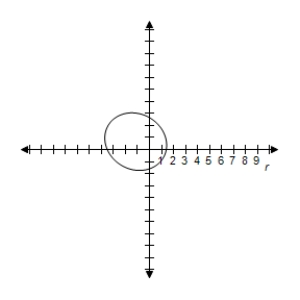
E)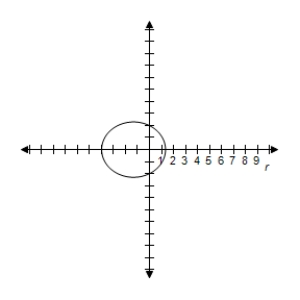
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 556 flashcards in this deck.
Unlock Deck
k this deck
31
Select correct graph to graph rotated conic.
A)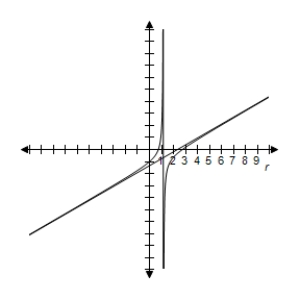
B)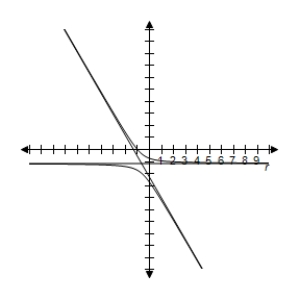
C)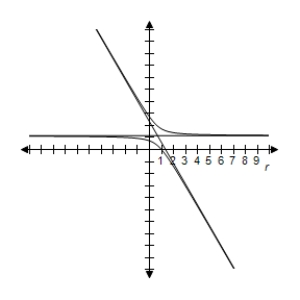
D)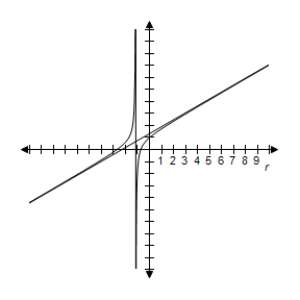
E)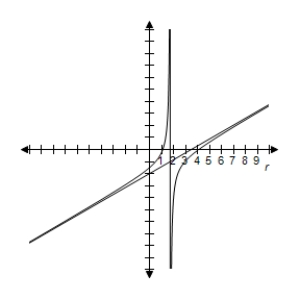
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 556 flashcards in this deck.
Unlock Deck
k this deck
32
By using a graphing utility select the correct graph of the polar equation.Identify the graph.
A)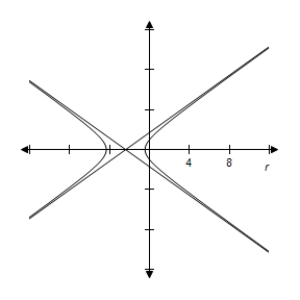 Hyperbola
Hyperbola
B)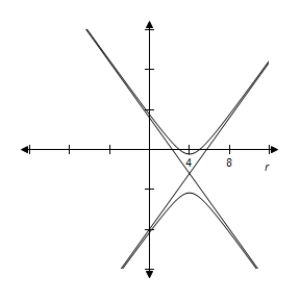 Hyperbola
Hyperbola
C)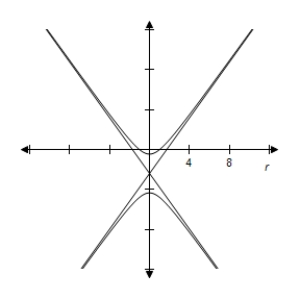 Hyperbola
Hyperbola
D)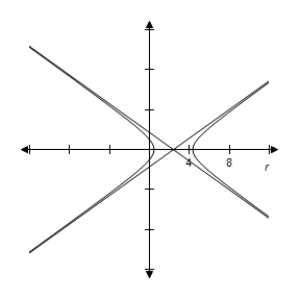 Hyperbola
Hyperbola
E)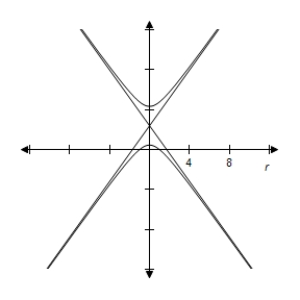 Hyperbola
Hyperbola
A)
 Hyperbola
HyperbolaB)
 Hyperbola
HyperbolaC)
 Hyperbola
HyperbolaD)
 Hyperbola
HyperbolaE)
 Hyperbola
Hyperbola
Unlock Deck
Unlock for access to all 556 flashcards in this deck.
Unlock Deck
k this deck
33
Select the polar equation of graph. 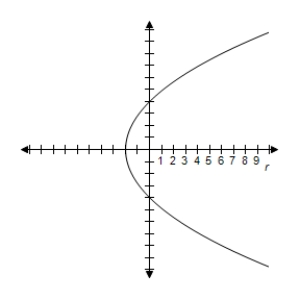
A)
B)
C)
D)
E)

A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 556 flashcards in this deck.
Unlock Deck
k this deck
34
By using a graphing utility select the correct graph of the polar equation.Identify the graph.
A)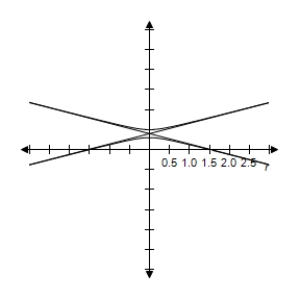 Hyperbola
Hyperbola
B)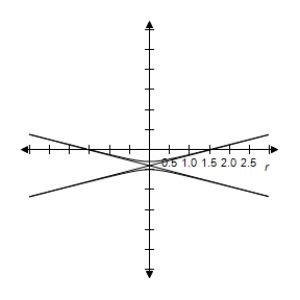 Hyperbola
Hyperbola
C)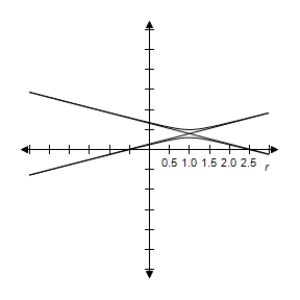 Hyperbola
Hyperbola
D)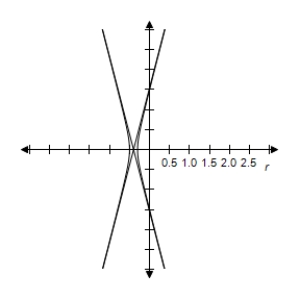 Hyperbola
Hyperbola
E)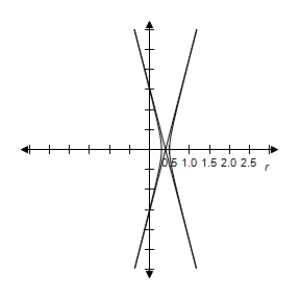 Hyperbola
Hyperbola
A)
 Hyperbola
HyperbolaB)
 Hyperbola
HyperbolaC)
 Hyperbola
HyperbolaD)
 Hyperbola
HyperbolaE)
 Hyperbola
Hyperbola
Unlock Deck
Unlock for access to all 556 flashcards in this deck.
Unlock Deck
k this deck
35
Find a polar equation of the conic with its focus at the pole.
Conics Vertex or vertices
Parabola ( 4,0 )
A)
B)
C)
D)
E)
Conics Vertex or vertices
Parabola ( 4,0 )
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 556 flashcards in this deck.
Unlock Deck
k this deck
36
Select correct graph to graph rotated conic.
A)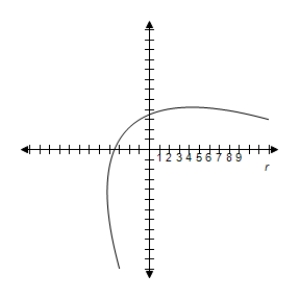
B)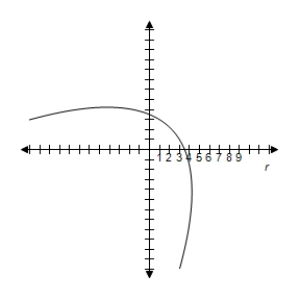
C)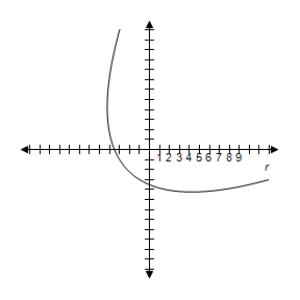
D)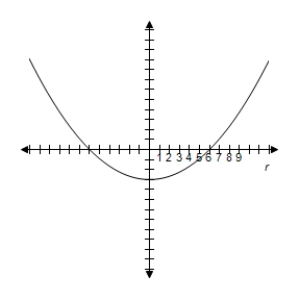
E)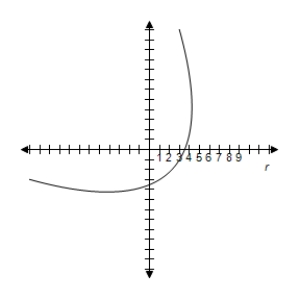
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 556 flashcards in this deck.
Unlock Deck
k this deck
37
Identify the conic and select its correct graph.
A) Hyperbola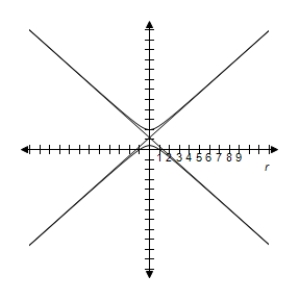
B) Hyperbola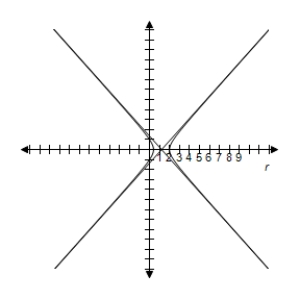
C) Hyperbola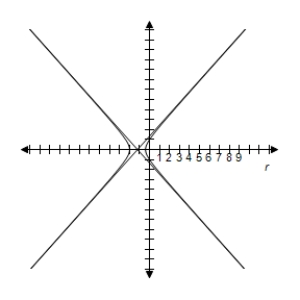
D) Hyperbola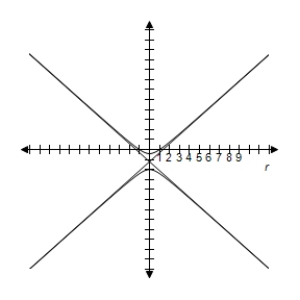
E) Hyperbola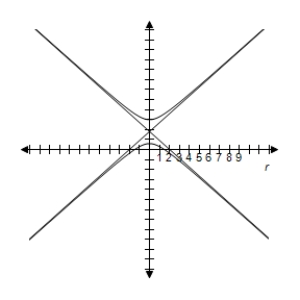
A) Hyperbola

B) Hyperbola

C) Hyperbola

D) Hyperbola

E) Hyperbola


Unlock Deck
Unlock for access to all 556 flashcards in this deck.
Unlock Deck
k this deck
38
Select the polar equation with graph. 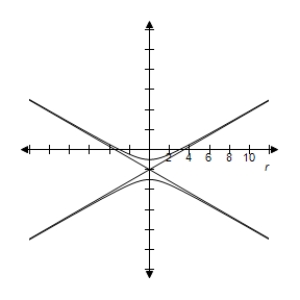
A)
B)
C)
D)
E)

A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 556 flashcards in this deck.
Unlock Deck
k this deck
39
By using a graphing utility select the correct graph of the polar equation.
A)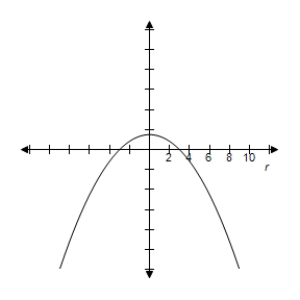
B)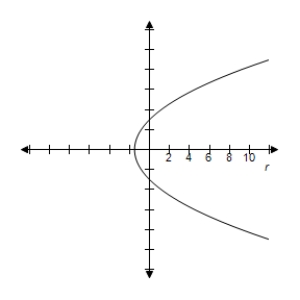
C)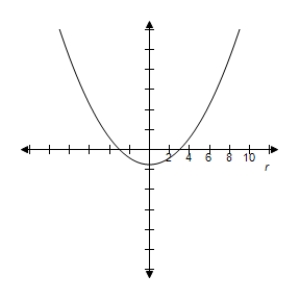
D)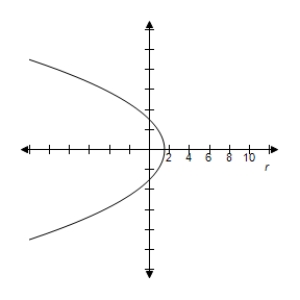
E)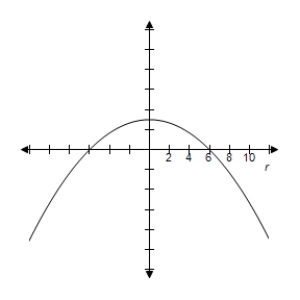
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 556 flashcards in this deck.
Unlock Deck
k this deck
40
Select the polar equation of graph. 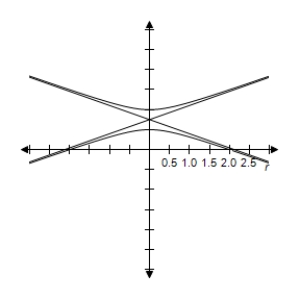
A)
B)
C)
D)
E)

A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 556 flashcards in this deck.
Unlock Deck
k this deck
41
A satellite in a 100-mile-high circular orbit around Earth has a velocity of approximately 17,500 miles per hour.If this velocity is multiplied by , the satellite will have the minimum velocity necessary to escape Earth's gravity and will follow a parabolic path with the center of Earth as the focus.(Hints: The radius of Earth is 4000 miles.) 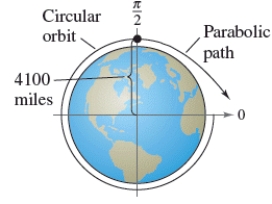 Find the distance between the surface of the Earth and the satellite when .
Find the distance between the surface of the Earth and the satellite when .
A)Distance between surface of Earth and satellite:4496 miles
B)Distance between surface of Earth and satellite:4322 miles
C)Distance between surface of Earth and satellite:1286 miles
D)Distance between surface of Earth and satellite:643 miles
E)Distance between surface of Earth and satellite:1492 miles
 Find the distance between the surface of the Earth and the satellite when .
Find the distance between the surface of the Earth and the satellite when .A)Distance between surface of Earth and satellite:4496 miles
B)Distance between surface of Earth and satellite:4322 miles
C)Distance between surface of Earth and satellite:1286 miles
D)Distance between surface of Earth and satellite:643 miles
E)Distance between surface of Earth and satellite:1492 miles

Unlock Deck
Unlock for access to all 556 flashcards in this deck.
Unlock Deck
k this deck
42
By using a graphing utility select the correct graph of the polar equation.Identify the graph.
A)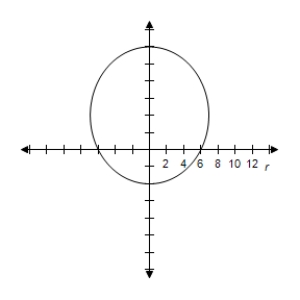 Ellipse
Ellipse
B)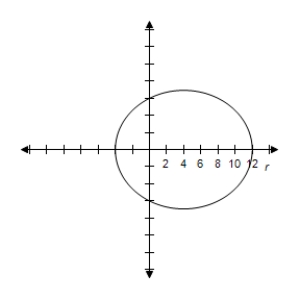 Ellipse
Ellipse
C)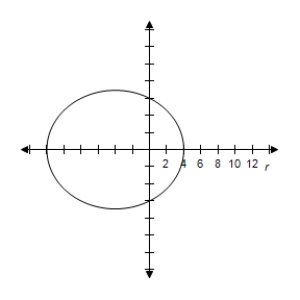 Ellipse
Ellipse
D)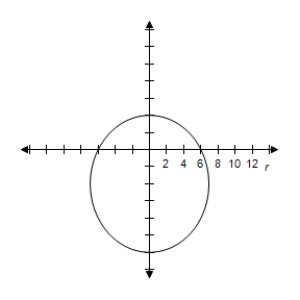 Ellipse
Ellipse
E)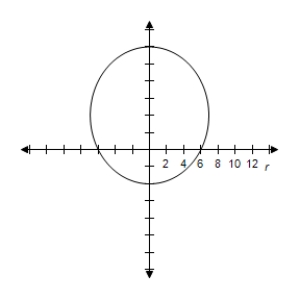 Ellipse
Ellipse
A)
 Ellipse
EllipseB)
 Ellipse
EllipseC)
 Ellipse
EllipseD)
 Ellipse
EllipseE)
 Ellipse
Ellipse
Unlock Deck
Unlock for access to all 556 flashcards in this deck.
Unlock Deck
k this deck
43
Select the graph of the polar equation using symmetry, zeros, maximum r-values, and any other additional points.
A)Symmetric with respect to polar axis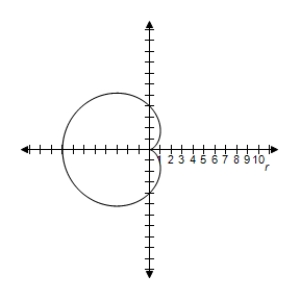
B)Symmetric with respect to polar axis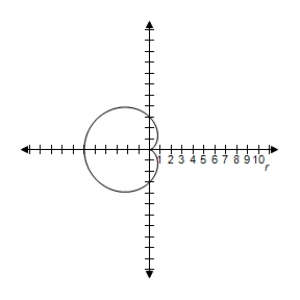
C)Symmetric with respect to polar axis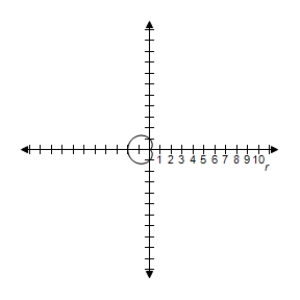
D)Symmetric with respect to polar axis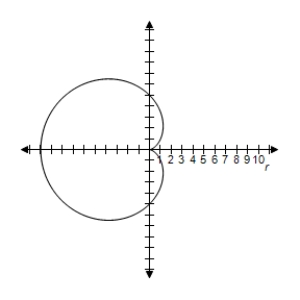
E)Symmetric with respect to polar axis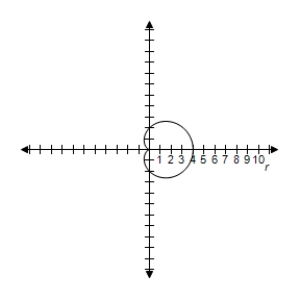
A)Symmetric with respect to polar axis

B)Symmetric with respect to polar axis

C)Symmetric with respect to polar axis

D)Symmetric with respect to polar axis

E)Symmetric with respect to polar axis


Unlock Deck
Unlock for access to all 556 flashcards in this deck.
Unlock Deck
k this deck
44
Find the polar equation of the planet's orbit and the perihelion and aphelion distances.
Earth miles
A) Perihelion distance: Aphelion distance:
B) Perihelion distance: Aphelion distance:
C) Perihelion distance: Aphelion distance:
D) Perihelion distance: Aphelion distance:
E) Perihelion distance: Aphelion distance:
Earth miles
A) Perihelion distance: Aphelion distance:
B) Perihelion distance: Aphelion distance:
C) Perihelion distance: Aphelion distance:
D) Perihelion distance: Aphelion distance:
E) Perihelion distance: Aphelion distance:

Unlock Deck
Unlock for access to all 556 flashcards in this deck.
Unlock Deck
k this deck
45
Select the graph of the polar equation using symmetry, zeros, maximum r-values, and any other additional points.
A)Symmetric with respect to , polar axis, poleCircle with radius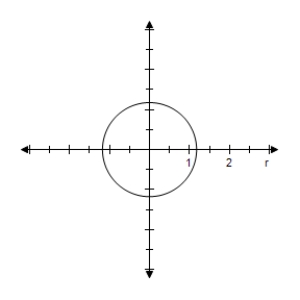
B)Symmetric with respect to , polar axis, poleCircle with radius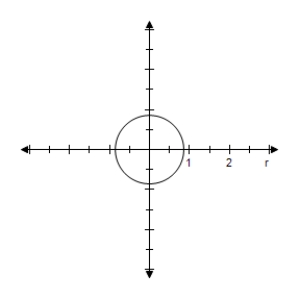
C)Symmetric with respect to , polar axis, poleCircle with radius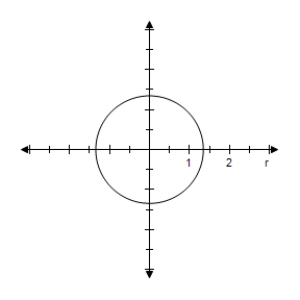
D)Symmetric with respect to , polar axis, poleCircle with radius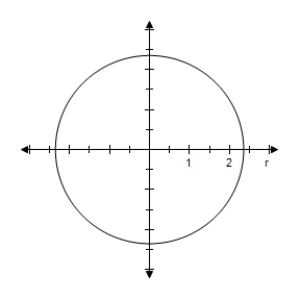
E)Symmetric with respect to , polar axis, poleCircle with radius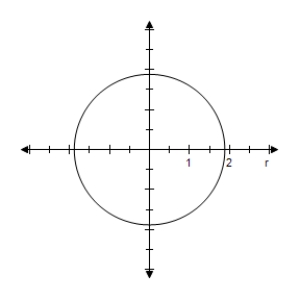
A)Symmetric with respect to , polar axis, poleCircle with radius

B)Symmetric with respect to , polar axis, poleCircle with radius

C)Symmetric with respect to , polar axis, poleCircle with radius

D)Symmetric with respect to , polar axis, poleCircle with radius

E)Symmetric with respect to , polar axis, poleCircle with radius


Unlock Deck
Unlock for access to all 556 flashcards in this deck.
Unlock Deck
k this deck
46
The Roman Coliseum is an elliptical amphitheater measuring approximately 188 meters long and 156 meters wide.Find an equation to model the coliseum that is of the form .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 556 flashcards in this deck.
Unlock Deck
k this deck
47
Select the graph of the polar equation using symmetry, zeros, maximum r-values, and any other additional points.
A)Symmetric with respect to , polar axis, poleCircle with radius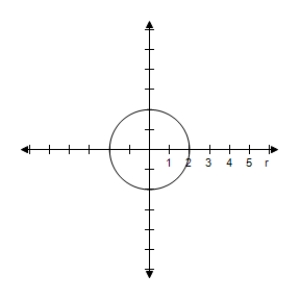
B)Symmetric with respect to , polar axis, poleCircle with radius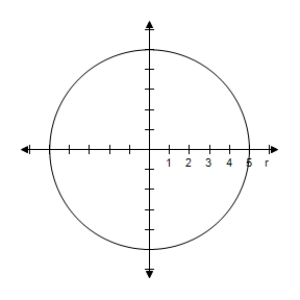
C)Symmetric with respect to , polar axis, poleCircle with radius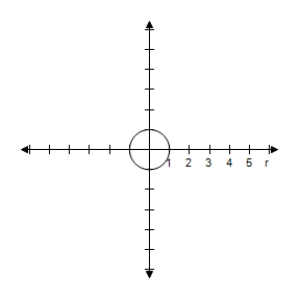
D)Symmetric with respect to , polar axis, poleCircle with radius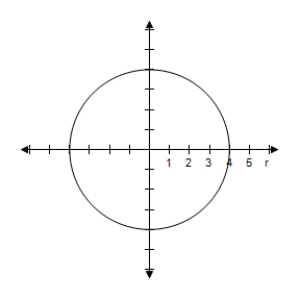
E)Symmetric with respect to , polar axis, poleCircle with radius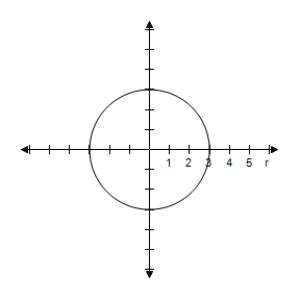
A)Symmetric with respect to , polar axis, poleCircle with radius

B)Symmetric with respect to , polar axis, poleCircle with radius

C)Symmetric with respect to , polar axis, poleCircle with radius

D)Symmetric with respect to , polar axis, poleCircle with radius

E)Symmetric with respect to , polar axis, poleCircle with radius


Unlock Deck
Unlock for access to all 556 flashcards in this deck.
Unlock Deck
k this deck
48
Select the graph of the polar equation using symmetry, zeros, maximum r-values, and any other additional points.
A)Symmetric with respect to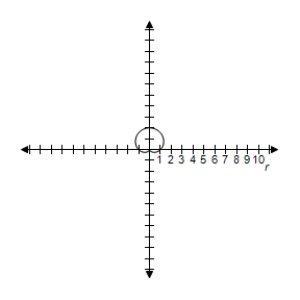
B)Symmetric with respect to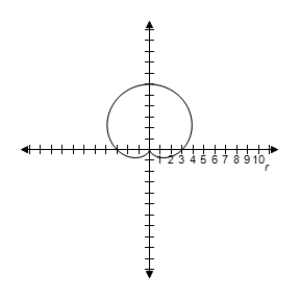
C)Symmetric with respect to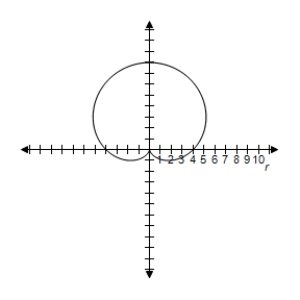
D)Symmetric with respect to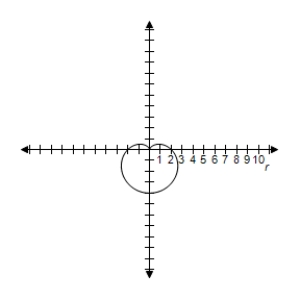
E)Symmetric with respect to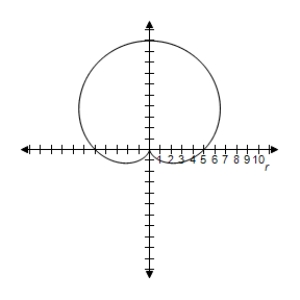
A)Symmetric with respect to

B)Symmetric with respect to

C)Symmetric with respect to

D)Symmetric with respect to

E)Symmetric with respect to


Unlock Deck
Unlock for access to all 556 flashcards in this deck.
Unlock Deck
k this deck
49
Select the graph of the polar equation using symmetry, zeros, maximum r-values, and any other additional points.
A)Symmetric with respect to , polar axis, poleCircle with radius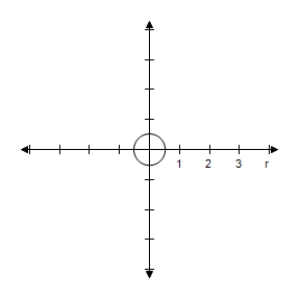
B)Symmetric with respect to , polar axis, poleCircle with radius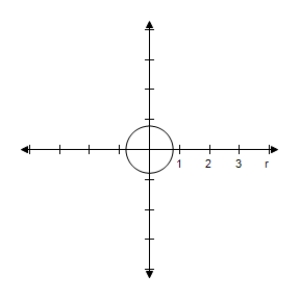
C)Symmetric with respect to , polar axis, poleCircle with radius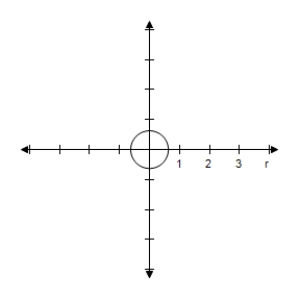
D)Symmetric with respect to , polar axis, poleCircle with radius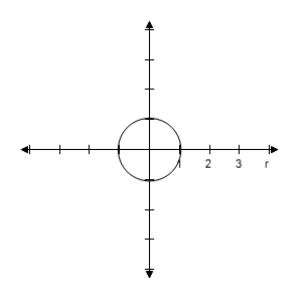
E)Symmetric with respect to , polar axis, poleCircle with radius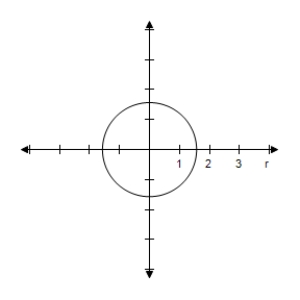
A)Symmetric with respect to , polar axis, poleCircle with radius

B)Symmetric with respect to , polar axis, poleCircle with radius

C)Symmetric with respect to , polar axis, poleCircle with radius

D)Symmetric with respect to , polar axis, poleCircle with radius

E)Symmetric with respect to , polar axis, poleCircle with radius


Unlock Deck
Unlock for access to all 556 flashcards in this deck.
Unlock Deck
k this deck
50
Select the graph of the polar equation using symmetry, zeros, maximum r-values, and any other additional points.
A)Symmetric with respect to when when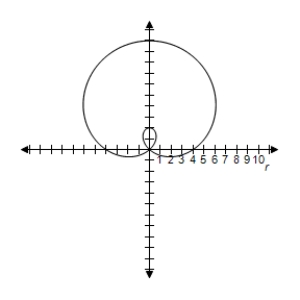
B)Symmetric with respect to when when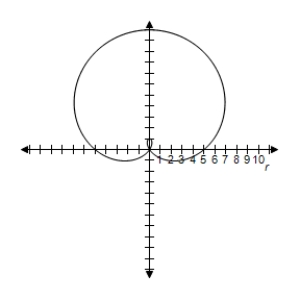
C)Symmetric with respect to when when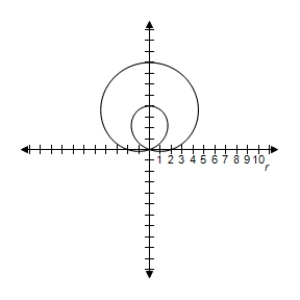
D)Symmetric with respect to when when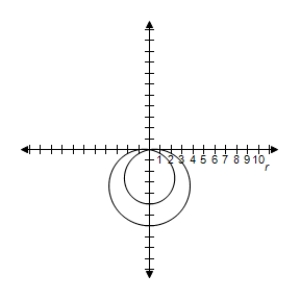
E)Symmetric with respect to when when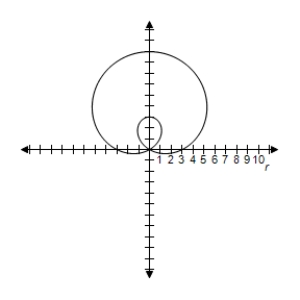
A)Symmetric with respect to when when

B)Symmetric with respect to when when

C)Symmetric with respect to when when

D)Symmetric with respect to when when

E)Symmetric with respect to when when


Unlock Deck
Unlock for access to all 556 flashcards in this deck.
Unlock Deck
k this deck
51
The Halley's Comet has an elliptical orbit with an eccentricity of .The length of the major axis of the orbit is approximately 35.1 astronomical units.Find a polar equation for the orbit.How close does the comet come to the sun
A) Closest point to the sun is 0.579 astronomical unit.
B) Closest point to the sun is 0.579 astronomical unit.
C) Closest point to the sun is 0.579 astronomical unit.
D) Closest point to the sun is 0.579 astronomical unit.
E) Closest point to the sun is 0.579 astronomical unit.
A) Closest point to the sun is 0.579 astronomical unit.
B) Closest point to the sun is 0.579 astronomical unit.
C) Closest point to the sun is 0.579 astronomical unit.
D) Closest point to the sun is 0.579 astronomical unit.
E) Closest point to the sun is 0.579 astronomical unit.

Unlock Deck
Unlock for access to all 556 flashcards in this deck.
Unlock Deck
k this deck
52
Use the following results the polar equation of the hyperbolla is to write the polar form of the equation of the conic .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 556 flashcards in this deck.
Unlock Deck
k this deck
53
Select the graph of the polar equation using symmetry, zeros, maximum r-values, and any other additional points.
A)Symmetric with respect to , polar axis, poleCircle with radius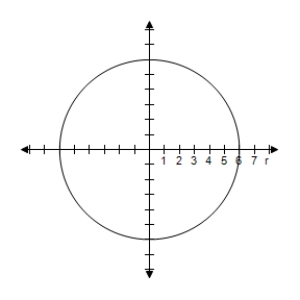
B)Symmetric with respect to , polar axis, poleCircle with radius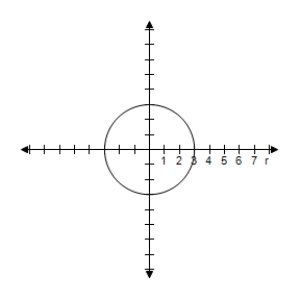
C)Symmetric with respect to , polar axis, poleCircle with radius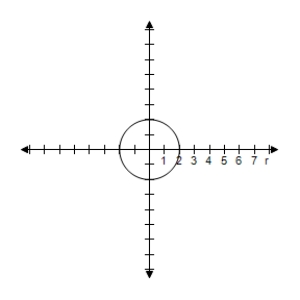
D)Symmetric with respect to , polar axis, poleCircle with radius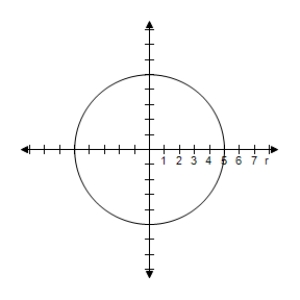
E)Symmetric with respect to , polar axis, poleCircle with radius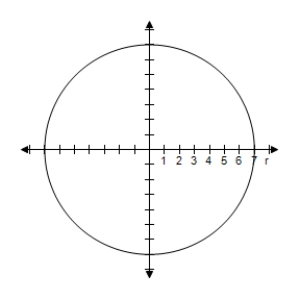
A)Symmetric with respect to , polar axis, poleCircle with radius

B)Symmetric with respect to , polar axis, poleCircle with radius

C)Symmetric with respect to , polar axis, poleCircle with radius

D)Symmetric with respect to , polar axis, poleCircle with radius

E)Symmetric with respect to , polar axis, poleCircle with radius


Unlock Deck
Unlock for access to all 556 flashcards in this deck.
Unlock Deck
k this deck
54
By using a graphing utility select the correct graph of the polar equation.Identify the graph.
A)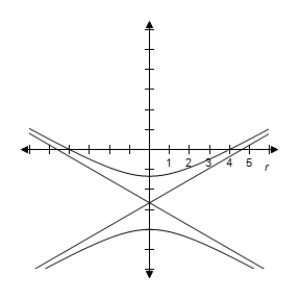 Hyperbola
Hyperbola
B)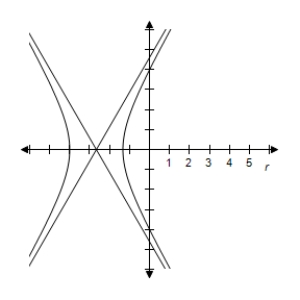 Hyperbola
Hyperbola
C)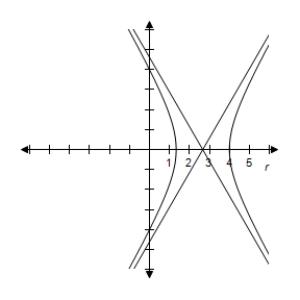 Hyperbola
Hyperbola
D)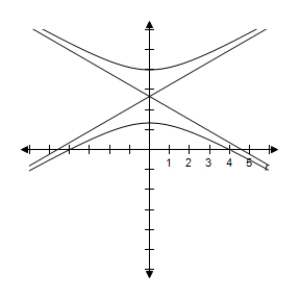 Hyperbola
Hyperbola
E)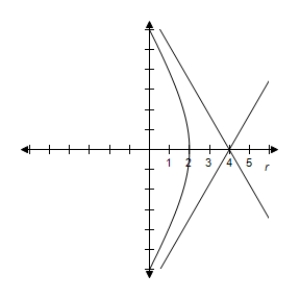 Hyperbola
Hyperbola
A)
 Hyperbola
HyperbolaB)
 Hyperbola
HyperbolaC)
 Hyperbola
HyperbolaD)
 Hyperbola
HyperbolaE)
 Hyperbola
Hyperbola
Unlock Deck
Unlock for access to all 556 flashcards in this deck.
Unlock Deck
k this deck
55
Select the graph of the polar equation using symmetry, zeros, maximum r-values, and any other additional points.
A)Symmetric with respect to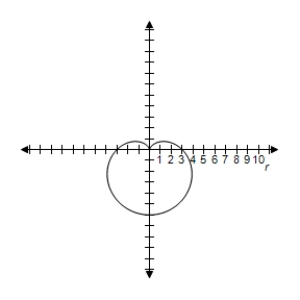
B)Symmetric with respect to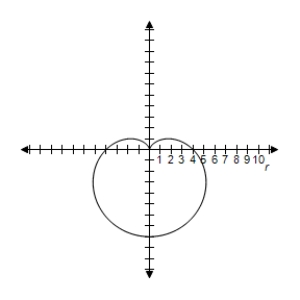
C)Symmetric with respect to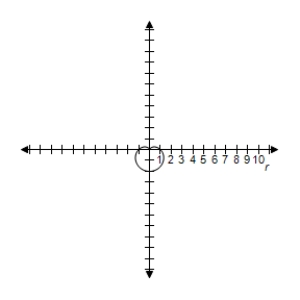
D)Symmetric with respect to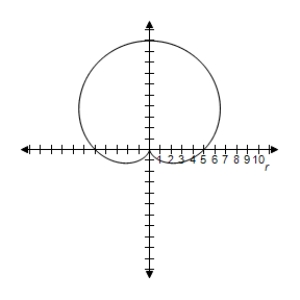
E)Symmetric with respect to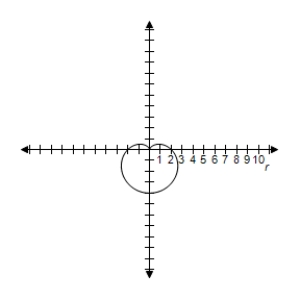
A)Symmetric with respect to

B)Symmetric with respect to

C)Symmetric with respect to

D)Symmetric with respect to

E)Symmetric with respect to


Unlock Deck
Unlock for access to all 556 flashcards in this deck.
Unlock Deck
k this deck
56
Select the graph of the polar equation using symmetry, zeros, maximum r-values, and any other additional points.
A)Symmetric with respect to polar axis
when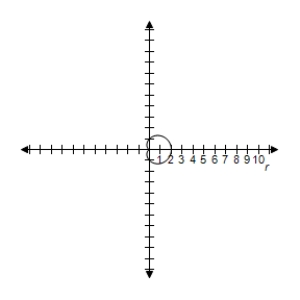
B)Symmetric with respect to polar axis
when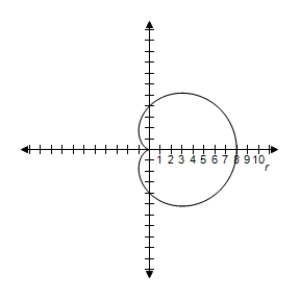
C)Symmetric with respect to polar axis
when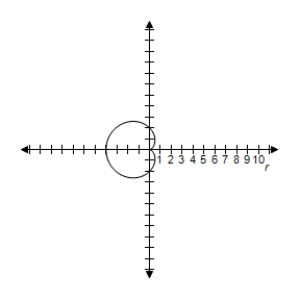
D)Symmetric with respect to polar axis
when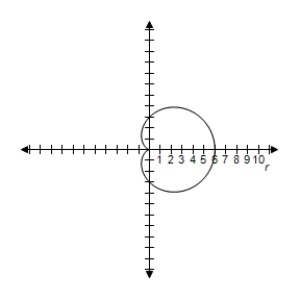
E)Symmetric with respect to polar axis
when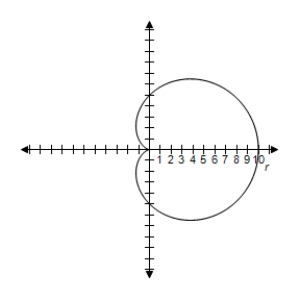
A)Symmetric with respect to polar axis
when

B)Symmetric with respect to polar axis
when

C)Symmetric with respect to polar axis
when

D)Symmetric with respect to polar axis
when

E)Symmetric with respect to polar axis
when


Unlock Deck
Unlock for access to all 556 flashcards in this deck.
Unlock Deck
k this deck
57
The Comet Borrelly has an elliptical orbit with an eccentricity of .The length of the major axis of the orbit is approximately 5.83 astronomical units.Find a polar equation for the orbit.How close does the comet come to the sun
A) Closest point to the sun is 1.096 astronomical unit.
B) Closest point to the sun is 1.096 astronomical unit.
C) Closest point to the sun is 1.096 astronomical unit.
D) Closest point to the sun is 1.096 astronomical unit.
E) Closest point to the sun is 1.096 astronomical unit.
A) Closest point to the sun is 1.096 astronomical unit.
B) Closest point to the sun is 1.096 astronomical unit.
C) Closest point to the sun is 1.096 astronomical unit.
D) Closest point to the sun is 1.096 astronomical unit.
E) Closest point to the sun is 1.096 astronomical unit.

Unlock Deck
Unlock for access to all 556 flashcards in this deck.
Unlock Deck
k this deck
58
Select the graph of the polar equation using symmetry, zeros, maximum r-values, and any other additional points.
A)Symmetric with respect to polar axisCircle with radius 2.5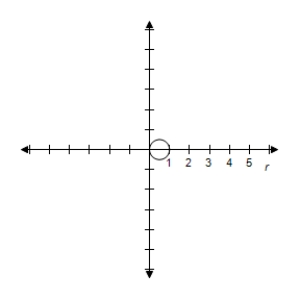
B)Symmetric with respect to polar axisCircle with radius 2.5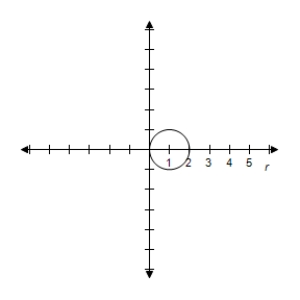
C)Symmetric with respect to polar axisCircle with radius 2.5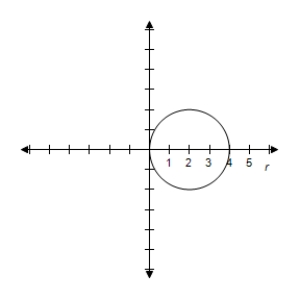
D)Symmetric with respect to polar axisCircle with radius 2.5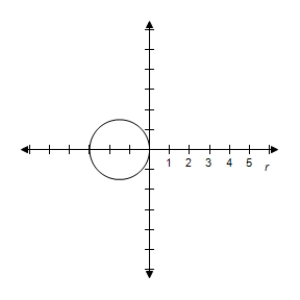
E)Symmetric with respect to polar axisCircle with radius 2.5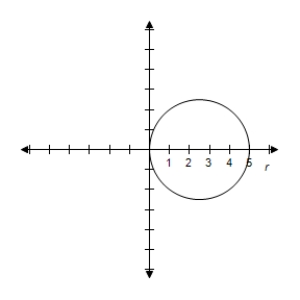
A)Symmetric with respect to polar axisCircle with radius 2.5

B)Symmetric with respect to polar axisCircle with radius 2.5

C)Symmetric with respect to polar axisCircle with radius 2.5

D)Symmetric with respect to polar axisCircle with radius 2.5

E)Symmetric with respect to polar axisCircle with radius 2.5


Unlock Deck
Unlock for access to all 556 flashcards in this deck.
Unlock Deck
k this deck
59
Use the following results the polar equation of the ellipse is to write the polar form of the equation of the conic .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 556 flashcards in this deck.
Unlock Deck
k this deck
60
Consider the polar equation:
Identify the conic without graphing the equation.
A) , the conic is a parabola.
B) , the conic is a circle.
C) , the conic is a hyperbola.
D) , the conic is an ellipse.
E)None of the above
Identify the conic without graphing the equation.
A) , the conic is a parabola.
B) , the conic is a circle.
C) , the conic is a hyperbola.
D) , the conic is an ellipse.
E)None of the above

Unlock Deck
Unlock for access to all 556 flashcards in this deck.
Unlock Deck
k this deck
61
Select the graph of over the interval.Describe the part of the graph obtained in this case.
A)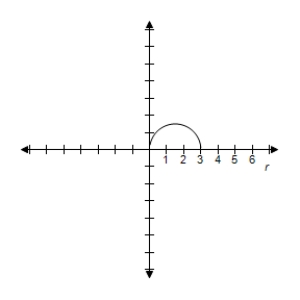 Upper half of circle
Upper half of circle
B)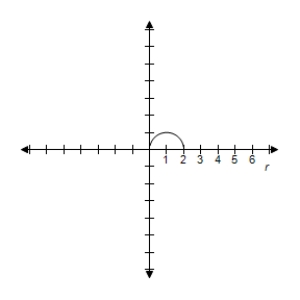 Upper half of circle
Upper half of circle
C)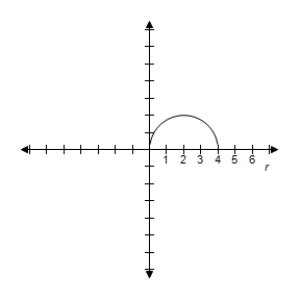 Upper half of circle
Upper half of circle
D)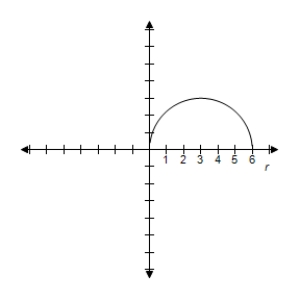 Upper half of circle
Upper half of circle
E)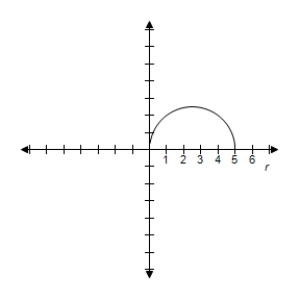 Upper half of circle
Upper half of circle
A)
 Upper half of circle
Upper half of circleB)
 Upper half of circle
Upper half of circleC)
 Upper half of circle
Upper half of circleD)
 Upper half of circle
Upper half of circleE)
 Upper half of circle
Upper half of circle
Unlock Deck
Unlock for access to all 556 flashcards in this deck.
Unlock Deck
k this deck
62
Select the graph of the polar equation using symmetry, zeros, maximum r-values, and any other additional points.
A)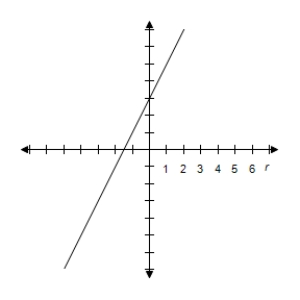
B)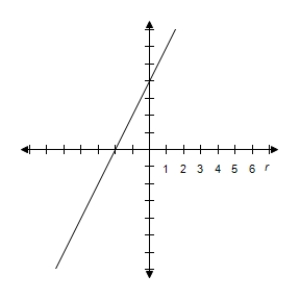
C)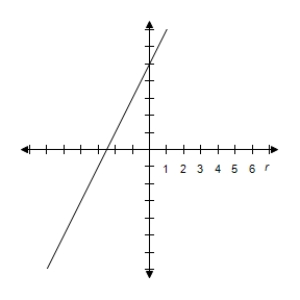
D)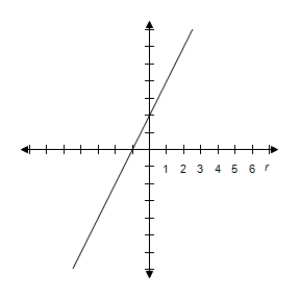
E)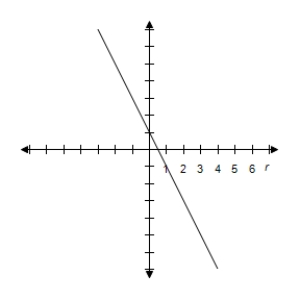
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 556 flashcards in this deck.
Unlock Deck
k this deck
63
Select the graph of the polar equation using symmetry, zeros, maximum r-values, and any other additional points.
A)Symmetric with respect to the polar axis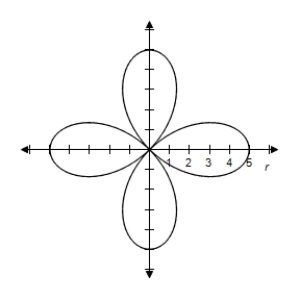
B)Symmetric with respect to the polar axis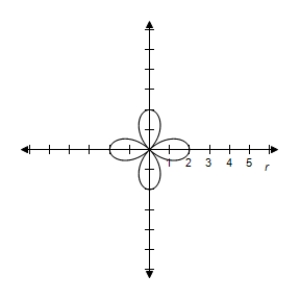
C)Symmetric with respect to the polar axis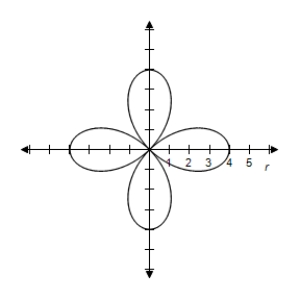
D)Symmetric with respect to the polar axis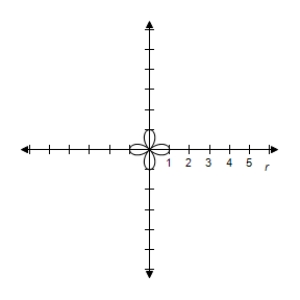
E)Symmetric with respect to the polar axis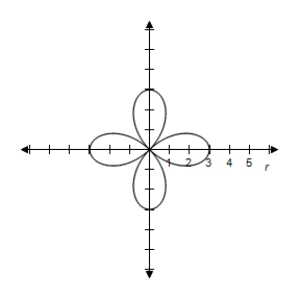
A)Symmetric with respect to the polar axis

B)Symmetric with respect to the polar axis

C)Symmetric with respect to the polar axis

D)Symmetric with respect to the polar axis

E)Symmetric with respect to the polar axis


Unlock Deck
Unlock for access to all 556 flashcards in this deck.
Unlock Deck
k this deck
64
Select the graph of the equation.
A)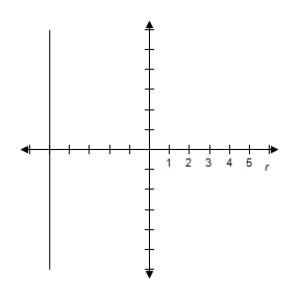
B)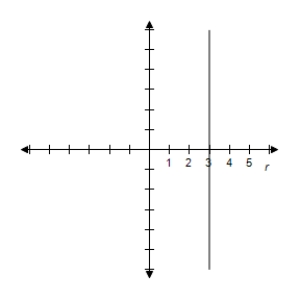
C)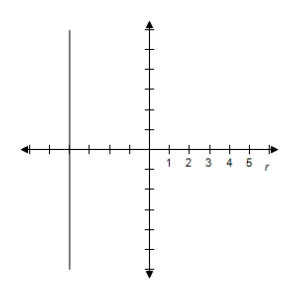
D)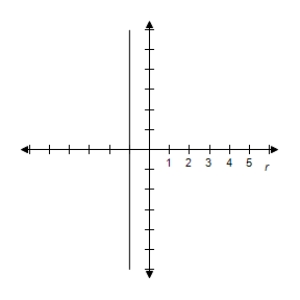
E)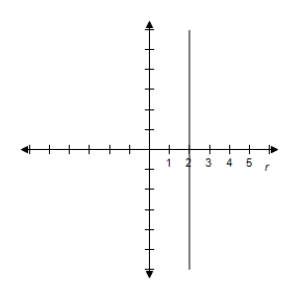
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 556 flashcards in this deck.
Unlock Deck
k this deck
65
Select the graph of over the interval.Describe the part of the graph obtained in this case.
A)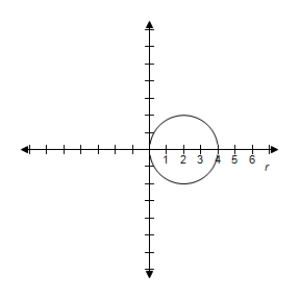 Entire circle
Entire circle
B)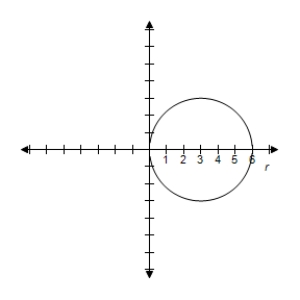 Entire circle
Entire circle
C)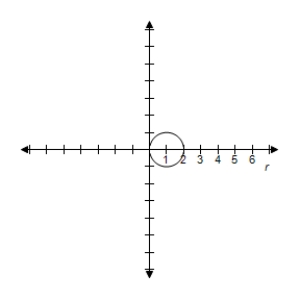 Entire circle
Entire circle
D)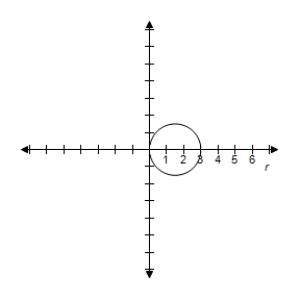 Entire circle
Entire circle
E)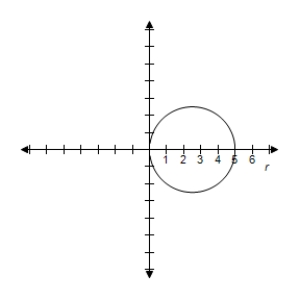 Entire circle
Entire circle
A)
 Entire circle
Entire circleB)
 Entire circle
Entire circleC)
 Entire circle
Entire circleD)
 Entire circle
Entire circleE)
 Entire circle
Entire circle
Unlock Deck
Unlock for access to all 556 flashcards in this deck.
Unlock Deck
k this deck
66
Select the graph of the polar equation using symmetry, zeros, maximum r-values, and any other additional points.
A)Symmetric with respect to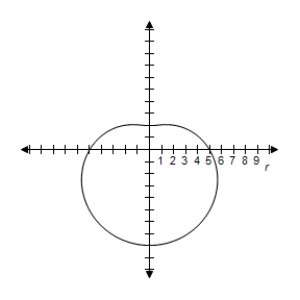
B)Symmetric with respect to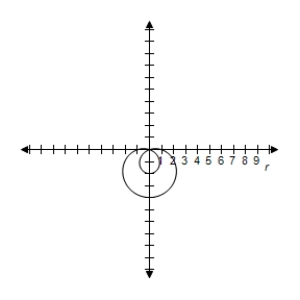
C)Symmetric with respect to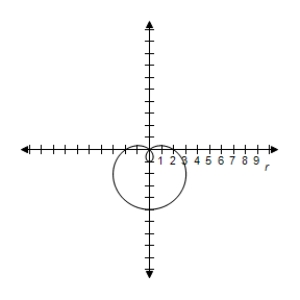
D)Symmetric with respect to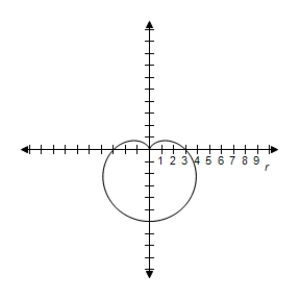
E)Symmetric with respect to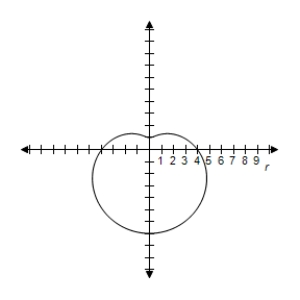
A)Symmetric with respect to

B)Symmetric with respect to

C)Symmetric with respect to

D)Symmetric with respect to

E)Symmetric with respect to


Unlock Deck
Unlock for access to all 556 flashcards in this deck.
Unlock Deck
k this deck
67
Select the graph of the polar equation using symmetry, zeros, maximum r-values, and any other additional points.
A)Symmetric with respect to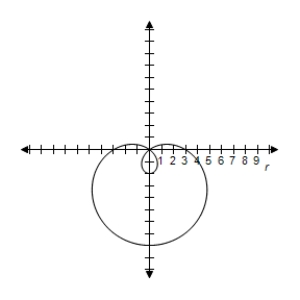
B)Symmetric with respect to
C)Symmetric with respect to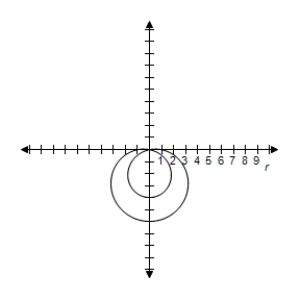
D)Symmetric with respect to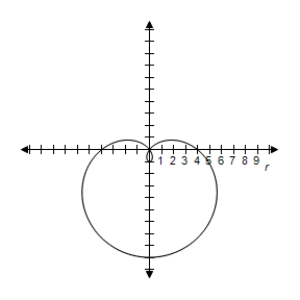
E)Symmetric with respect to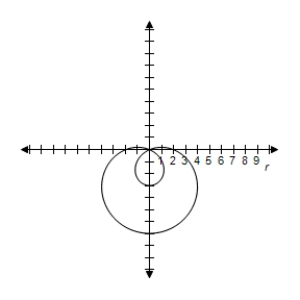
A)Symmetric with respect to

B)Symmetric with respect to

C)Symmetric with respect to

D)Symmetric with respect to

E)Symmetric with respect to


Unlock Deck
Unlock for access to all 556 flashcards in this deck.
Unlock Deck
k this deck
68
Select the graph of the polar equation using symmetry, zeros, maximum r-values, and any other additional points.
A)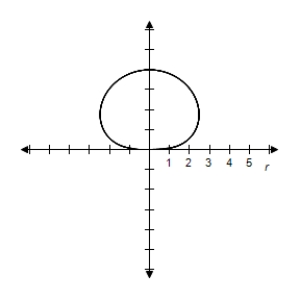
B)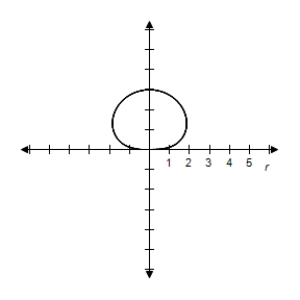
C)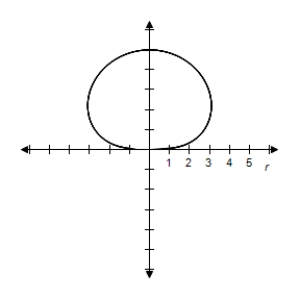
D)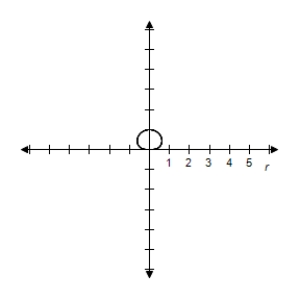
E)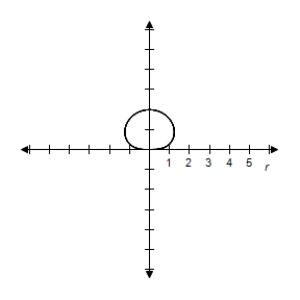
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 556 flashcards in this deck.
Unlock Deck
k this deck
69
Select the graph of the polar equation using symmetry, zeros, maximum r-values, and any other additional points.
A)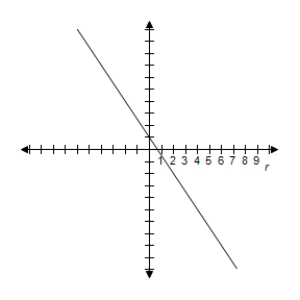
B)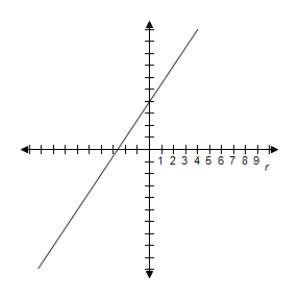
C)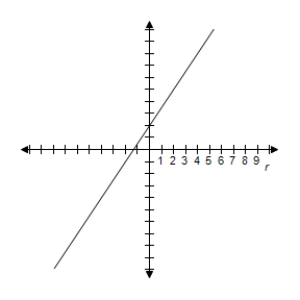
D)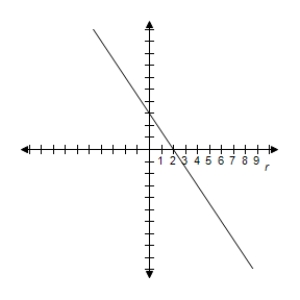
E)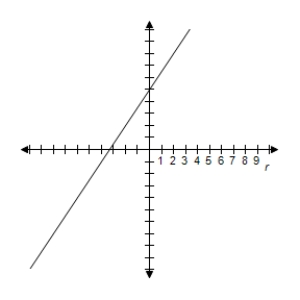
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 556 flashcards in this deck.
Unlock Deck
k this deck
70
Select the graph of the polar equation using symmetry, zeros, maximum r-values, and any other additional points.
A)Symmetric with respect to polar axis when
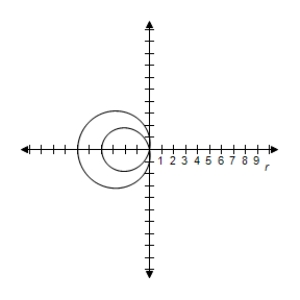
B)Symmetric with respect to polar axis when
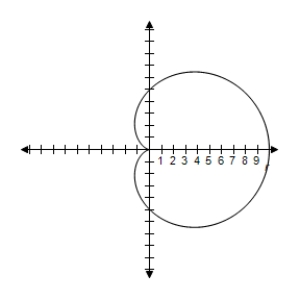
C)Symmetric with respect to polar axis when
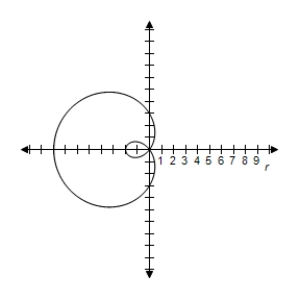
D)Symmetric with respect to polar axis when
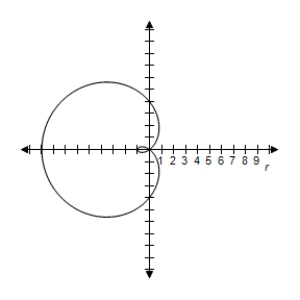
E)Symmetric with respect to polar axis when
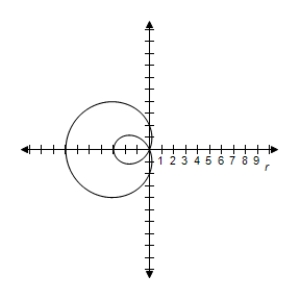
A)Symmetric with respect to polar axis when

B)Symmetric with respect to polar axis when

C)Symmetric with respect to polar axis when

D)Symmetric with respect to polar axis when

E)Symmetric with respect to polar axis when


Unlock Deck
Unlock for access to all 556 flashcards in this deck.
Unlock Deck
k this deck
71
Select the graph of over the interval.Describe the part of the graph obtained in this case.
A)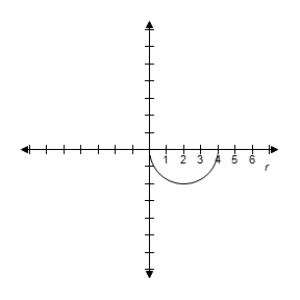 Lower half of circle
Lower half of circle
B)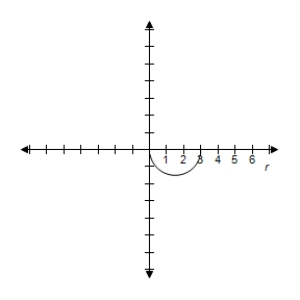 Lower half of circle
Lower half of circle
C)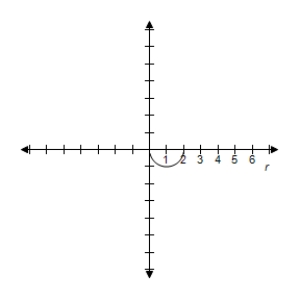 Lower half of circle
Lower half of circle
D)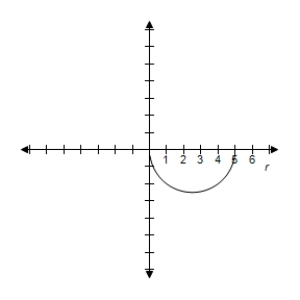 Lower half of circle
Lower half of circle
E)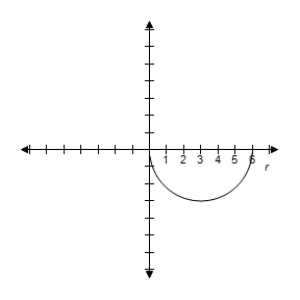 Lower half of circle
Lower half of circle
A)
 Lower half of circle
Lower half of circleB)
 Lower half of circle
Lower half of circleC)
 Lower half of circle
Lower half of circleD)
 Lower half of circle
Lower half of circleE)
 Lower half of circle
Lower half of circle
Unlock Deck
Unlock for access to all 556 flashcards in this deck.
Unlock Deck
k this deck
72
Select the graph of the polar equation using symmetry, zeros, maximum r-values, and any other additional points.
A)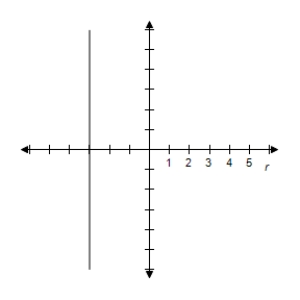
B)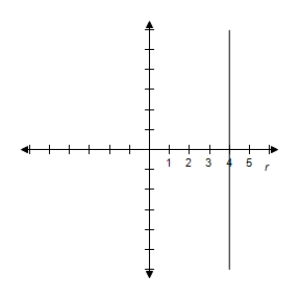
C)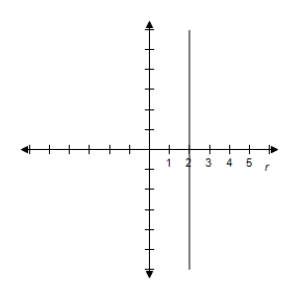
D)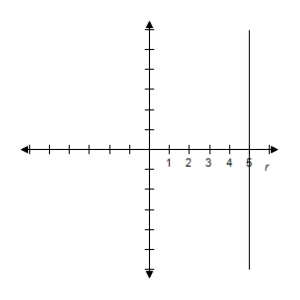
E)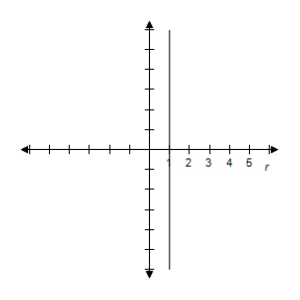
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 556 flashcards in this deck.
Unlock Deck
k this deck
73
Select the graph of the polar equation using symmetry, zeros, maximum r-values, and any other additional points.
A)Symmetric with respect to the polar axis when
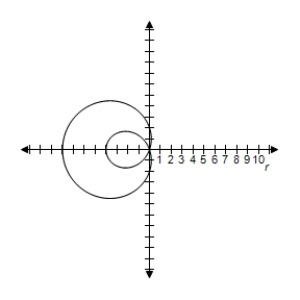
B)Symmetric with respect to the polar axis when
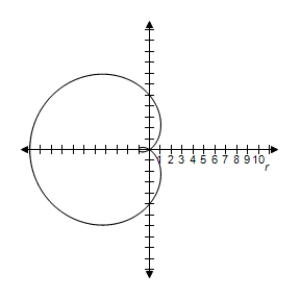
C)Symmetric with respect to the polar axis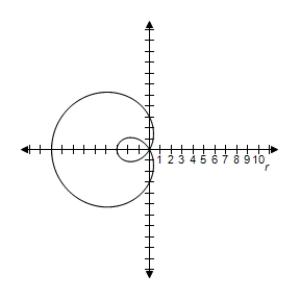
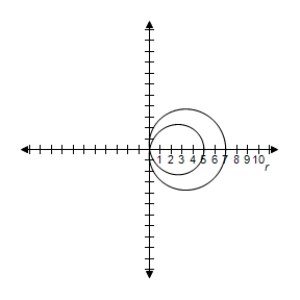
D)Symmetric with respect to the polar axis when
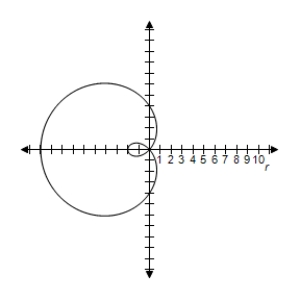
E)Symmetric with respect to the polar axis when
A)Symmetric with respect to the polar axis when

B)Symmetric with respect to the polar axis when

C)Symmetric with respect to the polar axis

D)Symmetric with respect to the polar axis when

E)Symmetric with respect to the polar axis when


Unlock Deck
Unlock for access to all 556 flashcards in this deck.
Unlock Deck
k this deck
74
Select the graph of the polar equation using symmetry, zeros, maximum r-values, and any other additional points.
A)Symmetric with respect to the polar axis when when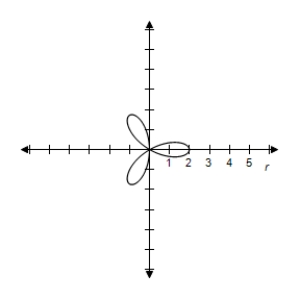
B)Symmetric with respect to the polar axis when when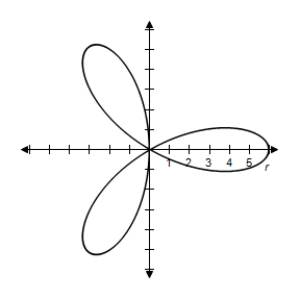
C)Symmetric with respect to the polar axis when
when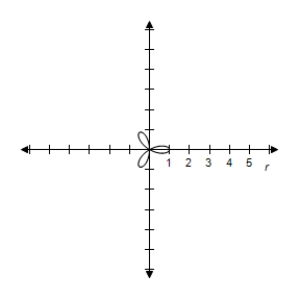
D)Symmetric with respect to the polar axis when
when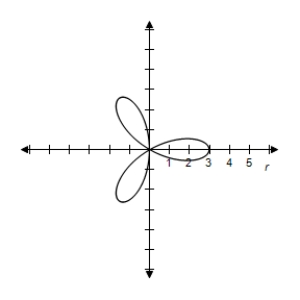
E)Symmetric with respect to the polar axis when
when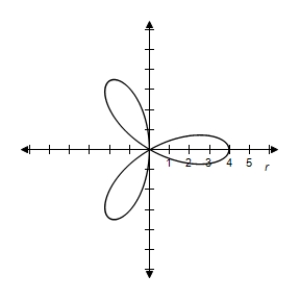
A)Symmetric with respect to the polar axis when when

B)Symmetric with respect to the polar axis when when

C)Symmetric with respect to the polar axis when
when

D)Symmetric with respect to the polar axis when
when

E)Symmetric with respect to the polar axis when
when


Unlock Deck
Unlock for access to all 556 flashcards in this deck.
Unlock Deck
k this deck
75
Select the graph of the polar equation using symmetry, zeros, maximum r-values, and any other additional points.
A)Symmetric with respectto the polar axis, , and the poleLemniscate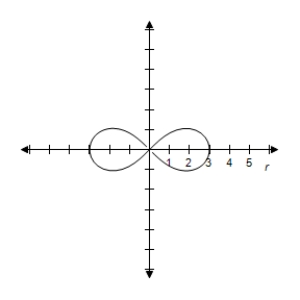
B)Symmetric with respectto the polar axis, , and the poleLemniscate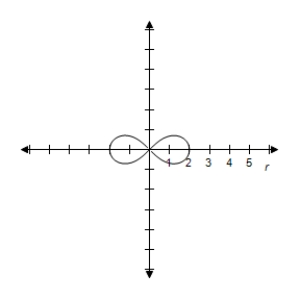
C)Symmetric with respectto the polar axis, , and the poleLemniscate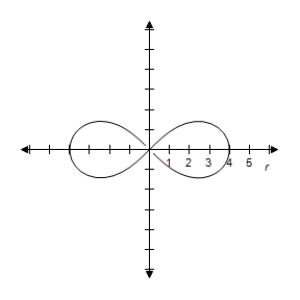
D)Symmetric with respectto the polar axis, , and the poleLemniscate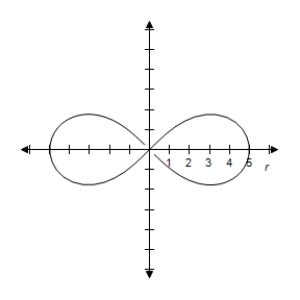
E)Symmetric with respectto the polar axis, , and the poleLemniscate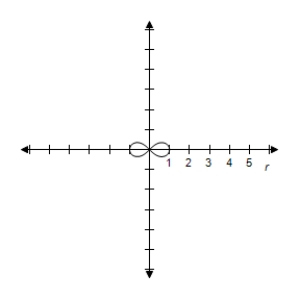
A)Symmetric with respectto the polar axis, , and the poleLemniscate

B)Symmetric with respectto the polar axis, , and the poleLemniscate

C)Symmetric with respectto the polar axis, , and the poleLemniscate

D)Symmetric with respectto the polar axis, , and the poleLemniscate

E)Symmetric with respectto the polar axis, , and the poleLemniscate


Unlock Deck
Unlock for access to all 556 flashcards in this deck.
Unlock Deck
k this deck
76
Select the graph of the polar equation using symmetry, zeros, maximum r-values, and any other additional points.
A)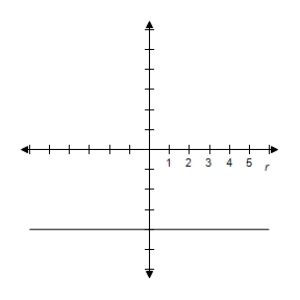
B)
C)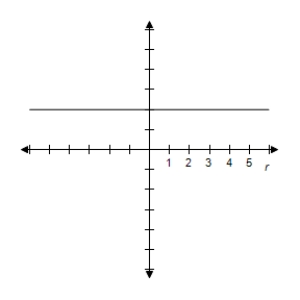
D)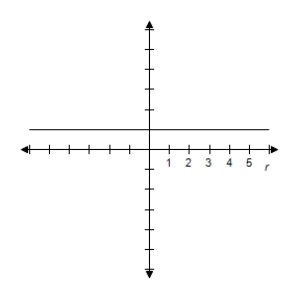
E)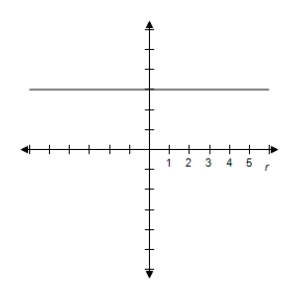
A)

B)

C)

D)

E)


Unlock Deck
Unlock for access to all 556 flashcards in this deck.
Unlock Deck
k this deck
77
Select the graph of over the interval.Describe the part of the graph obtained in this case.
A)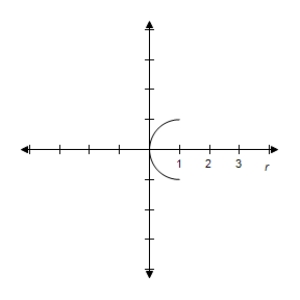 Left half of circle
Left half of circle
B)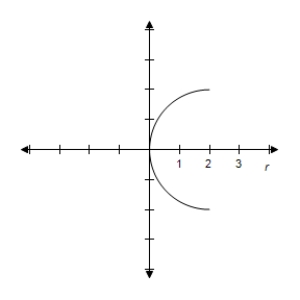 Left half of circle
Left half of circle
C)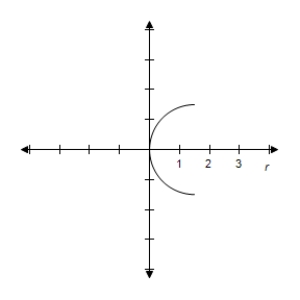 Left half of circle
Left half of circle
D)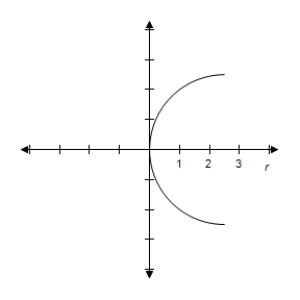 Left half of circle
Left half of circle
E)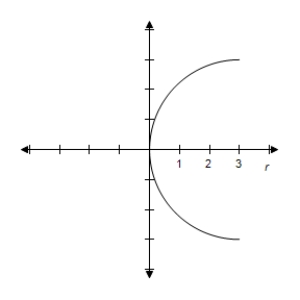 Left half of circle
Left half of circle
A)
 Left half of circle
Left half of circleB)
 Left half of circle
Left half of circleC)
 Left half of circle
Left half of circleD)
 Left half of circle
Left half of circleE)
 Left half of circle
Left half of circle
Unlock Deck
Unlock for access to all 556 flashcards in this deck.
Unlock Deck
k this deck
78
Select the graph of the polar equation using symmetry, zeros, maximum r-values, and any other additional points.
A)Symmetric with respect to , the polar axis, and the pole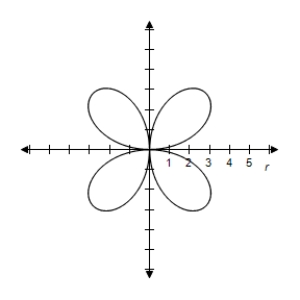
B)Symmetric with respect to , the polar axis, and the pole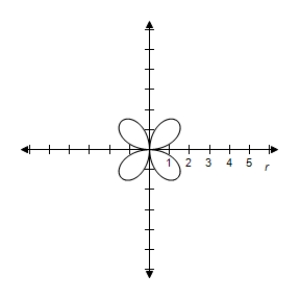
C)Symmetric with respect to , the polar axis, and the pole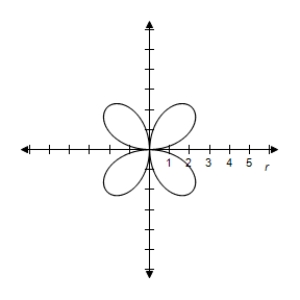
D)Symmetric with respect to , the polar axis, and the pole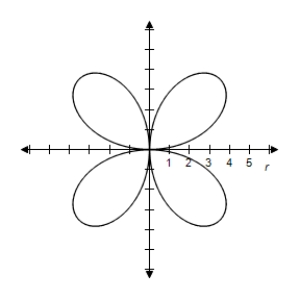
E)Symmetric with respect to , the polar axis, and the pole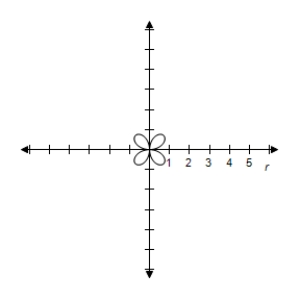
A)Symmetric with respect to , the polar axis, and the pole

B)Symmetric with respect to , the polar axis, and the pole

C)Symmetric with respect to , the polar axis, and the pole

D)Symmetric with respect to , the polar axis, and the pole

E)Symmetric with respect to , the polar axis, and the pole


Unlock Deck
Unlock for access to all 556 flashcards in this deck.
Unlock Deck
k this deck
79
Select the graph of the polar equation using symmetry, zeros, maximum r-values, and any other additional points.
A)Symmetric with respect to the polar axis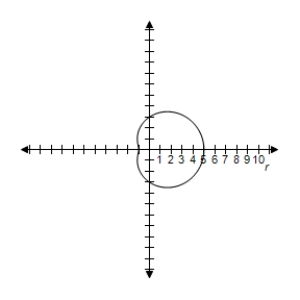
B)Symmetric with respect to the polar axis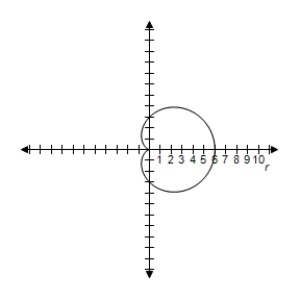
C)Symmetric with respect to the polar axis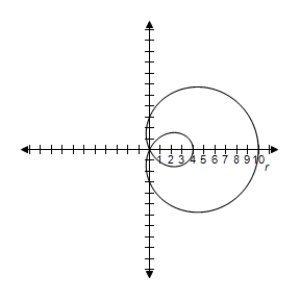
D)Symmetric with respect to the polar axis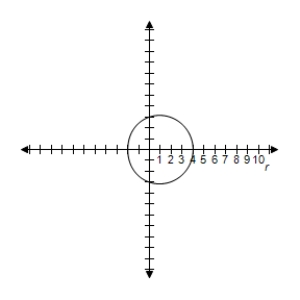
E)Symmetric with respect to the polar axis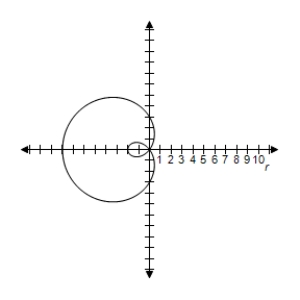
A)Symmetric with respect to the polar axis

B)Symmetric with respect to the polar axis

C)Symmetric with respect to the polar axis

D)Symmetric with respect to the polar axis

E)Symmetric with respect to the polar axis


Unlock Deck
Unlock for access to all 556 flashcards in this deck.
Unlock Deck
k this deck
80
Select the graph of the polar equation using symmetry, zeros, maximum r-values, and any other additional points.
A)Symmetric with respect to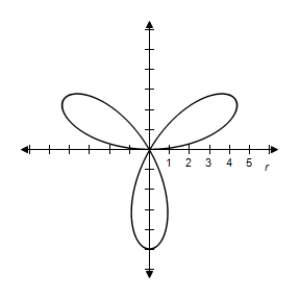
B)Symmetric with respect to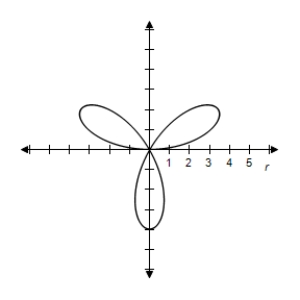
C)Symmetric with respect to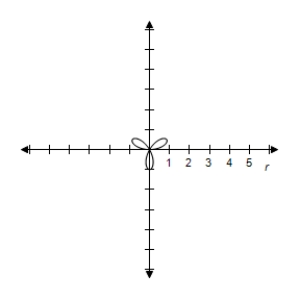
D)Symmetric with respect to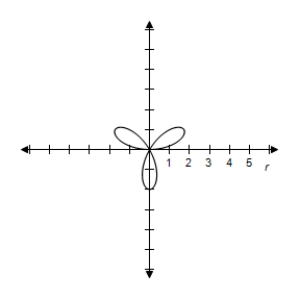
E)Symmetric with respect to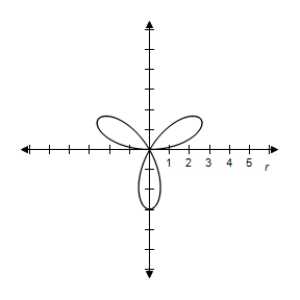
A)Symmetric with respect to

B)Symmetric with respect to

C)Symmetric with respect to

D)Symmetric with respect to

E)Symmetric with respect to


Unlock Deck
Unlock for access to all 556 flashcards in this deck.
Unlock Deck
k this deck



