Deck 8: Matrices and Determinants
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/283
Play
Full screen (f)
Deck 8: Matrices and Determinants
1
Use a graphing utility and Cramer's Rule to solve (if possible) the system of equations.
A)(-1, -1, 2)
B)(-1, -1, -1)
C)(-1, 1, -2)
D)(-1, -1, -2)
E)(-1, 1, 2)
A)(-1, -1, 2)
B)(-1, -1, -1)
C)(-1, 1, -2)
D)(-1, -1, -2)
E)(-1, 1, 2)
(-1, -1, 2)
2
Use a determinant and the given vertices of a triangle to find the area of the triangle.
(-5, 5), (3, 4), (4, -6)
A)
B)-
C)
D)79
E)
(-5, 5), (3, 4), (4, -6)
A)
B)-
C)
D)79
E)
3
Use Cramer's Rule to solve (if possible) the system of equations.
A)(-2, 3, -3)
B)(7, -3, 2)
C)(2, 3, -3)
D)(2, -3, -3)
E)(-2, -3, -3)
A)(-2, 3, -3)
B)(7, -3, 2)
C)(2, 3, -3)
D)(2, -3, -3)
E)(-2, -3, -3)
(-2, 3, -3)
4
Find a value of y such that the triangle with the given vertices has an area of 4 square units.
(-1, 8), (0, 4), (-1, y)
A)y = 16 or y = -8
B)y = 16 or y = 0
C)y = -8 or y = 0
D)y = -16 or y = 0
E)y = 8 or y = 0
(-1, 8), (0, 4), (-1, y)
A)y = 16 or y = -8
B)y = 16 or y = 0
C)y = -8 or y = 0
D)y = -16 or y = 0
E)y = 8 or y = 0

Unlock Deck
Unlock for access to all 283 flashcards in this deck.
Unlock Deck
k this deck
5
Use a determinant to find an equation of the line passing through the points.
(0, 0), (3, 5)
A)3x - 5y = 0
B)5x - 3y = 5
C)5x - 3y = 0
D)5x + 3y = 3
E)5x + 3y = 0
(0, 0), (3, 5)
A)3x - 5y = 0
B)5x - 3y = 5
C)5x - 3y = 0
D)5x + 3y = 3
E)5x + 3y = 0

Unlock Deck
Unlock for access to all 283 flashcards in this deck.
Unlock Deck
k this deck
6
Find y such that the points are collinear.
(-3, 3), (-4, y), (-2, 4)
A)y = 3
B)y = 4
C)y = -2
D)y = 2
E)y = 5
(-3, 3), (-4, y), (-2, 4)
A)y = 3
B)y = 4
C)y = -2
D)y = 2
E)y = 5

Unlock Deck
Unlock for access to all 283 flashcards in this deck.
Unlock Deck
k this deck
7
Use Cramer's Rule to solve (if possible) the system of equations.
A)(0, 2, -4)
B)(0, -2, 4)
C)(0, -2, -4)
D)(0, 2, 0)
E)(0, 2, 4)
A)(0, 2, -4)
B)(0, -2, 4)
C)(0, -2, -4)
D)(0, 2, 0)
E)(0, 2, 4)

Unlock Deck
Unlock for access to all 283 flashcards in this deck.
Unlock Deck
k this deck
8
Find y such that the points are collinear.
(5, -4), (3, y), (4, -3)
A)y = -2
B)y = 1
C)y = 0
D)y = -1
E)y = 2
(5, -4), (3, y), (4, -3)
A)y = -2
B)y = 1
C)y = 0
D)y = -1
E)y = 2

Unlock Deck
Unlock for access to all 283 flashcards in this deck.
Unlock Deck
k this deck
9
Use a determinant and the given vertices of a triangle to find the area of the triangle.
(-2, 4), (2, 5), (6, -4)
A)
B)20
C)
D)-20
E)40
(-2, 4), (2, 5), (6, -4)
A)
B)20
C)
D)-20
E)40

Unlock Deck
Unlock for access to all 283 flashcards in this deck.
Unlock Deck
k this deck
10
Use Cramer's Rule to solve (if possible) the system of equations.
A)(-6, -2)
B)(6, -2)
C)(-6, 2)
D)(6, 2)
E)(-2, -6)
A)(-6, -2)
B)(6, -2)
C)(-6, 2)
D)(6, 2)
E)(-2, -6)

Unlock Deck
Unlock for access to all 283 flashcards in this deck.
Unlock Deck
k this deck
11
Use Cramer's Rule to solve (if possible) the system of equations.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 283 flashcards in this deck.
Unlock Deck
k this deck
12
Use Cramer's Rule to solve (if possible) the system of equations.
A)(3, -6, -3)
B)(3, 6, -3)
C)(-3, 6, -3)
D)(-3, -6, -3)
E)(7, -6, -3)
A)(3, -6, -3)
B)(3, 6, -3)
C)(-3, 6, -3)
D)(-3, -6, -3)
E)(7, -6, -3)

Unlock Deck
Unlock for access to all 283 flashcards in this deck.
Unlock Deck
k this deck
13
Use Cramer's Rule to solve (if possible) the system of equations.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 283 flashcards in this deck.
Unlock Deck
k this deck
14
Use a determinant and the given vertices of a triangle to find the area of the triangle.
(0, -3), (-3, 3), (4, 4)
A)
B)
C)
D)
E)45
(0, -3), (-3, 3), (4, 4)
A)
B)
C)
D)
E)45

Unlock Deck
Unlock for access to all 283 flashcards in this deck.
Unlock Deck
k this deck
15
Use Cramer's Rule to solve (if possible) the system of equations.
A)(-8, -5)
B)(8, -5)
C)(-8, 5)
D)(8, 5)
E)(5, 8)
A)(-8, -5)
B)(8, -5)
C)(-8, 5)
D)(8, 5)
E)(5, 8)

Unlock Deck
Unlock for access to all 283 flashcards in this deck.
Unlock Deck
k this deck
16
Use a determinant and the given vertices of a triangle to find the area of the triangle.
(-2, 5), (3, 3), (-1, 6)
A)
B)
C)
D)
E)
(-2, 5), (3, 3), (-1, 6)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 283 flashcards in this deck.
Unlock Deck
k this deck
17
Use a determinant to find an equation of the line passing through the points.
(-5, 3), (1, 1)
A)2x -6y + 8 = 0
B)2x + 6y + 8 = 0
C)2x -6y - 8 = 0
D)2x + 6y - 8 = 0
E)2x + 6y - 8 = 5
(-5, 3), (1, 1)
A)2x -6y + 8 = 0
B)2x + 6y + 8 = 0
C)2x -6y - 8 = 0
D)2x + 6y - 8 = 0
E)2x + 6y - 8 = 5

Unlock Deck
Unlock for access to all 283 flashcards in this deck.
Unlock Deck
k this deck
18
Use a determinant to find an equation of the line passing through the points.
(8, 8), (-4, -8)
A)4x + 3y + 8 = 0
B)4x + 3y - 8 = 0
C)4x - 3y + 8 = 0
D)4x - 3y - 8 = 0
E)4x - 3y = 0
(8, 8), (-4, -8)
A)4x + 3y + 8 = 0
B)4x + 3y - 8 = 0
C)4x - 3y + 8 = 0
D)4x - 3y - 8 = 0
E)4x - 3y = 0

Unlock Deck
Unlock for access to all 283 flashcards in this deck.
Unlock Deck
k this deck
19
Use Cramer's Rule to solve (if possible) the system of equations.
A)(-6, 2)
B)(6, -2)
C)(6, 2)
D)(2, -6)
E)(-6, -2)
A)(-6, 2)
B)(6, -2)
C)(6, 2)
D)(2, -6)
E)(-6, -2)

Unlock Deck
Unlock for access to all 283 flashcards in this deck.
Unlock Deck
k this deck
20
Use Cramer's Rule to solve (if possible) the system of equations.
A)(6, 2, 6)
B)(6, -2, 6)
C)(-6, 2, 6)
D)(7, -2, 6)
E)(-6, -2, 6)
A)(6, 2, 6)
B)(6, -2, 6)
C)(-6, 2, 6)
D)(7, -2, 6)
E)(-6, -2, 6)

Unlock Deck
Unlock for access to all 283 flashcards in this deck.
Unlock Deck
k this deck
21
Find a value of y such that the triangle with the given vertices has an area of 26 square units.
(-6, -6), (2, -2), (-9, y)
A)y = -1 or y = -14
B)y = -1 or y = 14
C)y = -1 or y = 0
D)y = 1 or y = 14
E)y = 1 or y = -14
(-6, -6), (2, -2), (-9, y)
A)y = -1 or y = -14
B)y = -1 or y = 14
C)y = -1 or y = 0
D)y = 1 or y = 14
E)y = 1 or y = -14

Unlock Deck
Unlock for access to all 283 flashcards in this deck.
Unlock Deck
k this deck
22
A triangular region of farmland has become overrun with deer and will be open to the public for hunting to reduce the population.In order to know how many hunters to allow on the land at one time, you need to know the area of the region in square miles.In order to estimate the area of the region, you travel from the southernmost vertex C north 20 miles then west 24 miles (for vertex B), and from the southernmost vertex C you travel 54 miles north then 7 miles east (for vertex A).Use a graphing utility to approximate the number of square miles of land. 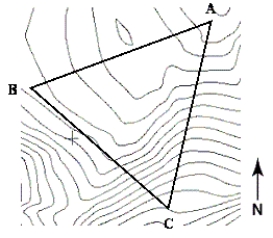
A)578 square miles
B)51 square miles
C)119 square miles
D)429 square miles
E)718 square miles

A)578 square miles
B)51 square miles
C)119 square miles
D)429 square miles
E)718 square miles

Unlock Deck
Unlock for access to all 283 flashcards in this deck.
Unlock Deck
k this deck
23
Use Cramer's Rule to solve the following system of linear equations:
A)
B)
C)
D)
E) .
A)
B)
C)
D)
E) .

Unlock Deck
Unlock for access to all 283 flashcards in this deck.
Unlock Deck
k this deck
24
Use a determinant to find y such that (8, -20), (16, y), and (20, -8) are collinear.
A)y = -12
B)y = 20
C)y = -44
D)y = 4
E)y = -8
A)y = -12
B)y = 20
C)y = -44
D)y = 4
E)y = -8

Unlock Deck
Unlock for access to all 283 flashcards in this deck.
Unlock Deck
k this deck
25
Find a value of y such that the triangle with the given vertices has an area of 8 square units.
(5, 6), (5, 8), (-3, y)
A)y = 10 or y = -9
B)y = -10 or y = -9
C)y = 10 or y = 9
D)y = 10 or y = 0
E)y = -10 or y = 9
(5, 6), (5, 8), (-3, y)
A)y = 10 or y = -9
B)y = -10 or y = -9
C)y = 10 or y = 9
D)y = 10 or y = 0
E)y = -10 or y = 9

Unlock Deck
Unlock for access to all 283 flashcards in this deck.
Unlock Deck
k this deck
26
Use a determinant and the given vertices of a triangle to find the area of the triangle. 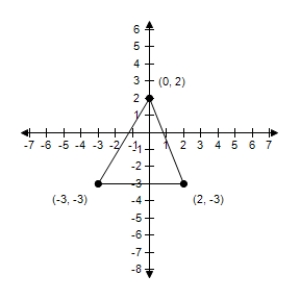
A)27
B)
C)29
D)
E)

A)27
B)
C)29
D)
E)

Unlock Deck
Unlock for access to all 283 flashcards in this deck.
Unlock Deck
k this deck
27
Use a determinant to determine whether the points (-5, 1), (-8, -1) and (1, 5) are collinear.
A)
B)
A)
B)

Unlock Deck
Unlock for access to all 283 flashcards in this deck.
Unlock Deck
k this deck
28
Use a determinant and the given vertices of a triangle to find the area of the triangle. 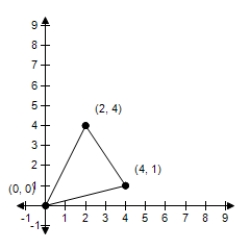
A)
B)4
C)
D)
E)7

A)
B)4
C)
D)
E)7

Unlock Deck
Unlock for access to all 283 flashcards in this deck.
Unlock Deck
k this deck
29
Use a determinant to find the area of the triangle shown below. 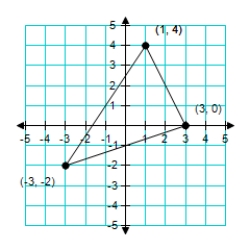
A)14 square units
B) square units
C) square units
D)12 square units
E) square units

A)14 square units
B) square units
C) square units
D)12 square units
E) square units

Unlock Deck
Unlock for access to all 283 flashcards in this deck.
Unlock Deck
k this deck
30
Determine a positive value for y such that a triangle with vertices (-4, 2), (6, 4) and (0, y) has an area of 16 square units.
A)11
B)8
C)6
D)9
E)1
A)11
B)8
C)6
D)9
E)1

Unlock Deck
Unlock for access to all 283 flashcards in this deck.
Unlock Deck
k this deck
31
Use a determinant and the given vertices of a triangle to find the area of the triangle. 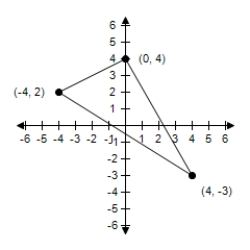
A)
B)
C)18
D)38
E)40

A)
B)
C)18
D)38
E)40

Unlock Deck
Unlock for access to all 283 flashcards in this deck.
Unlock Deck
k this deck
32
Use determinants to find the area of a triangle with the given vertices and confirm your answer by plotting the points in a coordinate plane and using the formula .
(5, -3), (2, -2), (7, 5)
A)21
B)13
C)15
D)19
E)17
(5, -3), (2, -2), (7, 5)
A)21
B)13
C)15
D)19
E)17

Unlock Deck
Unlock for access to all 283 flashcards in this deck.
Unlock Deck
k this deck
33
You inherited a triangular piece of property after your Uncle Izzy passed away.You want to know the size of land, so you "step it off" to estimate the square footage.From the southernmost vertex A, you travel north 300 feet then west 220 feet (for vertex C), and from the southernmost vertex A, you travel 420 feet north then 50 feet west (for vertex B).Use a graphing utility to approximate the number of square feet of land that you have inherited. 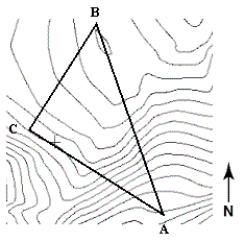
A)3,000 square feet
B)38,700 square feet
C)53,700 square feet
D)43,500 square feet
E)22,500 square feet

A)3,000 square feet
B)38,700 square feet
C)53,700 square feet
D)43,500 square feet
E)22,500 square feet

Unlock Deck
Unlock for access to all 283 flashcards in this deck.
Unlock Deck
k this deck
34
Use Cramer's Rule to solve the following system of linear equations:
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 283 flashcards in this deck.
Unlock Deck
k this deck
35
A large region of forest has been infested with gypsy moths.The region is roughly triangular, as shown in the figure on the next page.From the northernmost vertex A of the region, the distances to the other vertices are x = 25 miles south and 10 miles east (for vertex B), and 20 miles south and 28 miles east (for vertex C).Use a graphing utility to approximate the number of square miles in this region. 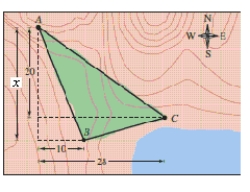
A)250 mi2
B)280 mi2
C)260 mi2
D)290 mi2
E)270 mi2

A)250 mi2
B)280 mi2
C)260 mi2
D)290 mi2
E)270 mi2

Unlock Deck
Unlock for access to all 283 flashcards in this deck.
Unlock Deck
k this deck
36
Use a determinant and the given vertices of a triangle to find the area of the triangle. 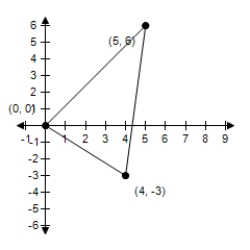
A)
B)21
C)
D)20
E)

A)
B)21
C)
D)20
E)

Unlock Deck
Unlock for access to all 283 flashcards in this deck.
Unlock Deck
k this deck
37
Use a determinant to find an equation of the line passing through the points.
(0, 0), (-8, 2)
A)2x + 8y = 0
B)8x - 2y = 2
C)2x + 8y = 8
D)8x - 2y = 0
E)2x - 8y = 0
(0, 0), (-8, 2)
A)2x + 8y = 0
B)8x - 2y = 2
C)2x + 8y = 8
D)8x - 2y = 0
E)2x - 8y = 0

Unlock Deck
Unlock for access to all 283 flashcards in this deck.
Unlock Deck
k this deck
38
A hair product company sells three types of hair products for $30, $20 and $10 per unit.In one year, the total revenue for the three products was $820,000 which corresponded to the sale of 42,000 units.The company sold half as many units of the $30 products as units of the $20 product.Use Cramer's Rule to solve a system of linear equations to find how many units of each product were sold.
A)10,000 units at $30, 20,000 units at $20, 12,000 units at $10.
B)10,000 units at $30, 20,000 units at $20, 18,000 units at $10.
C)10,000 units at $30, 20,000 units at $20, 20,000 units at $10.
D)10,000 units at $30, 20,000 units at $20, 14,000 units at $10.
E)10,000 units at $30, 20,000 units at $20, 16,000 units at $10.
A)10,000 units at $30, 20,000 units at $20, 12,000 units at $10.
B)10,000 units at $30, 20,000 units at $20, 18,000 units at $10.
C)10,000 units at $30, 20,000 units at $20, 20,000 units at $10.
D)10,000 units at $30, 20,000 units at $20, 14,000 units at $10.
E)10,000 units at $30, 20,000 units at $20, 16,000 units at $10.

Unlock Deck
Unlock for access to all 283 flashcards in this deck.
Unlock Deck
k this deck
39
Use a determinant and the given vertices of a triangle to find the area of the triangle. 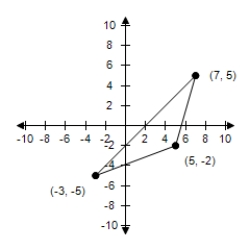
A)52
B)
C)25
D)
E)54

A)52
B)
C)25
D)
E)54

Unlock Deck
Unlock for access to all 283 flashcards in this deck.
Unlock Deck
k this deck
40
Find a value of y such that the triangle with the given vertices has an area of 40 square units.
(7, 0), (7, -5), (-9, y)
A)y = -9 or y = -10
B)y = 7 or y = -3
C)y = 9 or y = 0
D)y = 8 or y = 3
E)y = 10 or y = 8
(7, 0), (7, -5), (-9, y)
A)y = -9 or y = -10
B)y = 7 or y = -3
C)y = 9 or y = 0
D)y = 8 or y = 3
E)y = 10 or y = 8

Unlock Deck
Unlock for access to all 283 flashcards in this deck.
Unlock Deck
k this deck
41
Write a cryptogram for the message "TWO IF BY LAND" using the matrix 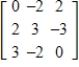 .Show all your work.
.Show all your work.
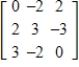 .Show all your work.
.Show all your work.
Unlock Deck
Unlock for access to all 283 flashcards in this deck.
Unlock Deck
k this deck
42
Write a cryptogram for the message "MERRY CHRISTMAS" using the matrix 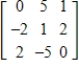 .Show all your work.
.Show all your work.
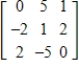 .Show all your work.
.Show all your work.

Unlock Deck
Unlock for access to all 283 flashcards in this deck.
Unlock Deck
k this deck
43
Write a cryptogram for the message "MERRY CHRISTMAS" using the matrix, by assigning a number to each letter in the alphabet (with 0 assigned to a blank space, 0 = _, 1 = A, 2 = B and so on.) .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 283 flashcards in this deck.
Unlock Deck
k this deck
44
Use a determinant to find an equation of the line passing through the points (-5, -1) and (2, 4).
A)5x + 7y - 18 = 0
B)-5x - 7y - 18 = 0
C)-5x + 7y + 18 = 0
D)5x - 7y - 18 = 0
E)-5x + 7y - 18 = 0
A)5x + 7y - 18 = 0
B)-5x - 7y - 18 = 0
C)-5x + 7y + 18 = 0
D)5x - 7y - 18 = 0
E)-5x + 7y - 18 = 0

Unlock Deck
Unlock for access to all 283 flashcards in this deck.
Unlock Deck
k this deck
45
Find the determinant of the matrix.
A)15
B)13
C)
D)
E)
A)15
B)13
C)
D)
E)

Unlock Deck
Unlock for access to all 283 flashcards in this deck.
Unlock Deck
k this deck
46
Determine a positive value for y such that a triangle with vertices , and has an area of 17 square units.
A)8
B)2
C)9
D)11
E)1
A)8
B)2
C)9
D)11
E)1

Unlock Deck
Unlock for access to all 283 flashcards in this deck.
Unlock Deck
k this deck
47
Use a determinant to determine whether the points 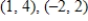 and
and 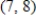 are collinear.Show all work.
are collinear.Show all work.
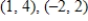 and
and 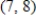 are collinear.Show all work.
are collinear.Show all work.

Unlock Deck
Unlock for access to all 283 flashcards in this deck.
Unlock Deck
k this deck
48
Write a cryptogram by assigning a number to each letter in the alphabet such as 1 = A, 2 = B, 3 = C and so on (with 0 assigned to a blank space) for the message "TWO IF BY LAND" using the following matrix.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 283 flashcards in this deck.
Unlock Deck
k this deck
49
Find the uncoded 1 × 3 row matrices for the message "TWO IF BY LAND" by assigning a number to each letter in the alphabet such as 1 = A, 2 = B, 3 = C and so on (with 0 assigned to a blank space);
Then encode the message using the encoding matrix .
A)Uncoded: Encoded:
B)Uncoded:![<strong>Find the uncoded 1 × 3 row matrices for the message TWO IF BY LAND by assigning a number to each letter in the alphabet such as 1 = A, 2 = B, 3 = C and so on (with 0 assigned to a blank space); Then encode the message using the encoding matrix \left[ \begin{array} { c c c } 0 & 3 & - 3 \\ - 3 & - 3 & 3 \\ - 3 & 3 & 0 \end{array} \right] . </strong> A)Uncoded: \begin{array} { l } { \left[ \begin{array} { l l l } 20 & 23 & 15 \end{array} \right] \left[ \begin{array} { l l l } 0 & 9 & 6 \end{array} \right] \left[ \begin{array} { l l l } 0 & 2 & 25 \end{array} \right] \left[ \begin{array} { l l l } 0 & 12 & 1 \end{array} \right] } \\ \begin{array} { l l l l l l l l l l l l } T & \text { W } & \text { O } &&& \text { IF } &&&& \text { B Y } &&& \text { L A} \end{array} \\ { \left[ \begin{array} { l l l } 14 & 4 & 0 \end{array} \right] } \\ \mathrm { N } \mathrm { D } \\ \end{array} Encoded: \begin{array} { l } { \left[ \begin{array} { l l l } - 114 & 36 & 9 \end{array} \right] \left[ \begin{array} { l l l } - 45 & - 9 & 27 \end{array} \right] \left[ \begin{array} { l l l } - 81 & 69 & 6 \end{array} \right] } \\ { \left[ \begin{array} { l l l } - 39 & - 33 & 36 \end{array} \right] \left[ \begin{array} { l l l } - 12 & 30 & - 30 \end{array} \right] } \end{array} B)Uncoded: \begin{array} { l } { \left[ \begin{array} { l l l } 20 & 23 & 15 \end{array} \right] \left[ \begin{array} { l l l } 0 & 9 & 6 \end{array} \right] \left[ \begin{array} { l l l } 0 & 2 & 25 \end{array} \right] \left[ \begin{array} { l l l } 0 & 12 & 1 \end{array} \right] } \\ \begin{array} { l l l l l l l l l l l l } T & \text { W } & \text { O } &&& \text { IF } &&&& \text { B Y } &&& \text { L A} \end{array} \\ { \left[ \begin{array} { l l l } 14 & 4 & 0 \end{array} \right] } \\ \mathrm { N } \mathrm { D } \\ \end{array} Encoded: \begin{array} { l } { \left[ \begin{array} { l l l } - 81 & 69 & 6 \end{array} \right] \left[ \begin{array} { l l l } - 39 & - 33 & 36 \end{array} \right] \left[ \begin{array} { l l l } - 12 & 30 & - 30 \end{array} \right] } \\ { \left[ \begin{array} { l l l l } - 114 & 36 & 9 \end{array} \right] \left[ \begin{array} { l l l } - 45 & - 9 & 27 \end{array} \right] } \end{array} C)Uncoded: \begin{array} { l } { \left[ \begin{array} { l l l } 20 & 23 & 15 \end{array} \right] \left[ \begin{array} { l l l } 0 & 9 & 6 \end{array} \right] \left[ \begin{array} { l l l } 0 & 2 & 25 \end{array} \right] \left[ \begin{array} { l l l } 0 & 12 & 1 \end{array} \right] } \\ \begin{array} { l l l l l l l l l l l l } T & \text { W } & \text { O } &&& \text { IF } &&&& \text { B Y } &&& \text { L A} \end{array} \\ { \left[ \begin{array} { l l l } 14 & 4 & 0 \end{array} \right] } \\ \mathrm { N } \mathrm { D } \\ \end{array} Encoded: \begin{array} { l } { \left[ \begin{array} { l l l } - 39 & - 33 & 36 \end{array} \right] \left[ \begin{array} { l l l } - 12 & 30 & - 30 \end{array} \right] \left[ \begin{array} { l l l l } - 114 & 36 & 9 \end{array} \right] } \\ { \left[ \begin{array} { l l l } - 45 & - 9 & 27 \end{array} \right] \left[ \begin{array} { l l l } - 81 & 69 & 6 \end{array} \right] } \end{array} D)Uncoded: \begin{array} { l } { \left[ \begin{array} { l l l } 20 & 23 & 15 \end{array} \right] \left[ \begin{array} { l l l } 0 & 9 & 6 \end{array} \right] \left[ \begin{array} { l l l } 0 & 2 & 25 \end{array} \right] \left[ \begin{array} { l l l } 0 & 12 & 1 \end{array} \right] } \\ \begin{array} { l l l l l l l l l l l l } T & \text { W } & \text { O } &&& \text { IF } &&&& \text { B Y } &&& \text { L A} \end{array} \\ { \left[ \begin{array} { l l l } 14 & 4 & 0 \end{array} \right] } \\ \mathrm { N } \mathrm { D } \\ \end{array} Encoded: \begin{array} { l } { \left[ \begin{array} { l l l } - 12 & 30 & - 30 \end{array} \right] \left[ \begin{array} { l l l l } - 114 & 36 & 9 \end{array} \right] \left[ \begin{array} { l l l } - 45 & - 9 & 27 \end{array} \right] } \\ { \left[ \begin{array} { l l l } - 81 & 69 & 6 \end{array} \right] \left[ \begin{array} { l l l l } - 39 & - 33 & 36 \end{array} \right] } \end{array} E)Uncoded: \begin{array} { l } { \left[ \begin{array} { l l l } 20 & 23 & 15 \end{array} \right] \left[ \begin{array} { l l l } 0 & 9 & 6 \end{array} \right] \left[ \begin{array} { l l l } 0 & 2 & 25 \end{array} \right] \left[ \begin{array} { l l l } 0 & 12 & 1 \end{array} \right] } \\ \begin{array} { l l l l l l l l l l l l } T & \text { W } & \text { O } &&& \text { IF } &&&& \text { B Y } &&& \text { L A} \end{array} \\ { \left[ \begin{array} { l l l } 14 & 4 & 0 \end{array} \right] } \\ \mathrm { N } \mathrm { D } \\ \end{array} Encoded: \begin{array} { l } { \left[ \begin{array} { l l l } - 45 & - 9 & 27 \end{array} \right] \left[ \begin{array} { l l l } - 81 & 69 & 6 \end{array} \right] \left[ \begin{array} { l l l } - 39 & - 33 & 36 \end{array} \right] } \\ { \left[ \begin{array} { l l l } - 12 & 30 & - 30 \end{array} \right] \left[ \begin{array} { l l l } - 114 & 36 & 9 \end{array} \right] } \end{array}](https://d2lvgg3v3hfg70.cloudfront.net/TB7698/11eae838_9cfa_8e21_a6c3_05353448a8d3_TB7698_00.jpg) Encoded:
Encoded:
C)Uncoded: Encoded:
D)Uncoded: Encoded:
E)Uncoded: Encoded:
Then encode the message using the encoding matrix .
A)Uncoded: Encoded:
B)Uncoded:
![<strong>Find the uncoded 1 × 3 row matrices for the message TWO IF BY LAND by assigning a number to each letter in the alphabet such as 1 = A, 2 = B, 3 = C and so on (with 0 assigned to a blank space); Then encode the message using the encoding matrix \left[ \begin{array} { c c c } 0 & 3 & - 3 \\ - 3 & - 3 & 3 \\ - 3 & 3 & 0 \end{array} \right] . </strong> A)Uncoded: \begin{array} { l } { \left[ \begin{array} { l l l } 20 & 23 & 15 \end{array} \right] \left[ \begin{array} { l l l } 0 & 9 & 6 \end{array} \right] \left[ \begin{array} { l l l } 0 & 2 & 25 \end{array} \right] \left[ \begin{array} { l l l } 0 & 12 & 1 \end{array} \right] } \\ \begin{array} { l l l l l l l l l l l l } T & \text { W } & \text { O } &&& \text { IF } &&&& \text { B Y } &&& \text { L A} \end{array} \\ { \left[ \begin{array} { l l l } 14 & 4 & 0 \end{array} \right] } \\ \mathrm { N } \mathrm { D } \\ \end{array} Encoded: \begin{array} { l } { \left[ \begin{array} { l l l } - 114 & 36 & 9 \end{array} \right] \left[ \begin{array} { l l l } - 45 & - 9 & 27 \end{array} \right] \left[ \begin{array} { l l l } - 81 & 69 & 6 \end{array} \right] } \\ { \left[ \begin{array} { l l l } - 39 & - 33 & 36 \end{array} \right] \left[ \begin{array} { l l l } - 12 & 30 & - 30 \end{array} \right] } \end{array} B)Uncoded: \begin{array} { l } { \left[ \begin{array} { l l l } 20 & 23 & 15 \end{array} \right] \left[ \begin{array} { l l l } 0 & 9 & 6 \end{array} \right] \left[ \begin{array} { l l l } 0 & 2 & 25 \end{array} \right] \left[ \begin{array} { l l l } 0 & 12 & 1 \end{array} \right] } \\ \begin{array} { l l l l l l l l l l l l } T & \text { W } & \text { O } &&& \text { IF } &&&& \text { B Y } &&& \text { L A} \end{array} \\ { \left[ \begin{array} { l l l } 14 & 4 & 0 \end{array} \right] } \\ \mathrm { N } \mathrm { D } \\ \end{array} Encoded: \begin{array} { l } { \left[ \begin{array} { l l l } - 81 & 69 & 6 \end{array} \right] \left[ \begin{array} { l l l } - 39 & - 33 & 36 \end{array} \right] \left[ \begin{array} { l l l } - 12 & 30 & - 30 \end{array} \right] } \\ { \left[ \begin{array} { l l l l } - 114 & 36 & 9 \end{array} \right] \left[ \begin{array} { l l l } - 45 & - 9 & 27 \end{array} \right] } \end{array} C)Uncoded: \begin{array} { l } { \left[ \begin{array} { l l l } 20 & 23 & 15 \end{array} \right] \left[ \begin{array} { l l l } 0 & 9 & 6 \end{array} \right] \left[ \begin{array} { l l l } 0 & 2 & 25 \end{array} \right] \left[ \begin{array} { l l l } 0 & 12 & 1 \end{array} \right] } \\ \begin{array} { l l l l l l l l l l l l } T & \text { W } & \text { O } &&& \text { IF } &&&& \text { B Y } &&& \text { L A} \end{array} \\ { \left[ \begin{array} { l l l } 14 & 4 & 0 \end{array} \right] } \\ \mathrm { N } \mathrm { D } \\ \end{array} Encoded: \begin{array} { l } { \left[ \begin{array} { l l l } - 39 & - 33 & 36 \end{array} \right] \left[ \begin{array} { l l l } - 12 & 30 & - 30 \end{array} \right] \left[ \begin{array} { l l l l } - 114 & 36 & 9 \end{array} \right] } \\ { \left[ \begin{array} { l l l } - 45 & - 9 & 27 \end{array} \right] \left[ \begin{array} { l l l } - 81 & 69 & 6 \end{array} \right] } \end{array} D)Uncoded: \begin{array} { l } { \left[ \begin{array} { l l l } 20 & 23 & 15 \end{array} \right] \left[ \begin{array} { l l l } 0 & 9 & 6 \end{array} \right] \left[ \begin{array} { l l l } 0 & 2 & 25 \end{array} \right] \left[ \begin{array} { l l l } 0 & 12 & 1 \end{array} \right] } \\ \begin{array} { l l l l l l l l l l l l } T & \text { W } & \text { O } &&& \text { IF } &&&& \text { B Y } &&& \text { L A} \end{array} \\ { \left[ \begin{array} { l l l } 14 & 4 & 0 \end{array} \right] } \\ \mathrm { N } \mathrm { D } \\ \end{array} Encoded: \begin{array} { l } { \left[ \begin{array} { l l l } - 12 & 30 & - 30 \end{array} \right] \left[ \begin{array} { l l l l } - 114 & 36 & 9 \end{array} \right] \left[ \begin{array} { l l l } - 45 & - 9 & 27 \end{array} \right] } \\ { \left[ \begin{array} { l l l } - 81 & 69 & 6 \end{array} \right] \left[ \begin{array} { l l l l } - 39 & - 33 & 36 \end{array} \right] } \end{array} E)Uncoded: \begin{array} { l } { \left[ \begin{array} { l l l } 20 & 23 & 15 \end{array} \right] \left[ \begin{array} { l l l } 0 & 9 & 6 \end{array} \right] \left[ \begin{array} { l l l } 0 & 2 & 25 \end{array} \right] \left[ \begin{array} { l l l } 0 & 12 & 1 \end{array} \right] } \\ \begin{array} { l l l l l l l l l l l l } T & \text { W } & \text { O } &&& \text { IF } &&&& \text { B Y } &&& \text { L A} \end{array} \\ { \left[ \begin{array} { l l l } 14 & 4 & 0 \end{array} \right] } \\ \mathrm { N } \mathrm { D } \\ \end{array} Encoded: \begin{array} { l } { \left[ \begin{array} { l l l } - 45 & - 9 & 27 \end{array} \right] \left[ \begin{array} { l l l } - 81 & 69 & 6 \end{array} \right] \left[ \begin{array} { l l l } - 39 & - 33 & 36 \end{array} \right] } \\ { \left[ \begin{array} { l l l } - 12 & 30 & - 30 \end{array} \right] \left[ \begin{array} { l l l } - 114 & 36 & 9 \end{array} \right] } \end{array}](https://d2lvgg3v3hfg70.cloudfront.net/TB7698/11eae838_9cfa_8e21_a6c3_05353448a8d3_TB7698_00.jpg) Encoded:
Encoded: C)Uncoded: Encoded:
D)Uncoded: Encoded:
E)Uncoded: Encoded:

Unlock Deck
Unlock for access to all 283 flashcards in this deck.
Unlock Deck
k this deck
50
Find the uncoded 1 × 3 row matrices for the message "MERRY CHRISTMAS" by assigning a number to each letter in the alphabet such as 1 = A, 2 = B, 3 = C and so on (with 0 assigned to a blank space);
Then encode the message using the encoding matrix .
A)Uncoded: Encoded:
B)Uncoded: Encoded:
C)Uncoded: Encoded:
D)Uncoded: Encoded:
E)Uncoded: Encoded:
Then encode the message using the encoding matrix .
A)Uncoded: Encoded:
B)Uncoded: Encoded:
C)Uncoded: Encoded:
D)Uncoded: Encoded:
E)Uncoded: Encoded:

Unlock Deck
Unlock for access to all 283 flashcards in this deck.
Unlock Deck
k this deck
51
Find the uncoded 1 × 3 row matrices for the message "MERRY CHRISTMAS"; then encode the message using the encoding matrix 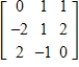 .Show all your work.
.Show all your work.
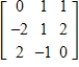 .Show all your work.
.Show all your work.
Unlock Deck
Unlock for access to all 283 flashcards in this deck.
Unlock Deck
k this deck
52
Find the determinant of the matrix.
A)0
B)
C)
D)
E)
A)0
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 283 flashcards in this deck.
Unlock Deck
k this deck
53
Use Cramer's rule to find the solution of the system, if possible.
A)x = 2, y = -2
B)x = 5, y = -12
C)x = 5, y = -5
D)The system is inconsistent.
E)The equations are dependent.
A)x = 2, y = -2
B)x = 5, y = -12
C)x = 5, y = -5
D)The system is inconsistent.
E)The equations are dependent.

Unlock Deck
Unlock for access to all 283 flashcards in this deck.
Unlock Deck
k this deck
54
Use determinants to find the area of the triangle with vertices at the given points.
P(0, 0), Q(4, 0), R(4, 3)
A)A = 12
B)A = 4
C)A = 6
D)A = 3
E)none of these
P(0, 0), Q(4, 0), R(4, 3)
A)A = 12
B)A = 4
C)A = 6
D)A = 3
E)none of these

Unlock Deck
Unlock for access to all 283 flashcards in this deck.
Unlock Deck
k this deck
55
Use a determinant to determine whether the points (-3, -9), (-5, -11) and (0, -7) are collinear.
A)
B)
A)
B)

Unlock Deck
Unlock for access to all 283 flashcards in this deck.
Unlock Deck
k this deck
56
Find the uncoded 1 × 3 row matrices for the message "TWO IF BY LAND"; then encode the message using the encoding matrix 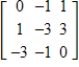 .Show all your work.
.Show all your work.
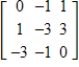 .Show all your work.
.Show all your work.
Unlock Deck
Unlock for access to all 283 flashcards in this deck.
Unlock Deck
k this deck
57
Find the determinant of the matrix.
A)32
B)
C)
D)0
E)
A)32
B)
C)
D)0
E)

Unlock Deck
Unlock for access to all 283 flashcards in this deck.
Unlock Deck
k this deck
58
Use Cramer's Rule to solve the following system of linear equations:
A) x = , y = , z =
B) x = , y = , z =
C) x = , y = , z =
D) x = , y = , z =
E) x = , y = , z =
A) x = , y = , z =
B) x = , y = , z =
C) x = , y = , z =
D) x = , y = , z =
E) x = , y = , z =

Unlock Deck
Unlock for access to all 283 flashcards in this deck.
Unlock Deck
k this deck
59
Find the determinant of the matrix.
A)
B)
C)
D)0
E)
A)
B)
C)
D)0
E)

Unlock Deck
Unlock for access to all 283 flashcards in this deck.
Unlock Deck
k this deck
60
Use a determinant to find y such that , and are collinear.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 283 flashcards in this deck.
Unlock Deck
k this deck
61
Find the determinant of the matrix by the method of expansion by cofactors.Expand using the column 2.
A)-75
B)75
C)-73
D)-74
E)-76
A)-75
B)75
C)-73
D)-74
E)-76

Unlock Deck
Unlock for access to all 283 flashcards in this deck.
Unlock Deck
k this deck
62
Find all minors of the matrix.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 283 flashcards in this deck.
Unlock Deck
k this deck
63
Find the determinant of the matrix.
A)
B)
C)0
D)16
E)
A)
B)
C)0
D)16
E)

Unlock Deck
Unlock for access to all 283 flashcards in this deck.
Unlock Deck
k this deck
64
Find all minors of the matrix.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 283 flashcards in this deck.
Unlock Deck
k this deck
65
Find the determinant of the matrix.
A)
B)0
C)
D)10
E)
A)
B)0
C)
D)10
E)

Unlock Deck
Unlock for access to all 283 flashcards in this deck.
Unlock Deck
k this deck
66
Find the determinant of the matrix by the method of expansion by cofactors.Expand using the row 1.
A)-73
B)75
C)-75
D)-74
E)-76
A)-73
B)75
C)-75
D)-74
E)-76

Unlock Deck
Unlock for access to all 283 flashcards in this deck.
Unlock Deck
k this deck
67
Use the matrix capabilities of a graphing utility to evaluate the determinant.
A)-72
B)-71
C)-70
D)-74
E)-73
A)-72
B)-71
C)-70
D)-74
E)-73

Unlock Deck
Unlock for access to all 283 flashcards in this deck.
Unlock Deck
k this deck
68
Find all the cofactors of the matrix.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 283 flashcards in this deck.
Unlock Deck
k this deck
69
Find all minors of the matrix.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 283 flashcards in this deck.
Unlock Deck
k this deck
70
Find the determinant of the matrix.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 283 flashcards in this deck.
Unlock Deck
k this deck
71
Find the determinant of the matrix.Expand by cofactors on the row or column that appears to make the computations easiest.
A)-64
B)-62
C)-66
D)-65
E)-63
A)-64
B)-62
C)-66
D)-65
E)-63

Unlock Deck
Unlock for access to all 283 flashcards in this deck.
Unlock Deck
k this deck
72
Find all the cofactors of the matrix.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 283 flashcards in this deck.
Unlock Deck
k this deck
73
Find all the cofactors of the matrix.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 283 flashcards in this deck.
Unlock Deck
k this deck
74
Find all minors of the matrix.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 283 flashcards in this deck.
Unlock Deck
k this deck
75
Find the determinant of the matrix.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 283 flashcards in this deck.
Unlock Deck
k this deck
76
Find .
A)-25
B)-26
C)-24
D)-23
E)-22
A)-25
B)-26
C)-24
D)-23
E)-22

Unlock Deck
Unlock for access to all 283 flashcards in this deck.
Unlock Deck
k this deck
77
Find all the cofactors of the matrix.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

Unlock Deck
Unlock for access to all 283 flashcards in this deck.
Unlock Deck
k this deck
78
Find the determinant of the matrix.
A)111
B)110
C)
D)
E)
A)111
B)110
C)
D)
E)

Unlock Deck
Unlock for access to all 283 flashcards in this deck.
Unlock Deck
k this deck
79
Find the determinant of the matrix.
A)
B)50
C)51
D)
E)
A)
B)50
C)51
D)
E)

Unlock Deck
Unlock for access to all 283 flashcards in this deck.
Unlock Deck
k this deck
80
Find the determinant of the matrix.
A)-6
B)-7
C)-4
D)-5
E)-8
A)-6
B)-7
C)-4
D)-5
E)-8

Unlock Deck
Unlock for access to all 283 flashcards in this deck.
Unlock Deck
k this deck



