Deck 2: Introduction to Management Science Models
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question
Question

Unlock Deck
Sign up to unlock the cards in this deck!
Unlock Deck
Unlock Deck
1/58
Play
Full screen (f)
Deck 2: Introduction to Management Science Models
1
Minimization linear programming models may not involve " " constraints.
False
2
Linear programming models are a subset of constrained optimization models that require the assumptions of continuity of the variables, certainty of the coefficients, additivity of terms, and proportionality of costs, profits, and the use of resources to the value of the decision variables.
True
3
Binary linear programming allows for the possibility of mutually exclusive constraints.
True
4
"Range of optimality" describes the impact of simultaneous changes in objective function values and right-hand-side values.

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
5
When specifying linear constraints, the modeler must take into account the unit specification of the decision variables so that the units represented by the left side of the constraints are consistent with the units represented by the right side of the constraints.

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
6
A linear programming problem with all " " functional constraints and nonnegative right hand side values will never be infeasible.

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
7
If two extreme points are optimal, then so is every point on the line segment connecting the two extreme points.

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
8
A non-binding constraint is always a redundant constraint.

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
9
In the branch-and-bound technique for solving integer linear programming models with a maximization objective function:
A)Once a feasible solution is found, it is optimal.
B)If the solution generated at one stage is X1 = 5.7, X2 = 3 but gives an objective function value higher than that of the best integer solution found to date, X2 must be either 0, 1, or 2 in the optimal solution.
C)If the solution generated at one stage is X1 = 5.7, X2 = 3 but gives an objective function value higher than that of the best integer solution found to date, a new linear programming problem is solved with two constraints added: X1 6 and X1 5.
D)If the solution generated at one stage is X1 = 5.7, X2 = 3 but gives an objective function value higher than that of the best integer solution found to date, two new linear programs are solved, one with the constraint
A)Once a feasible solution is found, it is optimal.
B)If the solution generated at one stage is X1 = 5.7, X2 = 3 but gives an objective function value higher than that of the best integer solution found to date, X2 must be either 0, 1, or 2 in the optimal solution.
C)If the solution generated at one stage is X1 = 5.7, X2 = 3 but gives an objective function value higher than that of the best integer solution found to date, a new linear programming problem is solved with two constraints added: X1 6 and X1 5.
D)If the solution generated at one stage is X1 = 5.7, X2 = 3 but gives an objective function value higher than that of the best integer solution found to date, two new linear programs are solved, one with the constraint

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
10
When compared with standard linear programming, integer linear programming typically has:
A)more feasible solution points to evaluate.
B)fewer feasible solution points to evaluate.
C)the same number of feasible solution points to evaluate.
D)more linear constraints.
A)more feasible solution points to evaluate.
B)fewer feasible solution points to evaluate.
C)the same number of feasible solution points to evaluate.
D)more linear constraints.

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
11
The complementary slackness principle states that either there is zero slack on a constraint or the reduced cost is zero.

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
12
Compared with standard linear programming algorithms, those for treating integer linear programming problems usually are:
A)less complex due to fewer possible solutions.
B)less amenable to "what if" analysis.
C)always heuristic.
D)easier to formulate.
A)less complex due to fewer possible solutions.
B)less amenable to "what if" analysis.
C)always heuristic.
D)easier to formulate.

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
13
One of the reasons we cannot use sensitivity analysis for an integer linear program is that the shadow prices do not produce linear effects.That is, although in a linear program the shadow price for a resource represents the marginal improvement for each added unit of that resource, in an integer linear program, we cannot assume that each added unit of a resource will produce the same marginal change.

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
14
Each decision variable must appear in at least two constraints.

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
15
The difference between a boundary point and an extreme point is the number of constraints satisfied.

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
16
In solving an integer linear programming problem, lifting the integer requirements and first solving the underlying standard linear programming problem:
A)never yields an optimal solution.
B)is not part of the branch-and-bound technique.
C)is not part of the cutting plane technique.
D)may yield an integer-valued solution.
A)never yields an optimal solution.
B)is not part of the branch-and-bound technique.
C)is not part of the cutting plane technique.
D)may yield an integer-valued solution.

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
17
An extreme point is an optimal solution.

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
18
An optimal solution must have no slack on at least one constraint.

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
19
Which of the following is not a necessary linear programming assumption?
A)The decision variable values are discrete.
B)The parameters are specified with certainty.
C)Constant returns to scale in the linear constraints and the object function coefficients.
D)No interactions permitted between decision variables.
A)The decision variable values are discrete.
B)The parameters are specified with certainty.
C)Constant returns to scale in the linear constraints and the object function coefficients.
D)No interactions permitted between decision variables.

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
20
Linear programming and integer linear programming both yield a great amount of sensitivity analysis.

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
21
Banner Tools produces two styles of steel hammers with wooden handles.The first sells for $6 and consists of .5 pounds of steel; the second sells for $15 and consists of 1 pound of steel.Since steel costs the firm $4 per pound and the handle, labor, and packaging costs amount to $1 for either hammer, the profits coefficients are $6 - .5($4) - $1 = $3 for the smaller hammer and $15 - 1($4) - $1 = $10 for the larger hammer.Thus the objective function for this model is MAX 3X1 + 10X2.Given that the shadow price for steel is $2, which of the following statements is correct?
A)Banner should not buy more steel.
B)Banner should buy all the steel it can only if it can purchase it for less than $2 per pound.
C)Banner should buy at least as much as the "ALLOWABLE INCREASE", but only if it can be purchased for less than $2 per pound.
D)Banner should buy at least as much as the "ALLOWABLE INCREASE", but only if it can be purchase for less than $6 per pound.
A)Banner should not buy more steel.
B)Banner should buy all the steel it can only if it can purchase it for less than $2 per pound.
C)Banner should buy at least as much as the "ALLOWABLE INCREASE", but only if it can be purchased for less than $2 per pound.
D)Banner should buy at least as much as the "ALLOWABLE INCREASE", but only if it can be purchase for less than $6 per pound.

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
22
The effect of deleting a linear constraint from a linear programming model depends on whether or not that constraint:
A)is a " " or a " " constraint.
B)had negative coefficients.
C)is redundant.
D)is binding.
A)is a " " or a " " constraint.
B)had negative coefficients.
C)is redundant.
D)is binding.

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
23
Compare the points that the Simplex method moves to with the points touched by the graphical method for solving a two variable linear program.

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
24
A "non-binding" constraint is:
A)redundant.
B)not satisfied with an equality at the optimal solution.
C)one having zero slack or surplus
D)never a non-negativity variable constraint.
A)redundant.
B)not satisfied with an equality at the optimal solution.
C)one having zero slack or surplus
D)never a non-negativity variable constraint.

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
25
Which statement is not true if a maximization problem has an unbounded solution?
A)A data entry error has been made or a limiting constraint has been omitted.
B)The objective function value goes to + .
C)The values of all decision variables go to + .
D)The feasible region is unbounded.
A)A data entry error has been made or a limiting constraint has been omitted.
B)The objective function value goes to + .
C)The values of all decision variables go to + .
D)The feasible region is unbounded.

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
26
Define the additivity assumption.

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
27
What is a linear programming model?

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
28
What is wrong with this linear programming model?

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
29
The feasible region does not include:
A)interior points.
B)boundary points.
C)points at which at least one of the decision variables is zero.
D)points which violate at least one of the functional or non-negativity constraints.
A)interior points.
B)boundary points.
C)points at which at least one of the decision variables is zero.
D)points which violate at least one of the functional or non-negativity constraints.

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
30
Dean Air uses a linear programming model to schedule flights, assign crews and meet passenger demand.The objective function is to minimize downtime.Constraints include a limit on pilot hours, meeting passenger demand, and scheduled downtime.Which of the following cannot be accomplished with sensitivity analysis?
A)Add a constraint of airport gate usage.
B)Change the objective to maximize profits.
C)Increase values for demand.
D)Drop the crew assignment constraint from the model.
A)Add a constraint of airport gate usage.
B)Change the objective to maximize profits.
C)Increase values for demand.
D)Drop the crew assignment constraint from the model.

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
31
The functional constraints of a linear model with nonnegative variables are 3X1 + 5X2 16 and 4X1 + X2 10.Which of the following points could not be an optimal solution for the model?
A)X1 = 2.5, X2 = 0
B)X1 = 0, X2 = 3.2
C)X1 = 1, X2 = 2.25
D)X1 = 2, X2 = 2
A)X1 = 2.5, X2 = 0
B)X1 = 0, X2 = 3.2
C)X1 = 1, X2 = 2.25
D)X1 = 2, X2 = 2

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
32
An over-constrained linear programming problem results in what type of solution?
A)Unbounded.
B)Degenerate.
C)Infeasible.
D)Sub-optimal.
A)Unbounded.
B)Degenerate.
C)Infeasible.
D)Sub-optimal.

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
33
Excel Solver reports "Solver could not find a feasible solution." What is your best logical alternative?
A)Change the objective function.
B)Run Excel Solver again.
C)Relax a constraint.
D)Add a constraint.
A)Change the objective function.
B)Run Excel Solver again.
C)Relax a constraint.
D)Add a constraint.

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
34
The objective function coefficients for X1, X2, and X3 are 15, 32, and 48 respectively.Excel prints that their ranges of optimality are from 10 to 20, from 30 to 40, and from - to 50 respectively.If the objective function coefficients are changed to 14, 31, and 45, the optimal solution:
A)will not change.
B)may not change.
C)will definitely change.
D)may change.
A)will not change.
B)may not change.
C)will definitely change.
D)may change.

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
35
If the points (5, 5, 5) and (7, 9, 5) are both optimal solutions to a linear programming problem, what other point is also optimal?
A)(6,7,8)
B)(6,7,5)
C)(7,5,5)
D)(5,9,5)
A)(6,7,8)
B)(6,7,5)
C)(7,5,5)
D)(5,9,5)

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
36
Squire Leathers produces two sizes of wallets from cowhide.The first requires 60 squire inches of cowhide and the second requires 100 square inches.The company has 1000 square feet of cowhide.Part of the model is:
A)60X1 + 100X2 144,000
B)60X1 + 100X2 144,000
C)60X1 + 100X2 = 144,000
D)60X1 144,000 and 100X2 144,000
A)60X1 + 100X2 144,000
B)60X1 + 100X2 144,000
C)60X1 + 100X2 = 144,000
D)60X1 144,000 and 100X2 144,000

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
37
Which of the following constraints is redundant?
A)X1 + X2 10
B)X1 - X2 10
C)X1 + 3X2 20
D)X1, X2 0
A)X1 + X2 10
B)X1 - X2 10
C)X1 + 3X2 20
D)X1, X2 0

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
38
Why would you not use linear programming to solve a problem with a single decision variable linear objective function and multiple constraints?

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
39
The principle of "complementary slackness" implies that:
A)if the reduced cost is not zero, than the value of the decision variable is zero.
B)if a decision variable is zero, then its reduced cost must be positive.
C)if a decision variable is zero, then its reduced cost must be non-zero.
D)if a decision variable is zero, then its reduced cost must be zero.
A)if the reduced cost is not zero, than the value of the decision variable is zero.
B)if a decision variable is zero, then its reduced cost must be positive.
C)if a decision variable is zero, then its reduced cost must be non-zero.
D)if a decision variable is zero, then its reduced cost must be zero.

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
40
In a model with two decision variables, the restriction 3X1 + 2X2 6 represents:
A)a straight line.
B)the region of infeasibility.
C)an extreme point.
D)a linear constraint.
A)a straight line.
B)the region of infeasibility.
C)an extreme point.
D)a linear constraint.

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
41
A farmer has three possible cereal grain crops to grow in the coming planting season: barley, oats, and wheat.He has 240 acres of arable land: 160 are considered high grade and the remainder is low grade.He has $42,000 in available capital and can hire virtually unlimited hours of field labor, locally, for $6 per hour.Relevant crop factors are: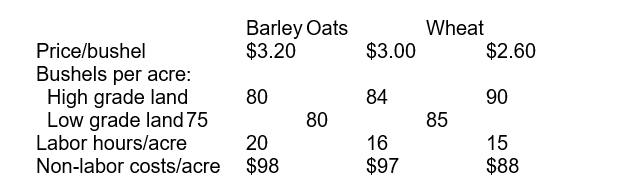 Formulate this as a linear programming model.
Formulate this as a linear programming model.
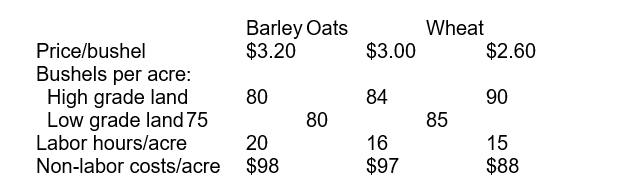 Formulate this as a linear programming model.
Formulate this as a linear programming model.
Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
42
The University of Iowa is experimenting with a blend of soil amendments to be used in an analysis of variance study of the response of tomatoes to various amounts of sunlight.To perform this study, all other elements must be controlled so that the only variable is the sunlight.The minimum requirements for calcium, phosphorous, and potassium are 125 pounds, 150 pounds, and 120 pounds respectively.The soil amendment mixture from Prairie Gold consist of 25% calcium, 25% phosphorous, 12½% potassium, and 37½% other ingredients.It costs $0.40 per ounce.The mixture from Grinell Grow is 20% calcium, 25% phosphorous, 25% potassium, and 30% other ingredients.It sells for $0.50 per ounce.Formulate a linear programming model that will allow the University of Iowa to conduct this experiment using a minimum cost blend of the two soil amendment mixtures.

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
43
Explain the different interpretations of shadow costs when the objective function is based on sunk costs versus included costs.

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
44
The Carolina Chemical Company (CCC) makes two products, soap and shampoo, both in liquid form.The same two raw material inputs, glycerin and potash, and the same production line are used in the manufacture of each.Each production day, one ton of glycerin and two tons of potash are available.Each gallon of soap requires 8 pounds of glycerin and 32 pounds of potash, while a gallon of shampoo uses 12 pounds of glycerin and 19 pounds of potash.
CCC's production day is 8 full hours (480 minutes).Its production line can turn out 25 gallons of soap, or 20 gallons of shampoo, per hour.Only one product can be made at a time, but the line is easily changed over at negligible cost from one item to the other.Forecasts indicate that demand for soap and shampoo far exceeds production capacity.The profit per gallon of soap is $30 and per gallon of shampoo is $36.
A.Formulate a linear programming model that will maximize CCC's daily profit.
B.Suppose that the production of soap also yields a byproduct called Blyx.The manufacturing process yields 0.3 units of Blyx for every unit of soap.Up to 15 gallons of Blyx can be sold per day for canine shampoo at a profit of $10 per gallon.CCC must dispose of any excess of Blyx at a removal cost of $4 per gallon.Using X3 = the number of gallons of Blyx produced up to 15 gallons, and X4 = the number of gallons of Blyx produced over 15 gallons, modify the linear programming model for this problem.
CCC's production day is 8 full hours (480 minutes).Its production line can turn out 25 gallons of soap, or 20 gallons of shampoo, per hour.Only one product can be made at a time, but the line is easily changed over at negligible cost from one item to the other.Forecasts indicate that demand for soap and shampoo far exceeds production capacity.The profit per gallon of soap is $30 and per gallon of shampoo is $36.
A.Formulate a linear programming model that will maximize CCC's daily profit.
B.Suppose that the production of soap also yields a byproduct called Blyx.The manufacturing process yields 0.3 units of Blyx for every unit of soap.Up to 15 gallons of Blyx can be sold per day for canine shampoo at a profit of $10 per gallon.CCC must dispose of any excess of Blyx at a removal cost of $4 per gallon.Using X3 = the number of gallons of Blyx produced up to 15 gallons, and X4 = the number of gallons of Blyx produced over 15 gallons, modify the linear programming model for this problem.

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
45
Explain the SUMPRODUCT function of Excel.

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
46
Office2000 produces expensive, quality 2-drawer and 4-drawer filing cabinets from solid oak for major corporations.A 2-drawer model utilizes 2 labor hours to produce and package for a net profit of $75.The 4-drawer model utilizes 3 labor hours to produce and package and nets a profit of $125.Each month Office2000 has 360 labor hours available and can obtain up to 200 2-drawer frames, 200 4-drawer frames, and 400 drawers from its oak supplier.

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
47
When running Excel, what does "The Set Cell values do not converge" mean?

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
48
What clue does Excel give to the possible existence of alternate optimal solutions?

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
49
"Allowable Decrease" and "Allowable Increase" refer to what range?

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
50
A small foundry has received an order for an iron alloy called Falloy which has the following specifications:
Falloy can be produced from the following inputs (balance to 100% consisting of iron).
Formulate the linear programming problem that will indicate the blending "recipe" that will minimize the cost of this alloy.
Falloy can be produced from the following inputs (balance to 100% consisting of iron).
Formulate the linear programming problem that will indicate the blending "recipe" that will minimize the cost of this alloy.

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
51
Jungle Figures, Inc.produces two models of its stuffed giraffes, which it markets to high-end retail stores.The large giraffe requires 2 pounds of stuffing material and 6 minutes of machine time.The small giraffe requires 1 pound of stuffing material and 12 minutes of machine time since its tighter stitching pattern requires it to be stitched twice.There are 800 pounds of stuffing and 70 machine hours available each week.By adhering to a policy of not producing more than twice the number of large giraffes as small giraffes (this is a constraint), Jungle Figures has been able to sell all the giraffes it produces at $12 per large giraffe and $9 per small giraffe.

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
52
The Finast Filter Company makes two types of filters for domestic air purifiers: the standard (nicknamed the Cleaner) for ordinary use, and the Scrubber for industrial-strength problems.The Cleaners bring in a profit of $0.50 each, while the Scrubbers command a profit of $1.25 each.Due to a higher piece-rate wage for the Scrubbers, the labor union was able to negotiate a requirement that each day the number of Scrubber filters produced will be at least as great as the number of Cleaners.The production line can turn out 25 Scrubbers per minute or 75 Cleaners per minute.Only one type of filter can be made at a time, but the production line is easily changed over at a negligible cost from one kind of filter production to the other.
FFC must produce at least 5,000 Cleaners today to fill a rush order.The production day is 8 full hours (480 minutes).
Formulate a linear programming model to determine how many of each filter should be produced today to maximize profit.
FFC must produce at least 5,000 Cleaners today to fill a rush order.The production day is 8 full hours (480 minutes).
Formulate a linear programming model to determine how many of each filter should be produced today to maximize profit.

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
53
Consider the following sensitivity report from Excel for the model in question 3, which has a profit of $5,400.
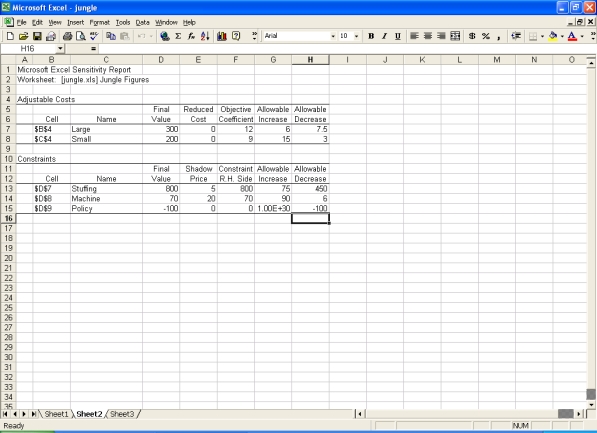
A.If Jungle Figures eliminates its policy of not producing more than twice the number of large giraffes as small giraffes, how will this affect the optimal solution?
B.Suppose 50 pounds of extra stuffing could be purchased for $200.Should Jungle do this? Would the optimal solution change?
C.Jungle is considering diverting 10 hours of machine time from the production of other products to produce giraffes.It figures it will lose $225 in gross profits from those products by this action.Should Jungle do this? Would the optimal solution change?
D.Suppose that demand is such that Jungle could effectively raise its prices to $15 on each giraffe (large or small).Would the optimal production schedule change?
E.Suppose demand for small giraffes waned, and Jungle cut its process so that its profit on small giraffes was reduced by 50% to $4.50.Would the optimal solution change?

A.If Jungle Figures eliminates its policy of not producing more than twice the number of large giraffes as small giraffes, how will this affect the optimal solution?
B.Suppose 50 pounds of extra stuffing could be purchased for $200.Should Jungle do this? Would the optimal solution change?
C.Jungle is considering diverting 10 hours of machine time from the production of other products to produce giraffes.It figures it will lose $225 in gross profits from those products by this action.Should Jungle do this? Would the optimal solution change?
D.Suppose that demand is such that Jungle could effectively raise its prices to $15 on each giraffe (large or small).Would the optimal production schedule change?
E.Suppose demand for small giraffes waned, and Jungle cut its process so that its profit on small giraffes was reduced by 50% to $4.50.Would the optimal solution change?

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
54
Ira Wax solved an integer linear programming problem by setting up a linear programming model without the integer constraints and rounding the solution.List the possible problems with this approach.

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
55
The Hawaii Surfboard Company produces three different styles of surfboards (the Oahu, the Kauai, and the Maui) made from combinations of three different materials, A, B, and C.The Oahu model nets a $35 profit and requires 1 unit of A, 1 unit of B, and 1 unit of C.The Kauai model nets a $75 profit and requires 4 units of A and 1 unit of B.The Maui model nets a $100 profit and requires 1 unit of A, 4 units of B, and 1 unit of C.There are 200 units of A, 200 units of B, and 100 units of C available weekly.
A.Formulate a linear programming model to determine the optimal weekly profit.
B.How would the model change if Hawaii Surfboard wanted to produce an equal number of each model?
A.Formulate a linear programming model to determine the optimal weekly profit.
B.How would the model change if Hawaii Surfboard wanted to produce an equal number of each model?

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
56
How do you designate variables as integers when using Excel Solver?

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
57
What is the major difference between a product mix problem and a diet problem?

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck
58
Spee-D Delivery Service wants to blend two gasolines (R and S) for use in its trucks.For the upcoming period, at least 10,000 gallons are required.Spee-D has a 20,000 gallon storage facility.Any required quantities of R and S gasoline may be purchased from a local refinery and mixed.R is 87 octane and costs $0.90 a gallon.S is 93 octane and costs $1.20 per gallon.Spee-D's fleet of 100 trucks requires an octane of no less than 89.Octane of the mixed output is linearly proportional to input octane.For example, equal parts of R and S would yield a 90 octane output.There is no gasoline loss in the blending process.
Formulate a linear programming model for this problem.Is a summation variable advisable here? Explain.
Formulate a linear programming model for this problem.Is a summation variable advisable here? Explain.

Unlock Deck
Unlock for access to all 58 flashcards in this deck.
Unlock Deck
k this deck



