
College Algebra in Context with Applications for the Managerial, Life, and Social Sciences 3rd Edition by Ronald J Harshbarger, Lisa Yocco
النسخة 3الرقم المعياري الدولي: 032157060X
College Algebra in Context with Applications for the Managerial, Life, and Social Sciences 3rd Edition by Ronald J Harshbarger, Lisa Yocco
النسخة 3الرقم المعياري الدولي: 032157060X تمرين 5
الحلول خطوة بخطوة
الخطوة 1 من3
Consider the following table gives projections of the U.S. population from 2000 to 2100.
| Year | Population (millions) | Year | Population (millions) |
| 2000 | 275.3 | 2060 | 432.0 |
| 2010 | 299.9 | 2070 | 463.6 |
| 2020 | 324.9 | 2080 | 497.8 |
| 2030 | 351.1 | 2090 | 533.6 |
| 2040 | 377.4 | 2100 | 571.0 |
| 2050 | 403.7 |
(a) Let us find a linear function that models the data, with x equals to the number of years after 2000 and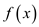 equal to the population in millions.
equal to the population in millions.
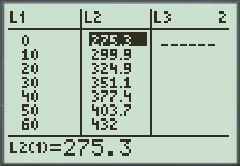
Enter the data from the above table in the lists of a graphing utility.
The figure below shows a partial list of the data points.
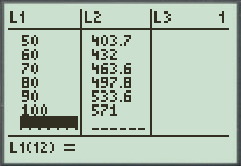
The equation that fits best for the data, found using linear regression with a graphing calculator.
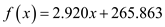 . Thus, the linear function that models the data is
. Thus, the linear function that models the data is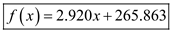 .
. الخطوة 2 من 3
الخطوة 3 من 3
College Algebra in Context with Applications for the Managerial, Life, and Social Sciences 3rd Edition by Ronald J Harshbarger, Lisa Yocco
لماذا لم يعجبك هذا التمرين؟
أخرى 8 أحرف كحد أدنى و 255 حرفاً كحد أقصى
حرف 255








