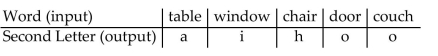
Determine Whether the Function Is One-To-One $\text { one-to-one; }$ B) Not One-To-One
C) One-To-One;
D) One-To-One;

Determine whether the function is one-to-one. If it is one-to-one find an equation or a set of ordered pairs that defines
the inverse function of the given function.
-

A) 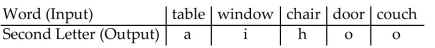$\text { one-to-one; }$


B) not one-to-one
C) one-to-one;
D) one-to-one;