Essay
In the simple linear regression model Y = the quantity E is a random variable, assumed to be normally distributed with E( ) = 0, and V( ) = . The estimated standard error of (the least squares estimated of ), denoted by 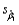 , is __________ divided by __________, where .
, is __________ divided by __________, where .
Correct Answer:
Verified
Related Questions
Q1: The estimated regression line or least squares
Q3: In the simple linear regression model
Q4: In simple linear regression analysis, a quantitative
Q5: The assumptions of the simple of the
Q6: If y = -2x - 8, then
Unlock this Answer For Free Now!
View this answer and more for free by performing one of the following actions

Scan the QR code to install the App and get 2 free unlocks

Unlock quizzes for free by uploading documents