A company wants to build a new factory in either Atlanta or Columbia. It is also considering building a warehouse in whichever city is selected for the new factory. The following table shows the net present value (NPV) and cost of each facility. The company wants to maximize the net present value of its facilities, but it only has $15 million to invest.
Based on this ILP formulation of the problem and the indicated optimal solution what values should go in cells B6:G14 of the following Excel spreadsheet?
Subject to:
Solution:
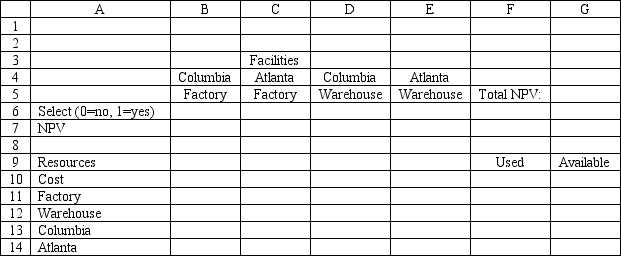
Correct Answer:
Verified
Q8: A company will be able to obtain
Q41: A company wants to build a
Q41:
The following questions pertain to the
Q43: An investor has $500,000 to invest
Q44: A small town wants to build
Q47: A city wants to locate 2
Q48: A company wants to build a
Q50: A city wants to locate 2
Q55: A sub-problem in a B & B
Q89: Exhibit 6.1
The following questions pertain to the
Unlock this Answer For Free Now!
View this answer and more for free by performing one of the following actions

Scan the QR code to install the App and get 2 free unlocks

Unlock quizzes for free by uploading documents