Deck 60: Introduction to Conics Parabolas
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال

فتح الحزمة
قم بالتسجيل لفتح البطاقات في هذه المجموعة!
Unlock Deck
Unlock Deck
1/124
العب
ملء الشاشة (f)
Deck 60: Introduction to Conics Parabolas
1
Find the vertex and focus of the parabola from the given equation and select its graph.
A)Vertex: (0,0) Focus: (0,-1.5)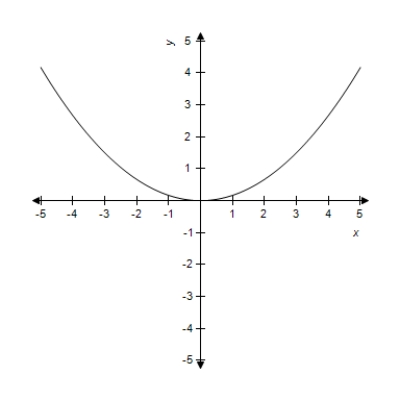
B)Vertex: (0,0) Focus: (-1.5,0)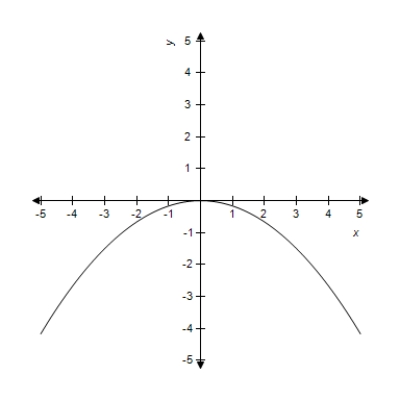
C)Vertex: (0,0) Focus: (0,1.5)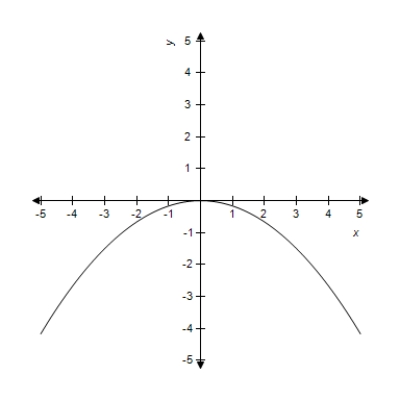
D)Vertex: (0,0) Focus: (0,1.5)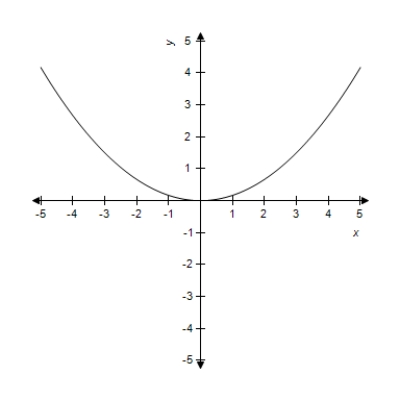
E)Vertex: (0,0) Focus: (0,-1.5)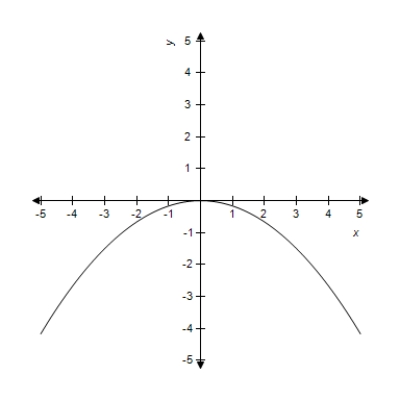
A)Vertex: (0,0) Focus: (0,-1.5)

B)Vertex: (0,0) Focus: (-1.5,0)

C)Vertex: (0,0) Focus: (0,1.5)

D)Vertex: (0,0) Focus: (0,1.5)

E)Vertex: (0,0) Focus: (0,-1.5)

Vertex: (0,0) Focus: (0,1.5) 

2
Find the vertex and focus of the parabola from the given equation and select its graph.
A)Vertex: (0,0) Focus: (4,0)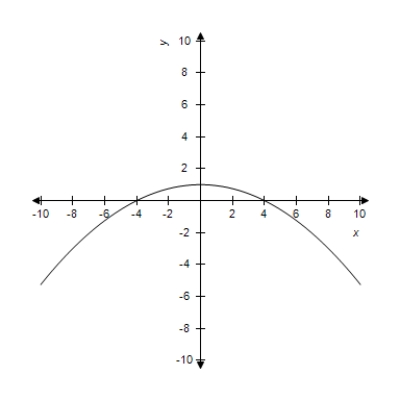
B)Vertex: (0,0) Focus: (-4,0)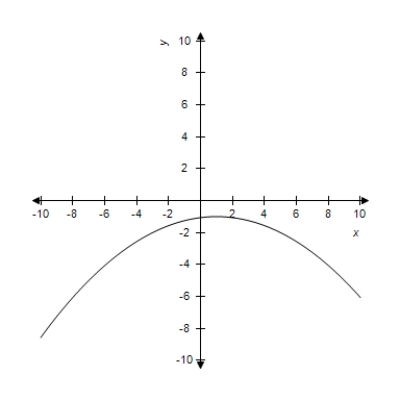
C)Vertex: (0,-4) Focus: (0,0)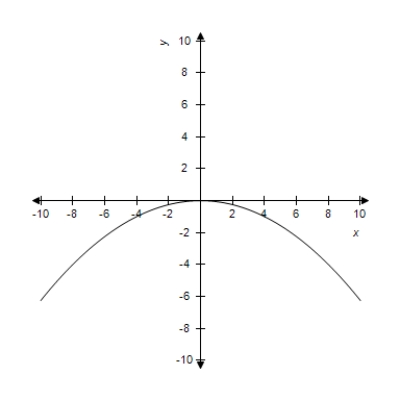
D)Vertex: (0,0) Focus: (0,4)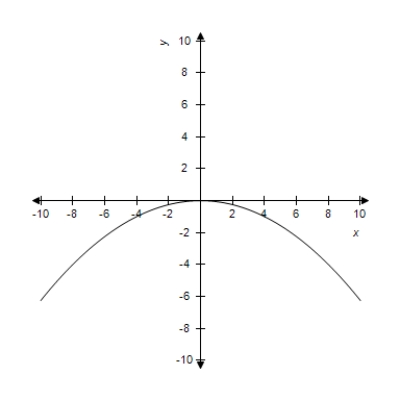
E)Vertex: (0,0) Focus: (0,-4)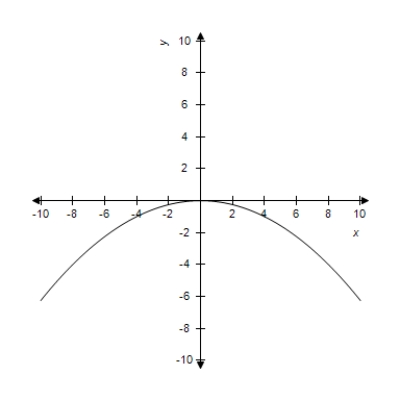
A)Vertex: (0,0) Focus: (4,0)

B)Vertex: (0,0) Focus: (-4,0)

C)Vertex: (0,-4) Focus: (0,0)

D)Vertex: (0,0) Focus: (0,4)

E)Vertex: (0,0) Focus: (0,-4)

Vertex: (0,0) Focus: (0,-4) 

3
Find the center and vertices which located on the major axis of the ellipse from given equation and select its graph.
A)Center: (0,0) Vertices: (-6,0)
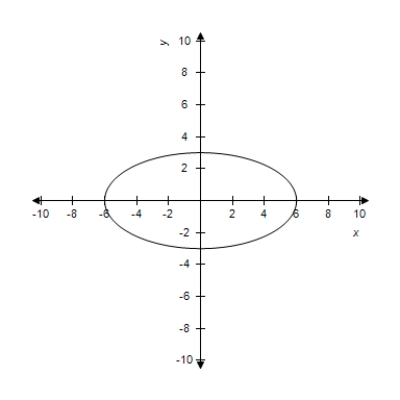
B)Center: (0,0) Vertices: (±6,0)
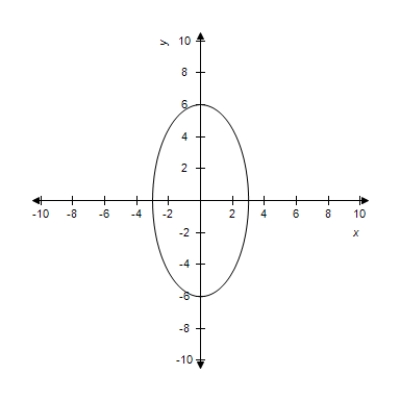
C)Center: (0,0) Vertices: (-6,0)
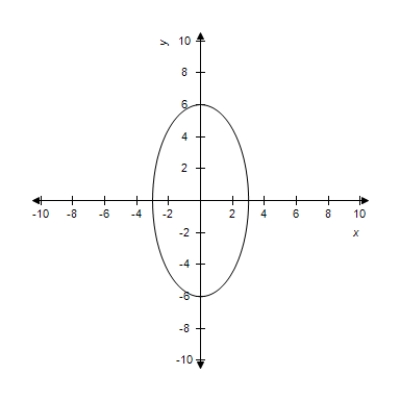
D)Center: (0,0) Vertices: (±6,0)
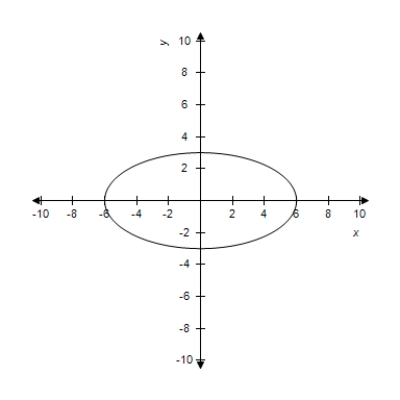
E)Center: (0,0) Vertices: (6,0)
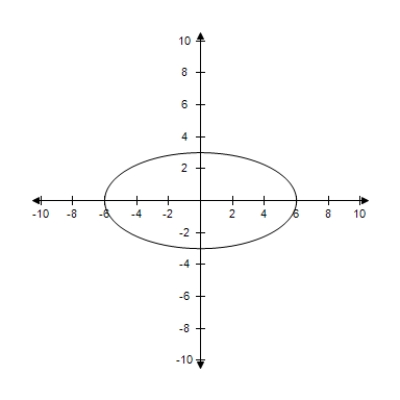
A)Center: (0,0) Vertices: (-6,0)

B)Center: (0,0) Vertices: (±6,0)

C)Center: (0,0) Vertices: (-6,0)

D)Center: (0,0) Vertices: (±6,0)

E)Center: (0,0) Vertices: (6,0)

Center: (0,0) Vertices: (±6,0)


4
Find the standard form of the equation of the parabola with the given characteristic(s)and vertex at the origin.
Directrix: y = 2
A)
B)
C)
D)
E)
Directrix: y = 2
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 124 في هذه المجموعة.
فتح الحزمة
k this deck
5
Find the standard form of the equation of the parabola with the given characteristic(s)and vertex at the origin.
Directrix: x = 3
A)
B)
C)
D)
E)
Directrix: x = 3
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 124 في هذه المجموعة.
فتح الحزمة
k this deck
6
Find the vertex and focus of the parabola from the given equation and select its graph.
A)Vertex: (0,0) Focus: (- ,0)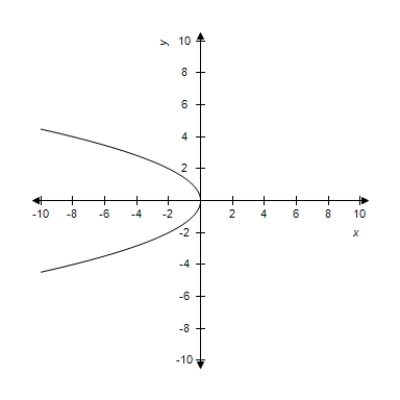
B)Vertex: (- ,0) Focus: (0,0)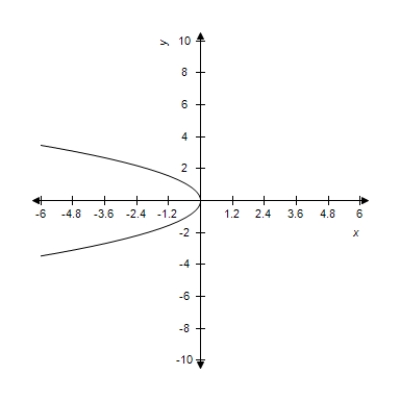
C)Vertex: (0,0) Focus: (0, )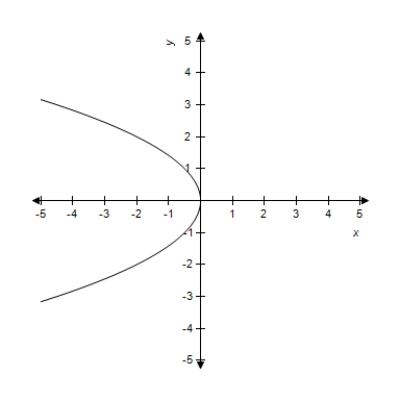
D)Vertex: (0,0) Focus: (0,- )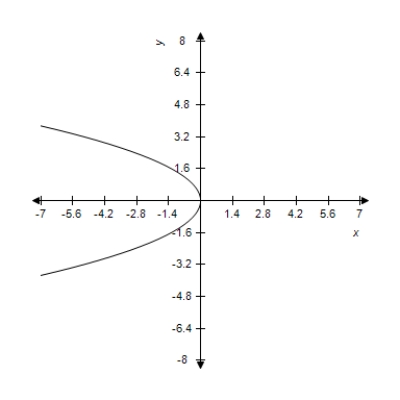
E)Vertex: (0,0) Focus: ( ,0)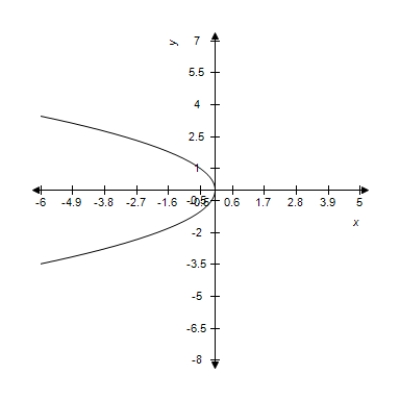
A)Vertex: (0,0) Focus: (- ,0)

B)Vertex: (- ,0) Focus: (0,0)

C)Vertex: (0,0) Focus: (0, )

D)Vertex: (0,0) Focus: (0,- )

E)Vertex: (0,0) Focus: ( ,0)


فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 124 في هذه المجموعة.
فتح الحزمة
k this deck
7
Find the standard form of the equation of the parabola with the given characteristic(s)and vertex at the origin.
Passes through the point (2,4);horizontal axis
A)
B)
C)
D)
E)
Passes through the point (2,4);horizontal axis
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 124 في هذه المجموعة.
فتح الحزمة
k this deck
8
Find the vertex and focus of the parabola for the given equation and select its graph.
A)Vertex: (0,0) Focus: (0,3)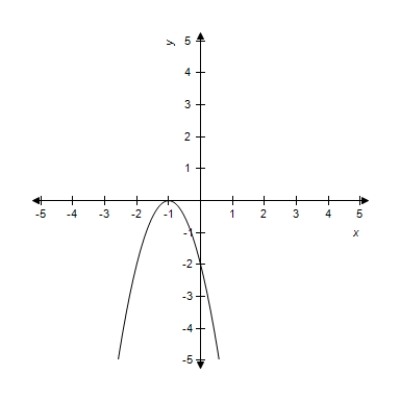
B)Vertex: (0,0) Focus: (0,-12)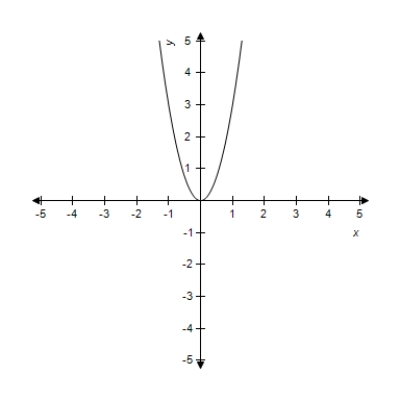
C)Vertex: (0,0) Focus: (0,-3)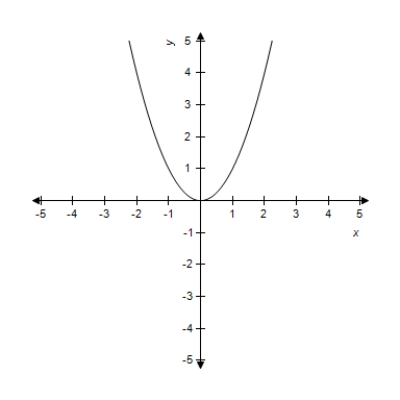
D)Vertex: (0,0) Focus: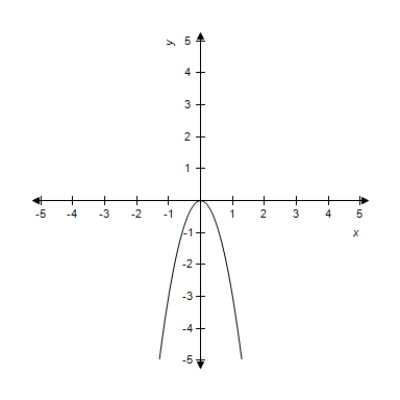
E)Vertex: (0,0) Focus: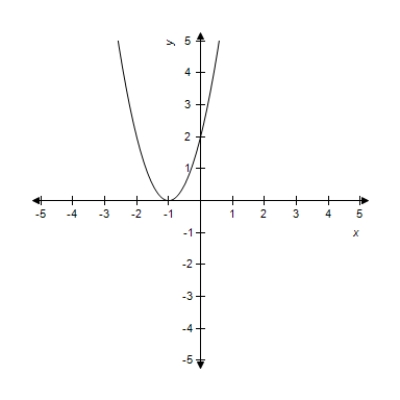
A)Vertex: (0,0) Focus: (0,3)

B)Vertex: (0,0) Focus: (0,-12)

C)Vertex: (0,0) Focus: (0,-3)

D)Vertex: (0,0) Focus:

E)Vertex: (0,0) Focus:


فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 124 في هذه المجموعة.
فتح الحزمة
k this deck
9
Find the standard form of the equation of the parabola with the given characteristic(s)and vertex at the origin.
Focus: (0, )
A)
B)
C)
D)
E)
Focus: (0, )
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 124 في هذه المجموعة.
فتح الحزمة
k this deck
10
Find the center and vertices which located on the major axis of the ellipse from given equation and select its graph.
A)Center: (0,0) Vertices: (±3,0)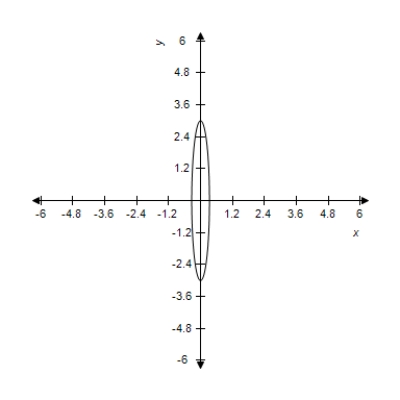
B)Center: (0,0) Vertices: (-3,0)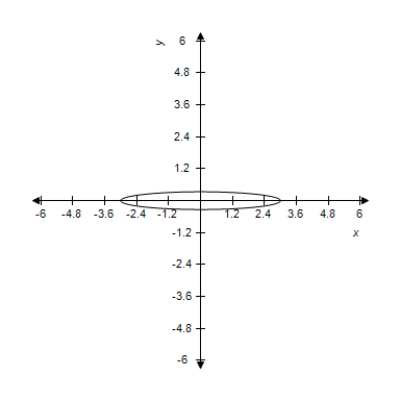
C)Center: (0,0) Vertices: (3,0)
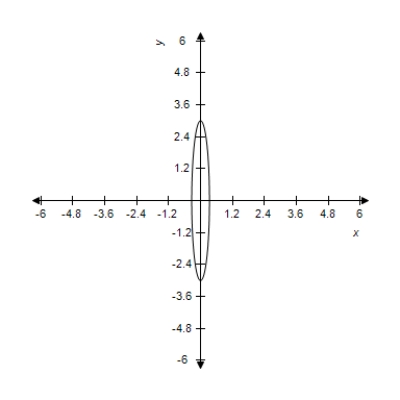
D)Center: (0,0) Vertices: (±3,0)
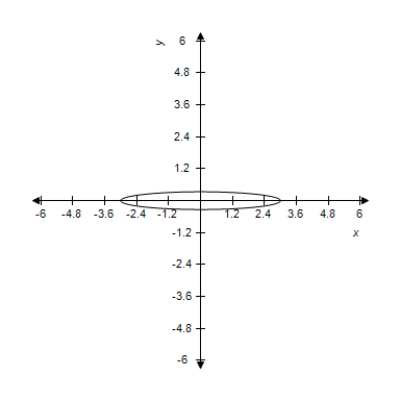
E)Center: (0,0) Vertices: (-3,0)
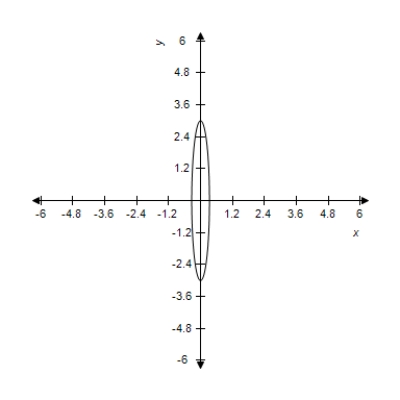
A)Center: (0,0) Vertices: (±3,0)

B)Center: (0,0) Vertices: (-3,0)

C)Center: (0,0) Vertices: (3,0)

D)Center: (0,0) Vertices: (±3,0)

E)Center: (0,0) Vertices: (-3,0)


فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 124 في هذه المجموعة.
فتح الحزمة
k this deck
11
Select the standard form of the equation of the parabola and determine the coordinates of the focus. 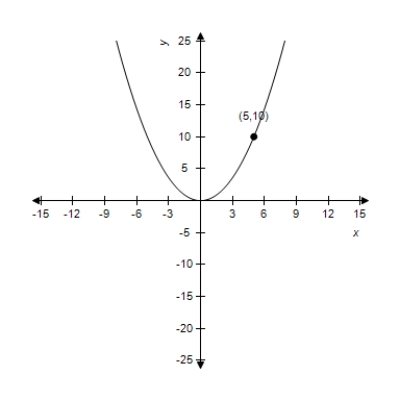
A) ;Focus:
B) ;Focus:
C) ;Focus:
D) ;Focus:
E) ;Focus:

A) ;Focus:
B) ;Focus:
C) ;Focus:
D) ;Focus:
E) ;Focus:

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 124 في هذه المجموعة.
فتح الحزمة
k this deck
12
Find the standard form of the equation of the parabola with the given characteristic(s)and vertex at the origin.
Focus:
A)
B)
C)
D)
E)
Focus:
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 124 في هذه المجموعة.
فتح الحزمة
k this deck
13
Find the vertex and focus of the parabola from the given equation and select its graph.
A)Vertex: (0,0) Focus: (- ,0)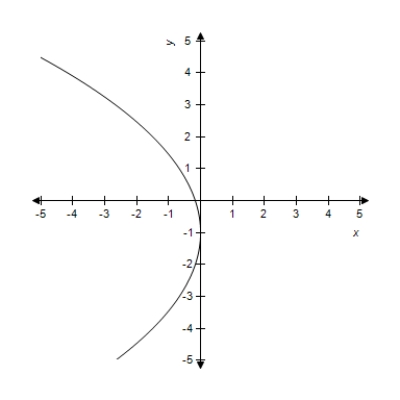
B)Vertex: (0,0) Focus: (0,- )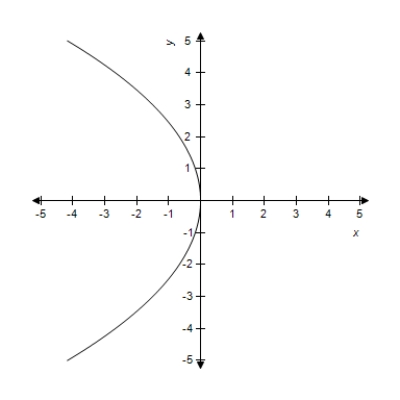
C)Vertex: (0,0) Focus: ( ,0)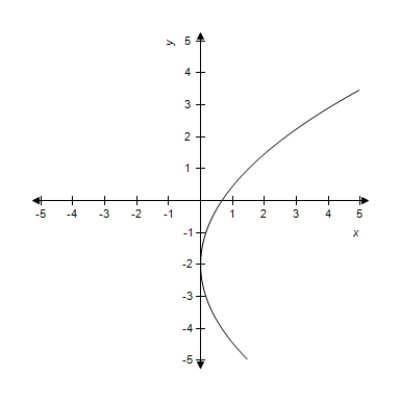
D)Vertex: (0,0) Focus: (0, )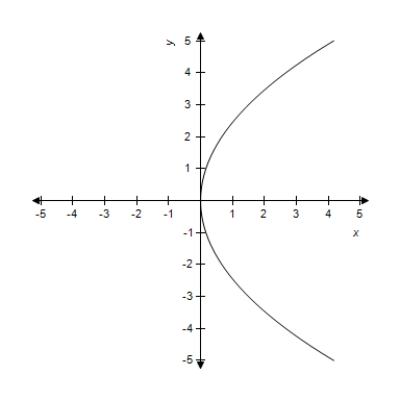
E)Vertex: (0,0) Focus: ( ,0)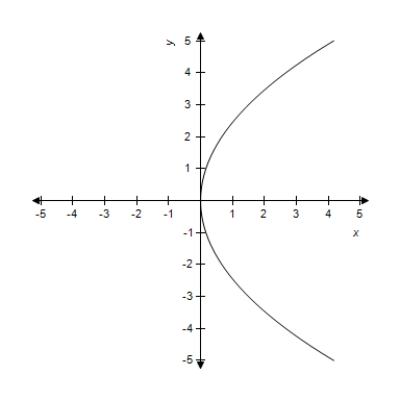
A)Vertex: (0,0) Focus: (- ,0)

B)Vertex: (0,0) Focus: (0,- )

C)Vertex: (0,0) Focus: ( ,0)

D)Vertex: (0,0) Focus: (0, )

E)Vertex: (0,0) Focus: ( ,0)


فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 124 في هذه المجموعة.
فتح الحزمة
k this deck
14
Find the standard form of the equation of the parabola with the given characteristic(s)and vertex at the origin.
Passes through the point ;vertical axis
A)
B)
C)
D)
E)
Passes through the point ;vertical axis
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 124 في هذه المجموعة.
فتح الحزمة
k this deck
15
Find the standard form of the equation of the parabola with the given characteristic(s)and vertex at the origin.
Directrix: x = -4
A)
B)
C)
D)
E)
Directrix: x = -4
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 124 في هذه المجموعة.
فتح الحزمة
k this deck
16
Find the standard form of the equation of the parabola with the given characteristic(s)and vertex at the origin.
Focus: (0,-2)
A)
B)
C)
D)
E)
Focus: (0,-2)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 124 في هذه المجموعة.
فتح الحزمة
k this deck
17
Find the vertex and focus of the parabola for the given equation and select its graph.
A)Vertex: (0,0) Focus: (1,0)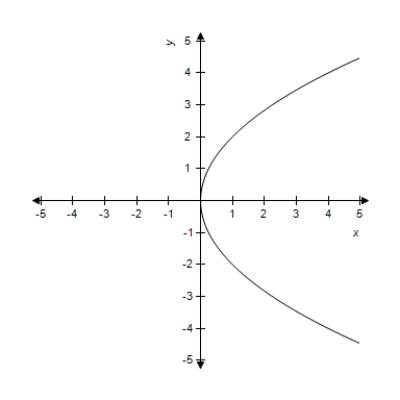
B)Vertex: (0,0) Focus: (1,0)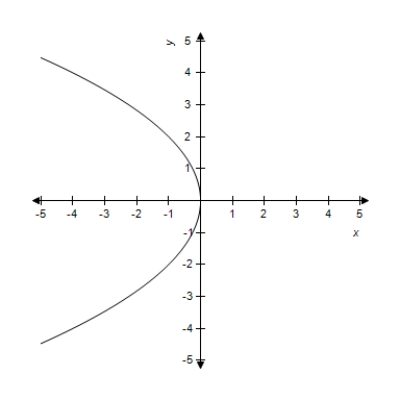
C)Vertex: (0,0) Focus: (-1,0)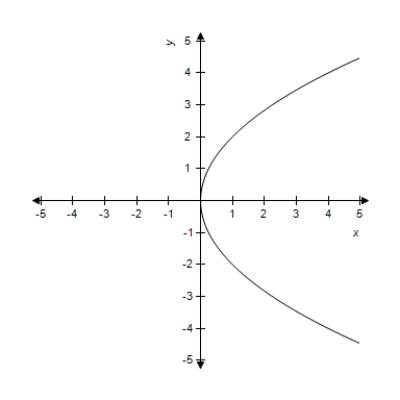
D)Vertex: (0,0) Focus: ( - 1,0)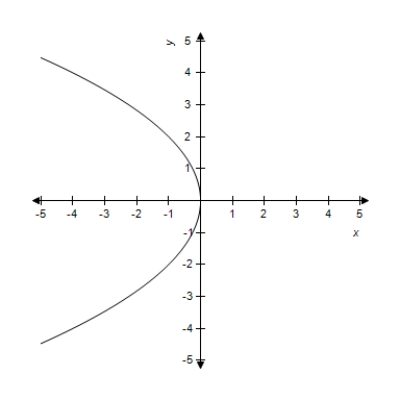
E)Vertex: (0,0) Focus: (0,1)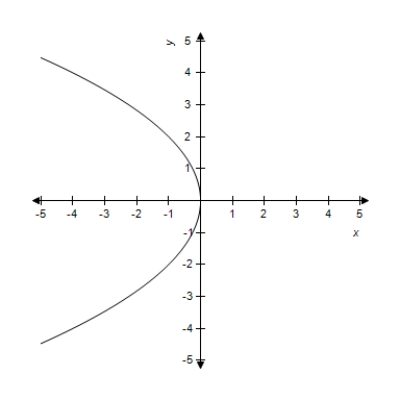
A)Vertex: (0,0) Focus: (1,0)

B)Vertex: (0,0) Focus: (1,0)

C)Vertex: (0,0) Focus: (-1,0)

D)Vertex: (0,0) Focus: ( - 1,0)

E)Vertex: (0,0) Focus: (0,1)


فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 124 في هذه المجموعة.
فتح الحزمة
k this deck
18
Find the center and vertices which located on the major axis of the ellipse from given equation and select its graph.
A)Center: (0,0) Vertices: (±10,0)
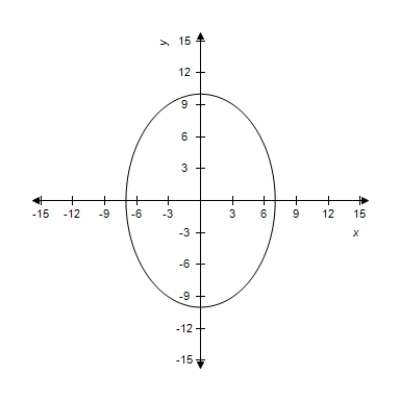
B)Center: (0,0) Vertices: (10,0)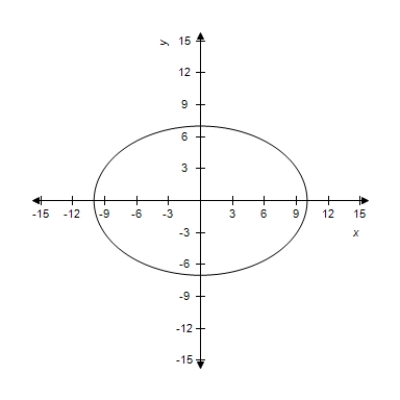
C)Center: (0,0) Vertices: (-10,0)
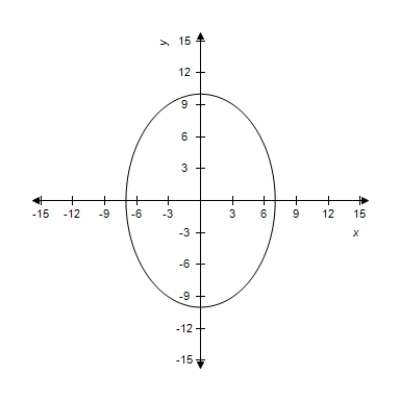
D)Center: (0,0) Vertices: (-10,0)
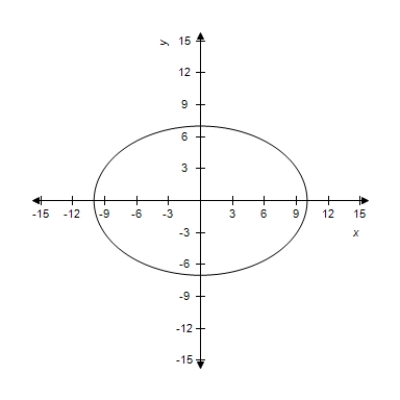
E)Center: (0,0) Vertices: (±10,0)
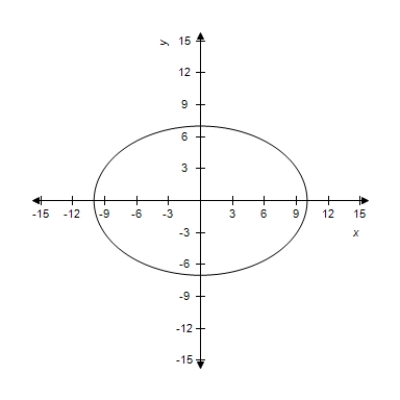
A)Center: (0,0) Vertices: (±10,0)

B)Center: (0,0) Vertices: (10,0)

C)Center: (0,0) Vertices: (-10,0)

D)Center: (0,0) Vertices: (-10,0)

E)Center: (0,0) Vertices: (±10,0)


فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 124 في هذه المجموعة.
فتح الحزمة
k this deck
19
Find the standard form of the equation of the parabola with the given characteristic(s)and vertex at the origin.
Focus: (-4,0)
A)
B)
C)
D)
E)
Focus: (-4,0)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 124 في هذه المجموعة.
فتح الحزمة
k this deck
20
Find the standard form of the equation of the parabola with the given characteristic(s)and vertex at the origin.
Directrix: y = -1
A)
B)
C)
D)
E)
Directrix: y = -1
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 124 في هذه المجموعة.
فتح الحزمة
k this deck
21
Find the standard form of the equation of the parabola with the given characteristic and vertex at the origin. focus: (0,-1)
A)y2 = -4x
B)x2 = -4y
C)x2 = y
D)y2 = -x
E)x2 = -y
A)y2 = -4x
B)x2 = -4y
C)x2 = y
D)y2 = -x
E)x2 = -y

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 124 في هذه المجموعة.
فتح الحزمة
k this deck
22
Identify the conic.
A)Ellipse
B)Circle
C)Parabola
D)Line
E)Hyperbola
A)Ellipse
B)Circle
C)Parabola
D)Line
E)Hyperbola

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 124 في هذه المجموعة.
فتح الحزمة
k this deck
23
Find the standard form of the equation of the parabola with the given characteristic and vertex at the origin. directrix: x = -5
A)y2 = 20x
B)y2 = -5x
C)x2 = -20y
D)x2 = -5y
E)x2 = 20y
A)y2 = 20x
B)y2 = -5x
C)x2 = -20y
D)x2 = -5y
E)x2 = 20y

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 124 في هذه المجموعة.
فتح الحزمة
k this deck
24
Find the standard form of the equation of the hyperbola with the given characteristics and center at the origin.
Vertices: (±3,0);passes through the point
A)
B)
C)
D)
E)
Vertices: (±3,0);passes through the point
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 124 في هذه المجموعة.
فتح الحزمة
k this deck
25
Find the center and vertices of the hyperbola and select its graph,using asymptotes as sketching aids.
A)Centre: (0,0) Vertices: (0,-5)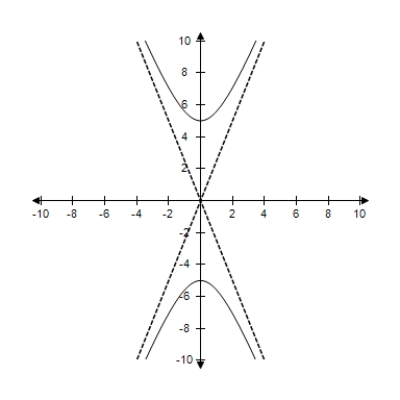
B)Centre: (0,0) Vertices: (±5,0)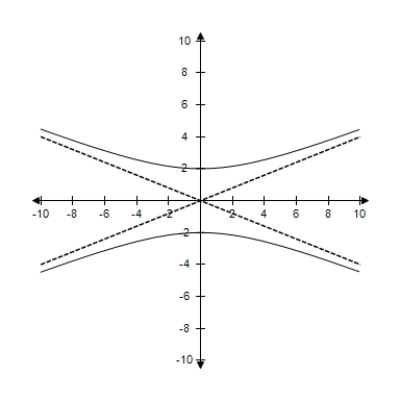
C)Centre: (0,0) Vertices: (0,±5)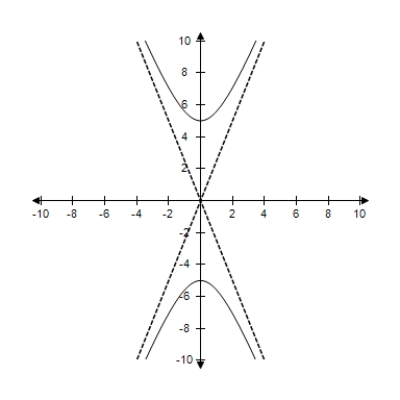
D)Centre: (0,0) Vertices: (0,5)
E)Centre: (0,0) Vertices: (5,0)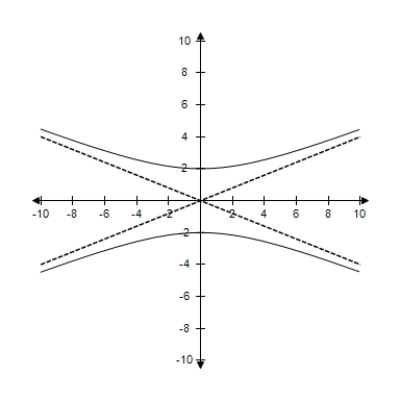
A)Centre: (0,0) Vertices: (0,-5)

B)Centre: (0,0) Vertices: (±5,0)

C)Centre: (0,0) Vertices: (0,±5)

D)Centre: (0,0) Vertices: (0,5)

E)Centre: (0,0) Vertices: (5,0)


فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 124 في هذه المجموعة.
فتح الحزمة
k this deck
26
Find the center and vertices of the hyperbola and sketch its graph,using asymptotes as sketching aids.
A)Center: (0,0) Vertices: (-5,0)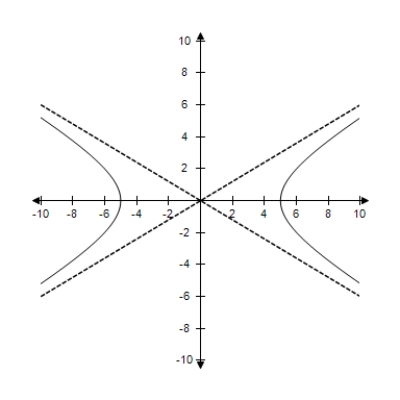
B)Center: (0,0) Vertices: (-3,0)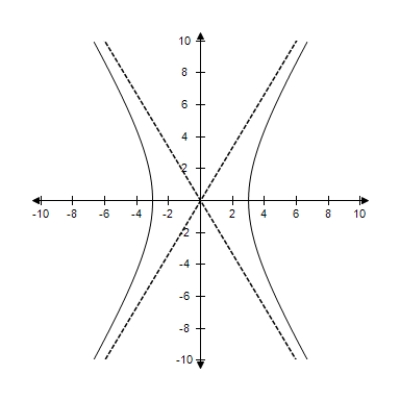
C)Center: (0,0) Vertices: (3,0)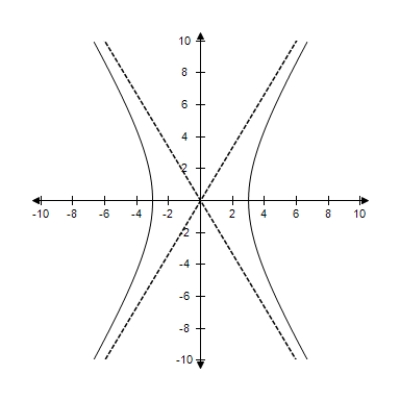
D)Center: (0,0) Vertices: (±5,0)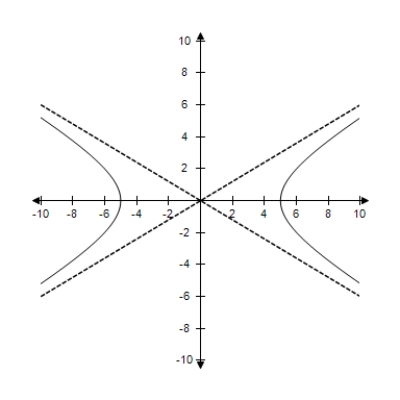
E)Center: (0,0) Vertices: (±3,0)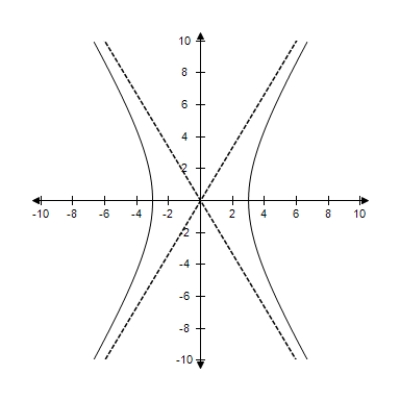
A)Center: (0,0) Vertices: (-5,0)

B)Center: (0,0) Vertices: (-3,0)

C)Center: (0,0) Vertices: (3,0)

D)Center: (0,0) Vertices: (±5,0)

E)Center: (0,0) Vertices: (±3,0)


فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 124 في هذه المجموعة.
فتح الحزمة
k this deck
27
Select the standard form of the equation of the parabola and determine the coordinates of the focus. 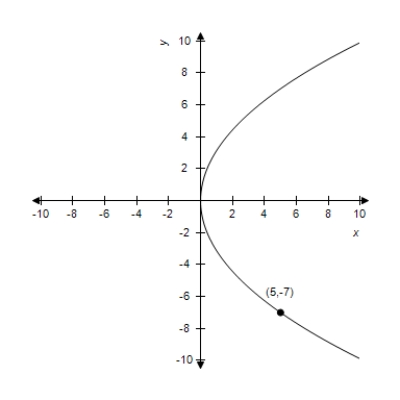
A) ;Focus:
B) ;Focus:
C) ;Focus:
D) Focus:
E) ;Focus:

A) ;Focus:
B) ;Focus:
C) ;Focus:
D) Focus:
E) ;Focus:

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 124 في هذه المجموعة.
فتح الحزمة
k this deck
28
Find the vertex and focus of the parabola.
A)vertex: (0,0)focus: (0,-2)
B)vertex: (2,0)focus: (0,0)
C)vertex: (0,0)focus: (-2,0)
D)vertex: (0,0)focus: (0,2)
E)vertex: (-2,0)focus: (0,0)
A)vertex: (0,0)focus: (0,-2)
B)vertex: (2,0)focus: (0,0)
C)vertex: (0,0)focus: (-2,0)
D)vertex: (0,0)focus: (0,2)
E)vertex: (-2,0)focus: (0,0)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 124 في هذه المجموعة.
فتح الحزمة
k this deck
29
Find the standard form of the equation of the hyperbola with the given characteristics and center at the origin.
Vertices: (0,±3);focies: (0,±7)
A)
B)
C)
D)
E)
Vertices: (0,±3);focies: (0,±7)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 124 في هذه المجموعة.
فتح الحزمة
k this deck
30
A solar oven uses a parabolic reflector to focus the sun's rays at a point 5 inches from the vertex of the reflector (see figure).Write an equation for a cross section of the oven's reflector with its focus on the positive y axis and its vertex at the origin. 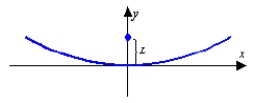 L = 5 inches
L = 5 inches
A)
B)
C)
D)
E)
 L = 5 inches
L = 5 inchesA)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 124 في هذه المجموعة.
فتح الحزمة
k this deck
31
Find the vertex and focus of the parabola.
A)vertex: (0,0)focus:
B)vertex: focus:
C)vertex: (0,0)focus:
D)vertex: focus:
E)vertex: (0,0)focus:
A)vertex: (0,0)focus:
B)vertex: focus:
C)vertex: (0,0)focus:
D)vertex: focus:
E)vertex: (0,0)focus:

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 124 في هذه المجموعة.
فتح الحزمة
k this deck
32
Find the standard form of the equation of the ellipse with the given characteristics and center at the origin.
Focies: (±7,0);major axis of length 16
A)
B)
C)
D)
E)
Focies: (±7,0);major axis of length 16
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 124 في هذه المجموعة.
فتح الحزمة
k this deck
33
A semielliptical arch over a tunnel for a one-way road through a mountain has a major axis of 20 feet and a height at the center of 4 feet.Select the arch of the tunnel on a rectangular coordinate system with the center of the road entering the tunnel at the origin.Identify the coordinates of the known points.
A)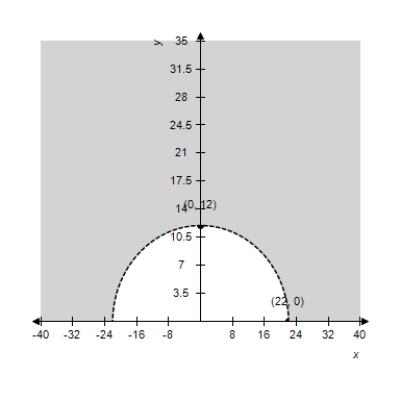
B)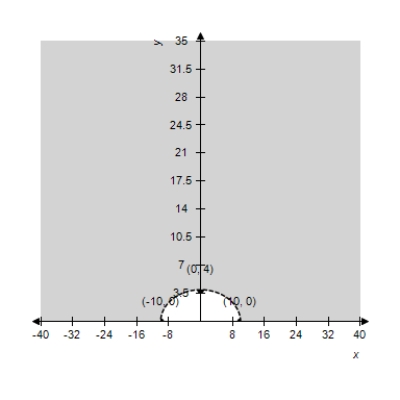
C)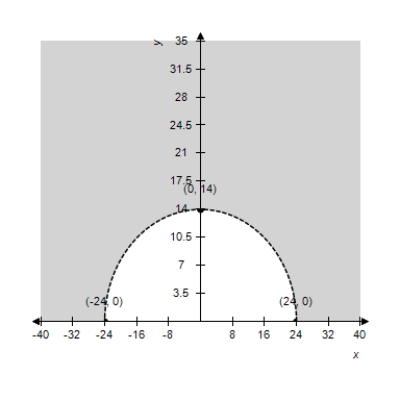
D)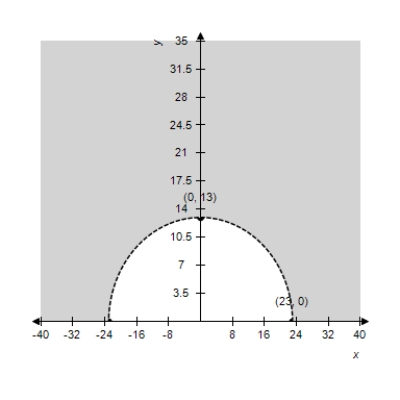
E)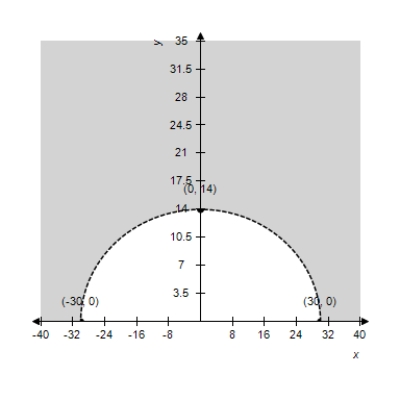
A)

B)

C)

D)

E)


فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 124 في هذه المجموعة.
فتح الحزمة
k this deck
34
Identify the conic.
A)Circle
B)Ellipse
C)Parabola
D)Hyperbola
E)Line
A)Circle
B)Ellipse
C)Parabola
D)Hyperbola
E)Line

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 124 في هذه المجموعة.
فتح الحزمة
k this deck
35
Find the standard form of the equation of the parabola and determine the coordinates of the focus. 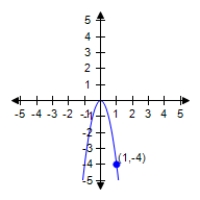
A) ,focus:
B) ,focus:
C) ,focus:
D) ,focus: (0,-4)
E) ,focus:

A) ,focus:
B) ,focus:
C) ,focus:
D) ,focus: (0,-4)
E) ,focus:

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 124 في هذه المجموعة.
فتح الحزمة
k this deck
36
Identify the conic.
A)Circle
B)Ellipse
C)Hyperbola
D)Parabola
E)Line
A)Circle
B)Ellipse
C)Hyperbola
D)Parabola
E)Line

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 124 في هذه المجموعة.
فتح الحزمة
k this deck
37
Find the standard form of the equation of the hyperbola with the given characteristics and center at the origin.
Vertices: (0,±5);focies: (0,±6)
A)
B)
C)
D)
E)
Vertices: (0,±5);focies: (0,±6)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 124 في هذه المجموعة.
فتح الحزمة
k this deck
38
Match the equation with its graph.
A)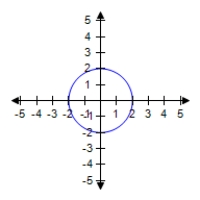
B)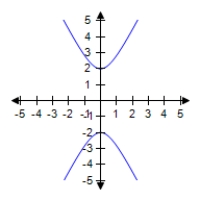
C)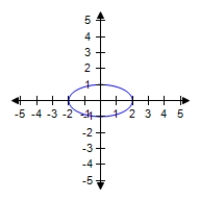
D)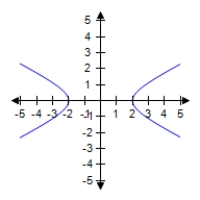
E)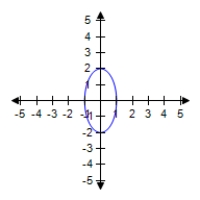
A)

B)

C)

D)

E)


فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 124 في هذه المجموعة.
فتح الحزمة
k this deck
39
Find the standard form of the equation of the ellipse with the given characteristics and center at the origin.
Vertices: (0,±7);focies: (0,±4)
A)
B)
C)
D)
E)
Vertices: (0,±7);focies: (0,±4)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 124 في هذه المجموعة.
فتح الحزمة
k this deck
40
Find the standard form of the equation of the hyperbola with the given characteristics and center at the origin.
Vertices: (0,±2);asymptotes: y = ± x
A)
B)
C)
D)
E)
Vertices: (0,±2);asymptotes: y = ± x
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 124 في هذه المجموعة.
فتح الحزمة
k this deck
41
Find the standard form of the equation of the hyperbola with the given characteristics. focies: (±4,0),asymptotes:
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 124 في هذه المجموعة.
فتح الحزمة
k this deck
42
Find the standard form of the equation of the hyperbola with the given characteristics. vertices: (0,±4)focies: (0,±5)
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 124 في هذه المجموعة.
فتح الحزمة
k this deck
43
Find the standard form of the equation of the ellipse with the following graph. 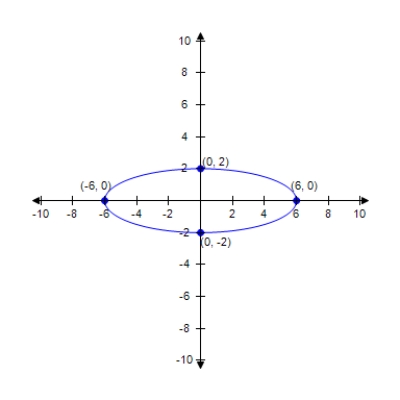
A) , ,
B) , ,
C) , ,
D) , ,
E) , ,

A) , ,
B) , ,
C) , ,
D) , ,
E) , ,

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 124 في هذه المجموعة.
فتح الحزمة
k this deck
44
Find the standard form of the equation of the parabola with the given characteristic and vertex at the origin. Focus:
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 124 في هذه المجموعة.
فتح الحزمة
k this deck
45
Find the standard form of the equation of the parabola with the given characteristic and vertex at the origin.
Vertical axis and passes through the point (6,8)
A)
B)
C)
D)
E)
Vertical axis and passes through the point (6,8)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 124 في هذه المجموعة.
فتح الحزمة
k this deck
46
Write an equation for a cross section of the parabolic ear (used to hear sounds from a distance)shown in the picture. 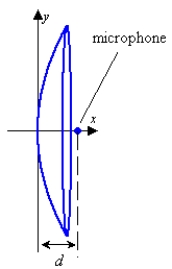 d = 2.25 inches
d = 2.25 inches
A)
B)
C)
D)
E)
 d = 2.25 inches
d = 2.25 inchesA)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 124 في هذه المجموعة.
فتح الحزمة
k this deck
47
Find the center and the vertices which located on the major axis of the ellipse.
A)center: (0,0)vertices: (-9,0), (9,0)
B)center: (0,0)vertices: (0,-9), (0,9)
C)center: (-9,9)vertices: (-1,0), (1,0)
D)center: (0,0)vertices: (-9,-1), (9,1)
E)center: (-9,9)vertices: (-1,-9), (1,9)
A)center: (0,0)vertices: (-9,0), (9,0)
B)center: (0,0)vertices: (0,-9), (0,9)
C)center: (-9,9)vertices: (-1,0), (1,0)
D)center: (0,0)vertices: (-9,-1), (9,1)
E)center: (-9,9)vertices: (-1,-9), (1,9)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 124 في هذه المجموعة.
فتح الحزمة
k this deck
48
Select the graph of the following equation.
A)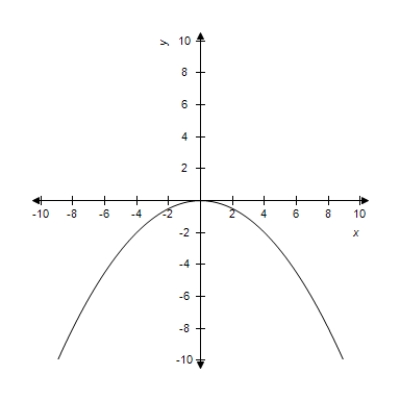
B)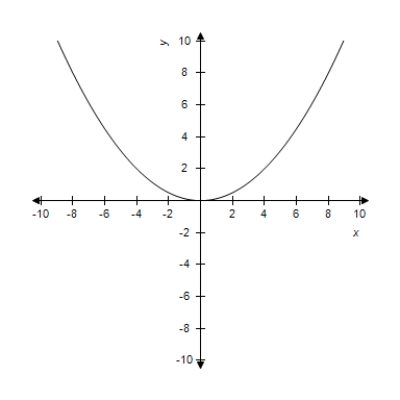
C)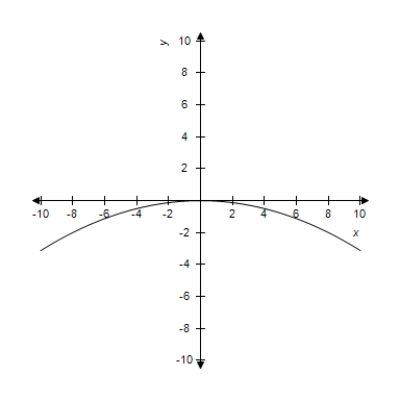
D)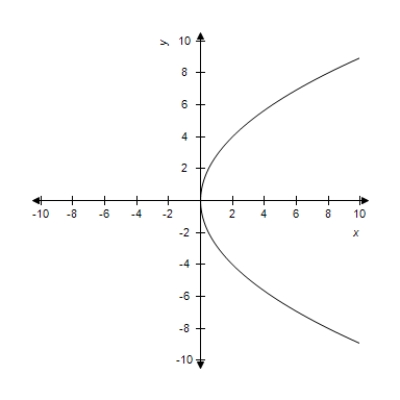
E)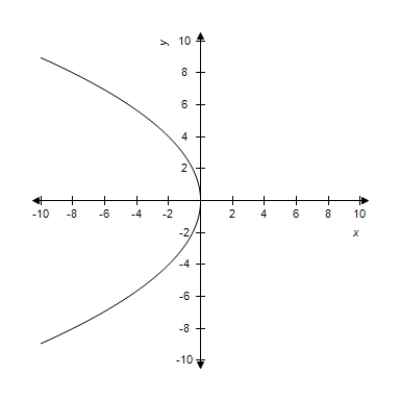
A)

B)

C)

D)

E)


فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 124 في هذه المجموعة.
فتح الحزمة
k this deck
49
Find the standard form of the equation of the parabola with the given characteristic and vertex at the origin. Focus:
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 124 في هذه المجموعة.
فتح الحزمة
k this deck
50
Find the standard form of the equation of the parabola with the given characteristic and vertex at the origin. Focus:
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 124 في هذه المجموعة.
فتح الحزمة
k this deck
51
Find the standard form of the equation of the ellipse with the following characteristics. focies: (±8,0)major axis of length: 22
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 124 في هذه المجموعة.
فتح الحزمة
k this deck
52
Find the standard form of the equation of the parabola with the given characteristic and vertex at the origin.
Directrix:
A)
B)
C)
D)
E)
Directrix:
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 124 في هذه المجموعة.
فتح الحزمة
k this deck
53
Sketch the graph of the ellipse,using the lateral recta.
A)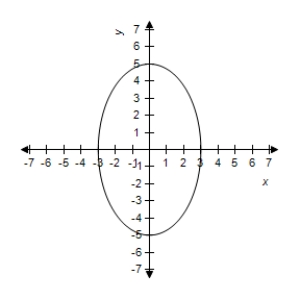
B)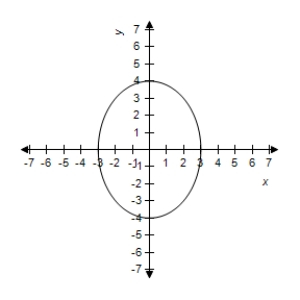
C)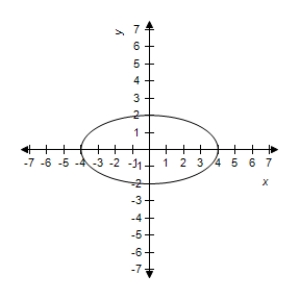
D)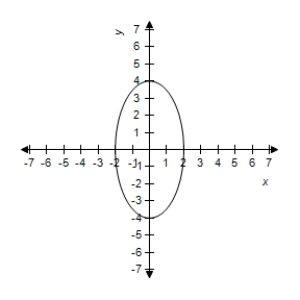
E)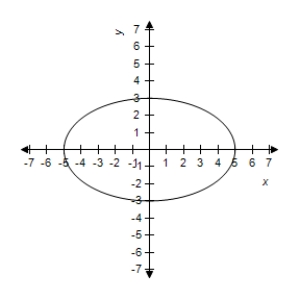
A)

B)

C)

D)

E)


فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 124 في هذه المجموعة.
فتح الحزمة
k this deck
54
Select the graph of the following equation:
A)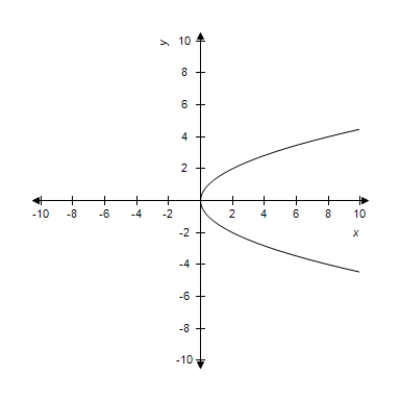
B)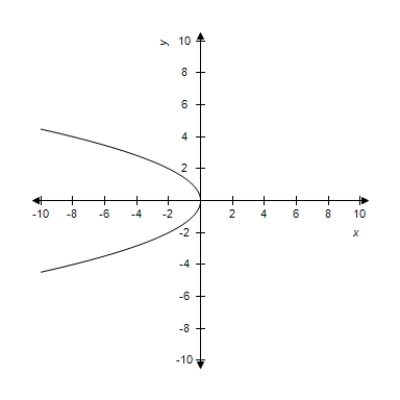
C)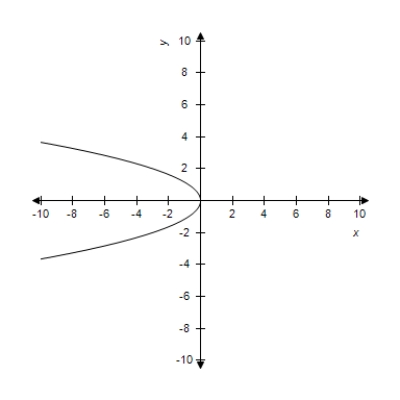
D)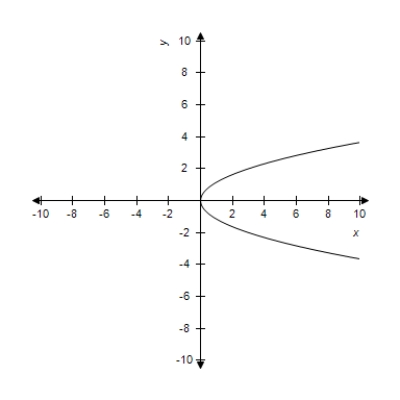
E)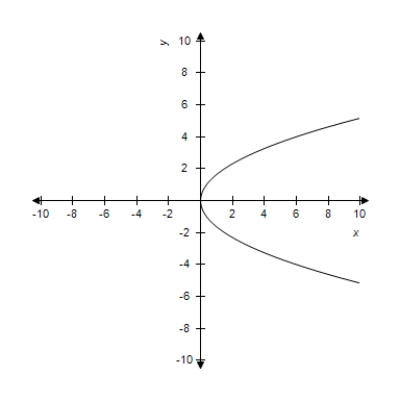
A)

B)

C)

D)

E)


فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 124 في هذه المجموعة.
فتح الحزمة
k this deck
55
Find the standard form of the equation of the parabola with the given characteristic and vertex at the origin.
Directrix:
A)
B)
C)
D)
E)
Directrix:
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 124 في هذه المجموعة.
فتح الحزمة
k this deck
56
Find the standard form of the equation of the parabola with the given characteristic and vertex at the origin.
Vertical axis and passes through the point (-8,-8)
A)
B)
C)
D)
E)
Vertical axis and passes through the point (-8,-8)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 124 في هذه المجموعة.
فتح الحزمة
k this deck
57
Find the vertices and asymptotes of the hyperbola.
A)vertices: (±2,0),asymptote:
B)vertices: (0,±2),asymptote:
C)vertices: (±2,0),asymptote:
D)vertices: (0,±2),asymptote:
E)vertices: (±2,5),asymptote:
A)vertices: (±2,0),asymptote:
B)vertices: (0,±2),asymptote:
C)vertices: (±2,0),asymptote:
D)vertices: (0,±2),asymptote:
E)vertices: (±2,5),asymptote:

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 124 في هذه المجموعة.
فتح الحزمة
k this deck
58
Find the center and vertices which located on the major axis of the ellipse.
A)center: (0,0)vertices: (0,-5), (0,5)
B)center: (0,0)vertices: (-3,0), (3,0)
C)center: (5,3)vertices: (-5,-3), (5,3)
D)center: (0,0)vertices: (-5,0), (5,0)
E)center: (5,0)vertices: (0,-3), (0,3)
A)center: (0,0)vertices: (0,-5), (0,5)
B)center: (0,0)vertices: (-3,0), (3,0)
C)center: (5,3)vertices: (-5,-3), (5,3)
D)center: (0,0)vertices: (-5,0), (5,0)
E)center: (5,0)vertices: (0,-3), (0,3)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 124 في هذه المجموعة.
فتح الحزمة
k this deck
59
Sketch the graph of the ellipse,using the lateral recta.
A)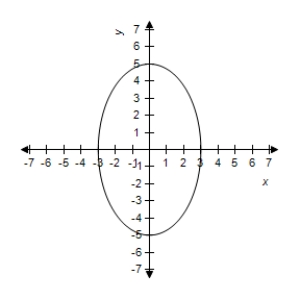
B)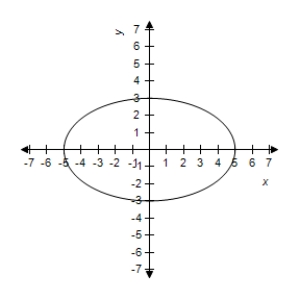
C)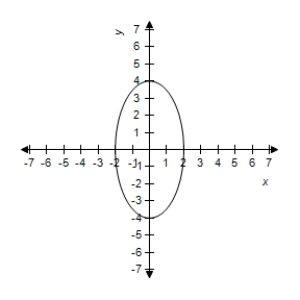
D)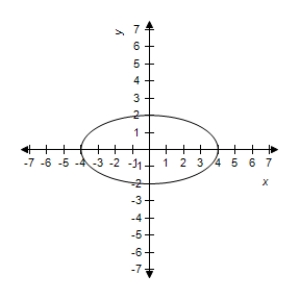
E)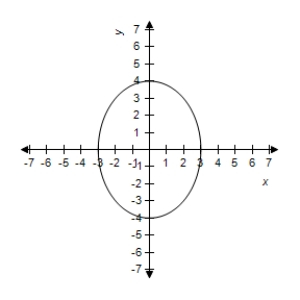
A)

B)

C)

D)

E)


فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 124 في هذه المجموعة.
فتح الحزمة
k this deck
60
Find the standard form of the equation of the parabola with the given characteristic and vertex at the origin. Focus:
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 124 في هذه المجموعة.
فتح الحزمة
k this deck
61
Find the standard form of the equation of the parabola with the given characteristics.
Vertex: ;directrix:
A)
B)
C)
D)
E)
Vertex: ;directrix:
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 124 في هذه المجموعة.
فتح الحزمة
k this deck
62
Find the standard form of the equation of the parabola with the given characteristics.
Vertex: ;focus:
A)
B)
C)
D)
E)
Vertex: ;focus:
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 124 في هذه المجموعة.
فتح الحزمة
k this deck
63
Find the vertex,focus,and directrix of the parabola.
A)Vertex: ;Focus: ;Directrix:
B)Vertex: ;Focus: ;Directrix:
C)Vertex: ;Focus: ;Directrix:
D)Vertex: ;Focus: ;Directrix:
E)Vertex: ;Focus: ;Directrix:
A)Vertex: ;Focus: ;Directrix:
B)Vertex: ;Focus: ;Directrix:
C)Vertex: ;Focus: ;Directrix:
D)Vertex: ;Focus: ;Directrix:
E)Vertex: ;Focus: ;Directrix:

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 124 في هذه المجموعة.
فتح الحزمة
k this deck
64
The equations of a parabola and a tangent line to the parabola are given.Select the correct graph of both equations in the same viewing window.
Parabola: Tangent Line:
A)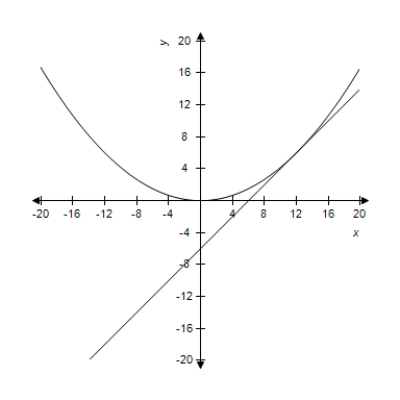
B)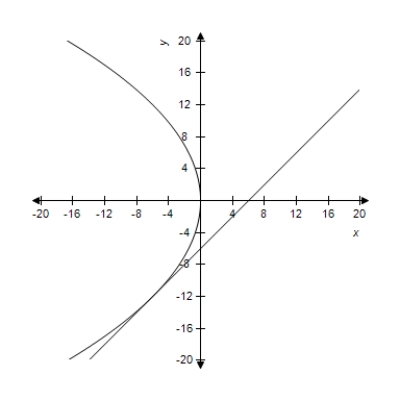
C)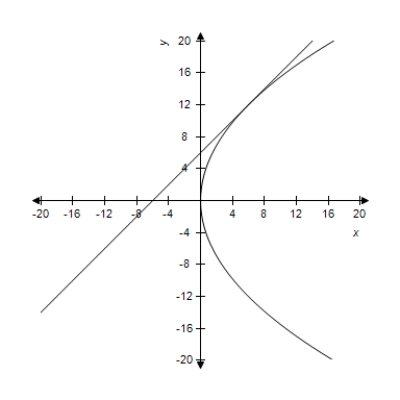
D)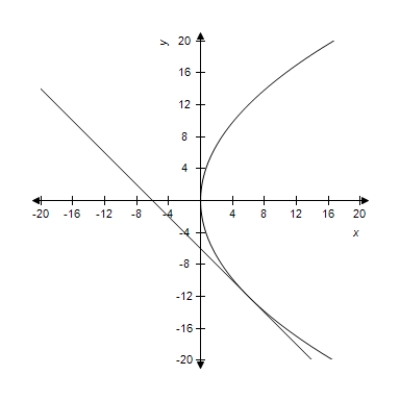
E)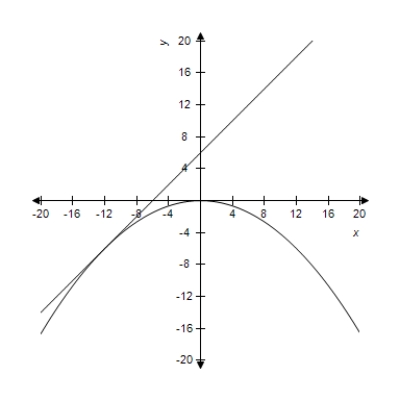
Parabola: Tangent Line:
A)

B)

C)

D)

E)


فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 124 في هذه المجموعة.
فتح الحزمة
k this deck
65
Find the equation of the parabola so that its graph matches the description. ;upper half of parabola
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 124 في هذه المجموعة.
فتح الحزمة
k this deck
66
Find the standard form of the equation of the parabola with the given characteristic and vertex at the origin.
Horizontal axis and passes through the point
A)
B)
C)
D)
E)
Horizontal axis and passes through the point
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 124 في هذه المجموعة.
فتح الحزمة
k this deck
67
Find the vertex,focus,and directrix of the parabola.
A)Vertex: (0,0);Focus: ;Directrix:
B)Vertex: (0,0);Focus: ;Directrix:
C)Vertex: (0,0);Focus: ;Directrix:
D)Vertex: (0,0);Focus: ;Directrix:
E)Vertex: (0,0);Focus: ;Directrix:
A)Vertex: (0,0);Focus: ;Directrix:
B)Vertex: (0,0);Focus: ;Directrix:
C)Vertex: (0,0);Focus: ;Directrix:
D)Vertex: (0,0);Focus: ;Directrix:
E)Vertex: (0,0);Focus: ;Directrix:

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 124 في هذه المجموعة.
فتح الحزمة
k this deck
68
Find the equation of the parabola so that its graph matches the description. ;lower half of parabola
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 124 في هذه المجموعة.
فتح الحزمة
k this deck
69
Find the vertex,focus,and directrix of the parabola.
A)Vertex: (0,0);Focus: ;Directrix:
B)Vertex: (0,0);Focus: ;Directrix:
C)Vertex: (0,0): Focus: Directrix:
D)Vertex: (0,0);Focus: ;Directrix:
E)Vertex: (0,0);Focus: ;Directrix:
A)Vertex: (0,0);Focus: ;Directrix:
B)Vertex: (0,0);Focus: ;Directrix:
C)Vertex: (0,0): Focus: Directrix:
D)Vertex: (0,0);Focus: ;Directrix:
E)Vertex: (0,0);Focus: ;Directrix:

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 124 في هذه المجموعة.
فتح الحزمة
k this deck
70
Find the vertex,focus,and directrix of the parabola.
A)Vertex: ;Focus: ;Directrix:
B)Vertex: ;Focus: ;Directrix:
C)Vertex: ;Focus: ;Directrix:
D)Vertex: ;Focus: ;Directrix:
E)Vertex: ;Focus: ;Directrix:
A)Vertex: ;Focus: ;Directrix:
B)Vertex: ;Focus: ;Directrix:
C)Vertex: ;Focus: ;Directrix:
D)Vertex: ;Focus: ;Directrix:
E)Vertex: ;Focus: ;Directrix:

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 124 في هذه المجموعة.
فتح الحزمة
k this deck
71
Find the vertex,focus,and directrix of the parabola.
A)Vertex: (0,0);Focus: ;Directrix:
B)Vertex:(0,0);Focus: ;Directrix:
C)Vertex: (0,0);Focus: ;Directrix:
D)Vertex: (0,0);Focus: ;Directrix:
E)Vertex: (0,0);Focus: ;Directrix:
A)Vertex: (0,0);Focus: ;Directrix:
B)Vertex:(0,0);Focus: ;Directrix:
C)Vertex: (0,0);Focus: ;Directrix:
D)Vertex: (0,0);Focus: ;Directrix:
E)Vertex: (0,0);Focus: ;Directrix:

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 124 في هذه المجموعة.
فتح الحزمة
k this deck
72
Find the vertex,focus,and directrix of the parabola.
A)Vertex: ;Focus: ;Directrix:
B)Vertex: ;Focus: ;Directrix:
C)Vertex: ;Focus: ;Directrix:
D)Vertex: ;Focus: ;Directrix:
E)Vertex: ;Focus: ;Directrix:
A)Vertex: ;Focus: ;Directrix:
B)Vertex: ;Focus: ;Directrix:
C)Vertex: ;Focus: ;Directrix:
D)Vertex: ;Focus: ;Directrix:
E)Vertex: ;Focus: ;Directrix:

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 124 في هذه المجموعة.
فتح الحزمة
k this deck
73
Find the standard form of the equation of the parabola with the given characteristics.
Vertex: ;directrix:
A)
B)
C)
D)
E)
Vertex: ;directrix:
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 124 في هذه المجموعة.
فتح الحزمة
k this deck
74
Find the vertex,focus,and directrix of the parabola.
A)Vertex: (0,0);Focus: ;Directrix:
B)Vertex: (0,0);Focus: ;Directrix:
C)Vertex: (0,0);Focus: ;Directrix:
D)Vertex: (0,0);Focus: ;Directrix:
E)Vertex: (0,0);Focus: ;Directrix:
A)Vertex: (0,0);Focus: ;Directrix:
B)Vertex: (0,0);Focus: ;Directrix:
C)Vertex: (0,0);Focus: ;Directrix:
D)Vertex: (0,0);Focus: ;Directrix:
E)Vertex: (0,0);Focus: ;Directrix:

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 124 في هذه المجموعة.
فتح الحزمة
k this deck
75
The equations of a parabola and a tangent line to the parabola are given.Select the correct graph of both equations in the same viewing window.
Parabola: Tangent Line:
A)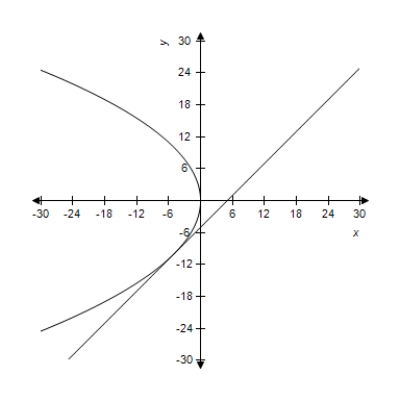
B)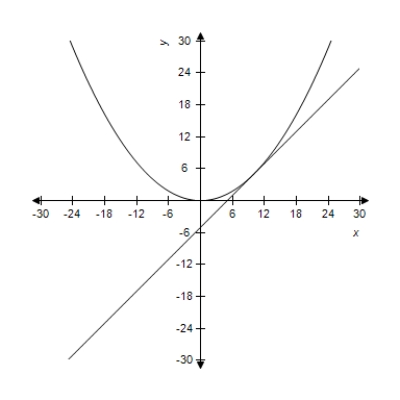
C)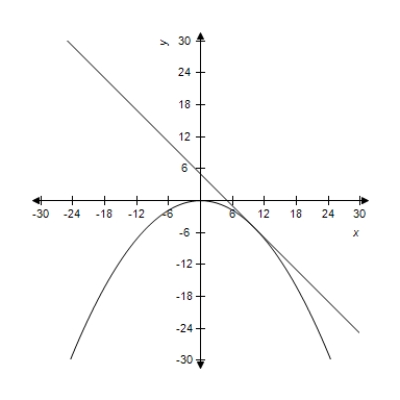
D)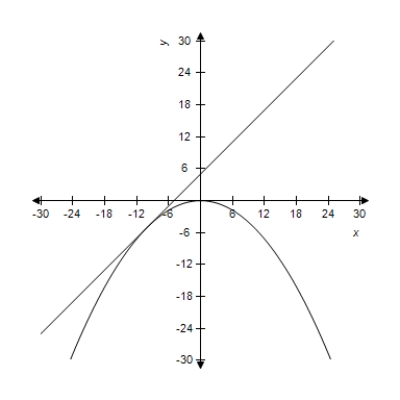
E)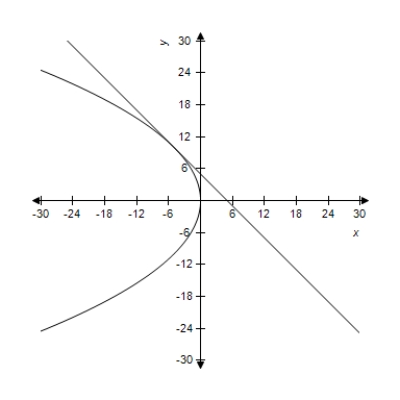
Parabola: Tangent Line:
A)

B)

C)

D)

E)


فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 124 في هذه المجموعة.
فتح الحزمة
k this deck
76
Find the vertex,focus,and directrix of the parabola.
A)Vertex: ;Focus: ;Directrix:
B)Vertex: ;Focus: ;Directrix:
C)Vertex: ;Focus: ;Directrix:
D)Vertex: ;Focus: ;Directrix:
E)Vertex: ;Focus: ;Directrix:
A)Vertex: ;Focus: ;Directrix:
B)Vertex: ;Focus: ;Directrix:
C)Vertex: ;Focus: ;Directrix:
D)Vertex: ;Focus: ;Directrix:
E)Vertex: ;Focus: ;Directrix:

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 124 في هذه المجموعة.
فتح الحزمة
k this deck
77
The revenue R (in dollars)generated by the sale of x units of a patio furniture set is given by .Select the correct graph of the function.
A)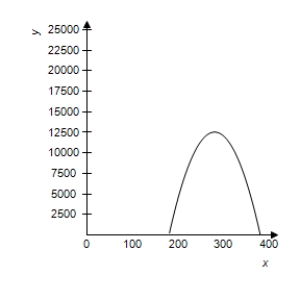
B)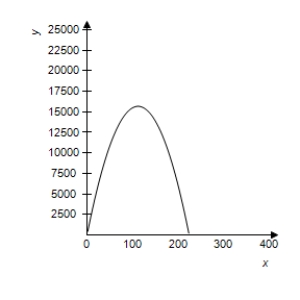
C)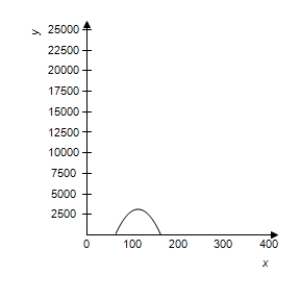 .
.
D)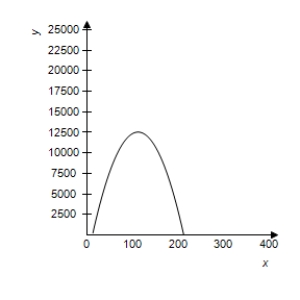
E)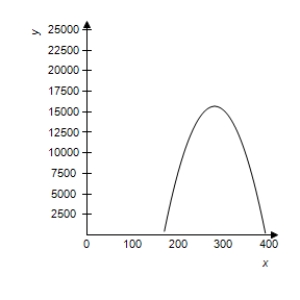
A)

B)

C)
 .
.D)

E)


فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 124 في هذه المجموعة.
فتح الحزمة
k this deck
78
Find the standard form of the equation of the parabola with the given characteristics.
Vertex: ;focus:
A)
B)
C)
D)
E)
Vertex: ;focus:
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 124 في هذه المجموعة.
فتح الحزمة
k this deck
79
The revenue R (in dollars)generated by the sale of x units of a patio furniture set is given by .Approximate the number of sales that will maximize revenue.
A) The revenue is maximum when units.
B) The revenue is maximum when units.
C) The revenue is maximum when units.
D) The revenue is maximum when units.
E) The revenue is maximum when units.
A) The revenue is maximum when units.
B) The revenue is maximum when units.
C) The revenue is maximum when units.
D) The revenue is maximum when units.
E) The revenue is maximum when units.

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 124 في هذه المجموعة.
فتح الحزمة
k this deck
80
Find the standard form of the equation of the parabola with the given characteristic and vertex at the origin.
Horizontal axis and passes through the point
A)
B)
C)
D)
E)
Horizontal axis and passes through the point
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 124 في هذه المجموعة.
فتح الحزمة
k this deck








