Deck 20: Properties of Logarithms
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال

فتح الحزمة
قم بالتسجيل لفتح البطاقات في هذه المجموعة!
Unlock Deck
Unlock Deck
1/57
العب
ملء الشاشة (f)
Deck 20: Properties of Logarithms
1
Rewrite the logarithm as a ratio of natural logarithms. ?
Log5 19
?
A)
B)
C)
D)
E)None of these
Log5 19
?
A)
B)
C)
D)
E)None of these
2
Rewrite the logarithm as a ratio of common logarithms. ?
Log5 16
?
A)
B)
C)
D)
E)None of these
Log5 16
?
A)
B)
C)
D)
E)None of these
3
Rewrite the logarithm as a ratio of natural logarithms. ?
Log1/5 x
?
A)
B)
C)
D)
E)None of these
Log1/5 x
?
A)
B)
C)
D)
E)None of these
4
Use the properties of logarithms to expand the expression as a sum,difference,and/or constant multiple of logarithms.(Assume all variables are positive. ) ?
Log 5x4y
?
A)log 5 × 4log x × log y
B)log 5 + 4log x - log y
C)log 5 + log x + 4log y
D)log 5 + 4log x + log y
E)
Log 5x4y
?
A)log 5 × 4log x × log y
B)log 5 + 4log x - log y
C)log 5 + log x + 4log y
D)log 5 + 4log x + log y
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 57 في هذه المجموعة.
فتح الحزمة
k this deck
5
Rewrite the logarithm as a ratio of common logarithms. ?
Log1/7 x
?
A)
B)
C)
D)
E)None of these
Log1/7 x
?
A)
B)
C)
D)
E)None of these

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 57 في هذه المجموعة.
فتح الحزمة
k this deck
6
Rewrite the logarithm as a ratio of common logarithms. ?
Log2.7 x
?
A)
B)?
C)
D)
E)None of these
Log2.7 x
?
A)
B)?
C)
D)
E)None of these

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 57 في هذه المجموعة.
فتح الحزمة
k this deck
7
Condense the expression to the logarithm of a single quantity. ?
Ln 8 + ln x
?
A)ln x8
B)ln 8x
C)
D)ln 8x
E)ln 8 × ln x
Ln 8 + ln x
?
A)ln x8
B)ln 8x
C)
D)ln 8x
E)ln 8 × ln x

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 57 في هذه المجموعة.
فتح الحزمة
k this deck
8
Use the properties of logarithms to expand the expression as a sum,difference,and/or constant multiple of logarithms.(Assume all variables are positive. ) ?
Log3 9x
?
A)
B)log3 9? × log3 x
C)log3 9? + log3 x
D)log3 9? - log3 x
E)None of these
Log3 9x
?
A)
B)log3 9? × log3 x
C)log3 9? + log3 x
D)log3 9? - log3 x
E)None of these

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 57 في هذه المجموعة.
فتح الحزمة
k this deck
9
Condense the expression to the logarithm of a single quantity. ?
Log x - 3log(x + 1)
?
A)3log(x(x + 1))
B)
C)
D)log(x(x + 1)3)
E)
Log x - 3log(x + 1)
?
A)3log(x(x + 1))
B)
C)
D)log(x(x + 1)3)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 57 في هذه المجموعة.
فتح الحزمة
k this deck
10
Rewrite the logarithm as a ratio of common logarithms.? ?
A)
B)
C)
D)
E)None of these
A)
B)
C)
D)
E)None of these

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 57 في هذه المجموعة.
فتح الحزمة
k this deck
11
Use the properties of logarithms to expand the expression as a sum,difference,and/or constant multiple of logarithms.(Assume all variables are positive. )? ?
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 57 في هذه المجموعة.
فتح الحزمة
k this deck
12
Rewrite the logarithm as a ratio of natural logarithms. ?
Log3.1 x
?
A)
B)
C)
D)
E)None of these
Log3.1 x
?
A)
B)
C)
D)
E)None of these

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 57 في هذه المجموعة.
فتح الحزمة
k this deck
13
Use the properties of logarithms to expand the expression as a sum,difference,and/or constant multiple of logarithms.(Assume all variables are positive. ) ?
Ln 9x
?
A)ln 9 - ln x
B)
C)ln 9 × ln x
D)ln 9 + ln x
E)None of these
Ln 9x
?
A)ln 9 - ln x
B)
C)ln 9 × ln x
D)ln 9 + ln x
E)None of these

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 57 في هذه المجموعة.
فتح الحزمة
k this deck
14
Condense the expression to the logarithm of a single quantity. ?
Log x - 2log y + 3log z
?
A)
B)
C)
D)
E)
Log x - 2log y + 3log z
?
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 57 في هذه المجموعة.
فتح الحزمة
k this deck
15
Find the exact value of the logarithmic expression without using a calculator.
Log6 216
A)3
B)6
C)216
D)35
E)None of these
Log6 216
A)3
B)6
C)216
D)35
E)None of these

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 57 في هذه المجموعة.
فتح الحزمة
k this deck
16
Rewrite the logarithm as a ratio of natural logarithms.? ?
A)
B)
C)
D)
E)None of these
A)
B)
C)
D)
E)None of these

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 57 في هذه المجموعة.
فتح الحزمة
k this deck
17
Find the exact value of the logarithmic expression without using a calculator.? ?
A)3
B)
C)7
D)?21
E)None of these
A)3
B)
C)7
D)?21
E)None of these

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 57 في هذه المجموعة.
فتح الحزمة
k this deck
18
Find the exact value of the logarithmic expression without using a calculator.
5 ln e7
A)7
B)35
C)5
D)e
E)1
5 ln e7
A)7
B)35
C)5
D)e
E)1

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 57 في هذه المجموعة.
فتح الحزمة
k this deck
19
Use the properties of logarithms to expand the expression as a sum,difference,and/or constant multiple of logarithms.(Assume all variables are positive. )? ?
A)3log8 x + 3log8 y + 3log8 z
B)3log8 x + 3log8 y - 3log8 z
C)
D)3log8 x - 3log8 y - 3log8 z
E)3log8 x - 3log8 y + 3log8 z
A)3log8 x + 3log8 y + 3log8 z
B)3log8 x + 3log8 y - 3log8 z
C)
D)3log8 x - 3log8 y - 3log8 z
E)3log8 x - 3log8 y + 3log8 z

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 57 في هذه المجموعة.
فتح الحزمة
k this deck
20
Condense the expression to the logarithm of a single quantity. ?
Log2 10 + log2 x
?
A)log2 (10 - x)
B)
C)log2 (10 + x)
D)log2 10x
E)log2 10x
Log2 10 + log2 x
?
A)log2 (10 - x)
B)
C)log2 (10 + x)
D)log2 10x
E)log2 10x

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 57 في هذه المجموعة.
فتح الحزمة
k this deck
21
Rewrite the logarithm log4 17 in terms of the natural logarithm.
A)
B)
C)
D)ln 17
E)ln 4 ln 17
A)
B)
C)
D)ln 17
E)ln 4 ln 17

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 57 في هذه المجموعة.
فتح الحزمة
k this deck
22
Find the exact value of without using a calculator.
A)
B)
C)
D)-1
E)
A)
B)
C)
D)-1
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 57 في هذه المجموعة.
فتح الحزمة
k this deck
23
Condense the expression to the logarithm of a single quantity.? ?
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 57 في هذه المجموعة.
فتح الحزمة
k this deck
24
Evaluate the logarithm using the change-of-base formula.Round your result to three decimal places.
Log15 1,500
A)5.701
B)3.701
C)2.701
D)6.701
E)4.701
Log15 1,500
A)5.701
B)3.701
C)2.701
D)6.701
E)4.701

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 57 في هذه المجموعة.
فتح الحزمة
k this deck
25
Evaluate the logarithm using the change-of-base formula.Round your result to three decimal places.
Log2 7
A)2.807
B)6.807
C)4.807
D)5.807
E)3.807
Log2 7
A)2.807
B)6.807
C)4.807
D)5.807
E)3.807

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 57 في هذه المجموعة.
فتح الحزمة
k this deck
26
Rewrite the logarithm log6 412 in terms of the common logarithm (base 10).
A)log 6 log 412
B)
C)
D)log 412
E)
A)log 6 log 412
B)
C)
D)log 412
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 57 في هذه المجموعة.
فتح الحزمة
k this deck
27
Use a graphing utility to graph the functions given by ?
Y1 = ln x - ln (x - 4)
?
And? ?
In the same viewing window.
?
A)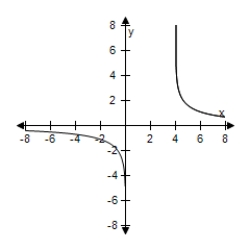
B)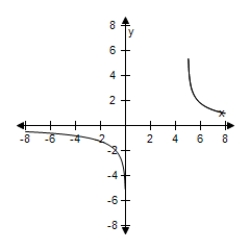
C)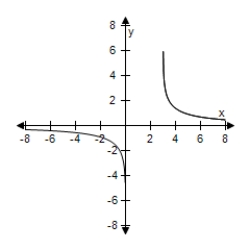
D)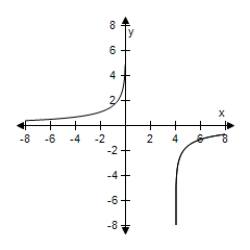
E)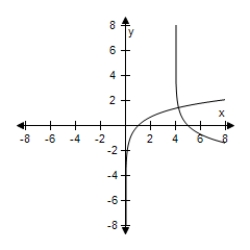
Y1 = ln x - ln (x - 4)
?
And? ?
In the same viewing window.
?
A)

B)

C)

D)

E)


فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 57 في هذه المجموعة.
فتح الحزمة
k this deck
28
Use the change-of-base formula to rewrite the logarithm as a ratio of logarithms.Then use a graphing utility to graph the ratio.
?
F(x)= log4 x
?
A)f(x)= log x + log 4 = ln x + ln 4 ?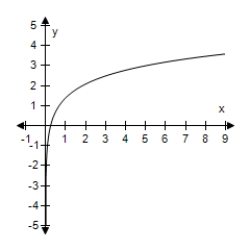
B)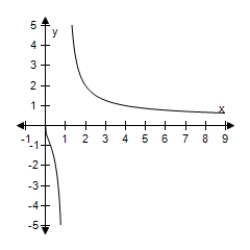
C)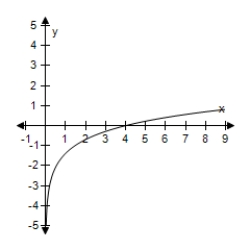
D)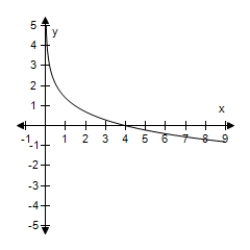
E)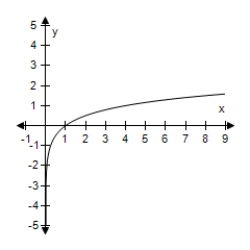 ?
?
?
F(x)= log4 x
?
A)f(x)= log x + log 4 = ln x + ln 4 ?

B)

C)

D)

E)
 ?
?
فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 57 في هذه المجموعة.
فتح الحزمة
k this deck
29
Evaluate the logarithm using the change-of-base formula.Round your result to three decimal places.? ?
A)-0.631
B)2.369
C)0.369
D)-1.631
E)1.369
A)-0.631
B)2.369
C)0.369
D)-1.631
E)1.369

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 57 في هذه المجموعة.
فتح الحزمة
k this deck
30
Use the change-of-base formula to rewrite the logarithm as a ratio of logarithms.Then use a graphing utility to graph the ratio.
?
F(x)= log11.7 x
?
A)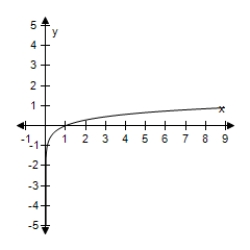
B)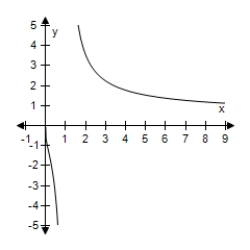
C)f(x)= log x? + log 11.7 = ln x? + ln 11.7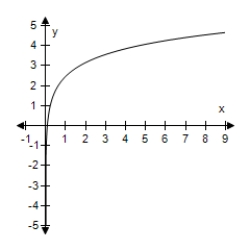
D)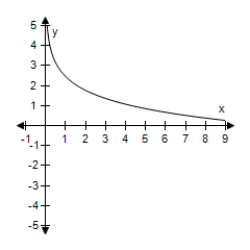
E) ?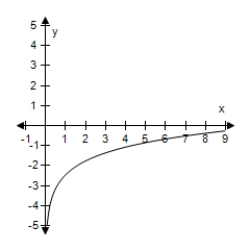
?
F(x)= log11.7 x
?
A)

B)

C)f(x)= log x? + log 11.7 = ln x? + ln 11.7

D)

E) ?


فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 57 في هذه المجموعة.
فتح الحزمة
k this deck
31
Use the change-of-base formula to rewrite the logarithm as a ratio of logarithms.Then use a graphing utility to graph the ratio.? ?
A)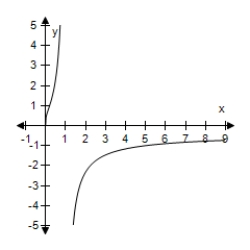
B)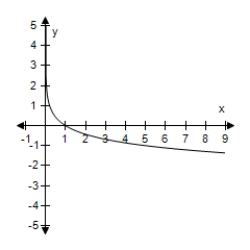
C)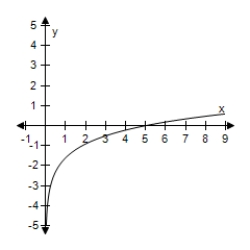
D)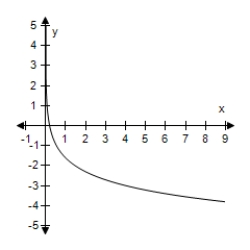
E)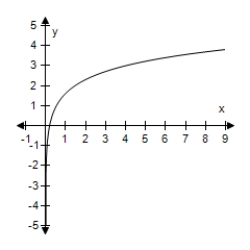
A)

B)

C)

D)

E)


فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 57 في هذه المجموعة.
فتح الحزمة
k this deck
32
Use the properties of logarithms to rewrite and simplify the logarithmic expression.
Ln (3e4)
A)ln 4
B)ln 3 + 4
C)ln 4 + 3
D)ln 7
E)ln 3
Ln (3e4)
A)ln 4
B)ln 3 + 4
C)ln 4 + 3
D)ln 7
E)ln 3

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 57 في هذه المجموعة.
فتح الحزمة
k this deck
33
Condense the expression to the logarithm of a single quantity.? ?
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 57 في هذه المجموعة.
فتح الحزمة
k this deck
34
Evaluate the logarithm log7 126 using the change of base formula.Round to 3 decimal places.
A)4.836
B)2.485
C)0.402
D)9.411
E)2.1
A)4.836
B)2.485
C)0.402
D)9.411
E)2.1

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 57 في هذه المجموعة.
فتح الحزمة
k this deck
35
Simplify the expression log5 175.
A)35log5 2
B)2 log5 7
C)7
D)2 + log5 7
E)The expression cannot be simplified.
A)35log5 2
B)2 log5 7
C)7
D)2 + log5 7
E)The expression cannot be simplified.

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 57 في هذه المجموعة.
فتح الحزمة
k this deck
36
Use the properties of logarithms to rewrite and simplify the logarithmic expression.
Log2 (42 · 74)
A)4 + 9 log2 4
B)9 + 4 log2 4
C)2 + 2 log2 7
D)4 + 4 log2 7
E)2 + 4 log2 7
Log2 (42 · 74)
A)4 + 9 log2 4
B)9 + 4 log2 4
C)2 + 2 log2 7
D)4 + 4 log2 7
E)2 + 4 log2 7

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 57 في هذه المجموعة.
فتح الحزمة
k this deck
37
Evaluate the logarithm log1/3 0.603 using the change of base formula.Round to 3 decimal places.
A)2.172
B)0.556
C)-0.506
D)-0.22
E)0.46
A)2.172
B)0.556
C)-0.506
D)-0.22
E)0.46

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 57 في هذه المجموعة.
فتح الحزمة
k this deck
38
Evaluate the logarithm using the change-of-base formula.Round your result to three decimal places.
Log9 0.1
A)2.952
B)-0.048
C)-1.048
D)1.952
E)0.952
Log9 0.1
A)2.952
B)-0.048
C)-1.048
D)1.952
E)0.952

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 57 في هذه المجموعة.
فتح الحزمة
k this deck
39
Condense the expression to the logarithm of a single quantity.? ?
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 57 في هذه المجموعة.
فتح الحزمة
k this deck
40
Use the properties of logarithms to rewrite and simplify the logarithmic expression.? ?
A)ln 2
B)ln 5
C)ln 2 - 3
D)ln 3 - 2
E)ln 3
A)ln 2
B)ln 5
C)ln 2 - 3
D)ln 3 - 2
E)ln 3

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 57 في هذه المجموعة.
فتح الحزمة
k this deck
41
Assume that x,y,and z are positive numbers.Use the properties of logarithms to write the expression as the logarithm of one quantity. ?
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 57 في هذه المجموعة.
فتح الحزمة
k this deck
42
Assume that x is a positive number.Use the properties of logarithms to write the expression ?
Logb (x + 8)- logb x as the logarithm of one quantity.
?
A)
B)
C)
D)
E)logb (x2 - 8x)
Logb (x + 8)- logb x as the logarithm of one quantity.
?
A)
B)
C)
D)
E)logb (x2 - 8x)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 57 في هذه المجموعة.
فتح الحزمة
k this deck
43
Condense the expression 7(log x - log y)to the logarithm of a single term.
A)
B)
C)
D)
E)7(log x - log y)
A)
B)
C)
D)
E)7(log x - log y)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 57 في هذه المجموعة.
فتح الحزمة
k this deck
44
Assume that x,y,and c are positive numbers.Use the properties of logarithms to write the expression in terms of the logarithms of x and y. ?
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 57 في هذه المجموعة.
فتح الحزمة
k this deck
45
Simplify the expression .
A)3
B)0
C)-9
D)-81
E)The expression cannot be simplified.
A)3
B)0
C)-9
D)-81
E)The expression cannot be simplified.

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 57 في هذه المجموعة.
فتح الحزمة
k this deck
46
Condense the expression to the logarithm of a single term.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 57 في هذه المجموعة.
فتح الحزمة
k this deck
47
Condense the expression 3(log x - log y)to the logarithm of a single term.
A)
B)
C)
D)3(log x - log y)
E)
A)
B)
C)
D)3(log x - log y)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 57 في هذه المجموعة.
فتح الحزمة
k this deck
48
Find the exact value of without using a calculator.
A)5
B)2.5
C)1.25
D)2
E)3
A)5
B)2.5
C)1.25
D)2
E)3

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 57 في هذه المجموعة.
فتح الحزمة
k this deck
49
Assume that x,y,and c are positive numbers.Use the properties of logarithms to write the expression in terms of the logarithms of x and y. ?
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 57 في هذه المجموعة.
فتح الحزمة
k this deck
50
Assume that x,y and c are positive numbers.Use the properties of logarithms to write the expression in terms of the logarithms of x and y. ?
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 57 في هذه المجموعة.
فتح الحزمة
k this deck
51
Assume that x,y,z and b are positive numbers.Use the properties of logarithms to write the expression in terms of the logarithms of x,y,and z. ?
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 57 في هذه المجموعة.
فتح الحزمة
k this deck
52
Put the expressions in the appropriate order: .
A)
B)
C)?
D)
E)The expressions are equivalent.
A)
B)
C)?
D)
E)The expressions are equivalent.

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 57 في هذه المجموعة.
فتح الحزمة
k this deck
53
Condense the expression to the logarithm of a single term.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 57 في هذه المجموعة.
فتح الحزمة
k this deck
54
Find the exact value of without using a calculator.
A)
B)
C)?
D)
E)-1
A)
B)
C)?
D)
E)-1

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 57 في هذه المجموعة.
فتح الحزمة
k this deck
55
The pH of an acidic solution is a measure of the concentration of the acid particles in the solution,with smaller values of the pH indicating higher acid concentration.In a laboratory experiment,the pH of a certain acid solution is changed by dissolving over-the-counter antacid tablets into the solution.In this experiment,the pH changes according to the equation , where x is the number of grams of antacid added to the solution.What is the pH of the solution after the addition of 0.05 grams of antacid tablet?
A)5.99
B)4.02
C)-0.85
D)4.29
E)5.14
A)5.99
B)4.02
C)-0.85
D)4.29
E)5.14

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 57 في هذه المجموعة.
فتح الحزمة
k this deck
56
Condense the expression log4 x + log4 3 to the logarithm of a single term.
A)log4 -3x
B)log4 3x
C)log4 x3
D)log(3x)4
E)log4 (x + 3)
A)log4 -3x
B)log4 3x
C)log4 x3
D)log(3x)4
E)log4 (x + 3)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 57 في هذه المجموعة.
فتح الحزمة
k this deck
57
Find the exact value of without using a calculator.
A)1
B)4
C)11
D)
E)
A)1
B)4
C)11
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 57 في هذه المجموعة.
فتح الحزمة
k this deck








