Deck 34: Sum and Difference Formulas
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال

فتح الحزمة
قم بالتسجيل لفتح البطاقات في هذه المجموعة!
Unlock Deck
Unlock Deck
1/62
العب
ملء الشاشة (f)
Deck 34: Sum and Difference Formulas
1
A weight is attached to a spring suspended vertically from a ceiling.When a driving force is applied to the system,the weight moves vertically from its equilibrium position,and this motion is modeled by where y is the distance from equilibrium (in feet)and t is the time (in seconds).
Use the identity where ,to write the model in the form .
A)
B)
C)
D)
E)
Use the identity where ,to write the model in the form .
A)
B)
C)
D)
E)
2
Find the expression as the sine of an angle.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)
3
Find the expression as the sine or cosine of an angle.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)
4
Simplify the expression algebraically.
A)
B)0
C)
D)1
E)6
A)
B)0
C)
D)1
E)6

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 62 في هذه المجموعة.
فتح الحزمة
k this deck
5
Simplify the expression algebraically.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 62 في هذه المجموعة.
فتح الحزمة
k this deck
6
Find the expression as the tangent of an angle.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 62 في هذه المجموعة.
فتح الحزمة
k this deck
7
Simplify the expression algebraically.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 62 في هذه المجموعة.
فتح الحزمة
k this deck
8
Simplify the expression algebraically.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 62 في هذه المجموعة.
فتح الحزمة
k this deck
9
Simplify the expression algebraically.
A)-
B)
C)
D)
E)
A)-
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 62 في هذه المجموعة.
فتح الحزمة
k this deck
10
Find the expression as the sine or cosine of an angle.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 62 في هذه المجموعة.
فتح الحزمة
k this deck
11
Find the expression as the tangent of an angle.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 62 في هذه المجموعة.
فتح الحزمة
k this deck
12
Simplify the expression algebraically.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 62 في هذه المجموعة.
فتح الحزمة
k this deck
13
Simplify the expression algebraically.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 62 في هذه المجموعة.
فتح الحزمة
k this deck
14
A weight is attached to a spring suspended vertically from a ceiling.When a driving force is applied to the system,the weight moves vertically from its equilibrium position,and this motion is modeled by where y is the distance from equilibrium (in feet)and t is the time (in seconds). Find the amplitude of the oscillations of the weight.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 62 في هذه المجموعة.
فتح الحزمة
k this deck
15
Find the expression as the tangent of an angle.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 62 في هذه المجموعة.
فتح الحزمة
k this deck
16
Simplify the expression algebraically.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 62 في هذه المجموعة.
فتح الحزمة
k this deck
17
Simplify the expression algebraically.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 62 في هذه المجموعة.
فتح الحزمة
k this deck
18
Find the expression as the cosine of an angle.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 62 في هذه المجموعة.
فتح الحزمة
k this deck
19
Simplify the expression algebraically.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 62 في هذه المجموعة.
فتح الحزمة
k this deck
20
Find the expression as the sine of an angle.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 62 في هذه المجموعة.
فتح الحزمة
k this deck
21
Use the formula ,where to rewrite the trigonometric expression in the following form.
A)
B)9
C)9
D)
E)2
A)
B)9
C)9
D)
E)2

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 62 في هذه المجموعة.
فتح الحزمة
k this deck
22
Simplify the following expression algebraically.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 62 في هذه المجموعة.
فتح الحزمة
k this deck
23
Use the formula ,where ,to rewrite the trigonometric expression in the following form.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 62 في هذه المجموعة.
فتح الحزمة
k this deck
24
Simplify the following expression algebraically.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 62 في هذه المجموعة.
فتح الحزمة
k this deck
25
Use the formula ,where ,to rewrite the trigonometric expression in the following form.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 62 في هذه المجموعة.
فتح الحزمة
k this deck
26
Simplify the following expression algebraically.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 62 في هذه المجموعة.
فتح الحزمة
k this deck
27
Use a graphing utility to select correct graph of and in the same viewing window.Use the graphs to determine whether .Explain your reasoning.
A)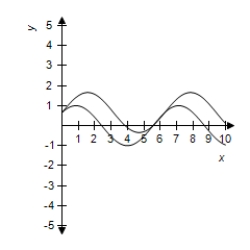 No, because their graphs are different.
No, because their graphs are different.
B)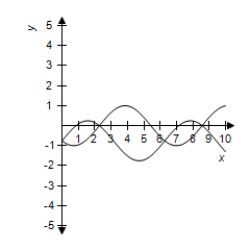 Yes, because their graphs are different.
Yes, because their graphs are different.
C)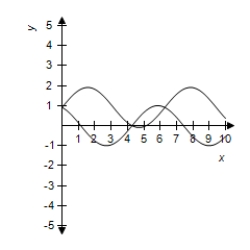 Yes, because their graphs are same.
Yes, because their graphs are same.
D)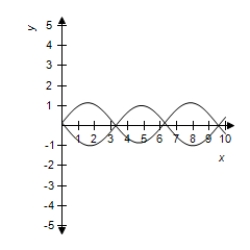 No, because their graphs are Same.
No, because their graphs are Same.
E)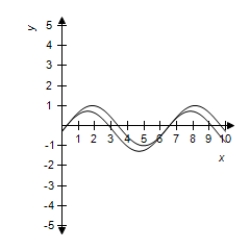 No, because their graphs are different.
No, because their graphs are different.
A)
 No, because their graphs are different.
No, because their graphs are different.B)
 Yes, because their graphs are different.
Yes, because their graphs are different.C)
 Yes, because their graphs are same.
Yes, because their graphs are same.D)
 No, because their graphs are Same.
No, because their graphs are Same.E)
 No, because their graphs are different.
No, because their graphs are different.
فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 62 في هذه المجموعة.
فتح الحزمة
k this deck
28
Use the formula ,where to rewrite the trigonometric expression in the following form.
A)
B)
C)3
D)
E)
A)
B)
C)3
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 62 في هذه المجموعة.
فتح الحزمة
k this deck
29
Simplify the following expression algebraically.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 62 في هذه المجموعة.
فتح الحزمة
k this deck
30
Use a graphing utility to select the correct graph of and in the same viewing window.Use the graphs to determine whether .Explain your reasoning.
A)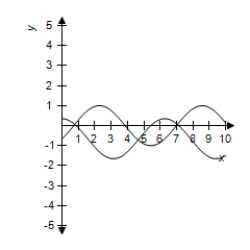 No, because their graphs are different.
No, because their graphs are different.
B)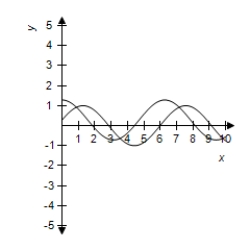 No, because their graphs are Same.
No, because their graphs are Same.
C)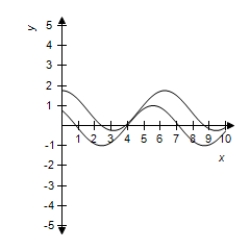 Yes, because their graphs are different.
Yes, because their graphs are different.
D)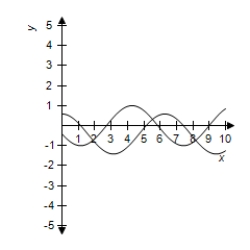 Yes, because their graphs are same.
Yes, because their graphs are same.
E)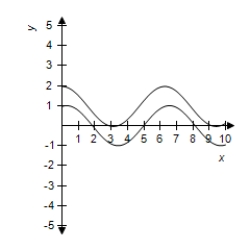 No, because their graphs are different.
No, because their graphs are different.
A)
 No, because their graphs are different.
No, because their graphs are different.B)
 No, because their graphs are Same.
No, because their graphs are Same.C)
 Yes, because their graphs are different.
Yes, because their graphs are different.D)
 Yes, because their graphs are same.
Yes, because their graphs are same.E)
 No, because their graphs are different.
No, because their graphs are different.
فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 62 في هذه المجموعة.
فتح الحزمة
k this deck
31
Use the formula ,where to rewrite the trigonometric expression in the following form.
A)6
B)
C)
D)13
E)13
A)6
B)
C)
D)13
E)13

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 62 في هذه المجموعة.
فتح الحزمة
k this deck
32
Use the formula ,where ,to rewrite the trigonometric expression in the form.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 62 في هذه المجموعة.
فتح الحزمة
k this deck
33
Use the formula ,where ,to rewrite the trigonometric expression in the form 9
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 62 في هذه المجموعة.
فتح الحزمة
k this deck
34
Find the exact value of the given expression using a sum or difference formula.
A)
B)
C)
D)
A)
B)
C)
D)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 62 في هذه المجموعة.
فتح الحزمة
k this deck
35
Find the exact value of the given expression.
A)
B)
C)
D)
A)
B)
C)
D)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 62 في هذه المجموعة.
فتح الحزمة
k this deck
36
Use the formula ,where ,to rewrite the trigonometric expression in the following form.
A)2
B)
C)
D)2
E)8
A)2
B)
C)
D)2
E)8

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 62 في هذه المجموعة.
فتح الحزمة
k this deck
37
Find the exact value of the given expression.
A)
B)
C)
D)
A)
B)
C)
D)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 62 في هذه المجموعة.
فتح الحزمة
k this deck
38
Use the formula ,where to rewrite the trigonometric expression in the following form.
A)
B)
C)7
D)3
E)3
A)
B)
C)7
D)3
E)3

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 62 في هذه المجموعة.
فتح الحزمة
k this deck
39
Use the formula ,where ,to rewrite the trigonometric expression in the following form.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 62 في هذه المجموعة.
فتح الحزمة
k this deck
40
Find the exact value of the given expression using a sum or difference formula.
A)
B)
C)
D)
A)
B)
C)
D)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 62 في هذه المجموعة.
فتح الحزمة
k this deck
41
Find the exact value of given that and .(Both u and v are in Quadrant II. )
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 62 في هذه المجموعة.
فتح الحزمة
k this deck
42
Find the exact value of the given expression.
A)
B)
C)
D)
A)
B)
C)
D)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 62 في هذه المجموعة.
فتح الحزمة
k this deck
43
Simplify the given expression algebraically.
A)
B)
C)
D)1
E)
A)
B)
C)
D)1
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 62 في هذه المجموعة.
فتح الحزمة
k this deck
44
Write the given expression as the cosine of an angle.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 62 في هذه المجموعة.
فتح الحزمة
k this deck
45
Find the exact value of the given expression.
A)
B)
C)
D)
A)
B)
C)
D)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 62 في هذه المجموعة.
فتح الحزمة
k this deck
46
Write the given expression as the tangent of an angle.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 62 في هذه المجموعة.
فتح الحزمة
k this deck
47
Find the exact solutions of the given equation in the interval [0,2π). sin 2x = sin x
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 62 في هذه المجموعة.
فتح الحزمة
k this deck
48
Simplify the given expression algebraically.
A)
B)
C)
D)
E)1
A)
B)
C)
D)
E)1

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 62 في هذه المجموعة.
فتح الحزمة
k this deck
49
Write the given expression as the cosine of an angle.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 62 في هذه المجموعة.
فتح الحزمة
k this deck
50
Write the given expression as the sine of an angle.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 62 في هذه المجموعة.
فتح الحزمة
k this deck
51
Write the given expression as an algebraic expression. cos(arccos x - arcsin x)
A)
B)
C)
D)1
E)
A)
B)
C)
D)1
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 62 في هذه المجموعة.
فتح الحزمة
k this deck
52
Find the exact value of given that and .(Both u and v are in Quadrant II. )
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 62 في هذه المجموعة.
فتح الحزمة
k this deck
53
Find the exact value of the given expression.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 62 في هذه المجموعة.
فتح الحزمة
k this deck
54
Find the exact value of given that and .(Both u and v are in Quadrant II. )
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 62 في هذه المجموعة.
فتح الحزمة
k this deck
55
Write the given expression as the tangent of an angle.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 62 في هذه المجموعة.
فتح الحزمة
k this deck
56
Find the exact value of the given expression using a sum or difference formula.
A)
B)
C)
D)
A)
B)
C)
D)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 62 في هذه المجموعة.
فتح الحزمة
k this deck
57
Write the given expression as the sine of an angle.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 62 في هذه المجموعة.
فتح الحزمة
k this deck
58
Evaluate the expression.
A)
B)0
C)
D)
E)
A)
B)0
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 62 في هذه المجموعة.
فتح الحزمة
k this deck
59
Find the exact value of given that and .(Both u and v are in Quadrant II. )
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 62 في هذه المجموعة.
فتح الحزمة
k this deck
60
Find the exact value of the given expression using a sum or difference formula.
A)
B)
C)
D)
A)
B)
C)
D)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 62 في هذه المجموعة.
فتح الحزمة
k this deck
61
Find the exact solutions of the given equation in the interval . sin 4x= -2sin 2x
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 62 في هذه المجموعة.
فتح الحزمة
k this deck
62
Verify the given identity. 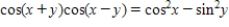
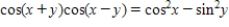

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 62 في هذه المجموعة.
فتح الحزمة
k this deck








