Deck 35: Multiple Angle and Product to Sum Formulas
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال

فتح الحزمة
قم بالتسجيل لفتح البطاقات في هذه المجموعة!
Unlock Deck
Unlock Deck
1/50
العب
ملء الشاشة (f)
Deck 35: Multiple Angle and Product to Sum Formulas
1
Use the product-to-sum formulas to rewrite the product as a sum or difference.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)
2
Use the sum-to-product formulas to rewrite the sum or difference as a product.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)
3
Use the product-to-sum formulas to rewrite the product as a sum or difference.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)
4
Use the product-to-sum formulas to rewrite the product as a sum or difference.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 50 في هذه المجموعة.
فتح الحزمة
k this deck
5
Use a double-angle formula to rewrite the expression.
10 cos2 x - 5
A)5 cos x
B)cos 5x
C)10 cos 2x
D)10 cos x
E)5 cos 2x
10 cos2 x - 5
A)5 cos x
B)cos 5x
C)10 cos 2x
D)10 cos x
E)5 cos 2x

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 50 في هذه المجموعة.
فتح الحزمة
k this deck
6
Use the sum-to-product formulas to rewrite the sum or difference as a product.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 50 في هذه المجموعة.
فتح الحزمة
k this deck
7
Use the product-to-sum formulas to rewrite the product as a sum or difference.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 50 في هذه المجموعة.
فتح الحزمة
k this deck
8
Use a double-angle formula to rewrite the expression.
3 - 6 sin2 x
A)6 cos x
B)3 sin 2x
C)3 sin x
D)3 cos 2x
E)6 cos 2x
3 - 6 sin2 x
A)6 cos x
B)3 sin 2x
C)3 sin x
D)3 cos 2x
E)6 cos 2x

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 50 في هذه المجموعة.
فتح الحزمة
k this deck
9
Use the figure to find the exact value of the trigonometric function.
Csc 2θ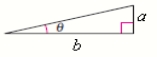
A = 1,b = 6
A)
B)
C)
D)
E)
Csc 2θ

A = 1,b = 6
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 50 في هذه المجموعة.
فتح الحزمة
k this deck
10
Use the half-angle formulas to simplify the expression.
A)|sin 5x|
B)- |sin x|
C)|sin 10x|
D)- |sin 5x|
E)|sin x|
A)|sin 5x|
B)- |sin x|
C)|sin 10x|
D)- |sin 5x|
E)|sin x|

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 50 في هذه المجموعة.
فتح الحزمة
k this deck
11
Use the figure to find the exact value of the trigonometric function.
Cot 2θ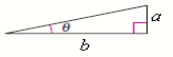
A = 1,b = 6
A)
B)
C)
D)
E)
Cot 2θ

A = 1,b = 6
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 50 في هذه المجموعة.
فتح الحزمة
k this deck
12
Use the figure to find the exact value of the trigonometric function.
Tan 2θ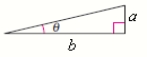
A = 1,b = 6
A)
B)
C)
D)
E)
Tan 2θ

A = 1,b = 6
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 50 في هذه المجموعة.
فتح الحزمة
k this deck
13
Use the half-angle formulas to simplify the expression.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 50 في هذه المجموعة.
فتح الحزمة
k this deck
14
Use the figure to find the exact value of the trigonometric function.
Cos 2θ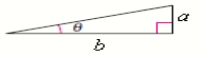
A = 1,b = 2
A)
B)
C)
D)
E)
Cos 2θ

A = 1,b = 2
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 50 في هذه المجموعة.
فتح الحزمة
k this deck
15
Use a double-angle formula to rewrite the expression.
A)sin x
B)2 sin x
C)2 sin 2x
D) sin x
E) sin 2x
A)sin x
B)2 sin x
C)2 sin 2x
D) sin x
E) sin 2x

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 50 في هذه المجموعة.
فتح الحزمة
k this deck
16
Use the figure to find the exact value of the trigonometric function.
Sec 2θ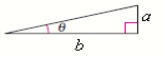
A = 1,b = 8
A)
B)
C)
D)
E)
Sec 2θ

A = 1,b = 8
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 50 في هذه المجموعة.
فتح الحزمة
k this deck
17
Use the half-angle formulas to simplify the expression.
A)- |cos x|
B)|cos x|
C)|cos 4x|
D)- |cos 4x|
E)- |cos 8x|
A)- |cos x|
B)|cos x|
C)|cos 4x|
D)- |cos 4x|
E)- |cos 8x|

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 50 في هذه المجموعة.
فتح الحزمة
k this deck
18
Use the half-angle formulas to simplify the expression.
A)- |cot x|
B)- |3 tan x|
C)- |3 tan 6x|
D)- |3 cot 3x|
E)- |cot 3x|
A)- |cot x|
B)- |3 tan x|
C)- |3 tan 6x|
D)- |3 cot 3x|
E)- |cot 3x|

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 50 في هذه المجموعة.
فتح الحزمة
k this deck
19
Use a double-angle formula to rewrite the expression.
2 sin2 x - 1
A)cos x
B)cos 2x
C)-2 cos x
D)2 cos 2x
E)- cos 2x
2 sin2 x - 1
A)cos x
B)cos 2x
C)-2 cos x
D)2 cos 2x
E)- cos 2x

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 50 في هذه المجموعة.
فتح الحزمة
k this deck
20
Use the figure to find the exact value of the trigonometric function.
Sin 2θ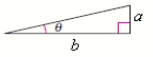
A = 1,b = 2
A)
B)
C)
Sin 2θ

A = 1,b = 2
A)
B)
C)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 50 في هذه المجموعة.
فتح الحزمة
k this deck
21
Use the figure below to determine the exact value of the given function. 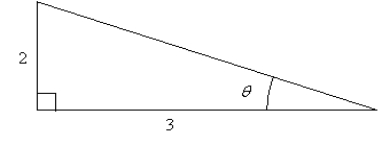
A)
B)
C) .
D)
E)

A)
B)
C) .
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 50 في هذه المجموعة.
فتح الحزمة
k this deck
22
Use a double angle formula to rewrite the given expression.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 50 في هذه المجموعة.
فتح الحزمة
k this deck
23
Convert the expression.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 50 في هذه المجموعة.
فتح الحزمة
k this deck
24
Find the exact solutions of the given equation in the interval [0,2π).
A)
B)
C)
D)x = 0
E)
A)
B)
C)
D)x = 0
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 50 في هذه المجموعة.
فتح الحزمة
k this deck
25
Convert the expression.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 50 في هذه المجموعة.
فتح الحزمة
k this deck
26
The mach number M of an airplane is the ratio of its speed to the speed of sound.When an airplane travels faster than the speed of sound,the sound waves form a cone behind the airplane (see figure).The mach number is related to the apex angle θ of the cone by . 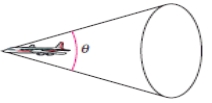 Rewrite the equation in terms of θ.
Rewrite the equation in terms of θ.
A)
B)
C)
D)
E)
 Rewrite the equation in terms of θ.
Rewrite the equation in terms of θ.
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 50 في هذه المجموعة.
فتح الحزمة
k this deck
27
Use the sum-to-product formulas to rewrite the sum or difference as a product.
Cos 3θ + cos 8θ
A)
B)
C)
D)
E)
Cos 3θ + cos 8θ
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 50 في هذه المجموعة.
فتح الحزمة
k this deck
28
Use the figure to find the exact value of the trigonometric function. 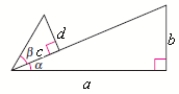 sin 2α
sin 2α
A)
B)
C)
D)
E)
 sin 2α
sin 2α
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 50 في هذه المجموعة.
فتح الحزمة
k this deck
29
Convert the expression.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 50 في هذه المجموعة.
فتح الحزمة
k this deck
30
Convert the expression.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 50 في هذه المجموعة.
فتح الحزمة
k this deck
31
Convert the expression.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 50 في هذه المجموعة.
فتح الحزمة
k this deck
32
Use the sum-to-product formulas to rewrite the sum or difference as a product.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 50 في هذه المجموعة.
فتح الحزمة
k this deck
33
Convert the expression.
A)2 cos b
B)cos 2b
C)cos b
D)2 cos 2b
E)4 cos b
A)2 cos b
B)cos 2b
C)cos b
D)2 cos 2b
E)4 cos b

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 50 في هذه المجموعة.
فتح الحزمة
k this deck
34
When two railroad tracks merge,the overlapping portions of the tracks are in the shapes of circular arcs (see figure).The radius of each arc r (in feet)and the angle θ are related by Write a formula for x in terms of cos θ. 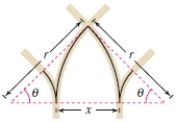
A)
B)
C)
D)
E)

A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 50 في هذه المجموعة.
فتح الحزمة
k this deck
35
Convert the expression.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 50 في هذه المجموعة.
فتح الحزمة
k this deck
36
Use a double-angle formula to find the exact value of cos2u when
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 50 في هذه المجموعة.
فتح الحزمة
k this deck
37
Use the figure to find the exact value of the trigonometric function. 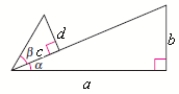 cos 2β
cos 2β
A)
B)
C)
D)
E)
 cos 2β
cos 2β
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 50 في هذه المجموعة.
فتح الحزمة
k this deck
38
Convert the expression.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 50 في هذه المجموعة.
فتح الحزمة
k this deck
39
Find the exact solutions of the given equation in the interval [0,2π).
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 50 في هذه المجموعة.
فتح الحزمة
k this deck
40
Use the sum-to-product formulas to select the sum or difference as a product.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 50 في هذه المجموعة.
فتح الحزمة
k this deck
41
Use the half-angle formulas to determine the exact value of the given trigonometric expression.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 50 في هذه المجموعة.
فتح الحزمة
k this deck
42
Use the figure below to find the exact value of the given trigonometric expression.  10 24
10 24
(figure not necessarily to scale)
A)
B)
C)
D)
E)
 10 24
10 24(figure not necessarily to scale)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 50 في هذه المجموعة.
فتح الحزمة
k this deck
43
Use the half-angle formula to simplify the given expression.
A)cos |2x|
B)cos |8x|
C)cos |16x|
D)cos |32x|
E)cos |4x|
A)cos |2x|
B)cos |8x|
C)cos |16x|
D)cos |32x|
E)cos |4x|

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 50 في هذه المجموعة.
فتح الحزمة
k this deck
44
Use the figure below to find the exact value of the given trigonometric expression. 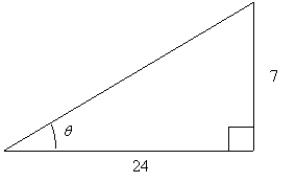
A)
B)
C)
D)
E)

A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 50 في هذه المجموعة.
فتح الحزمة
k this deck
45
The range of a projectile fired at an angle θ with the horizontal and with an initial velocity of v0 feet per second is where r is measured in feet.A golfer strikes a golf ball at 90 feet per second.Ignoring the effects of air resistance,at what angle must the golfer hit the ball so that it travels 150 feet? (Round your answer to the nearest degree. )
A)14°
B)36°
C)18°
D)27°
E)41°
A)14°
B)36°
C)18°
D)27°
E)41°

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 50 في هذه المجموعة.
فتح الحزمة
k this deck
46
Find all solutions of the given equation in the interval [0,2π).
A)
B)
C)
D)
E)x = 0
A)
B)
C)
D)
E)x = 0

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 50 في هذه المجموعة.
فتح الحزمة
k this deck
47
Find all solutions of the given equation in the interval [0,2π).
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 50 في هذه المجموعة.
فتح الحزمة
k this deck
48
Use the product-to-sum formula to write the given product as a sum or difference.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 50 في هذه المجموعة.
فتح الحزمة
k this deck
49
Use the sum-to-product formulas to find the exact value of the given expression.
A)
B)1
C)
D)-1
E)0
A)
B)1
C)
D)-1
E)0

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 50 في هذه المجموعة.
فتح الحزمة
k this deck
50
Use the sum-to-product formulas to write the given expression as a product.
A)2 sin 4θ cos θ
B)-2 sin 4θ sin θ
C)-2 cos 4θ cos θ
D)2 cos 4θ sin θ
E)2 cos 4θ cos θ
A)2 sin 4θ cos θ
B)-2 sin 4θ sin θ
C)-2 cos 4θ cos θ
D)2 cos 4θ sin θ
E)2 cos 4θ cos θ

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 50 في هذه المجموعة.
فتح الحزمة
k this deck








