Deck 10: Additional Topics in Trigonometry
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال

فتح الحزمة
قم بالتسجيل لفتح البطاقات في هذه المجموعة!
Unlock Deck
Unlock Deck
1/25
العب
ملء الشاشة (f)
Deck 10: Additional Topics in Trigonometry
1
The initial point for the vector is the origin, and denotes the angle (measured counterclockwise) from the -axis to the vector. The magnitude of is cm/sec, and Compute the horizontal and vertical components of the given vector. (Round your answers to two decimal places.)
A) cm/sec cm/sec
B) cm/sec cm/sec
C) cm/sec cm/sec
D) cm/sec cm/sec
E)
A) cm/sec cm/sec
B) cm/sec cm/sec
C) cm/sec cm/sec
D) cm/sec cm/sec
E)
cm/sec cm/sec
2
On a sheet of paper, graph the parametric equation after eliminating the parameter ). Specify the approximate direction on the curve corresponding to increasing values of .
A) counterclockwise
B) clockwise
A) counterclockwise
B) clockwise
clockwise
3
Convert the given rectangular coordinates to polar coordinates. Express the answer in such a way that r is nonnegative and .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)
4
Convert the given rectangular coordinates to polar coordinates. Express the answer in such a way that r is nonnegative and .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 25 في هذه المجموعة.
فتح الحزمة
k this deck
5
Two points P and Q are on opposite sides of a river (see the sketch). From P to another point R on the same side is 340 ft. Angles and are found to be and , respectively. Compute the distance from P to Q, across the river. (Round your answer to the nearest foot.) 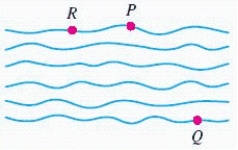
A) ft.
B) ft.
C) ft.
D) ft.
E) ft.

A) ft.
B) ft.
C) ft.
D) ft.
E) ft.

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 25 في هذه المجموعة.
فتح الحزمة
k this deck
6
Determine the graph that reflects the polar equation.
A)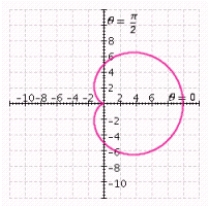
B)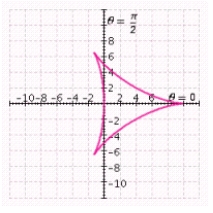
C)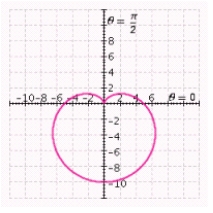
D)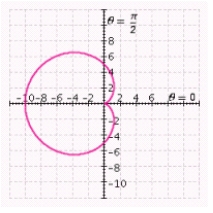
E)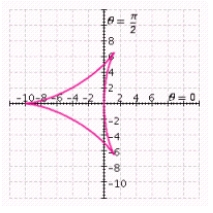
A)

B)

C)

D)

E)


فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 25 في هذه المجموعة.
فتح الحزمة
k this deck
7
An airplane crashes in a lake and is spotted by observers at lighthouses A and B along the coast. Lighthouse B is 1.10 miles due east of lighthouse A. The bearing of the airplane from lighthouse A is ; the bearing of the plane from lighthouse B is . Find the distance from each lighthouse to the crash site.
A) Distance from lighthouse : 0.95 miles, Distance from lighthouse : 1.28 miles
B) Distance from lighthouse : 1.35 miles, Distance from lighthouse : 0.97 miles
C) Distance from lighthouse : 0.92 miles, Distance from lighthouse : 0.76 miles
D) Distance from lighthouse : 1.43 miles, Distance from lighthouse : 0.84 miles
E) Distance from lighthouse : 0.97miles, Distance from lighthouse : 1.19 miles
A) Distance from lighthouse : 0.95 miles, Distance from lighthouse : 1.28 miles
B) Distance from lighthouse : 1.35 miles, Distance from lighthouse : 0.97 miles
C) Distance from lighthouse : 0.92 miles, Distance from lighthouse : 0.76 miles
D) Distance from lighthouse : 1.43 miles, Distance from lighthouse : 0.84 miles
E) Distance from lighthouse : 0.97miles, Distance from lighthouse : 1.19 miles

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 25 في هذه المجموعة.
فتح الحزمة
k this deck
8
Assume that the vectors , , and are defined as follows: Compute .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 25 في هذه المجموعة.
فتح الحزمة
k this deck
9
Convert to rectangular form.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 25 في هذه المجموعة.
فتح الحزمة
k this deck
10
Determine the graph that reflects the polar equation.
A)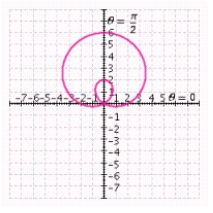
B)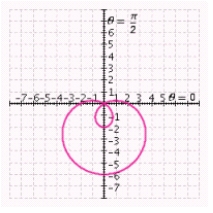
C)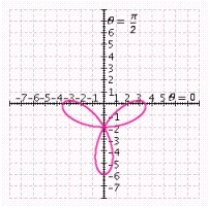
D)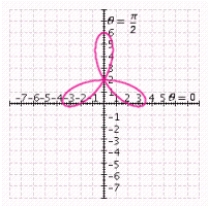
E)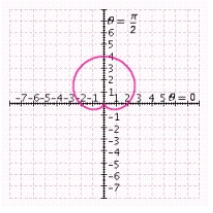
A)

B)

C)

D)

E)


فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 25 في هذه المجموعة.
فتح الحزمة
k this deck
11
Assume that the vectors , , and are defined as follows:
Compute
A)
B)
C)
D)
E)
Compute
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 25 في هذه المجموعة.
فتح الحزمة
k this deck
12
Refer to the figure. If and , find . 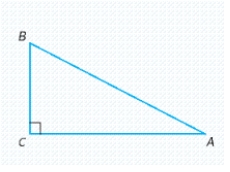
A)
B)
C)
D)
E)

A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 25 في هذه المجموعة.
فتح الحزمة
k this deck
13
Graph the parametric equations using the given range for the parameter . Begin with the standard viewing rectangle and then make adjustments, as necessary, so that the graph utilizes as much of the viewing screen as possible. For example, in graphing the circle given by and it would be natural to choose a viewing rectangle extending from -1 to 1 in both the - and -directions. Graph the parametric equations on a graphing utility. Sketch the result. and , (one-quarter of an ellipse)
A)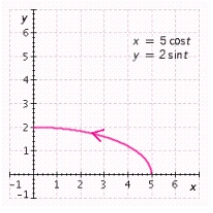
B)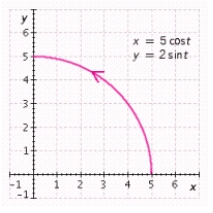
C)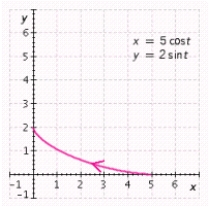
D)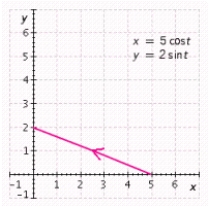
E)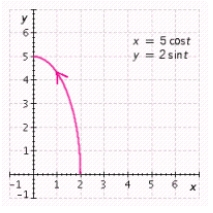
A)

B)

C)

D)

E)


فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 25 في هذه المجموعة.
فتح الحزمة
k this deck
14
Assume that the coordinates of the points and are as follows: Draw the vector (using graph paper) and compute its magnitude.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 25 في هذه المجموعة.
فتح الحزمة
k this deck
15
Use the given information to find the cosines of angles in . cm, cm, cm
A) , ,
B)
C) , ,
D) , ,
E) , ,
A) , ,
B)
C) , ,
D) , ,
E) , ,

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 25 في هذه المجموعة.
فتح الحزمة
k this deck
16
Use the equation to determine polar coordinates of the point B. 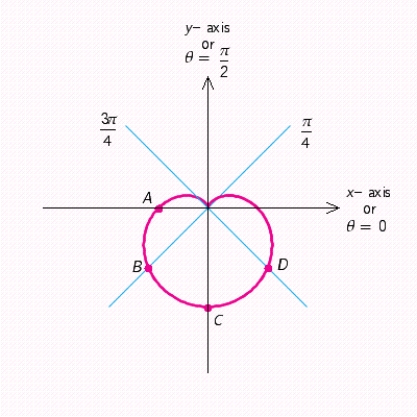
A)
B)
C)
D)
E)

A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 25 في هذه المجموعة.
فتح الحزمة
k this deck
17
On a sheet of paper, graph the parametric equation after eliminating the parameter ( ). Specify the approximate direction on the curve corresponding to increasing values of .
A) clockwise
B) counterclockwise
A) clockwise
B) counterclockwise

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 25 في هذه المجموعة.
فتح الحزمة
k this deck
18
Determine the graph that reflects the polar equation. (five-leafed rose)
A)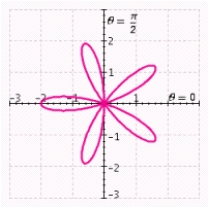
B)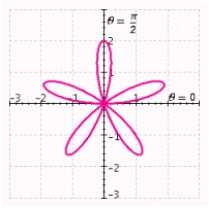
C)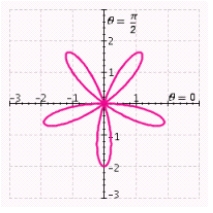
D)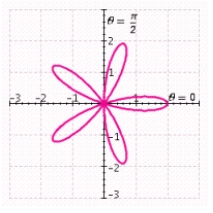
E)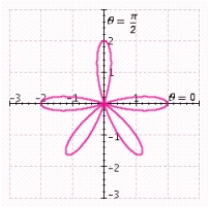
A)

B)

C)

D)

E)


فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 25 في هذه المجموعة.
فتح الحزمة
k this deck
19
Convert to rectangular form.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 25 في هذه المجموعة.
فتح الحزمة
k this deck
20
The accompanying figure shows two ships at points and , which are in the same vertical plane as an airplane at point . When the height of the airplane is 4,000 ft, the angle of depression to is 38° and that to is 15°. Find the distance between the two ships. 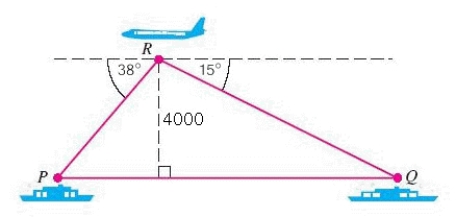
A) 54,900 ft
B) 2,050 ft
C) 20,050 ft
D) 80,430 ft
E) 4,200 ft

A) 54,900 ft
B) 2,050 ft
C) 20,050 ft
D) 80,430 ft
E) 4,200 ft

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 25 في هذه المجموعة.
فتح الحزمة
k this deck
21
Convert from rectangular to trigonometric form. (Choose an argument such that .)
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 25 في هذه المجموعة.
فتح الحزمة
k this deck
22
Determine the graph of the equation.
A)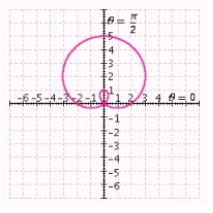
B)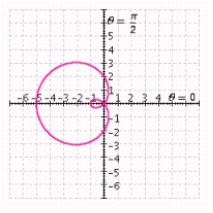
C)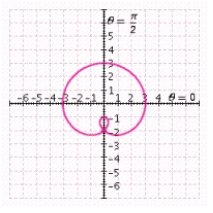
D)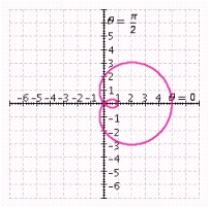
E)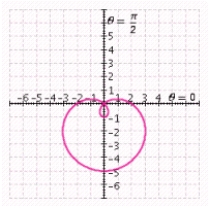
A)

B)

C)

D)

E)


فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 25 في هذه المجموعة.
فتح الحزمة
k this deck
23
Convert from rectangular to trigonometric form. (Choose an argument such that .) 6
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 25 في هذه المجموعة.
فتح الحزمة
k this deck
24
Carry out the indicated operations.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 25 في هذه المجموعة.
فتح الحزمة
k this deck
25
Simplify.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 25 في هذه المجموعة.
فتح الحزمة
k this deck








