Deck 6: An Introduction to Trigonometry Via Right Triangles
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال

فتح الحزمة
قم بالتسجيل لفتح البطاقات في هذه المجموعة!
Unlock Deck
Unlock Deck
1/25
العب
ملء الشاشة (f)
Deck 6: An Introduction to Trigonometry Via Right Triangles
1
From a point on ground level, you measure the angle of elevation to the top of a mountain to be . Then you walk farther away from the mountain and find that the angle of elevation is now . Find the height of the mountain.
A) m
B) m
C) m
D) m
E) m
A) m
B) m
C) m
D) m
E) m
m
2
In , you are given and . If is a point on and , find .
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)
3
The radius of the circle in the figure is 2 units. Express the length in terms of . 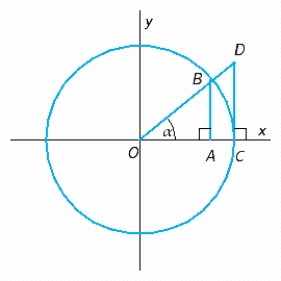
A)
B)
C)
D)
E)

A)
B)
C)
D)
E)
4
Find the area of the triangle. Use a calculator and round your final answer to two decimal places. 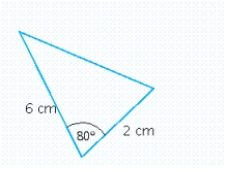
A)
B)
C)
D)
E)

A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 25 في هذه المجموعة.
فتح الحزمة
k this deck
5
Refer to the figure. If and , find . 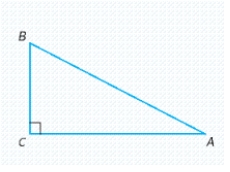
A) cm
B) cm
C) cm
D) cm
E) cm

A) cm
B) cm
C) cm
D) cm
E) cm

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 25 في هذه المجموعة.
فتح الحزمة
k this deck
6
Use the definitions to evaluate the six trigonometric functions of . In cases in which a radical occurs in a denominator, rationalize the denominator. 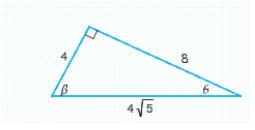
A)
B)
C)
D)
E)

A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 25 في هذه المجموعة.
فتح الحزمة
k this deck
7
Evaluate the expression using the concept of a reference angle.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 25 في هذه المجموعة.
فتح الحزمة
k this deck
8
Suppose that is a right triangle with . If and , find the quantities.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 25 في هذه المجموعة.
فتح الحزمة
k this deck
9
Use the following information to express the remaining five trigonometric values as functions of . Assume that is positive. Rationalize any denominators that contain radicals.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 25 في هذه المجموعة.
فتح الحزمة
k this deck
10
Use the definitions (not a calculator) to evaluate the six trigonometric functions of the angle.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 25 في هذه المجموعة.
فتح الحزمة
k this deck
11
Determine whether the equation is correct by evaluating each side. Do not use a calculator. Note: Notation such as stands for .

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 25 في هذه المجموعة.
فتح الحزمة
k this deck
12
An observer in a lighthouse is above the surface of the water. The observer sees a ship and finds the angle of depression to be . Estimate the distance of the ship from the base of the lighthouse.
A) ft
B) ft
C) ft
D) ft
E) ft
A) ft
B) ft
C) ft
D) ft
E) ft

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 25 في هذه المجموعة.
فتح الحزمة
k this deck
13
Determine whether the equation is correct by evaluating each side. Do not use a calculator.

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 25 في هذه المجموعة.
فتح الحزمة
k this deck
14
Evaluate the expression using the concept of a reference angle.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 25 في هذه المجموعة.
فتح الحزمة
k this deck
15
Use the following information to determine the remaining five trigonometric values. Rationalize any denominators that contain radicals.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 25 في هذه المجموعة.
فتح الحزمة
k this deck
16
Use the definitions (not a calculator) to evaluate the six trigonometric functions of the angle.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 25 في هذه المجموعة.
فتح الحزمة
k this deck
17
The accompanying figure shows two ships at points and , which are in the same vertical plane as an airplane at point . When the height of the airplane is , the angle of depression to is and that to is .Find the distance between the two ships. 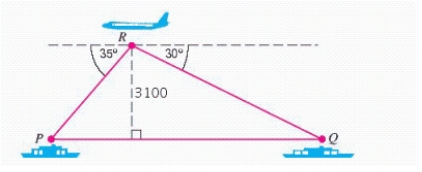
A) ft
B) ft
C) ft
D) ft
E) ft

A) ft
B) ft
C) ft
D) ft
E) ft

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 25 في هذه المجموعة.
فتح الحزمة
k this deck
18
Use the following formation to determine the remaining five trigonometric values. Rationalize any denominators that contain radicals.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 25 في هذه المجموعة.
فتح الحزمة
k this deck
19
Evaluate the expression using the concept of a reference angle.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 25 في هذه المجموعة.
فتح الحزمة
k this deck
20
Use the definitions (not a calculator) to evaluate the six trigonometric functions of the angle.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 25 في هذه المجموعة.
فتح الحزمة
k this deck
21
Determine the answer that establishes an identity.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 25 في هذه المجموعة.
فتح الحزمة
k this deck
22
Determine the answer that establishes an identity.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 25 في هذه المجموعة.
فتح الحزمة
k this deck
23
Use the following information to express the remaining five trigonometric values as functions of . Assume that is positive. Rationalize any denominators that contain radicals.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 25 في هذه المجموعة.
فتح الحزمة
k this deck
24
Use the following information to express the remaining five trigonometric values as functions of . Assume that is positive. Rationalize any denominators that contain radicals.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 25 في هذه المجموعة.
فتح الحزمة
k this deck
25
Determine the answer that establishes an identity.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 25 في هذه المجموعة.
فتح الحزمة
k this deck








