Deck 13: Introduction to Multiple Regression

Question 1

Instruction 13.3
An economist is interested to see how consumption for an economy (in $ billions) is influenced by gross domestic product ($ billions) and aggregate price (consumer price index). The Microsoft Excel output of this regression is partially reproduced below.
OUTPUT
SUMMARY
Regression Statistics
$\begin{array} { l l } \text { MultipleR } & 0.991 \\ \text { R Square } & 0.982 \\ \text { Adj. R Square } & 0.976 \\ \text { Std. Error } & 0.299 \\ \text { Observations } & 10 \end{array}$
ANOVA
$\begin{array} { l l l l l l } & d f & \text { SS } & \text { MS } & F & \text { Signif F } \\ \text { Regression } & 2 & 33.4163 & 16.7082 & 186.325 & 0.0001 \\ \text { Residual } & 7 & 0.6277 & 0.0897 & & \\ \text { Total } & 9 & 34.0440 & & & \\ & & & & & \\ & \text { Coeff } & \text { StdError } & t \text { Stat } & p \text { value } & \\ \text { Intercept } & - 1.6335 & 0.5674 & - 0.152 & 0.8837 & \\ \text { GDP } & 0.7654 & 0.0574 & 13.340 & 0.0001 & \\ \text { Price } & - 0.0006 & 0.0028 & - 0.219 & 0.8330 & \end{array}$ Note: Adj. R Square = Adjusted R Square; Std. Error = Standard Error

-Referring to Instruction 13.3,what is the predicted consumption level for an economy with GDP equal to $4 billion and an aggregate price index of 150?

A) $1.39 billion.
B) $2.89 billion.
C) $4.75 billion.
D) $9.45 billion.

Answer 1

$2.89 billion.

Question 2

Instruction 13.4
A real estate builder wishes to determine how house size (House) is influenced by family income (Income), family size (Size) and education of the head of household (School). House size is measured in hundreds of square metres, income is measured in thousands of dollars and education is in years. The builder randomly selected 50 families and ran the multiple regression. Microsoft Excel output is provided below:
OUTPUT
SUMMARY
Regression Statistics
$\begin{array} { l l } \text { Multiple R } & 0.865 \\ \text { R Square } & 0.748 \\ \text { Adj. R Square } & 0.726 \\ \text { Std. Error } & 5.195 \\ \text { Observations } & 50 \end{array}$
ANOVA
$\begin{array} { l l l l l l } & d f & \text { SS } & \text { MS } & F & \text { Signif F} \\ \text { Regression } & & 3605.7736 & 901.4434 & & 0.0001 \\ \text { Residual } & & 1214.2264 & 26.9828 & & \\ \text { Total } & 49 & 4820.0000 & & & \\ & & & & & \\ & \text { Coeff } & \text { StdError } & t \text { Stat } & p \text { value } & \\ \text { Intercept } & - 1.6335 & 5.8078 & - 0.281 & 0.7798 & \\ \text { Income } & 0.4485 & 0.1137 & 3.9545 & 0.0003 & \\ \text { Size } & 4.2615 & 0.8062 & 5.286 & 0.0001 & \\ \text { School } & - 0.6517 & 0.4319 & - 1.509 & 0.1383 & \end{array}$ Note: Adj. R Square = Adjusted R Square; Std. Error = Standard Error

-Referring to Instruction 13.4,what minimum annual income would an individual with a family size of 9 and 10 years of education need to attain a predicted 5,000 square metre home (House = 50)?

A) $56.75 thousand.
B) $211.85 thousand.
C) $178.33 thousand.
D) $44.14 thousand.

Answer 2

$44.14 thousand.

Question 3

Instruction 13.2
A lecturer in industrial relations believes that an individual's wage rate at a factory (Y) depends on his performance rating (X₁) and the number of economics courses the employee successfully completed at university (X₂). The lecturer randomly selects six workers and collects the following information:
$$\begin{array} { | l | l | l | l | } \hline \text { Employee } & Y ( \$ ) & X 1 & X 2 \\\hline 1 & 10 & 3 & 0 \\\hline 2 & 12 & 1 & 5 \\\hline 3 & 15 & 8 & 1 \\\hline 4 & 17 & 5 & 8 \\\hline 5 & 20 & 7 & 12 \\\hline 6 & 25 & 10 & 9 \\\hline\end{array}$$

-Referring to Instruction 13.2,for these data,what is the estimated coefficient for performance rating,b₁?

A) 9.103
B) 0.616
C) 6.932
D) 1.054

Answer 3

1.054

Question 4

Instruction 13.4
A real estate builder wishes to determine how house size (House) is influenced by family income (Income), family size (Size) and education of the head of household (School). House size is measured in hundreds of square metres, income is measured in thousands of dollars and education is in years. The builder randomly selected 50 families and ran the multiple regression. Microsoft Excel output is provided below:
OUTPUT
SUMMARY
Regression Statistics
$\begin{array} { l l } \text { Multiple R } & 0.865 \\ \text { R Square } & 0.748 \\ \text { Adj. R Square } & 0.726 \\ \text { Std. Error } & 5.195 \\ \text { Observations } & 50 \end{array}$
ANOVA
$\begin{array} { l l l l l l } & d f & \text { SS } & \text { MS } & F & \text { Signif F} \\ \text { Regression } & & 3605.7736 & 901.4434 & & 0.0001 \\ \text { Residual } & & 1214.2264 & 26.9828 & & \\ \text { Total } & 49 & 4820.0000 & & & \\ & & & & & \\ & \text { Coeff } & \text { StdError } & t \text { Stat } & p \text { value } & \\ \text { Intercept } & - 1.6335 & 5.8078 & - 0.281 & 0.7798 & \\ \text { Income } & 0.4485 & 0.1137 & 3.9545 & 0.0003 & \\ \text { Size } & 4.2615 & 0.8062 & 5.286 & 0.0001 & \\ \text { School } & - 0.6517 & 0.4319 & - 1.509 & 0.1383 & \end{array}$ Note: Adj. R Square = Adjusted R Square; Std. Error = Standard Error

-Referring to Instruction 13.4,what minimum annual income would an individual with a family size of 4 and 16 years of education need to attain a predicted 10,000 square metre home (House = 100)?

A) $178.33 thousand.
B) $211.85 thousand.
C) $44.14 thousand.
D) $56.75 thousand.

Question 5

Instruction 13.1
A manager of a product sales group believes the number of sales made by an employee (Y) depends on how many years that employee has been with the company (X₁) and how he/she scored on a business aptitude test (X₂). A random sample of 8 employees provides the following:
$$\begin{array} { | l | l | l | l | } \hline \text { Employee } & Y & X 1 & X 2 \\\hline 1 & 100 & 10 & 7 \\\hline 2 & 90 & 3 & 10 \\\hline 3 & 80 & 8 & 9 \\\hline 4 & 70 & 5 & 4 \\\hline 5 & 60 & 5 & 8 \\\hline 6 & 50 & 7 & 5 \\\hline 7 & 40 & 1 & 4 \\\hline 8 & 30 & 1 & 1 \\\hline\end{array}$$

-Referring to Instruction 13.1,for these data,what is the value for the regression constant,b₀?

A) 0.998
B) 21.293
C) 3.103
D) 4.698

Question 6

Instruction 13.1
A manager of a product sales group believes the number of sales made by an employee (Y) depends on how many years that employee has been with the company (X₁) and how he/she scored on a business aptitude test (X₂). A random sample of 8 employees provides the following:
$$\begin{array} { | l | l | l | l | } \hline \text { Employee } & Y & X 1 & X 2 \\\hline 1 & 100 & 10 & 7 \\\hline 2 & 90 & 3 & 10 \\\hline 3 & 80 & 8 & 9 \\\hline 4 & 70 & 5 & 4 \\\hline 5 & 60 & 5 & 8 \\\hline 6 & 50 & 7 & 5 \\\hline 7 & 40 & 1 & 4 \\\hline 8 & 30 & 1 & 1 \\\hline\end{array}$$

-Referring to Instruction 13.1,for these data,what is the estimated coefficient for the variable representing years an employee has been with the company,b₁?

A) 3.103
B) 4.698
C) 21.293
D) 0.998

Question 7

Instruction 13.1
A manager of a product sales group believes the number of sales made by an employee (Y) depends on how many years that employee has been with the company (X₁) and how he/she scored on a business aptitude test (X₂). A random sample of 8 employees provides the following:
$$\begin{array} { | l | l | l | l | } \hline \text { Employee } & Y & X 1 & X 2 \\\hline 1 & 100 & 10 & 7 \\\hline 2 & 90 & 3 & 10 \\\hline 3 & 80 & 8 & 9 \\\hline 4 & 70 & 5 & 4 \\\hline 5 & 60 & 5 & 8 \\\hline 6 & 50 & 7 & 5 \\\hline 7 & 40 & 1 & 4 \\\hline 8 & 30 & 1 & 1 \\\hline\end{array}$$

-Referring to Instruction 13.1,for these data,what is the estimated coefficient for the variable representing scores on the aptitude test,b₂?

A) 21.293
B) 0.998
C) 3.103
D) 4.698

Question 8

Multiple regression is the process of using several independent variables to predict a number of dependent variables.

Question 9

A multiple regression is called 'multiple' because it has several data points.

Question 10

Instruction 13.3
An economist is interested to see how consumption for an economy (in $ billions) is influenced by gross domestic product ($ billions) and aggregate price (consumer price index). The Microsoft Excel output of this regression is partially reproduced below.
OUTPUT
SUMMARY
Regression Statistics
$\begin{array} { l l } \text { MultipleR } & 0.991 \\ \text { R Square } & 0.982 \\ \text { Adj. R Square } & 0.976 \\ \text { Std. Error } & 0.299 \\ \text { Observations } & 10 \end{array}$
ANOVA
$\begin{array} { l l l l l l } & d f & \text { SS } & \text { MS } & F & \text { Signif F } \\ \text { Regression } & 2 & 33.4163 & 16.7082 & 186.325 & 0.0001 \\ \text { Residual } & 7 & 0.6277 & 0.0897 & & \\ \text { Total } & 9 & 34.0440 & & & \\ & & & & & \\ & \text { Coeff } & \text { StdError } & t \text { Stat } & p \text { value } & \\ \text { Intercept } & - 1.6335 & 0.5674 & - 0.152 & 0.8837 & \\ \text { GDP } & 0.7654 & 0.0574 & 13.340 & 0.0001 & \\ \text { Price } & - 0.0006 & 0.0028 & - 0.219 & 0.8330 & \end{array}$ Note: Adj. R Square = Adjusted R Square; Std. Error = Standard Error

-Referring to Instruction 13.3,what is the estimated average consumption level for an economy with GDP equal to $4 billion and an aggregate price index of 150?

A) $1.39 billion.
B) $2.89 billion.
C) $4.75 billion.
D) $9.45 billion.

Question 11

Instruction 13.2
A lecturer in industrial relations believes that an individual's wage rate at a factory (Y) depends on his performance rating (X₁) and the number of economics courses the employee successfully completed at university (X₂). The lecturer randomly selects six workers and collects the following information:
$$\begin{array} { | l | l | l | l | } \hline \text { Employee } & Y ( \$ ) & X 1 & X 2 \\\hline 1 & 10 & 3 & 0 \\\hline 2 & 12 & 1 & 5 \\\hline 3 & 15 & 8 & 1 \\\hline 4 & 17 & 5 & 8 \\\hline 5 & 20 & 7 & 12 \\\hline 6 & 25 & 10 & 9 \\\hline\end{array}$$

-Referring to Instruction 13.2,suppose an employee had never taken an economics course and managed to score a 5 on his performance rating.What is his estimated expected wage rate?

A) $12.20
B) $25.11
C) $10.90
D) $17.23

Question 12

Instruction 13.3
An economist is interested to see how consumption for an economy (in $ billions) is influenced by gross domestic product ($ billions) and aggregate price (consumer price index). The Microsoft Excel output of this regression is partially reproduced below.
OUTPUT
SUMMARY
Regression Statistics
$\begin{array} { l l } \text { MultipleR } & 0.991 \\ \text { R Square } & 0.982 \\ \text { Adj. R Square } & 0.976 \\ \text { Std. Error } & 0.299 \\ \text { Observations } & 10 \end{array}$
ANOVA
$\begin{array} { l l l l l l } & d f & \text { SS } & \text { MS } & F & \text { Signif F } \\ \text { Regression } & 2 & 33.4163 & 16.7082 & 186.325 & 0.0001 \\ \text { Residual } & 7 & 0.6277 & 0.0897 & & \\ \text { Total } & 9 & 34.0440 & & & \\ & & & & & \\ & \text { Coeff } & \text { StdError } & t \text { Stat } & p \text { value } & \\ \text { Intercept } & - 1.6335 & 0.5674 & - 0.152 & 0.8837 & \\ \text { GDP } & 0.7654 & 0.0574 & 13.340 & 0.0001 & \\ \text { Price } & - 0.0006 & 0.0028 & - 0.219 & 0.8330 & \end{array}$ Note: Adj. R Square = Adjusted R Square; Std. Error = Standard Error

-Referring to Instruction 13.3,the p-value for the regression model as a whole is

A) 0.01.
B) 0.001.
C) 0.05.
D) None of the above.

Question 13

The interpretation of the slope is different in a multiple linear regression model as compared to a simple linear regression model.

Question 14

Instruction 13.2
A lecturer in industrial relations believes that an individual's wage rate at a factory (Y) depends on his performance rating (X₁) and the number of economics courses the employee successfully completed at university (X₂). The lecturer randomly selects six workers and collects the following information:
$$\begin{array} { | l | l | l | l | } \hline \text { Employee } & Y ( \$ ) & X 1 & X 2 \\\hline 1 & 10 & 3 & 0 \\\hline 2 & 12 & 1 & 5 \\\hline 3 & 15 & 8 & 1 \\\hline 4 & 17 & 5 & 8 \\\hline 5 & 20 & 7 & 12 \\\hline 6 & 25 & 10 & 9 \\\hline\end{array}$$

-Referring to Instruction 13.2,for these data,what is the value for the regression constant,b₀?

A) 9.103
B) 6.932
C) 1.054
D) 0.616

Question 15

Instruction 13.1
A manager of a product sales group believes the number of sales made by an employee (Y) depends on how many years that employee has been with the company (X₁) and how he/she scored on a business aptitude test (X₂). A random sample of 8 employees provides the following:
$$\begin{array} { | l | l | l | l | } \hline \text { Employee } & Y & X 1 & X 2 \\\hline 1 & 100 & 10 & 7 \\\hline 2 & 90 & 3 & 10 \\\hline 3 & 80 & 8 & 9 \\\hline 4 & 70 & 5 & 4 \\\hline 5 & 60 & 5 & 8 \\\hline 6 & 50 & 7 & 5 \\\hline 7 & 40 & 1 & 4 \\\hline 8 & 30 & 1 & 1 \\\hline\end{array}$$

-Referring to Instruction 13.1,if an employee who had been with the company for five years scored a 9 on the aptitude test,what would his estimated expected sales be?

A) 60.88
B) 17.98
C) 55.62
D) 79.09

Question 16

A multiple regression is called 'multiple' because it has several explanatory variables.

Question 17

Instruction 13.2
A lecturer in industrial relations believes that an individual's wage rate at a factory (Y) depends on his performance rating (X₁) and the number of economics courses the employee successfully completed at university (X₂). The lecturer randomly selects six workers and collects the following information:
$$\begin{array} { | l | l | l | l | } \hline \text { Employee } & Y ( \$ ) & X 1 & X 2 \\\hline 1 & 10 & 3 & 0 \\\hline 2 & 12 & 1 & 5 \\\hline 3 & 15 & 8 & 1 \\\hline 4 & 17 & 5 & 8 \\\hline 5 & 20 & 7 & 12 \\\hline 6 & 25 & 10 & 9 \\\hline\end{array}$$

-Referring to Instruction 13.2,an employee who took 12 economics courses scores 10 on the performance rating.What is her estimated expected wage rate?

A) $25.70
B) $10.90
C) $24.87
D) $12.20

Question 18

Instruction 13.2
A lecturer in industrial relations believes that an individual's wage rate at a factory (Y) depends on his performance rating (X₁) and the number of economics courses the employee successfully completed at university (X₂). The lecturer randomly selects six workers and collects the following information:
$$\begin{array} { | l | l | l | l | } \hline \text { Employee } & Y ( \$ ) & X 1 & X 2 \\\hline 1 & 10 & 3 & 0 \\\hline 2 & 12 & 1 & 5 \\\hline 3 & 15 & 8 & 1 \\\hline 4 & 17 & 5 & 8 \\\hline 5 & 20 & 7 & 12 \\\hline 6 & 25 & 10 & 9 \\\hline\end{array}$$

-Referring to Instruction 13.2,for these data,what is the estimated coefficient for the number of economics courses taken,b₂?

A) 6.932
B) 1.054
C) 9.103
D) 0.616

Question 19

In a multiple regression problem involving two independent variables,if b₁ is computed to be +2.0,it means that

A) the estimated mean of Y increases by 2 units for each increase of 1 unit of X₁, without regard to X₂.
B) the estimated mean of Y increases by 2 units for each increase of 1 unit of X₁, holding X₂ constant.
C) the estimated mean of Y is 2 when X₁ equals zero.
D) the relationship between X₁ and Y is significant.

Question 20

Instruction 13.3
An economist is interested to see how consumption for an economy (in $ billions) is influenced by gross domestic product ($ billions) and aggregate price (consumer price index). The Microsoft Excel output of this regression is partially reproduced below.
OUTPUT
SUMMARY
Regression Statistics
$\begin{array} { l l } \text { MultipleR } & 0.991 \\ \text { R Square } & 0.982 \\ \text { Adj. R Square } & 0.976 \\ \text { Std. Error } & 0.299 \\ \text { Observations } & 10 \end{array}$
ANOVA
$\begin{array} { l l l l l l } & d f & \text { SS } & \text { MS } & F & \text { Signif F } \\ \text { Regression } & 2 & 33.4163 & 16.7082 & 186.325 & 0.0001 \\ \text { Residual } & 7 & 0.6277 & 0.0897 & & \\ \text { Total } & 9 & 34.0440 & & & \\ & & & & & \\ & \text { Coeff } & \text { StdError } & t \text { Stat } & p \text { value } & \\ \text { Intercept } & - 1.6335 & 0.5674 & - 0.152 & 0.8837 & \\ \text { GDP } & 0.7654 & 0.0574 & 13.340 & 0.0001 & \\ \text { Price } & - 0.0006 & 0.0028 & - 0.219 & 0.8330 & \end{array}$ Note: Adj. R Square = Adjusted R Square; Std. Error = Standard Error

-Referring to Instruction 13.3,what is the estimated average consumption level for an economy with GDP equal to $2 billion and an aggregate price index of 90?

A) $1.39 billion.
B) $2.89 billion.
C) $4.75 billion.
D) $9.45 billion.

Question 21

AU: Question 37 is the same as Question 36. Please check.
Instruction 13.12
AU: Please advise if Instruction 13.12 can be renumbered to Instruction 13.11 and further questions renumbered. Or advise whether there shall be new Instruction 13.11 included.
The Head of the Accounting Department wanted to see if she could predict the average grade of students using the number of course units (credits) and total university entrance exam scores of each. She takes a sample of students and generates the following Microsoft Excel output:
OUTPUT
SUMMARY
$\begin{array} { l l } \text { Regression Statistics } & \\ \text { MultipleR } & 0.916 \\ \text { R Square } & 0.839 \\ \text { Adj. R Square } & 0.732 \\ \text { Std. Error } & 0.24685 \\ \text { Observations } & 6 \end{array}$

ANOVA
$\begin{array} { l l l l l l } & d f & \text { SS } & \text { MS } & F & \text { Signiff } \\ \text { Regression } & 2 & 0.95219 & 0.47610 & 7.813 & 0.0646 \\ \text { Residual } & 3 & 0.18281 & 0.06094 & & \\ \text { Total } & 5 & 1.13500 & & & \\ & & & & & \\ & \text { Coeff } & \text { StdError } & t \text { Stat } & p \text { value } & \\ \text { Intercept } & 4.593897 & 1.13374542 & 4.052 & 0.0271 & \\ \text { GDP } & - 0.247270 & 0.06268485 & - 3.945 & 0.0290 & \\ \text { Price } & 0.001443 & 0.00101241 & 1.425 & 0.2494 & \end{array}$ Note: Adj. R Square = Adjusted R Square; Std. Error = Standard Error

-Referring to Instruction 13.12,the predicted mean grade for a student carrying 15 course units and who has a total university entrance exam score of 1,100 is ___________.

Question 22

AU: Question 37 is the same as Question 36. Please check.
Instruction 13.12
AU: Please advise if Instruction 13.12 can be renumbered to Instruction 13.11 and further questions renumbered. Or advise whether there shall be new Instruction 13.11 included.
The Head of the Accounting Department wanted to see if she could predict the average grade of students using the number of course units (credits) and total university entrance exam scores of each. She takes a sample of students and generates the following Microsoft Excel output:
OUTPUT
SUMMARY
$\begin{array} { l l } \text { Regression Statistics } & \\ \text { MultipleR } & 0.916 \\ \text { R Square } & 0.839 \\ \text { Adj. R Square } & 0.732 \\ \text { Std. Error } & 0.24685 \\ \text { Observations } & 6 \end{array}$

ANOVA
$\begin{array} { l l l l l l } & d f & \text { SS } & \text { MS } & F & \text { Signiff } \\ \text { Regression } & 2 & 0.95219 & 0.47610 & 7.813 & 0.0646 \\ \text { Residual } & 3 & 0.18281 & 0.06094 & & \\ \text { Total } & 5 & 1.13500 & & & \\ & & & & & \\ & \text { Coeff } & \text { StdError } & t \text { Stat } & p \text { value } & \\ \text { Intercept } & 4.593897 & 1.13374542 & 4.052 & 0.0271 & \\ \text { GDP } & - 0.247270 & 0.06268485 & - 3.945 & 0.0290 & \\ \text { Price } & 0.001443 & 0.00101241 & 1.425 & 0.2494 & \end{array}$ Note: Adj. R Square = Adjusted R Square; Std. Error = Standard Error

-Referring to Instruction 13.12,the net regression coefficient of X₂ is___________.

Question 23

Instruction 13.10
The education department's regional executive officer wanted to predict the percentage of students passing a Grade 6 proficiency test. She obtained the data on percentage of students passing the proficiency test (% Passing), daily average of the percentage of students attending class (% Attendance), average teacher salary in dollars (Salaries) and instructional spending per pupil in dollars (Spending) of 47 schools in the state.
Following is the multiple regression output with Y = % Passing as the dependent variable, X₁ = % Attendance, X₂ = Salaries and X₃ = Spending:
$\begin{array}{|l|r|}\hline\text { Regression } & \text { Statistics } \\\hline \text { Multiple R } & 0.7930 \\\hline \text { R Square } & 0.6288 \\\hline \text { Adj. R Square } & 0.602 \\\hline \text { Standard } & \\\text { Error } & 10.4570 \\\hline \text { Observations } & 47\\\hline\end{array}$

$\begin{array}{|l|r|r|r|r|r|}\hline \text { ANOVA } & & & & \\\hline & \text { d } & \text { SS } & \text { MS } & \text { F } & \text { Signif F } \\\hline \text { Regression } & 3 & 7965.08 & 2655.03 & 24.2802 & 0.0000 \\\hline \text { Residual } & 43 & 4702.02 & 109.35 & & \\\hline \text { Total } & 46 & 12667.11 & & & \\\hline\end{array}$


$\begin{array}{|l|r|r|r|r|r|r|} \hline & \text { Coeff } & \text { StolFrro } & \text { tSta } & \text { p-va/ue } & \text { Lower 95\% } & \text { Upper 95\% } \\\hline \text { Intercept } & -753.4225 & 101.1149 & -7.4511 & 0.0000 & -957.3401 & -549.5050 \\\hline \text { \% Attendance } & 8.5014 & 1.0771 & 7.8929 & 0.0000 & 6.3292 & 10.6735 \\\hline \text { Salary } & 0.000000685 & 0.0006 & 0.0011 & 0.9991 & -0.0013 & 0.0013 \\\hline \text { Spending } & 0.0060 & 0.0046 & 1.2879 & 0.2047 & -0.0034 & 0.0153 \\\hline\end{array}$

-Referring to Instruction 13.10,predict the percentage of students passing the proficiency test for a school which has a daily mean of 95% of students attending class,an average teacher salary of 40,000 dollars and an instructional spending per pupil of 2000 dollars.

Question 24

Instruction 13.7
You worked as an intern at We Always Win Car Insurance Company last summer. You notice that individual car insurance premium depends very much on the age of the individual, the number of traffic tickets received by the individual and the population density of the city in which the individual lives. You performed a regression analysis in Microsoft Excel and obtained the following information:
$\begin{array}{|l|l|l|l|l|l|l|}\hline \text {Regression}&\text {Analysis }\\\hline \text { MultipleR } &\quad & 0.63 \\\hline \text { R Square } && 0.40 \\\hline \text { Adj. R Square } && 0.23 \\\hline \text { Standard } & \\\text { Error } & & 50.00\\\hline \text { Observations } & & 15.00 \\\hline & & & & & \\\hline \text { ANOVA } &\\\hline & \text { df}& \text { SS } & \text { MS } & F & \text { Signif F } \\\hline \text { Regression } & 3 & & 5994.24 & 2.40 & 0.12 \\\hline \text { Residual } & 11 & 27496.82 & & & \\\hline \text { Total } & & 45479.54 & & & \\\hline & & & & & \\\hline& \text { Coeff } & \text { StdError } & \text { t Stat } & \text { p-value } & \text { Lower 99.0\%}&\text { Upper 99.0\% } \\\hline \text { Intercept } & 123.80 & 48.71 & 2.54 & 0.03 & -27.47 & 275.07 \\\hline \text { AGE } & 0.82 & 0.87 & -0.95 & 0.36 & -3.51 & 1.87 \\\hline \text { TICKETS } & 11.25 & 10.66 & 1.99 & 0.07 & -11.86 & 54.37 \\\hline \text { DENSITY } & -3.14 & 6.46 & -0.49 & 0.64 & -23.19 & 16.91\\\hline\end{array}$

-Referring to Instruction 13.7,the proportion of the total variability in insurance premiums that can be explained by AGE,TICKETS and DENSITY is ______.

Question 25

Instruction 13.8
A weight-loss clinic wants to use regression analysis to build a model for weight-loss of a client (measured in kilograms). Two variables thought to effect weight-loss are client's length of time on the weight loss program and time of session. These variables are described below:
Y = Weight-loss (in kilograms)
X₁ = Length of time in weight-loss program (in months)
X₂ = 1 if morning session, 0 if not
X₃ = 1 if afternoon session, 0 if not (Base level = evening session)
Data for 12 clients on a weight-loss program at the clinic were collected and used to fit the interaction model:
Y = ?0 + ?₁X₁ + ?₂X₂ + ?₃X₃ + ?₄X₁X₂ + ?₅X₁X₃ + ?
Partial output from Microsoft Excel follows:
$\begin{array}{ll}\text { Regression}\\\text { Statistics}\\ \text { MultipleR } & 0.73514 \\\text { R Square } & 0.540438 \\\text { Adjusted R Square } & 0.157469 \\\text { Standard Error } & 12.4147 \\\text { Observations } & 12\end{array}$

$\begin{array}{ll}\text { ANOVA }\\ F=5.41118 & \text { Significance } F= \\& 0.040201\end{array}$


$\begin{array}{lllll}&\text { Coeff }&\text { StdError }&\text { t Stat }&\text { p-value }\\\text { Intercept } & 0.089744 & 14.127 & 0.0060 & 0.9951 \\\text { Length }\left(X_{1}\right) & 6.22538 & 2.43473 & 2.54956 & 0.0479 \\\text { Morn Ses }\left(X_{2}\right) & 2.217272 & 22.1416 & 0.100141 & 0.9235 \\\text { Aft Ses }\left(X_{3}\right) & 11.8233 & 3.1545 & 3.558901 & 0.0165 \\\text { Length* Morn Ses } & 0.77058 & 3.562 & 0.216334 & 0.8359 \\\text { Length*Aft Ses } & -0.54147 & 3.35988 & -0.161158 & 0.8773\end{array}$

-Referring to Instruction 13.8,what is the experimental unit for this analysis?

A) A client on a weight-loss program.
B) A month.
C) A morning, afternoon, or evening session.
D) A clinic.

Question 26

Instruction 13.7
You worked as an intern at We Always Win Car Insurance Company last summer. You notice that individual car insurance premium depends very much on the age of the individual, the number of traffic tickets received by the individual and the population density of the city in which the individual lives. You performed a regression analysis in Microsoft Excel and obtained the following information:
$\begin{array}{|l|l|l|l|l|l|l|}\hline \text {Regression}&\text {Analysis }\\\hline \text { MultipleR } &\quad & 0.63 \\\hline \text { R Square } && 0.40 \\\hline \text { Adj. R Square } && 0.23 \\\hline \text { Standard } & \\\text { Error } & & 50.00\\\hline \text { Observations } & & 15.00 \\\hline & & & & & \\\hline \text { ANOVA } &\\\hline & \text { df}& \text { SS } & \text { MS } & F & \text { Signif F } \\\hline \text { Regression } & 3 & & 5994.24 & 2.40 & 0.12 \\\hline \text { Residual } & 11 & 27496.82 & & & \\\hline \text { Total } & & 45479.54 & & & \\\hline & & & & & \\\hline& \text { Coeff } & \text { StdError } & \text { t Stat } & \text { p-value } & \text { Lower 99.0\%}&\text { Upper 99.0\% } \\\hline \text { Intercept } & 123.80 & 48.71 & 2.54 & 0.03 & -27.47 & 275.07 \\\hline \text { AGE } & 0.82 & 0.87 & -0.95 & 0.36 & -3.51 & 1.87 \\\hline \text { TICKETS } & 11.25 & 10.66 & 1.99 & 0.07 & -11.86 & 54.37 \\\hline \text { DENSITY } & -3.14 & 6.46 & -0.49 & 0.64 & -23.19 & 16.91\\\hline\end{array}$

-Referring to Instruction 13.7,to test the significance of the multiple regression model,the value of the test statistic is ___________.

Question 27

Instruction 13.4
A real estate builder wishes to determine how house size (House) is influenced by family income (Income), family size (Size) and education of the head of household (School). House size is measured in hundreds of square metres, income is measured in thousands of dollars and education is in years. The builder randomly selected 50 families and ran the multiple regression. Microsoft Excel output is provided below:
OUTPUT
SUMMARY
Regression Statistics
$\begin{array} { l l } \text { Multiple R } & 0.865 \\ \text { R Square } & 0.748 \\ \text { Adj. R Square } & 0.726 \\ \text { Std. Error } & 5.195 \\ \text { Observations } & 50 \end{array}$
ANOVA
$\begin{array} { l l l l l l } & d f & \text { SS } & \text { MS } & F & \text { Signif F} \\ \text { Regression } & & 3605.7736 & 901.4434 & & 0.0001 \\ \text { Residual } & & 1214.2264 & 26.9828 & & \\ \text { Total } & 49 & 4820.0000 & & & \\ & & & & & \\ & \text { Coeff } & \text { StdError } & t \text { Stat } & p \text { value } & \\ \text { Intercept } & - 1.6335 & 5.8078 & - 0.281 & 0.7798 & \\ \text { Income } & 0.4485 & 0.1137 & 3.9545 & 0.0003 & \\ \text { Size } & 4.2615 & 0.8062 & 5.286 & 0.0001 & \\ \text { School } & - 0.6517 & 0.4319 & - 1.509 & 0.1383 & \end{array}$ Note: Adj. R Square = Adjusted R Square; Std. Error = Standard Error

-Referring to Instruction 13.4,what is the predicted house size (in hundreds of square metres)for an individual earning an annual income of $40,000,having a family size of 4 and going to school a total of 13 years?

A) 15.15
B) 53.87
C) 11.43
D) 24.88

Question 28

AU: Question 37 is the same as Question 36. Please check.
Instruction 13.12
AU: Please advise if Instruction 13.12 can be renumbered to Instruction 13.11 and further questions renumbered. Or advise whether there shall be new Instruction 13.11 included.
The Head of the Accounting Department wanted to see if she could predict the average grade of students using the number of course units (credits) and total university entrance exam scores of each. She takes a sample of students and generates the following Microsoft Excel output:
OUTPUT
SUMMARY
$\begin{array} { l l } \text { Regression Statistics } & \\ \text { MultipleR } & 0.916 \\ \text { R Square } & 0.839 \\ \text { Adj. R Square } & 0.732 \\ \text { Std. Error } & 0.24685 \\ \text { Observations } & 6 \end{array}$

ANOVA
$\begin{array} { l l l l l l } & d f & \text { SS } & \text { MS } & F & \text { Signiff } \\ \text { Regression } & 2 & 0.95219 & 0.47610 & 7.813 & 0.0646 \\ \text { Residual } & 3 & 0.18281 & 0.06094 & & \\ \text { Total } & 5 & 1.13500 & & & \\ & & & & & \\ & \text { Coeff } & \text { StdError } & t \text { Stat } & p \text { value } & \\ \text { Intercept } & 4.593897 & 1.13374542 & 4.052 & 0.0271 & \\ \text { GDP } & - 0.247270 & 0.06268485 & - 3.945 & 0.0290 & \\ \text { Price } & 0.001443 & 0.00101241 & 1.425 & 0.2494 & \end{array}$ Note: Adj. R Square = Adjusted R Square; Std. Error = Standard Error

-Referring to Instruction 13.12,the estimate of the unit change in the mean of Y per unit change in X₁,holding X₂ constant,is___________.

Question 29

Instruction 13.7
You worked as an intern at We Always Win Car Insurance Company last summer. You notice that individual car insurance premium depends very much on the age of the individual, the number of traffic tickets received by the individual and the population density of the city in which the individual lives. You performed a regression analysis in Microsoft Excel and obtained the following information:
$\begin{array}{|l|l|l|l|l|l|l|}\hline \text {Regression}&\text {Analysis }\\\hline \text { MultipleR } &\quad & 0.63 \\\hline \text { R Square } && 0.40 \\\hline \text { Adj. R Square } && 0.23 \\\hline \text { Standard } & \\\text { Error } & & 50.00\\\hline \text { Observations } & & 15.00 \\\hline & & & & & \\\hline \text { ANOVA } &\\\hline & \text { df}& \text { SS } & \text { MS } & F & \text { Signif F } \\\hline \text { Regression } & 3 & & 5994.24 & 2.40 & 0.12 \\\hline \text { Residual } & 11 & 27496.82 & & & \\\hline \text { Total } & & 45479.54 & & & \\\hline & & & & & \\\hline& \text { Coeff } & \text { StdError } & \text { t Stat } & \text { p-value } & \text { Lower 99.0\%}&\text { Upper 99.0\% } \\\hline \text { Intercept } & 123.80 & 48.71 & 2.54 & 0.03 & -27.47 & 275.07 \\\hline \text { AGE } & 0.82 & 0.87 & -0.95 & 0.36 & -3.51 & 1.87 \\\hline \text { TICKETS } & 11.25 & 10.66 & 1.99 & 0.07 & -11.86 & 54.37 \\\hline \text { DENSITY } & -3.14 & 6.46 & -0.49 & 0.64 & -23.19 & 16.91\\\hline\end{array}$

-Referring to Instruction 13.7,the total degrees of freedom that are missing in the ANOVA table should be ___________.

Question 30

Instruction 13.7
You worked as an intern at We Always Win Car Insurance Company last summer. You notice that individual car insurance premium depends very much on the age of the individual, the number of traffic tickets received by the individual and the population density of the city in which the individual lives. You performed a regression analysis in Microsoft Excel and obtained the following information:
$\begin{array}{|l|l|l|l|l|l|l|}\hline \text {Regression}&\text {Analysis }\\\hline \text { MultipleR } &\quad & 0.63 \\\hline \text { R Square } && 0.40 \\\hline \text { Adj. R Square } && 0.23 \\\hline \text { Standard } & \\\text { Error } & & 50.00\\\hline \text { Observations } & & 15.00 \\\hline & & & & & \\\hline \text { ANOVA } &\\\hline & \text { df}& \text { SS } & \text { MS } & F & \text { Signif F } \\\hline \text { Regression } & 3 & & 5994.24 & 2.40 & 0.12 \\\hline \text { Residual } & 11 & 27496.82 & & & \\\hline \text { Total } & & 45479.54 & & & \\\hline & & & & & \\\hline& \text { Coeff } & \text { StdError } & \text { t Stat } & \text { p-value } & \text { Lower 99.0\%}&\text { Upper 99.0\% } \\\hline \text { Intercept } & 123.80 & 48.71 & 2.54 & 0.03 & -27.47 & 275.07 \\\hline \text { AGE } & 0.82 & 0.87 & -0.95 & 0.36 & -3.51 & 1.87 \\\hline \text { TICKETS } & 11.25 & 10.66 & 1.99 & 0.07 & -11.86 & 54.37 \\\hline \text { DENSITY } & -3.14 & 6.46 & -0.49 & 0.64 & -23.19 & 16.91\\\hline\end{array}$

-Referring to Instruction 13.7,the standard error of the estimate is ___________.

Question 31

Instruction 13.5
A microeconomist wants to determine how corporate sales are influenced by capital and wage spending by companies. She proceeds to randomly select 26 large corporations and record information in millions of dollars. The Microsoft Excel output below shows results of this multiple regression.
OUTPUT
SUMMARY
$\begin{array} { l l } \text { Regression Statistics } & \\ \text { Multiple R } & 0.830 \\ \text { R Square } & 0.689 \\ \text { Adj. R Square } & 0.662 \\ \text { Std. Error } & 17501.643 \\ \text { Observations } & 26 \end{array}$

ANOVA
$\begin{array}{llllll} & d f& SS &{ MS } & F & \text{Signif F} \\\text{Regression} & 2 & 15579777040&7789888520 & 25.432 & 0.0001 \\\text{Residual }& 23 & 7045072780&306307512 & & \\\text{Total }& 25 & 22624849820 & &\end{array}$


$\begin{array}{lllll} & \text { Coeff } & \text { StdError } & \text { t Stat } & p \text {-value } \\\text { Intercept } & 15800.0000 & 6038.2999 & 2.617 & 0.0154 \\\text { Capital } & 0.1245 & 0.2045 & 0.609 & 0.5485 \\\text { Wages } & 7.0762 & 1.4729 & 4.804 & 0.0001\end{array}$ Note: Adj. R Square = Adjusted R Square; Std. Error = Standard Error

-Referring to Instruction 13.5,what are the predicted sales (in millions of dollars)for a company spending $100 million on capital and $100 million on wages?

A) 20,455.98
B) 17,277.49
C) 16,520.07
D) 15,800.00

Question 32

Instruction 13.7
You worked as an intern at We Always Win Car Insurance Company last summer. You notice that individual car insurance premium depends very much on the age of the individual, the number of traffic tickets received by the individual and the population density of the city in which the individual lives. You performed a regression analysis in Microsoft Excel and obtained the following information:
$\begin{array}{|l|l|l|l|l|l|l|}\hline \text {Regression}&\text {Analysis }\\\hline \text { MultipleR } &\quad & 0.63 \\\hline \text { R Square } && 0.40 \\\hline \text { Adj. R Square } && 0.23 \\\hline \text { Standard } & \\\text { Error } & & 50.00\\\hline \text { Observations } & & 15.00 \\\hline & & & & & \\\hline \text { ANOVA } &\\\hline & \text { df}& \text { SS } & \text { MS } & F & \text { Signif F } \\\hline \text { Regression } & 3 & & 5994.24 & 2.40 & 0.12 \\\hline \text { Residual } & 11 & 27496.82 & & & \\\hline \text { Total } & & 45479.54 & & & \\\hline & & & & & \\\hline& \text { Coeff } & \text { StdError } & \text { t Stat } & \text { p-value } & \text { Lower 99.0\%}&\text { Upper 99.0\% } \\\hline \text { Intercept } & 123.80 & 48.71 & 2.54 & 0.03 & -27.47 & 275.07 \\\hline \text { AGE } & 0.82 & 0.87 & -0.95 & 0.36 & -3.51 & 1.87 \\\hline \text { TICKETS } & 11.25 & 10.66 & 1.99 & 0.07 & -11.86 & 54.37 \\\hline \text { DENSITY } & -3.14 & 6.46 & -0.49 & 0.64 & -23.19 & 16.91\\\hline\end{array}$

-Referring to Instruction 13.7,the adjusted r² is____________.

Question 33

Instruction 13.5
A microeconomist wants to determine how corporate sales are influenced by capital and wage spending by companies. She proceeds to randomly select 26 large corporations and record information in millions of dollars. The Microsoft Excel output below shows results of this multiple regression.
OUTPUT
SUMMARY
$\begin{array} { l l } \text { Regression Statistics } & \\ \text { Multiple R } & 0.830 \\ \text { R Square } & 0.689 \\ \text { Adj. R Square } & 0.662 \\ \text { Std. Error } & 17501.643 \\ \text { Observations } & 26 \end{array}$

ANOVA
$\begin{array}{llllll} & d f& SS &{ MS } & F & \text{Signif F} \\\text{Regression} & 2 & 15579777040&7789888520 & 25.432 & 0.0001 \\\text{Residual }& 23 & 7045072780&306307512 & & \\\text{Total }& 25 & 22624849820 & &\end{array}$


$\begin{array}{lllll} & \text { Coeff } & \text { StdError } & \text { t Stat } & p \text {-value } \\\text { Intercept } & 15800.0000 & 6038.2999 & 2.617 & 0.0154 \\\text { Capital } & 0.1245 & 0.2045 & 0.609 & 0.5485 \\\text { Wages } & 7.0762 & 1.4729 & 4.804 & 0.0001\end{array}$ Note: Adj. R Square = Adjusted R Square; Std. Error = Standard Error

-Referring to Instruction 13.5,what are the predicted sales (in millions of dollars)for a company spending $500 million on capital and $200 million on wages?

A) 17,277.49
B) 15,800.00
C) 16,520.07
D) 20,455.98

Question 34

Instruction 13.7
You worked as an intern at We Always Win Car Insurance Company last summer. You notice that individual car insurance premium depends very much on the age of the individual, the number of traffic tickets received by the individual and the population density of the city in which the individual lives. You performed a regression analysis in Microsoft Excel and obtained the following information:
$\begin{array}{|l|l|l|l|l|l|l|}\hline \text {Regression}&\text {Analysis }\\\hline \text { MultipleR } &\quad & 0.63 \\\hline \text { R Square } && 0.40 \\\hline \text { Adj. R Square } && 0.23 \\\hline \text { Standard } & \\\text { Error } & & 50.00\\\hline \text { Observations } & & 15.00 \\\hline & & & & & \\\hline \text { ANOVA } &\\\hline & \text { df}& \text { SS } & \text { MS } & F & \text { Signif F } \\\hline \text { Regression } & 3 & & 5994.24 & 2.40 & 0.12 \\\hline \text { Residual } & 11 & 27496.82 & & & \\\hline \text { Total } & & 45479.54 & & & \\\hline & & & & & \\\hline& \text { Coeff } & \text { StdError } & \text { t Stat } & \text { p-value } & \text { Lower 99.0\%}&\text { Upper 99.0\% } \\\hline \text { Intercept } & 123.80 & 48.71 & 2.54 & 0.03 & -27.47 & 275.07 \\\hline \text { AGE } & 0.82 & 0.87 & -0.95 & 0.36 & -3.51 & 1.87 \\\hline \text { TICKETS } & 11.25 & 10.66 & 1.99 & 0.07 & -11.86 & 54.37 \\\hline \text { DENSITY } & -3.14 & 6.46 & -0.49 & 0.64 & -23.19 & 16.91\\\hline\end{array}$

-Referring to Instruction 13.7,the estimated mean change in insurance premiums for every two additional tickets received is ___________.

Question 35

Instruction 13.9
As a project for his business statistics class, a student examined the factors that determined parking meter rates throughout the campus area. Data were collected for the price per hour of parking, number of city blocks to the centre of the university and one of the three jurisdictions: on campus, in the CBD and off campus or outside of the CBD and off campus. The population regression model hypothesised is:
Yi = ? + ?₁x_1i + ?₂x_2i + ?₃x_2i + ?_i
Where
Y is the meter price
x₁ is the number of blocks to the centre of the university
x₂ is a dummy variable that takes the value 1 if the meter is located in the CBD and off campus and the value 0 otherwise
x₃ is a dummy variable that takes the value 1 if the meter is located outside of the CBD and off campus, and the value 0 otherwise
The following Excel results are obtained:
$\begin{array}{|l|r|}\hline\text { Regression } & \text { Statistics } \\\hline \text { Multiple R } & 0.9659 \\\hline \text { R Square } & 0.9331 \\\hline \text { Adj. R Square } & 0.9294 \\\hline \text { Standard } & \\\text { Error } & 0.0327 \\\hline \text { Observations } & 58\\\hline\end{array}$

$\begin{array}{|c|c|c|c|c|c|}\hline\text { ANOVA }\\\hline & d & \text { SS } & \mathrm{MS} & F & \text { Signif } F \\\hline \text { Regression } & 3 & 0.8094 & 0.2698 & 251.1995 & 0.0000 \\\hline \text { Residual } & 54 & 0.058 \mathrm{~d} & 0.0010 & & \\\hline \text { Total } & 57 & 0.8675 & & & \\\hline\end{array}$

$\begin{array}{|l|r|r|r|r|}\hline & \text { Coef } & \text { Stol Error } & \text { t Stat } & p \text {-value } \\\hline \text { Intercept } & 0.5118 & 0.013 & 37.4675 & 2.4904 \\\hline X_{1} & -0.0045 & 0.0034 & -1.3276 & 0.1898 \\\hline X_{2} & -0.2392 & 0.0123 & -19.3942 & 0.0000 \\\hline X_{3} & -0.0002 & 0.0123 & -0.0214 & 0.9829 \\\hline\end{array}$

-Referring to Instruction 13.9,predict the meter rate per hour if one parks outside of the CBD and off campus three blocks from the centre of the university.

A) $0.4981
B) $0.2589
C) $0.0139
D) $0.2604

Question 36

Instruction 13.7
You worked as an intern at We Always Win Car Insurance Company last summer. You notice that individual car insurance premium depends very much on the age of the individual, the number of traffic tickets received by the individual and the population density of the city in which the individual lives. You performed a regression analysis in Microsoft Excel and obtained the following information:
$\begin{array}{|l|l|l|l|l|l|l|}\hline \text {Regression}&\text {Analysis }\\\hline \text { MultipleR } &\quad & 0.63 \\\hline \text { R Square } && 0.40 \\\hline \text { Adj. R Square } && 0.23 \\\hline \text { Standard } & \\\text { Error } & & 50.00\\\hline \text { Observations } & & 15.00 \\\hline & & & & & \\\hline \text { ANOVA } &\\\hline & \text { df}& \text { SS } & \text { MS } & F & \text { Signif F } \\\hline \text { Regression } & 3 & & 5994.24 & 2.40 & 0.12 \\\hline \text { Residual } & 11 & 27496.82 & & & \\\hline \text { Total } & & 45479.54 & & & \\\hline & & & & & \\\hline& \text { Coeff } & \text { StdError } & \text { t Stat } & \text { p-value } & \text { Lower 99.0\%}&\text { Upper 99.0\% } \\\hline \text { Intercept } & 123.80 & 48.71 & 2.54 & 0.03 & -27.47 & 275.07 \\\hline \text { AGE } & 0.82 & 0.87 & -0.95 & 0.36 & -3.51 & 1.87 \\\hline \text { TICKETS } & 11.25 & 10.66 & 1.99 & 0.07 & -11.86 & 54.37 \\\hline \text { DENSITY } & -3.14 & 6.46 & -0.49 & 0.64 & -23.19 & 16.91\\\hline\end{array}$

-Referring to Instruction 13.7,to test the significance of the multiple regression model,the p-value of the test statistic in the sample is___________.

Question 37

Instruction 13.10
The education department's regional executive officer wanted to predict the percentage of students passing a Grade 6 proficiency test. She obtained the data on percentage of students passing the proficiency test (% Passing), daily average of the percentage of students attending class (% Attendance), average teacher salary in dollars (Salaries) and instructional spending per pupil in dollars (Spending) of 47 schools in the state.
Following is the multiple regression output with Y = % Passing as the dependent variable, X₁ = % Attendance, X₂ = Salaries and X₃ = Spending:
$\begin{array}{|l|r|}\hline\text { Regression } & \text { Statistics } \\\hline \text { Multiple R } & 0.7930 \\\hline \text { R Square } & 0.6288 \\\hline \text { Adj. R Square } & 0.602 \\\hline \text { Standard } & \\\text { Error } & 10.4570 \\\hline \text { Observations } & 47\\\hline\end{array}$

$\begin{array}{|l|r|r|r|r|r|}\hline \text { ANOVA } & & & & \\\hline & \text { d } & \text { SS } & \text { MS } & \text { F } & \text { Signif F } \\\hline \text { Regression } & 3 & 7965.08 & 2655.03 & 24.2802 & 0.0000 \\\hline \text { Residual } & 43 & 4702.02 & 109.35 & & \\\hline \text { Total } & 46 & 12667.11 & & & \\\hline\end{array}$


$\begin{array}{|l|r|r|r|r|r|r|} \hline & \text { Coeff } & \text { StolFrro } & \text { tSta } & \text { p-va/ue } & \text { Lower 95\% } & \text { Upper 95\% } \\\hline \text { Intercept } & -753.4225 & 101.1149 & -7.4511 & 0.0000 & -957.3401 & -549.5050 \\\hline \text { \% Attendance } & 8.5014 & 1.0771 & 7.8929 & 0.0000 & 6.3292 & 10.6735 \\\hline \text { Salary } & 0.000000685 & 0.0006 & 0.0011 & 0.9991 & -0.0013 & 0.0013 \\\hline \text { Spending } & 0.0060 & 0.0046 & 1.2879 & 0.2047 & -0.0034 & 0.0153 \\\hline\end{array}$

-Referring to Instruction 13.10,estimate the mean percentage of students passing the proficiency test for all the schools that have a daily mean of 95% of students attending class,a mean teacher salary of 40,000 dollars and an instructional spending per pupil of 2,000 dollars.

Question 38

Instruction 13.6
One of the most common questions of prospective house buyers pertains to the average cost of heating in dollars (Y). To provide its customers with information on that matter, a large real estate firm used the following four variables to predict heating costs: the daily minimum outside temperature in degrees of Celsius (X₁), the amount of insulation in cm (X₂), the number of windows in the house (X₃) and the age of the furnace in years (X₄). Given below are the Microsoft Excel outputs of two regression models.

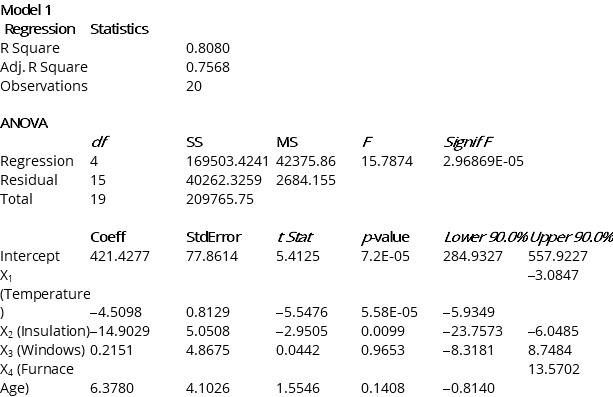
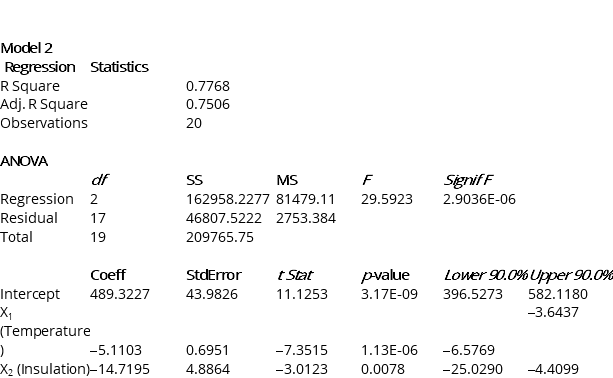
Referring to Instruction 13.6,the estimated value of the partial regression parameter β₁ in Model 1 means that

A) holding the effect of the other independent variables constant, an estimated expected $1 increase in heating costs is associated with a decrease in the daily minimum outside temperature by 4.51 degrees.
B) holding the effect of the other independent variables constant, a 1% increase in the daily minimum outside temperature results in an estimated expected decrease in heating costs by 4.51%.
C) holding the effect of the other independent variables constant, a 1 degree increase in the daily minimum outside temperature results in a decrease in heating costs by $4.51.
D) holding the effect of the other independent variables constant, a 1 degree increase in the daily minimum outside temperature results in an estimated expected decrease in heating costs by $4.51.

Question 39

Instruction 13.10
The education department's regional executive officer wanted to predict the percentage of students passing a Grade 6 proficiency test. She obtained the data on percentage of students passing the proficiency test (% Passing), daily average of the percentage of students attending class (% Attendance), average teacher salary in dollars (Salaries) and instructional spending per pupil in dollars (Spending) of 47 schools in the state.
Following is the multiple regression output with Y = % Passing as the dependent variable, X₁ = % Attendance, X₂ = Salaries and X₃ = Spending:
$\begin{array}{|l|r|}\hline\text { Regression } & \text { Statistics } \\\hline \text { Multiple R } & 0.7930 \\\hline \text { R Square } & 0.6288 \\\hline \text { Adj. R Square } & 0.602 \\\hline \text { Standard } & \\\text { Error } & 10.4570 \\\hline \text { Observations } & 47\\\hline\end{array}$

$\begin{array}{|l|r|r|r|r|r|}\hline \text { ANOVA } & & & & \\\hline & \text { d } & \text { SS } & \text { MS } & \text { F } & \text { Signif F } \\\hline \text { Regression } & 3 & 7965.08 & 2655.03 & 24.2802 & 0.0000 \\\hline \text { Residual } & 43 & 4702.02 & 109.35 & & \\\hline \text { Total } & 46 & 12667.11 & & & \\\hline\end{array}$


$\begin{array}{|l|r|r|r|r|r|r|} \hline & \text { Coeff } & \text { StolFrro } & \text { tSta } & \text { p-va/ue } & \text { Lower 95\% } & \text { Upper 95\% } \\\hline \text { Intercept } & -753.4225 & 101.1149 & -7.4511 & 0.0000 & -957.3401 & -549.5050 \\\hline \text { \% Attendance } & 8.5014 & 1.0771 & 7.8929 & 0.0000 & 6.3292 & 10.6735 \\\hline \text { Salary } & 0.000000685 & 0.0006 & 0.0011 & 0.9991 & -0.0013 & 0.0013 \\\hline \text { Spending } & 0.0060 & 0.0046 & 1.2879 & 0.2047 & -0.0034 & 0.0153 \\\hline\end{array}$

-Referring to Instruction 13.10,which of the following is a correct statement?

A) The daily mean of the percentage of students attending class is expected to go up by an estimated 8.50% when the percentage of students passing the proficiency test increases by 1% holding constant the effects of all the remaining independent variables.
B) The mean percentage of students passing the proficiency test is estimated to go up by 8.50% when daily mean of percentage of students attending class increases by 1%.
C) The daily mean of the percentage of students attending class is expected to go up by an estimated 8.50% when the percentage of students passing the proficiency test increases by 1%.
D) The mean percentage of students passing the proficiency test is estimated to go up by 8.50% when daily mean of the percentage of students attending class increases by 1% holding constant the effects of all the remaining independent variables.

Question 40

Instruction 13.7
You worked as an intern at We Always Win Car Insurance Company last summer. You notice that individual car insurance premium depends very much on the age of the individual, the number of traffic tickets received by the individual and the population density of the city in which the individual lives. You performed a regression analysis in Microsoft Excel and obtained the following information:
$\begin{array}{|l|l|l|l|l|l|l|}\hline \text {Regression}&\text {Analysis }\\\hline \text { MultipleR } &\quad & 0.63 \\\hline \text { R Square } && 0.40 \\\hline \text { Adj. R Square } && 0.23 \\\hline \text { Standard } & \\\text { Error } & & 50.00\\\hline \text { Observations } & & 15.00 \\\hline & & & & & \\\hline \text { ANOVA } &\\\hline & \text { df}& \text { SS } & \text { MS } & F & \text { Signif F } \\\hline \text { Regression } & 3 & & 5994.24 & 2.40 & 0.12 \\\hline \text { Residual } & 11 & 27496.82 & & & \\\hline \text { Total } & & 45479.54 & & & \\\hline & & & & & \\\hline& \text { Coeff } & \text { StdError } & \text { t Stat } & \text { p-value } & \text { Lower 99.0\%}&\text { Upper 99.0\% } \\\hline \text { Intercept } & 123.80 & 48.71 & 2.54 & 0.03 & -27.47 & 275.07 \\\hline \text { AGE } & 0.82 & 0.87 & -0.95 & 0.36 & -3.51 & 1.87 \\\hline \text { TICKETS } & 11.25 & 10.66 & 1.99 & 0.07 & -11.86 & 54.37 \\\hline \text { DENSITY } & -3.14 & 6.46 & -0.49 & 0.64 & -23.19 & 16.91\\\hline\end{array}$

-Referring to Instruction 13.7,the regression sum of squares that is missing in the ANOVA table should be ___________.

Question 41

AU: Question 37 is the same as Question 36. Please check.
Instruction 13.12
AU: Please advise if Instruction 13.12 can be renumbered to Instruction 13.11 and further questions renumbered. Or advise whether there shall be new Instruction 13.11 included.
The Head of the Accounting Department wanted to see if she could predict the average grade of students using the number of course units (credits) and total university entrance exam scores of each. She takes a sample of students and generates the following Microsoft Excel output:
OUTPUT
SUMMARY
$\begin{array} { l l } \text { Regression Statistics } & \\ \text { MultipleR } & 0.916 \\ \text { R Square } & 0.839 \\ \text { Adj. R Square } & 0.732 \\ \text { Std. Error } & 0.24685 \\ \text { Observations } & 6 \end{array}$

ANOVA
$\begin{array} { l l l l l l } & d f & \text { SS } & \text { MS } & F & \text { Signiff } \\ \text { Regression } & 2 & 0.95219 & 0.47610 & 7.813 & 0.0646 \\ \text { Residual } & 3 & 0.18281 & 0.06094 & & \\ \text { Total } & 5 & 1.13500 & & & \\ & & & & & \\ & \text { Coeff } & \text { StdError } & t \text { Stat } & p \text { value } & \\ \text { Intercept } & 4.593897 & 1.13374542 & 4.052 & 0.0271 & \\ \text { GDP } & - 0.247270 & 0.06268485 & - 3.945 & 0.0290 & \\ \text { Price } & 0.001443 & 0.00101241 & 1.425 & 0.2494 & \end{array}$ Note: Adj. R Square = Adjusted R Square; Std. Error = Standard Error

-Referring to Instruction 13.12,the value of the adjusted coefficient of multiple determination,r²adj,is ___________.

Question 42

Instruction 13.13
A financial analyst wanted to examine the relationship between salary (in $1,000) and four variables: age (X₁ = Age), experience in the field (X₂ = Exper), number of degrees (X₃ = Degrees) and number of previous jobs in the field (X₄ = Prevjobs). He took a sample of 20 employees and obtained the following Microsoft Excel output:
$\text { OUTPUT }$
$\begin{array}{ll}\text { SUMMARY}\\\text { Regression }&\text { Statistics}\\\text { Multiple R } & 0.992 \\\text { R Square } & 0.984 \\\text { Adj. R Square } & 0.979 \\\text { Std. Error } & 2.26743 \\\text { Observations } & 20\end{array}$

$\begin{array}{l}\text { ANOVA }\\\begin{array}{llllll} & \text { df } & \text { SS } & \text { MS } & F & \text { Signif F } \\\text { Regression } & 4 & 4609.83164 & 1152.45791 & 224.160 & 0.0001 \\\text { Residual } & 15 & 77.11836 & 5.14122 & & \\\text { Total } & 19 & 4686.95000 & & &\end{array}\end{array}$

$\begin{array}{lllll} & \text { Coeff } & \text { Std Error } & t \text { Stat } & p \text { value } \\\text { Intercept } & -9.611198 & 2.77988638 & -3.457 & 0.0035 \\\text { Age } & 1.327695 & 0.11491930 & 11.553 & 0.0001 \\\text { Exper } & -0.106705 & 0.14265559 & -0.748 & 0.4660 \\\text { Degrees } & 7.311332 & 0.80324187 & 9.102 & 0.0001 \\\text { Prevjobs } & -0.504168 & 0.44771573 & -1.126 & 0.2778\end{array}$

Note: Adj. R Square = Adjusted R Square; Std. Error = Standard Error

-Referring to Instruction 13.13,the estimate of the unit change in the mean of Y per unit change in X₄,taking into account the effects of the other three variables,is ___________.

Question 43

AU: Question 37 is the same as Question 36. Please check.
Instruction 13.12
AU: Please advise if Instruction 13.12 can be renumbered to Instruction 13.11 and further questions renumbered. Or advise whether there shall be new Instruction 13.11 included.
The Head of the Accounting Department wanted to see if she could predict the average grade of students using the number of course units (credits) and total university entrance exam scores of each. She takes a sample of students and generates the following Microsoft Excel output:
OUTPUT
SUMMARY
$\begin{array} { l l } \text { Regression Statistics } & \\ \text { MultipleR } & 0.916 \\ \text { R Square } & 0.839 \\ \text { Adj. R Square } & 0.732 \\ \text { Std. Error } & 0.24685 \\ \text { Observations } & 6 \end{array}$

ANOVA
$\begin{array} { l l l l l l } & d f & \text { SS } & \text { MS } & F & \text { Signiff } \\ \text { Regression } & 2 & 0.95219 & 0.47610 & 7.813 & 0.0646 \\ \text { Residual } & 3 & 0.18281 & 0.06094 & & \\ \text { Total } & 5 & 1.13500 & & & \\ & & & & & \\ & \text { Coeff } & \text { StdError } & t \text { Stat } & p \text { value } & \\ \text { Intercept } & 4.593897 & 1.13374542 & 4.052 & 0.0271 & \\ \text { GDP } & - 0.247270 & 0.06268485 & - 3.945 & 0.0290 & \\ \text { Price } & 0.001443 & 0.00101241 & 1.425 & 0.2494 & \end{array}$ Note: Adj. R Square = Adjusted R Square; Std. Error = Standard Error

-Referring to Instruction 13.12,the Head of Department wants to test H₀: $\beta$ ₁ = $\beta$ ₂ = 0.The appropriate alternative hypothesis is ___________.

Question 44

Instruction 13.13
A financial analyst wanted to examine the relationship between salary (in $1,000) and four variables: age (X₁ = Age), experience in the field (X₂ = Exper), number of degrees (X₃ = Degrees) and number of previous jobs in the field (X₄ = Prevjobs). He took a sample of 20 employees and obtained the following Microsoft Excel output:
$\text { OUTPUT }$
$\begin{array}{ll}\text { SUMMARY}\\\text { Regression }&\text { Statistics}\\\text { Multiple R } & 0.992 \\\text { R Square } & 0.984 \\\text { Adj. R Square } & 0.979 \\\text { Std. Error } & 2.26743 \\\text { Observations } & 20\end{array}$

$\begin{array}{l}\text { ANOVA }\\\begin{array}{llllll} & \text { df } & \text { SS } & \text { MS } & F & \text { Signif F } \\\text { Regression } & 4 & 4609.83164 & 1152.45791 & 224.160 & 0.0001 \\\text { Residual } & 15 & 77.11836 & 5.14122 & & \\\text { Total } & 19 & 4686.95000 & & &\end{array}\end{array}$

$\begin{array}{lllll} & \text { Coeff } & \text { Std Error } & t \text { Stat } & p \text { value } \\\text { Intercept } & -9.611198 & 2.77988638 & -3.457 & 0.0035 \\\text { Age } & 1.327695 & 0.11491930 & 11.553 & 0.0001 \\\text { Exper } & -0.106705 & 0.14265559 & -0.748 & 0.4660 \\\text { Degrees } & 7.311332 & 0.80324187 & 9.102 & 0.0001 \\\text { Prevjobs } & -0.504168 & 0.44771573 & -1.126 & 0.2778\end{array}$

Note: Adj. R Square = Adjusted R Square; Std. Error = Standard Error

-Referring to Instruction 13.13,the critical value of an F test on the entire regression for a level of significance of 0.01 is ___________.

Question 45

Instruction 13.13
A financial analyst wanted to examine the relationship between salary (in $1,000) and four variables: age (X₁ = Age), experience in the field (X₂ = Exper), number of degrees (X₃ = Degrees) and number of previous jobs in the field (X₄ = Prevjobs). He took a sample of 20 employees and obtained the following Microsoft Excel output:
$\text { OUTPUT }$
$\begin{array}{ll}\text { SUMMARY}\\\text { Regression }&\text { Statistics}\\\text { Multiple R } & 0.992 \\\text { R Square } & 0.984 \\\text { Adj. R Square } & 0.979 \\\text { Std. Error } & 2.26743 \\\text { Observations } & 20\end{array}$

$\begin{array}{l}\text { ANOVA }\\\begin{array}{llllll} & \text { df } & \text { SS } & \text { MS } & F & \text { Signif F } \\\text { Regression } & 4 & 4609.83164 & 1152.45791 & 224.160 & 0.0001 \\\text { Residual } & 15 & 77.11836 & 5.14122 & & \\\text { Total } & 19 & 4686.95000 & & &\end{array}\end{array}$

$\begin{array}{lllll} & \text { Coeff } & \text { Std Error } & t \text { Stat } & p \text { value } \\\text { Intercept } & -9.611198 & 2.77988638 & -3.457 & 0.0035 \\\text { Age } & 1.327695 & 0.11491930 & 11.553 & 0.0001 \\\text { Exper } & -0.106705 & 0.14265559 & -0.748 & 0.4660 \\\text { Degrees } & 7.311332 & 0.80324187 & 9.102 & 0.0001 \\\text { Prevjobs } & -0.504168 & 0.44771573 & -1.126 & 0.2778\end{array}$

Note: Adj. R Square = Adjusted R Square; Std. Error = Standard Error

-Referring to Instruction 13.13,the p-value of the F test for the significance of the entire regression is ___________.

Question 46

Instruction 13.13
A financial analyst wanted to examine the relationship between salary (in $1,000) and four variables: age (X₁ = Age), experience in the field (X₂ = Exper), number of degrees (X₃ = Degrees) and number of previous jobs in the field (X₄ = Prevjobs). He took a sample of 20 employees and obtained the following Microsoft Excel output:
$\text { OUTPUT }$
$\begin{array}{ll}\text { SUMMARY}\\\text { Regression }&\text { Statistics}\\\text { Multiple R } & 0.992 \\\text { R Square } & 0.984 \\\text { Adj. R Square } & 0.979 \\\text { Std. Error } & 2.26743 \\\text { Observations } & 20\end{array}$

$\begin{array}{l}\text { ANOVA }\\\begin{array}{llllll} & \text { df } & \text { SS } & \text { MS } & F & \text { Signif F } \\\text { Regression } & 4 & 4609.83164 & 1152.45791 & 224.160 & 0.0001 \\\text { Residual } & 15 & 77.11836 & 5.14122 & & \\\text { Total } & 19 & 4686.95000 & & &\end{array}\end{array}$

$\begin{array}{lllll} & \text { Coeff } & \text { Std Error } & t \text { Stat } & p \text { value } \\\text { Intercept } & -9.611198 & 2.77988638 & -3.457 & 0.0035 \\\text { Age } & 1.327695 & 0.11491930 & 11.553 & 0.0001 \\\text { Exper } & -0.106705 & 0.14265559 & -0.748 & 0.4660 \\\text { Degrees } & 7.311332 & 0.80324187 & 9.102 & 0.0001 \\\text { Prevjobs } & -0.504168 & 0.44771573 & -1.126 & 0.2778\end{array}$

Note: Adj. R Square = Adjusted R Square; Std. Error = Standard Error

-Referring to Instruction 13.13,the net regression coefficient of X₂ is ___________.

Question 47

Instruction 13.13
A financial analyst wanted to examine the relationship between salary (in $1,000) and four variables: age (X₁ = Age), experience in the field (X₂ = Exper), number of degrees (X₃ = Degrees) and number of previous jobs in the field (X₄ = Prevjobs). He took a sample of 20 employees and obtained the following Microsoft Excel output:
$\text { OUTPUT }$
$\begin{array}{ll}\text { SUMMARY}\\\text { Regression }&\text { Statistics}\\\text { Multiple R } & 0.992 \\\text { R Square } & 0.984 \\\text { Adj. R Square } & 0.979 \\\text { Std. Error } & 2.26743 \\\text { Observations } & 20\end{array}$

$\begin{array}{l}\text { ANOVA }\\\begin{array}{llllll} & \text { df } & \text { SS } & \text { MS } & F & \text { Signif F } \\\text { Regression } & 4 & 4609.83164 & 1152.45791 & 224.160 & 0.0001 \\\text { Residual } & 15 & 77.11836 & 5.14122 & & \\\text { Total } & 19 & 4686.95000 & & &\end{array}\end{array}$

$\begin{array}{lllll} & \text { Coeff } & \text { Std Error } & t \text { Stat } & p \text { value } \\\text { Intercept } & -9.611198 & 2.77988638 & -3.457 & 0.0035 \\\text { Age } & 1.327695 & 0.11491930 & 11.553 & 0.0001 \\\text { Exper } & -0.106705 & 0.14265559 & -0.748 & 0.4660 \\\text { Degrees } & 7.311332 & 0.80324187 & 9.102 & 0.0001 \\\text { Prevjobs } & -0.504168 & 0.44771573 & -1.126 & 0.2778\end{array}$

Note: Adj. R Square = Adjusted R Square; Std. Error = Standard Error

-Referring to Instruction 13.13,the value of the adjusted coefficient of multiple determination,adjusted r²,is ___________.

Question 48

Instruction 13.13
A financial analyst wanted to examine the relationship between salary (in $1,000) and four variables: age (X₁ = Age), experience in the field (X₂ = Exper), number of degrees (X₃ = Degrees) and number of previous jobs in the field (X₄ = Prevjobs). He took a sample of 20 employees and obtained the following Microsoft Excel output:
$\text { OUTPUT }$
$\begin{array}{ll}\text { SUMMARY}\\\text { Regression }&\text { Statistics}\\\text { Multiple R } & 0.992 \\\text { R Square } & 0.984 \\\text { Adj. R Square } & 0.979 \\\text { Std. Error } & 2.26743 \\\text { Observations } & 20\end{array}$

$\begin{array}{l}\text { ANOVA }\\\begin{array}{llllll} & \text { df } & \text { SS } & \text { MS } & F & \text { Signif F } \\\text { Regression } & 4 & 4609.83164 & 1152.45791 & 224.160 & 0.0001 \\\text { Residual } & 15 & 77.11836 & 5.14122 & & \\\text { Total } & 19 & 4686.95000 & & &\end{array}\end{array}$

$\begin{array}{lllll} & \text { Coeff } & \text { Std Error } & t \text { Stat } & p \text { value } \\\text { Intercept } & -9.611198 & 2.77988638 & -3.457 & 0.0035 \\\text { Age } & 1.327695 & 0.11491930 & 11.553 & 0.0001 \\\text { Exper } & -0.106705 & 0.14265559 & -0.748 & 0.4660 \\\text { Degrees } & 7.311332 & 0.80324187 & 9.102 & 0.0001 \\\text { Prevjobs } & -0.504168 & 0.44771573 & -1.126 & 0.2778\end{array}$

Note: Adj. R Square = Adjusted R Square; Std. Error = Standard Error

-Referring to Instruction 13.13,the value of the coefficient of multiple determination,r²_Y.1234,is ___________.

Question 49

Instruction 13.14
Given below are results from the regression analysis where the dependent variable is the number of weeks a worker is unemployed due to a layoff (Unemploy) and the independent variables are the age of the worker (Age), the number of years of education received (Edu), the number of years at the previous job (Job Yr), a dummy variable for marital status (Married: 1 = married, 0 = otherwise), a dummy variable for head of household (Head: 1 = yes, 0 = no) and a dummy variable for management position (Manager: 1 = yes, 0 = no). We shall call this Model 1.
Model 1
Regression Statistics
$\begin{array} { l l } \text { Multiple R } & 0.7035 \\ \text { R Square } & 0.4949 \\ \text { Adj. R Square } & 0.4030 \\ \text { Std. Error } & 18.4861 \\ \text { Observations } & 40 \end{array}$

ANOVA
$\begin{array} { l l l l l l l } & d f & \text { SS } & \text { MS } & F & \text { Signiff } & \\ \text { Regression } & 6 & 11048.6415 & 1841.4402 & 5.3885 & 0.00057 & \\ \text { Residual } & 33 & 11277.2586 & 341.7351 & & & \\ \text { Total } & 39 & 223325.9 & & & & \\ & & & & & & \\ & \text { Coeff } & \text { StdError } & \text { tStat } & p \text { value } & \text { Lower 95\% } & \text { Upper95\% } \\ \text { Intercept } & 32.6595 & 23.18302 & 1.4088 & 0.1683 & - 14.5067 & 79.8257 \\ \text { Age } & 1.2915 & 0.3599 & 3.5883 & 0.0011 & 0.5592 & 2.0238 \\ \text { Edu } & - 1.3537 & 1.1766 & - 1.1504 & 0.2582 & - 3.7476 & 1.0402 \\ \text { Job Yr } & 0.6171 & 0.5940 & 1.0389 & 0.3064 & - 0.5914 & 1.8257 \\ \text { Married } & - 5.2189 & 7.6068 & - 0.6861 & 0.4974 & - 20.6950 & 10.2571 \\ \text { Head } & - 14.2978 & 7.6479 & - 1.8695 & 0.0704 & - 29.8575 & 1.2618 \\ \text { Manager } & - 24.8203 & 11.6932 & - 2.1226 & 0.0414 & - 48.6102 & - 1.0303 \end{array}$ Model 2 is the regression analysis where the dependent variable is Unemploy and the independent variables are Age and Manager. The results of the regression analysis are given below:
Mode 2
Regression Statistics
$\begin{array} { l l } \text { Multiple R } & 0.6391 \\ \text { R Square } & 0.4085 \\ \text { Adj. R Square } & 0.3765 \\ \text { Std. Error } & 18.8929 \\ \text { Observations } & 40 \end{array}$
ANOVA
$\begin{array} { l l l l l l } & d f & \text { SS } & \text { MS } & F & \text { Signiff } \\ \text { Regression } & 2 & 9119.0897 & 4559.5448 & 12.7740 & 0.0000 \\ \text { Residual } & 37 & 13206.8103 & 356.9408 & & \\ \text { Total } & 39 & 22325.9 & & & \\ & & & & & \\ & \text { Coeff } & \text { StdError } & t \text { Stat } & p \text { value } & \\ \text { Intercept } & - 0.2143 & 11.5796 & - 0.0185 & 0.9853 & \\ \text { Age } & 1.4448 & 0.3160 & 4.5717 & 0.0000 & \\ \text { Manager } & - 22.5761 & 11.3488 & - 1.9893 & 0.0541 & \end{array}$

-Referring to Instruction 13.14 Model 1,predict the number of weeks being unemployed due to a layoff for a worker who is a 30-year-old,has 10 years of education,has 15 years of experience at the previous job,is married,is the head of household and is a manager.

Question 50

Instruction 13.13
A financial analyst wanted to examine the relationship between salary (in $1,000) and four variables: age (X₁ = Age), experience in the field (X₂ = Exper), number of degrees (X₃ = Degrees) and number of previous jobs in the field (X₄ = Prevjobs). He took a sample of 20 employees and obtained the following Microsoft Excel output:
$\text { OUTPUT }$
$\begin{array}{ll}\text { SUMMARY}\\\text { Regression }&\text { Statistics}\\\text { Multiple R } & 0.992 \\\text { R Square } & 0.984 \\\text { Adj. R Square } & 0.979 \\\text { Std. Error } & 2.26743 \\\text { Observations } & 20\end{array}$

$\begin{array}{l}\text { ANOVA }\\\begin{array}{llllll} & \text { df } & \text { SS } & \text { MS } & F & \text { Signif F } \\\text { Regression } & 4 & 4609.83164 & 1152.45791 & 224.160 & 0.0001 \\\text { Residual } & 15 & 77.11836 & 5.14122 & & \\\text { Total } & 19 & 4686.95000 & & &\end{array}\end{array}$

$\begin{array}{lllll} & \text { Coeff } & \text { Std Error } & t \text { Stat } & p \text { value } \\\text { Intercept } & -9.611198 & 2.77988638 & -3.457 & 0.0035 \\\text { Age } & 1.327695 & 0.11491930 & 11.553 & 0.0001 \\\text { Exper } & -0.106705 & 0.14265559 & -0.748 & 0.4660 \\\text { Degrees } & 7.311332 & 0.80324187 & 9.102 & 0.0001 \\\text { Prevjobs } & -0.504168 & 0.44771573 & -1.126 & 0.2778\end{array}$

Note: Adj. R Square = Adjusted R Square; Std. Error = Standard Error

-Referring to Instruction 13.13,the predicted salary for a 35-year-old person with 10 years of experience,3 degrees and 1 previous job is ___________.

Question 51

AU: Question 37 is the same as Question 36. Please check.
Instruction 13.12
AU: Please advise if Instruction 13.12 can be renumbered to Instruction 13.11 and further questions renumbered. Or advise whether there shall be new Instruction 13.11 included.
The Head of the Accounting Department wanted to see if she could predict the average grade of students using the number of course units (credits) and total university entrance exam scores of each. She takes a sample of students and generates the following Microsoft Excel output:
OUTPUT
SUMMARY
$\begin{array} { l l } \text { Regression Statistics } & \\ \text { MultipleR } & 0.916 \\ \text { R Square } & 0.839 \\ \text { Adj. R Square } & 0.732 \\ \text { Std. Error } & 0.24685 \\ \text { Observations } & 6 \end{array}$

ANOVA
$\begin{array} { l l l l l l } & d f & \text { SS } & \text { MS } & F & \text { Signiff } \\ \text { Regression } & 2 & 0.95219 & 0.47610 & 7.813 & 0.0646 \\ \text { Residual } & 3 & 0.18281 & 0.06094 & & \\ \text { Total } & 5 & 1.13500 & & & \\ & & & & & \\ & \text { Coeff } & \text { StdError } & t \text { Stat } & p \text { value } & \\ \text { Intercept } & 4.593897 & 1.13374542 & 4.052 & 0.0271 & \\ \text { GDP } & - 0.247270 & 0.06268485 & - 3.945 & 0.0290 & \\ \text { Price } & 0.001443 & 0.00101241 & 1.425 & 0.2494 & \end{array}$ Note: Adj. R Square = Adjusted R Square; Std. Error = Standard Error

-Referring to Instruction 13.12,the Head of Department wants to test H₀: $\beta$ ₁ = $\beta$ ₂ = 0.The critical value of the F test for a level of significance of 0.05 is ___________

Question 52

AU: Question 37 is the same as Question 36. Please check.
Instruction 13.12
AU: Please advise if Instruction 13.12 can be renumbered to Instruction 13.11 and further questions renumbered. Or advise whether there shall be new Instruction 13.11 included.
The Head of the Accounting Department wanted to see if she could predict the average grade of students using the number of course units (credits) and total university entrance exam scores of each. She takes a sample of students and generates the following Microsoft Excel output:
OUTPUT
SUMMARY
$\begin{array} { l l } \text { Regression Statistics } & \\ \text { MultipleR } & 0.916 \\ \text { R Square } & 0.839 \\ \text { Adj. R Square } & 0.732 \\ \text { Std. Error } & 0.24685 \\ \text { Observations } & 6 \end{array}$

ANOVA
$\begin{array} { l l l l l l } & d f & \text { SS } & \text { MS } & F & \text { Signiff } \\ \text { Regression } & 2 & 0.95219 & 0.47610 & 7.813 & 0.0646 \\ \text { Residual } & 3 & 0.18281 & 0.06094 & & \\ \text { Total } & 5 & 1.13500 & & & \\ & & & & & \\ & \text { Coeff } & \text { StdError } & t \text { Stat } & p \text { value } & \\ \text { Intercept } & 4.593897 & 1.13374542 & 4.052 & 0.0271 & \\ \text { GDP } & - 0.247270 & 0.06268485 & - 3.945 & 0.0290 & \\ \text { Price } & 0.001443 & 0.00101241 & 1.425 & 0.2494 & \end{array}$ Note: Adj. R Square = Adjusted R Square; Std. Error = Standard Error

-Referring to Instruction 13.12,the Head of Department wants to test H₀: $\beta$ ₁ = $\beta$ ₂ = 0.The p-value of the test is ___________.

Question 53

Instruction 13.14
Given below are results from the regression analysis where the dependent variable is the number of weeks a worker is unemployed due to a layoff (Unemploy) and the independent variables are the age of the worker (Age), the number of years of education received (Edu), the number of years at the previous job (Job Yr), a dummy variable for marital status (Married: 1 = married, 0 = otherwise), a dummy variable for head of household (Head: 1 = yes, 0 = no) and a dummy variable for management position (Manager: 1 = yes, 0 = no). We shall call this Model 1.
Model 1
Regression Statistics
$\begin{array} { l l } \text { Multiple R } & 0.7035 \\ \text { R Square } & 0.4949 \\ \text { Adj. R Square } & 0.4030 \\ \text { Std. Error } & 18.4861 \\ \text { Observations } & 40 \end{array}$

ANOVA
$\begin{array} { l l l l l l l } & d f & \text { SS } & \text { MS } & F & \text { Signiff } & \\ \text { Regression } & 6 & 11048.6415 & 1841.4402 & 5.3885 & 0.00057 & \\ \text { Residual } & 33 & 11277.2586 & 341.7351 & & & \\ \text { Total } & 39 & 223325.9 & & & & \\ & & & & & & \\ & \text { Coeff } & \text { StdError } & \text { tStat } & p \text { value } & \text { Lower 95\% } & \text { Upper95\% } \\ \text { Intercept } & 32.6595 & 23.18302 & 1.4088 & 0.1683 & - 14.5067 & 79.8257 \\ \text { Age } & 1.2915 & 0.3599 & 3.5883 & 0.0011 & 0.5592 & 2.0238 \\ \text { Edu } & - 1.3537 & 1.1766 & - 1.1504 & 0.2582 & - 3.7476 & 1.0402 \\ \text { Job Yr } & 0.6171 & 0.5940 & 1.0389 & 0.3064 & - 0.5914 & 1.8257 \\ \text { Married } & - 5.2189 & 7.6068 & - 0.6861 & 0.4974 & - 20.6950 & 10.2571 \\ \text { Head } & - 14.2978 & 7.6479 & - 1.8695 & 0.0704 & - 29.8575 & 1.2618 \\ \text { Manager } & - 24.8203 & 11.6932 & - 2.1226 & 0.0414 & - 48.6102 & - 1.0303 \end{array}$ Model 2 is the regression analysis where the dependent variable is Unemploy and the independent variables are Age and Manager. The results of the regression analysis are given below:
Mode 2
Regression Statistics
$\begin{array} { l l } \text { Multiple R } & 0.6391 \\ \text { R Square } & 0.4085 \\ \text { Adj. R Square } & 0.3765 \\ \text { Std. Error } & 18.8929 \\ \text { Observations } & 40 \end{array}$
ANOVA
$\begin{array} { l l l l l l } & d f & \text { SS } & \text { MS } & F & \text { Signiff } \\ \text { Regression } & 2 & 9119.0897 & 4559.5448 & 12.7740 & 0.0000 \\ \text { Residual } & 37 & 13206.8103 & 356.9408 & & \\ \text { Total } & 39 & 22325.9 & & & \\ & & & & & \\ & \text { Coeff } & \text { StdError } & t \text { Stat } & p \text { value } & \\ \text { Intercept } & - 0.2143 & 11.5796 & - 0.0185 & 0.9853 & \\ \text { Age } & 1.4448 & 0.3160 & 4.5717 & 0.0000 & \\ \text { Manager } & - 22.5761 & 11.3488 & - 1.9893 & 0.0541 & \end{array}$

-Referring to Instruction 13.14 Model 1,estimate the mean number of weeks being unemployed due to a layoff for a worker who is a 30-year-old,has 10 years of education,has 15 years of experience at the previous job,is married,is the head of household and is a manager.

Question 54

When an additional explanatory variable is introduced into a multiple regression model,the coefficient of multiple determination will never decrease.

Question 55

Instruction 13.14
Given below are results from the regression analysis where the dependent variable is the number of weeks a worker is unemployed due to a layoff (Unemploy) and the independent variables are the age of the worker (Age), the number of years of education received (Edu), the number of years at the previous job (Job Yr), a dummy variable for marital status (Married: 1 = married, 0 = otherwise), a dummy variable for head of household (Head: 1 = yes, 0 = no) and a dummy variable for management position (Manager: 1 = yes, 0 = no). We shall call this Model 1.
Model 1
Regression Statistics
$\begin{array} { l l } \text { Multiple R } & 0.7035 \\ \text { R Square } & 0.4949 \\ \text { Adj. R Square } & 0.4030 \\ \text { Std. Error } & 18.4861 \\ \text { Observations } & 40 \end{array}$

ANOVA
$\begin{array} { l l l l l l l } & d f & \text { SS } & \text { MS } & F & \text { Signiff } & \\ \text { Regression } & 6 & 11048.6415 & 1841.4402 & 5.3885 & 0.00057 & \\ \text { Residual } & 33 & 11277.2586 & 341.7351 & & & \\ \text { Total } & 39 & 223325.9 & & & & \\ & & & & & & \\ & \text { Coeff } & \text { StdError } & \text { tStat } & p \text { value } & \text { Lower 95\% } & \text { Upper95\% } \\ \text { Intercept } & 32.6595 & 23.18302 & 1.4088 & 0.1683 & - 14.5067 & 79.8257 \\ \text { Age } & 1.2915 & 0.3599 & 3.5883 & 0.0011 & 0.5592 & 2.0238 \\ \text { Edu } & - 1.3537 & 1.1766 & - 1.1504 & 0.2582 & - 3.7476 & 1.0402 \\ \text { Job Yr } & 0.6171 & 0.5940 & 1.0389 & 0.3064 & - 0.5914 & 1.8257 \\ \text { Married } & - 5.2189 & 7.6068 & - 0.6861 & 0.4974 & - 20.6950 & 10.2571 \\ \text { Head } & - 14.2978 & 7.6479 & - 1.8695 & 0.0704 & - 29.8575 & 1.2618 \\ \text { Manager } & - 24.8203 & 11.6932 & - 2.1226 & 0.0414 & - 48.6102 & - 1.0303 \end{array}$ Model 2 is the regression analysis where the dependent variable is Unemploy and the independent variables are Age and Manager. The results of the regression analysis are given below:
Mode 2
Regression Statistics
$\begin{array} { l l } \text { Multiple R } & 0.6391 \\ \text { R Square } & 0.4085 \\ \text { Adj. R Square } & 0.3765 \\ \text { Std. Error } & 18.8929 \\ \text { Observations } & 40 \end{array}$
ANOVA
$\begin{array} { l l l l l l } & d f & \text { SS } & \text { MS } & F & \text { Signiff } \\ \text { Regression } & 2 & 9119.0897 & 4559.5448 & 12.7740 & 0.0000 \\ \text { Residual } & 37 & 13206.8103 & 356.9408 & & \\ \text { Total } & 39 & 22325.9 & & & \\ & & & & & \\ & \text { Coeff } & \text { StdError } & t \text { Stat } & p \text { value } & \\ \text { Intercept } & - 0.2143 & 11.5796 & - 0.0185 & 0.9853 & \\ \text { Age } & 1.4448 & 0.3160 & 4.5717 & 0.0000 & \\ \text { Manager } & - 22.5761 & 11.3488 & - 1.9893 & 0.0541 & \end{array}$

-Referring to Instruction 13.14 Model 1,which of the following is a correct statement?

A) On average, a worker who is a year older is estimated to stay jobless shorter by approximately 1.35 weeks, while holding constant the effects of all the remaining independent variables.
B) On average, a worker who is a year older is estimated to stay jobless longer by approximately 0.62 weeks, while holding constant the effects of all the remaining independent variables.
C) On average, a worker who is a year older is estimated to stay jobless longer by approximately 1.29 weeks, while holding constant the effects of all the remaining independent variables.
D) On average, a worker who is a year older is estimated to stay jobless longer by approximately 32.66 weeks, while holding constant the effects of all the remaining independent variables.

Question 56

AU: Question 37 is the same as Question 36. Please check.
Instruction 13.12
AU: Please advise if Instruction 13.12 can be renumbered to Instruction 13.11 and further questions renumbered. Or advise whether there shall be new Instruction 13.11 included.
The Head of the Accounting Department wanted to see if she could predict the average grade of students using the number of course units (credits) and total university entrance exam scores of each. She takes a sample of students and generates the following Microsoft Excel output:
OUTPUT
SUMMARY
$\begin{array} { l l } \text { Regression Statistics } & \\ \text { MultipleR } & 0.916 \\ \text { R Square } & 0.839 \\ \text { Adj. R Square } & 0.732 \\ \text { Std. Error } & 0.24685 \\ \text { Observations } & 6 \end{array}$

ANOVA
$\begin{array} { l l l l l l } & d f & \text { SS } & \text { MS } & F & \text { Signiff } \\ \text { Regression } & 2 & 0.95219 & 0.47610 & 7.813 & 0.0646 \\ \text { Residual } & 3 & 0.18281 & 0.06094 & & \\ \text { Total } & 5 & 1.13500 & & & \\ & & & & & \\ & \text { Coeff } & \text { StdError } & t \text { Stat } & p \text { value } & \\ \text { Intercept } & 4.593897 & 1.13374542 & 4.052 & 0.0271 & \\ \text { GDP } & - 0.247270 & 0.06268485 & - 3.945 & 0.0290 & \\ \text { Price } & 0.001443 & 0.00101241 & 1.425 & 0.2494 & \end{array}$ Note: Adj. R Square = Adjusted R Square; Std. Error = Standard Error

-Referring to Instruction 13.12,the Head of Department wants to test H₀: $\beta$ ₁ = $\beta$ ₂ = 0.The value of the F test statistic is ___________.

Question 57

The coefficient of multiple determination r² measures the proportion of variation in Y that is explained by X₁ and X₂.

Question 58

Instruction 13.13
A financial analyst wanted to examine the relationship between salary (in $1,000) and four variables: age (X₁ = Age), experience in the field (X₂ = Exper), number of degrees (X₃ = Degrees) and number of previous jobs in the field (X₄ = Prevjobs). He took a sample of 20 employees and obtained the following Microsoft Excel output:
$\text { OUTPUT }$
$\begin{array}{ll}\text { SUMMARY}\\\text { Regression }&\text { Statistics}\\\text { Multiple R } & 0.992 \\\text { R Square } & 0.984 \\\text { Adj. R Square } & 0.979 \\\text { Std. Error } & 2.26743 \\\text { Observations } & 20\end{array}$

$\begin{array}{l}\text { ANOVA }\\\begin{array}{llllll} & \text { df } & \text { SS } & \text { MS } & F & \text { Signif F } \\\text { Regression } & 4 & 4609.83164 & 1152.45791 & 224.160 & 0.0001 \\\text { Residual } & 15 & 77.11836 & 5.14122 & & \\\text { Total } & 19 & 4686.95000 & & &\end{array}\end{array}$

$\begin{array}{lllll} & \text { Coeff } & \text { Std Error } & t \text { Stat } & p \text { value } \\\text { Intercept } & -9.611198 & 2.77988638 & -3.457 & 0.0035 \\\text { Age } & 1.327695 & 0.11491930 & 11.553 & 0.0001 \\\text { Exper } & -0.106705 & 0.14265559 & -0.748 & 0.4660 \\\text { Degrees } & 7.311332 & 0.80324187 & 9.102 & 0.0001 \\\text { Prevjobs } & -0.504168 & 0.44771573 & -1.126 & 0.2778\end{array}$

Note: Adj. R Square = Adjusted R Square; Std. Error = Standard Error

-Referring to Instruction 13.13,the value of the F statistic for testing the significance of the entire regression is ___________.

Question 59

Instruction 13.13
A financial analyst wanted to examine the relationship between salary (in $1,000) and four variables: age (X₁ = Age), experience in the field (X₂ = Exper), number of degrees (X₃ = Degrees) and number of previous jobs in the field (X₄ = Prevjobs). He took a sample of 20 employees and obtained the following Microsoft Excel output:
$\text { OUTPUT }$
$\begin{array}{ll}\text { SUMMARY}\\\text { Regression }&\text { Statistics}\\\text { Multiple R } & 0.992 \\\text { R Square } & 0.984 \\\text { Adj. R Square } & 0.979 \\\text { Std. Error } & 2.26743 \\\text { Observations } & 20\end{array}$

$\begin{array}{l}\text { ANOVA }\\\begin{array}{llllll} & \text { df } & \text { SS } & \text { MS } & F & \text { Signif F } \\\text { Regression } & 4 & 4609.83164 & 1152.45791 & 224.160 & 0.0001 \\\text { Residual } & 15 & 77.11836 & 5.14122 & & \\\text { Total } & 19 & 4686.95000 & & &\end{array}\end{array}$

$\begin{array}{lllll} & \text { Coeff } & \text { Std Error } & t \text { Stat } & p \text { value } \\\text { Intercept } & -9.611198 & 2.77988638 & -3.457 & 0.0035 \\\text { Age } & 1.327695 & 0.11491930 & 11.553 & 0.0001 \\\text { Exper } & -0.106705 & 0.14265559 & -0.748 & 0.4660 \\\text { Degrees } & 7.311332 & 0.80324187 & 9.102 & 0.0001 \\\text { Prevjobs } & -0.504168 & 0.44771573 & -1.126 & 0.2778\end{array}$

Note: Adj. R Square = Adjusted R Square; Std. Error = Standard Error

-Referring to Instruction 13.13,the analyst wants to use an F test to test H₀: $\beta$ ₁ = $\beta$ ₂ = $\beta$ ₃ = $\beta$ ₄ = 0.The appropriate alternative hypothesis is ___________

Question 60

AU: Question 37 is the same as Question 36. Please check.
Instruction 13.12
AU: Please advise if Instruction 13.12 can be renumbered to Instruction 13.11 and further questions renumbered. Or advise whether there shall be new Instruction 13.11 included.
The Head of the Accounting Department wanted to see if she could predict the average grade of students using the number of course units (credits) and total university entrance exam scores of each. She takes a sample of students and generates the following Microsoft Excel output:
OUTPUT
SUMMARY
$\begin{array} { l l } \text { Regression Statistics } & \\ \text { MultipleR } & 0.916 \\ \text { R Square } & 0.839 \\ \text { Adj. R Square } & 0.732 \\ \text { Std. Error } & 0.24685 \\ \text { Observations } & 6 \end{array}$

ANOVA
$\begin{array} { l l l l l l } & d f & \text { SS } & \text { MS } & F & \text { Signiff } \\ \text { Regression } & 2 & 0.95219 & 0.47610 & 7.813 & 0.0646 \\ \text { Residual } & 3 & 0.18281 & 0.06094 & & \\ \text { Total } & 5 & 1.13500 & & & \\ & & & & & \\ & \text { Coeff } & \text { StdError } & t \text { Stat } & p \text { value } & \\ \text { Intercept } & 4.593897 & 1.13374542 & 4.052 & 0.0271 & \\ \text { GDP } & - 0.247270 & 0.06268485 & - 3.945 & 0.0290 & \\ \text { Price } & 0.001443 & 0.00101241 & 1.425 & 0.2494 & \end{array}$ Note: Adj. R Square = Adjusted R Square; Std. Error = Standard Error

-Referring to Instruction 13.12,the value of the coefficient of multiple determination,r²_Y.12,is ___________.

Question 61

In calculating the standard error of the estimate,S_YX = $$\sqrt { \mathrm { MSE } }$$ there are n * k * 1 degrees of freedom,where n is the sample size and k represents the number of independent variables in the model.

Question 62

In a multiple regression model,which of the following is correct regarding the value of the adjusted r²?

A) It has to be larger than the coefficient of multiple determination.
B) It can be larger than 1.
C) It can be negative.
D) It has to be positive.

Question 63

The variation attribuInstruction to factors other than the relationship between the independent variables and the explained variable in a regression analysis is represented by

A) regression mean squares.
B) total sum of squares.
C) regression sum of squares.
D) error sum of squares.

Question 64

From the coefficient of multiple determination,you cannot detect the strength of the relationship between Y and any individual independent variable.

Question 65

Instruction 13.15
An economist is interested to see how consumption for an economy (in $ billions) is influenced by gross domestic product ($ billions) and aggregate price (consumer price index). The Microsoft Excel output of this regression is partially reproduced below.
OUTPUT
SUMMARY
Regression Statistics
$\begin{array} { l l } \text { MultipleR } & 0.991 \\ \text { R Square } & 0.982 \\ \text { Adj. R Square } & 0.976 \\ \text { Std. Error } & 0.299 \\ \text { Observations } & 10 \end{array}$

ANOVA
$\begin{array} { l l l l l l } & d f & \text { SS } & \text { MS } & F & \text { Signiff } \\ \text { Regression } & 2 & 33.4163 & 16.7082 & 186.325 & 0.0001 \\ \text { Residual } & 7 & 0.6277 & 0.0897 & & \\ \text { Total } & 9 & 34.0440 & & & \\ & & & & & \\ & \text { Coeff } & \text { StdError } & t \text { Stat } & p \text { value } & \\ \text { Intercept } & - 1.6335 & 0.5674 & - 0.152 & 0.8837 & \\ \text { GDP } & 0.7654 & 0.0574 & 13.340 & 0.0001 & \\ \text { Price } & - 0.0006 & 0.0028 & - 0.219 & 0.8330 & \end{array}$ Note: Adj. R Square = Adjusted R Square; Std. Error = Standard Error

-Referring to Instruction 13.15,when the economist used a simple linear regression model with consumption as the dependent variable and GDP as the independent variable,he obtained an r² value of 0.971.What additional percentage of the total variation of consumption has been explained by including aggregate prices in the multiple regression?

A) 98.2
B) 1.1
C) 11.1
D) 2.8

Question 66

Instruction 13.15
An economist is interested to see how consumption for an economy (in $ billions) is influenced by gross domestic product ($ billions) and aggregate price (consumer price index). The Microsoft Excel output of this regression is partially reproduced below.
OUTPUT
SUMMARY
Regression Statistics
$\begin{array} { l l } \text { MultipleR } & 0.991 \\ \text { R Square } & 0.982 \\ \text { Adj. R Square } & 0.976 \\ \text { Std. Error } & 0.299 \\ \text { Observations } & 10 \end{array}$

ANOVA
$\begin{array} { l l l l l l } & d f & \text { SS } & \text { MS } & F & \text { Signiff } \\ \text { Regression } & 2 & 33.4163 & 16.7082 & 186.325 & 0.0001 \\ \text { Residual } & 7 & 0.6277 & 0.0897 & & \\ \text { Total } & 9 & 34.0440 & & & \\ & & & & & \\ & \text { Coeff } & \text { StdError } & t \text { Stat } & p \text { value } & \\ \text { Intercept } & - 1.6335 & 0.5674 & - 0.152 & 0.8837 & \\ \text { GDP } & 0.7654 & 0.0574 & 13.340 & 0.0001 & \\ \text { Price } & - 0.0006 & 0.0028 & - 0.219 & 0.8330 & \end{array}$ Note: Adj. R Square = Adjusted R Square; Std. Error = Standard Error

-Referring to Instruction 13.15,the p-value for GDP is

A) 0.01.
B) 0.05.
C) 0.001.
D) None of the above.

Question 67

The total sum of squares (SST)in a regression model will never exceed the regression sum of squares (SSR).

Question 68

Instruction 13.16
A real estate builder wishes to determine how house size (House) is influenced by family income (Income), family size (Size) and education of the head of household (School). House size is measured in hundreds of square metres, income is measured in thousands of dollars and education is in years. The builder randomly selected 50 families and ran the multiple regression. Microsoft Excel output is provided below:
OUTPUT
SUMMARY
Regression Statistics
$\begin{array} { l l } \text { Multiple R } & 0.865 \\ \text { R Square } & 0.748 \\ \text { Adj. R Square } & 0.726 \\ \text { Std. Error } & 5.195 \\ \text { Observations } & 50 \end{array}$

ANOVA
$\begin{array} { l l l l l l } & d f & \text { SS } & \text { MS } & F & \text { Signiff } \\ \text { Regression } & & 3605.7736 & 901.4434 & & 0.0001 \\ \text { Residual } & & 1214.2264 & 26.9828 & & \\ \text { Total } & 49 & 4820.0000 & & & \\ & & & & & \\ & \text { Coeff } & \text { StdError } & t \text { Stat } & p \text { value } & \\ \text { Intercept } & - 1.6335 & 5.8078 & - 0.281 & 0.7798 & \\ \text { Income } & 0.4485 & 0.1137 & 3.9545 & 0.0003 & \\ \text { Size } & 4.2615 & 0.8062 & 5.286 & 0.0001 & \\ \text { School } & - 0.6517 & 0.4319 & - 1.509 & 0.1383 & \end{array}$ Note: Adj. R Square = Adjusted R Square; Std. Error = Standard Error

-Referring to Instruction 13.16,what fraction of the variability in house size is explained by income,size of family and education?

A) 74.8%
B) 33.4%
C) 27.0%
D) 86.5%

Question 69

A regression had the following results: SST = 102.55,SSE = 82.04.It can be said that 90.0% of the variation in the dependent variable is explained by the independent variables in the regression.

Question 70

When an explanatory variable is dropped from a multiple regression model,the coefficient of multiple determination can increase.

Question 71

A regression had the following results: SST = 102.55,SSE = 82.04.It can be said that 20.0% of the variation in the dependent variable is explained by the independent variables in the regression.

Question 72

The coefficient of multiple determination is calculated by taking the ratio of the regression sum of squares over the total sum of squares (SSR/SST)and subtracting that value from 1.

Question 73

A regression had the following results: SST = 82.55,SSE = 29.85.It can be said that 63.84% of the variation in the dependent variable is explained by the independent variables in the regression.

Question 74

You have just computed a regression in which the value of coefficient of multiple determination is 0.57.To determine if this indicates that the independent variables explain a significant portion of the variation in the dependent variable,you would perform an F test.

Question 75

When an additional explanatory variable is introduced into a multiple regression model,the adjusted r² can never decrease.

Question 76

The coefficient of multiple determination measures the proportion of the total variation in the dependent variable that is explained by a set of independent variables.

Question 77

The coefficient of multiple determination r²_Y.12

A) measures the proportion of variation in Y that is explained by X₁ and X₂.
B) measures the proportion of variation in Y that is explained by X₁ holding X₂ constant.
C) will have the same sign as b₁.
D) measures the variation around the predicted regression equation.

Question 78

When an explanatory variable is dropped from a multiple regression model,the adjusted r² can increase.

Question 79

Instruction 13.15
An economist is interested to see how consumption for an economy (in $ billions) is influenced by gross domestic product ($ billions) and aggregate price (consumer price index). The Microsoft Excel output of this regression is partially reproduced below.
OUTPUT
SUMMARY
Regression Statistics
$\begin{array} { l l } \text { MultipleR } & 0.991 \\ \text { R Square } & 0.982 \\ \text { Adj. R Square } & 0.976 \\ \text { Std. Error } & 0.299 \\ \text { Observations } & 10 \end{array}$

ANOVA
$\begin{array} { l l l l l l } & d f & \text { SS } & \text { MS } & F & \text { Signiff } \\ \text { Regression } & 2 & 33.4163 & 16.7082 & 186.325 & 0.0001 \\ \text { Residual } & 7 & 0.6277 & 0.0897 & & \\ \text { Total } & 9 & 34.0440 & & & \\ & & & & & \\ & \text { Coeff } & \text { StdError } & t \text { Stat } & p \text { value } & \\ \text { Intercept } & - 1.6335 & 0.5674 & - 0.152 & 0.8837 & \\ \text { GDP } & 0.7654 & 0.0574 & 13.340 & 0.0001 & \\ \text { Price } & - 0.0006 & 0.0028 & - 0.219 & 0.8330 & \end{array}$ Note: Adj. R Square = Adjusted R Square; Std. Error = Standard Error

-Referring to Instruction 13.15,the p-value for the aggregated price index is

A) 0.001.
B) 0.05.
C) 0.01.
D) None of the above.

Question 80

In a multiple regression model,the value of the coefficient of multiple determination

A) can fall between any pair of real numbers.
B) has to fall between -1 and +1.
C) has to fall between -1 and 0.
D) has to fall between 0 and +1.