Deck 3: Polynomial and Rational Functions
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال

فتح الحزمة
قم بالتسجيل لفتح البطاقات في هذه المجموعة!
Unlock Deck
Unlock Deck
1/96
العب
ملء الشاشة (f)
Deck 3: Polynomial and Rational Functions
1
State whether the function is a polynomial function or not. If it is, give its degree. If it is not, tell why not.
A) Yes; degree 1
B) No; x has a fractional coefficient
C) Yes; degree 3
D) Yes; degree 0
A) Yes; degree 1
B) No; x has a fractional coefficient
C) Yes; degree 3
D) Yes; degree 0
A
2

f(x) = (x + 2)5 + 4
 A)
A)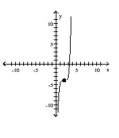
B)
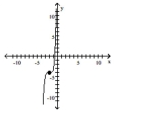
C)
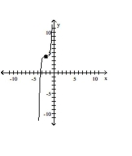
D)
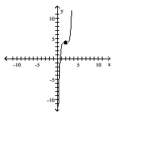
C
3
State whether the function is a polynomial function or not. If it is, give its degree. If it is not, tell why not.
f(x) = 4x + 2x3
A) Yes; degree 3
B) Yes; degree 2
C) Yes; degree 1
D) Yes; degree 4
f(x) = 4x + 2x3
A) Yes; degree 3
B) Yes; degree 2
C) Yes; degree 1
D) Yes; degree 4
A
4
State whether the function is a polynomial function or not. If it is, give its degree. If it is not, tell why not.
A) No; x is a negative term
B) Yes; degree 2
C) Yes; degree 1
D) No; it is a ratio
A) No; x is a negative term
B) Yes; degree 2
C) Yes; degree 1
D) No; it is a ratio

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 96 في هذه المجموعة.
فتح الحزمة
k this deck
5

f(x) = (x + 5)5
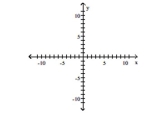 A)
A)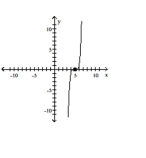
B)
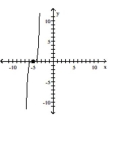
C)
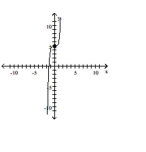
D)
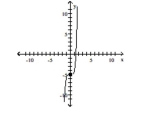

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 96 في هذه المجموعة.
فتح الحزمة
k this deck
6

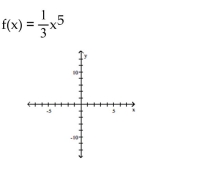 A)
A)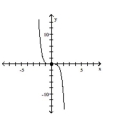
B)
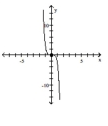
C)
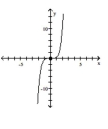
D)
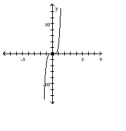

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 96 في هذه المجموعة.
فتح الحزمة
k this deck
7
State whether the function is a polynomial function or not. If it is, give its degree. If it is not, tell why not.
f(x) = 19x5 + 9x4 + 2
A) Yes; degree 9
B) No; the last term has no variable
C) Yes; degree 10
D) Yes; degree 5
f(x) = 19x5 + 9x4 + 2
A) Yes; degree 9
B) No; the last term has no variable
C) Yes; degree 10
D) Yes; degree 5

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 96 في هذه المجموعة.
فتح الحزمة
k this deck
8

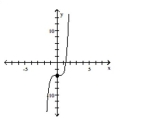
f(x) = x5 + 4
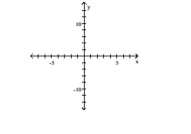 A)
A)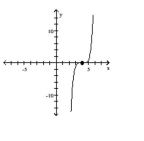
B)
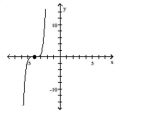
C)
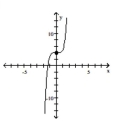
D)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 96 في هذه المجموعة.
فتح الحزمة
k this deck
9

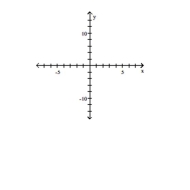 A)
A)
B)
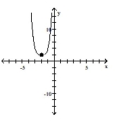
C)
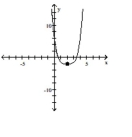
D)
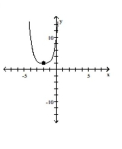

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 96 في هذه المجموعة.
فتح الحزمة
k this deck
10

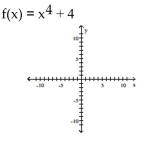 A)
A)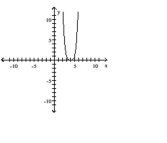
B)
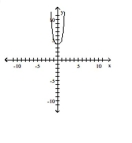
C)
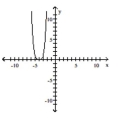
D)
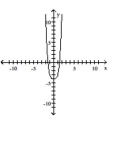

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 96 في هذه المجموعة.
فتح الحزمة
k this deck
11

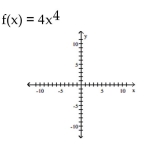 A)
A)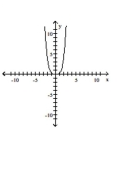
B)
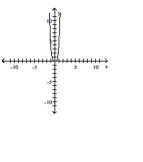
C)
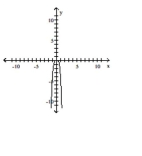
D)
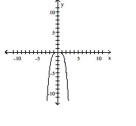

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 96 في هذه المجموعة.
فتح الحزمة
k this deck
12

f(x) = (x - 3)4 + 4
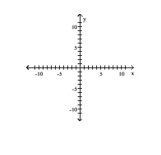 A)
A)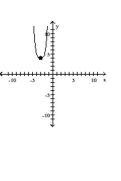
B)
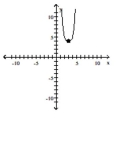
C)
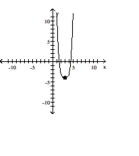
D)
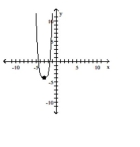

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 96 في هذه المجموعة.
فتح الحزمة
k this deck
13

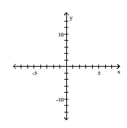
A)
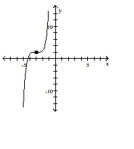
B)
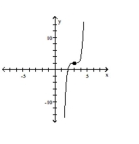
C)
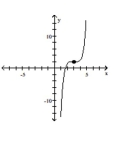
D)
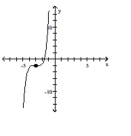

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 96 في هذه المجموعة.
فتح الحزمة
k this deck
14

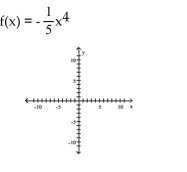 A)
A)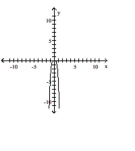
B)
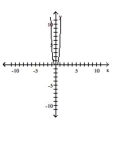
C)
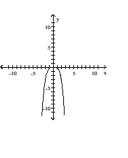
D)
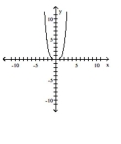

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 96 في هذه المجموعة.
فتح الحزمة
k this deck
15
State whether the function is a polynomial function or not. If it is, give its degree. If it is not, tell why not.
A) Yes; degree 7
B) Yes; degree 0
C) Yes; degree 1
D) No; x is raised to a negative power
A) Yes; degree 7
B) Yes; degree 0
C) Yes; degree 1
D) No; x is raised to a negative power

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 96 في هذه المجموعة.
فتح الحزمة
k this deck
16

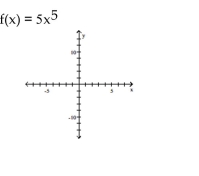 A)
A)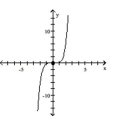
B)
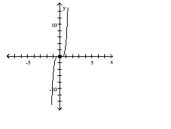
C)
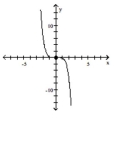
D)
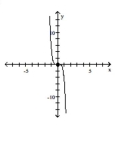

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 96 في هذه المجموعة.
فتح الحزمة
k this deck
17
State whether the function is a polynomial function or not. If it is, give its degree. If it is not, tell why not.
f(x) = x(x - 9)
A) Yes; degree 1
B) Yes; degree 2
C) Yes; degree 0
D) No; it is a product
f(x) = x(x - 9)
A) Yes; degree 1
B) Yes; degree 2
C) Yes; degree 0
D) No; it is a product

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 96 في هذه المجموعة.
فتح الحزمة
k this deck
18

f(x) = 3 - (x - 4)4
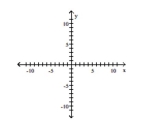 A)
A)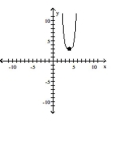
B)
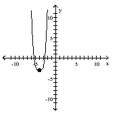
C)
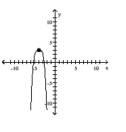
D)


فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 96 في هذه المجموعة.
فتح الحزمة
k this deck
19

f(x) = -2(x - 5)4 + 3
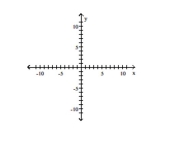 A)
A)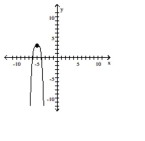
B)
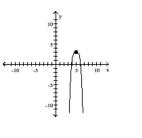
C)
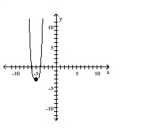
D)
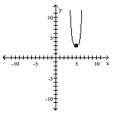

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 96 في هذه المجموعة.
فتح الحزمة
k this deck
20
State whether the function is a polynomial function or not. If it is, give its degree. If it is not, tell why not.
f(x) = 15
A) No; it contains no variables
B) No; it is a constant
C) Yes; degree 1
D) Yes; degree 0
f(x) = 15
A) No; it contains no variables
B) No; it is a constant
C) Yes; degree 1
D) Yes; degree 0

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 96 في هذه المجموعة.
فتح الحزمة
k this deck
21
Determine the maximum number of turning points of f.
f(x) = -x2 (x + 4)3(x2 - 1)
A) 6
B) 2
C) 5
D) 7
f(x) = -x2 (x + 4)3(x2 - 1)
A) 6
B) 2
C) 5
D) 7

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 96 في هذه المجموعة.
فتح الحزمة
k this deck
22
Find the x- and y-intercepts of f.
A) x-intercepts: -intercept: 0
B) x-intercepts: -intercept: 0
C) x-intercepts: -intercept: 6
D) -intercepts: -intercept: 6
A) x-intercepts: -intercept: 0
B) x-intercepts: -intercept: 0
C) x-intercepts: -intercept: 6
D) -intercepts: -intercept: 6

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 96 في هذه المجموعة.
فتح الحزمة
k this deck
23

A) , multiplicity 1 , crosses -axis; , multiplicity 1 , crosses -axis; 2 , multiplicity 2 , touches -axis; , multiplicity 2 , touches -axis
B) -6, multiplicity 1 , touches -axis; , multiplicity 2, crosses -axis
C) -6, multiplicity 1 , crosses -axis; , multiplicity 2 , touches -axis
D) No real zeros

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 96 في هذه المجموعة.
فتح الحزمة
k this deck
24
Determine the maximum number of turning points of f.
A) above the -axis:
below the -axis:
B) above the -axis:
below the -axis:
C) above the -axis:
below the -axis:
D) above the x-axis:
below the -axis:
A) above the -axis:
below the -axis:
B) above the -axis:
below the -axis:
C) above the -axis:
below the -axis:
D) above the x-axis:
below the -axis:

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 96 في هذه المجموعة.
فتح الحزمة
k this deck
25
Find the x- and y-intercepts of f.
A) x-intercepts: -6, 0, 1; y-intercept: -6
B) x-intercepts: -1, 0, 1, 6; y-intercept: 0
C) x-intercepts: -6, -1, 0, 1; y-intercept: 0
D) x-intercepts: -6, -1, 0, 1; y-intercept: -6
A) x-intercepts: -6, 0, 1; y-intercept: -6
B) x-intercepts: -1, 0, 1, 6; y-intercept: 0
C) x-intercepts: -6, -1, 0, 1; y-intercept: 0
D) x-intercepts: -6, -1, 0, 1; y-intercept: -6

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 96 في هذه المجموعة.
فتح الحزمة
k this deck
26
Find the x- and y-intercepts of f.
f(x) = (x - 3)(x - 1)
A) x-intercepts: 3, 1; y-intercept: -4
B) x-intercepts: -3, -1; y-intercept: -4
C) x-intercepts: 3, 1; y-intercept: 3
D) x-intercepts: -3, -1; y-intercept: 3
f(x) = (x - 3)(x - 1)
A) x-intercepts: 3, 1; y-intercept: -4
B) x-intercepts: -3, -1; y-intercept: -4
C) x-intercepts: 3, 1; y-intercept: 3
D) x-intercepts: -3, -1; y-intercept: 3

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 96 في هذه المجموعة.
فتح الحزمة
k this deck
27
Determine the maximum number of turning points of f.
f(x) = 8x - x3
A) 1
B) 3
C) 2
D) 4
f(x) = 8x - x3
A) 1
B) 3
C) 2
D) 4

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 96 في هذه المجموعة.
فتح الحزمة
k this deck
28

A) 0 , multiplicity 2 , touches -axis;
3 , multiplicity 1 , crosses -axis;
, multiplicity 1 , crosses -axis
, multiplicity 1 , crosses -axis
B) 0 , multiplicity 2 , crosses -axis;
3 , multiplicity 1 , touches -axis;
, multiplicity 1 , touches -axis;
, multiplicity 1 , touches -axis
C) 0 , multiplicity 2, crosses x-axis;
3 , multiplicity 1 , touches -axis
D) 0 , multiplicity 2 , touches -axis;
3 , multiplicity 1 , crosses -axis

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 96 في هذه المجموعة.
فتح الحزمة
k this deck
29

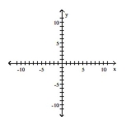
A)
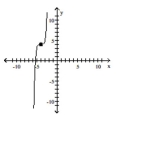
B)
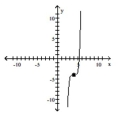
C)
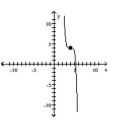
D)
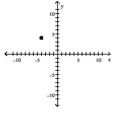

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 96 في هذه المجموعة.
فتح الحزمة
k this deck
30

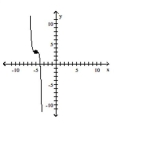
A)
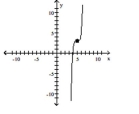
B)
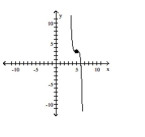
C)
D)
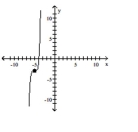

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 96 في هذه المجموعة.
فتح الحزمة
k this deck
31
Find the x- and y-intercepts of f.
A) x-intercepts: 0, -5; y-intercept: 2
B) x-intercepts: 0, 5; y-intercept: 2
C) x-intercepts: 0, -5; y-intercept: 0
D) x-intercepts: 0, 5; y-intercept: 0
A) x-intercepts: 0, -5; y-intercept: 2
B) x-intercepts: 0, 5; y-intercept: 2
C) x-intercepts: 0, -5; y-intercept: 0
D) x-intercepts: 0, 5; y-intercept: 0

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 96 في هذه المجموعة.
فتح الحزمة
k this deck
32
Determine the maximum number of turning points of f.
f(x) = (x - 3)2(x + 5)2
A) 3
B) 1
C) 4
D) 2
f(x) = (x - 3)2(x + 5)2
A) 3
B) 1
C) 4
D) 2

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 96 في هذه المجموعة.
فتح الحزمة
k this deck
33
Find the x- and y-intercepts of f.
A) x-intercepts: -6, -1, 0; y-intercept: 6
B) x-intercepts: -6, -1, 0; y-intercept: -6
C) x-intercepts: -6, -1, 0, 1; y-intercept: 0
D) x-intercepts: -6, 0; y-intercept: 0
A) x-intercepts: -6, -1, 0; y-intercept: 6
B) x-intercepts: -6, -1, 0; y-intercept: -6
C) x-intercepts: -6, -1, 0, 1; y-intercept: 0
D) x-intercepts: -6, 0; y-intercept: 0

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 96 في هذه المجموعة.
فتح الحزمة
k this deck
34
Determine the maximum number of turning points of f.
Answer: (a) For large values of , the graph of will resemble the graph of .
(b) -intercept: , -intercepts: and
(c) The graph of crosses the -axis at and touches the -axis at .
(e) Local minimum at , Local maximum at
(f)
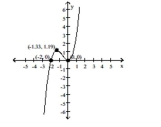
(g) Domain of f: all real numbers; range of f: all real numbers
(h) f is increasing on (-, -1.33) and (0, ); f is decreasing on (-1.33, 0)
Answer: (a) For large values of , the graph of will resemble the graph of .
(b) -intercept: , -intercepts: and
(c) The graph of crosses the -axis at and touches the -axis at .
(e) Local minimum at , Local maximum at
(f)

(g) Domain of f: all real numbers; range of f: all real numbers
(h) f is increasing on (-, -1.33) and (0, ); f is decreasing on (-1.33, 0)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 96 في هذه المجموعة.
فتح الحزمة
k this deck
35

A) 0 , multiplicity 4 , touches -axis;
, multiplicity 1 , crosses -axis;
, multiplicity 1 , crosses -axis
B) 0 , multiplicity 4 , crosses -axis
C) 0 , multiplicity 4 , crosses -axis;
, multiplicity 1 , touches -axis;
D) 0 , multiplicity 4 , touches -axis
, multiplicity 1 , touches -axis

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 96 في هذه المجموعة.
فتح الحزمة
k this deck
36
Determine the maximum number of turning points of f.
A) above the -axis:
below the -axis:
B) above the -axis:
below the -axis: no intervals
C) above the x-axis: no intervals
below the -axis:
D) above the -axis:
below the -axis:
A) above the -axis:
below the -axis:
B) above the -axis:
below the -axis: no intervals
C) above the x-axis: no intervals
below the -axis:
D) above the -axis:
below the -axis:

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 96 في هذه المجموعة.
فتح الحزمة
k this deck
37
Find the x- and y-intercepts of f.
A) x-intercepts: -3, 3, 5; y-intercept: -45
B) x-intercepts: -5, -3, 3; y-intercept: -45
C) x-intercepts: -5, -3, 3; y-intercept: 45
D) x-intercepts: -3, 3, 5; y-intercept: 45
A) x-intercepts: -3, 3, 5; y-intercept: -45
B) x-intercepts: -5, -3, 3; y-intercept: -45
C) x-intercepts: -5, -3, 3; y-intercept: 45
D) x-intercepts: -3, 3, 5; y-intercept: 45

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 96 في هذه المجموعة.
فتح الحزمة
k this deck
38
Find the x- and y-intercepts of f.
A) x-intercepts: -1, 1, -2; y-intercept: 2
B) x-intercepts: -1, 1, 2; y-intercept: 2
C) x-intercepts: -1, 1, -2; y-intercept: -2
D) x-intercepts: -1, 1, 2; y-intercept: -2
A) x-intercepts: -1, 1, -2; y-intercept: 2
B) x-intercepts: -1, 1, 2; y-intercept: 2
C) x-intercepts: -1, 1, -2; y-intercept: -2
D) x-intercepts: -1, 1, 2; y-intercept: -2

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 96 في هذه المجموعة.
فتح الحزمة
k this deck
39
Find the x- and y-intercepts of f.
A) x-intercept: -6; y-intercept: 36
B) x-intercept: -6; y-intercept: 0
C) x-intercept: 6; y-intercept: 36
D) x-intercept: 6; y-intercept: 0
A) x-intercept: -6; y-intercept: 36
B) x-intercept: -6; y-intercept: 0
C) x-intercept: 6; y-intercept: 36
D) x-intercept: 6; y-intercept: 0

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 96 في هذه المجموعة.
فتح الحزمة
k this deck
40
Determine the maximum number of turning points of f.
A) above the -axis:
below the x-axis:
B) above the x-axis:
below the -axis:
C) above the -axis:
below the -axis: no intervals
D) above the -axis: no intervals
below the -axis:
A) above the -axis:
below the x-axis:
B) above the x-axis:
below the -axis:
C) above the -axis:
below the -axis: no intervals
D) above the -axis: no intervals
below the -axis:

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 96 في هذه المجموعة.
فتح الحزمة
k this deck
41
Use Descartes' Rule of Signs and the Rational Zeros Theorem to find all the real zeros of the polynomial function. Use the
zeros to factor f over the real numbers.
A)
B)
C)
D)
zeros to factor f over the real numbers.
A)
B)
C)
D)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 96 في هذه المجموعة.
فتح الحزمة
k this deck
42
List the potential rational zeros of the polynomial function. Do not find the zeros.
A)
B)
C)
D)
A)
B)
C)
D)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 96 في هذه المجموعة.
فتح الحزمة
k this deck
43
Find the x- and y-intercepts of f.
A) x-intercept: 13; y-intercept: 169
B) x-intercept: -13; y-intercept: 0
C) x-intercept: -13; y-intercept: 169
D) x-intercept: 13; y-intercept: 0
A) x-intercept: 13; y-intercept: 169
B) x-intercept: -13; y-intercept: 0
C) x-intercept: -13; y-intercept: 169
D) x-intercept: 13; y-intercept: 0

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 96 في هذه المجموعة.
فتح الحزمة
k this deck
44
List the potential rational zeros of the polynomial function. Do not find the zeros.
A)
B)
C)
D)
A)
B)
C)
D)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 96 في هذه المجموعة.
فتح الحزمة
k this deck
45
List the potential rational zeros of the polynomial function. Do not find the zeros.
A)
B)
C)
D)
A)
B)
C)
D)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 96 في هذه المجموعة.
فتح الحزمة
k this deck
46
Solve the problem.
The profits (in millions) for a company for 8 years was as follows: Find the cubic function of best fit to the data.
The profits (in millions) for a company for 8 years was as follows: Find the cubic function of best fit to the data.

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 96 في هذه المجموعة.
فتح الحزمة
k this deck
47
Solve the problem.
For the polynomial funct a) Find the x- and y-intercepts of the graph of f. Round to two decimal places, if necessary.
b) Determine whether the graph crosses or touches the x-axis at each x-intercept.
c) End behavior: find the power function that the graph of f resembles for large values of |x|.
d) Use a graphing utility to graph the function.Approximate the local maxima rounded to two decimal places,
if necessary. Approximate the local minima rounded to two decimal places, if necessary.
e) Determine the number of turning points on the graph.
f) Put all the information together, and connect the points with a smooth, continuous curve to obtain the
graph of f.
For the polynomial funct a) Find the x- and y-intercepts of the graph of f. Round to two decimal places, if necessary.
b) Determine whether the graph crosses or touches the x-axis at each x-intercept.
c) End behavior: find the power function that the graph of f resembles for large values of |x|.
d) Use a graphing utility to graph the function.Approximate the local maxima rounded to two decimal places,
if necessary. Approximate the local minima rounded to two decimal places, if necessary.
e) Determine the number of turning points on the graph.
f) Put all the information together, and connect the points with a smooth, continuous curve to obtain the
graph of f.


فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 96 في هذه المجموعة.
فتح الحزمة
k this deck
48
Find the x- and y-intercepts of f.
f(x) = (x + 6)(x - 2)(x + 2)
A) x-intercepts: -6, -2, 2; y-intercept: -24
B) x-intercepts: -2, 2, 6; y-intercept: 24
C) x-intercepts: -2, 2, 6; y-intercept: -24
D) x-intercepts: -6, -2, 2; y-intercept: 24
f(x) = (x + 6)(x - 2)(x + 2)
A) x-intercepts: -6, -2, 2; y-intercept: -24
B) x-intercepts: -2, 2, 6; y-intercept: 24
C) x-intercepts: -2, 2, 6; y-intercept: -24
D) x-intercepts: -6, -2, 2; y-intercept: 24

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 96 في هذه المجموعة.
فتح الحزمة
k this deck
49
Use Descartes' Rule of Signs and the Rational Zeros Theorem to find all the real zeros of the polynomial function. Use the
zeros to factor f over the real numbers.
A)
B)
C)
D)
zeros to factor f over the real numbers.
A)
B)
C)
D)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 96 في هذه المجموعة.
فتح الحزمة
k this deck
50
Use Descartes' Rule of Signs and the Rational Zeros Theorem to find all the real zeros of the polynomial function. Use the
zeros to factor f over the real numbers.
A)
B)
C)
D)
zeros to factor f over the real numbers.
A)
B)
C)
D)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 96 في هذه المجموعة.
فتح الحزمة
k this deck
51
Find the x- and y-intercepts of f.
f(x) = 4x2 (x - 4)5
A) x-intercepts: 0, -4; y-intercept: 0
B) x-intercepts: 0, 4; y-intercept: 4
C) x-intercepts: 0, -4; y-intercept: 4
D) x-intercepts: 0, 4; y-intercept: 0
f(x) = 4x2 (x - 4)5
A) x-intercepts: 0, -4; y-intercept: 0
B) x-intercepts: 0, 4; y-intercept: 4
C) x-intercepts: 0, -4; y-intercept: 4
D) x-intercepts: 0, 4; y-intercept: 0

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 96 في هذه المجموعة.
فتح الحزمة
k this deck
52
Solve the problem.
The amount of water (in gallons) in a leaky bathtub is given in the table below. Using a graphing utility, fit the data to a third degree polynomial (or a cubic). Then approximate the time at which there is maximum amount
Of water in the tub, and estimate the time when the water runs out of the tub. Express all your answers rounded
To two decimal places.
A) maximum amount of water after 8.23 minutes; water runs out after 19.73 minutes
B) maximum amount of water after 5.31 minutes; water runs out after 8.23 minutes
C) maximum amount of water after 5.37 minutes; water runs out after 11.06 minutes
D) maximum amount of water after 5.31 minutes; water never runs out
The amount of water (in gallons) in a leaky bathtub is given in the table below. Using a graphing utility, fit the data to a third degree polynomial (or a cubic). Then approximate the time at which there is maximum amount
Of water in the tub, and estimate the time when the water runs out of the tub. Express all your answers rounded
To two decimal places.
A) maximum amount of water after 8.23 minutes; water runs out after 19.73 minutes
B) maximum amount of water after 5.31 minutes; water runs out after 8.23 minutes
C) maximum amount of water after 5.37 minutes; water runs out after 11.06 minutes
D) maximum amount of water after 5.31 minutes; water never runs out

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 96 في هذه المجموعة.
فتح الحزمة
k this deck
53
List the potential rational zeros of the polynomial function. Do not find the zeros.
A)
B)
C)
D)
A)
B)
C)
D)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 96 في هذه المجموعة.
فتح الحزمة
k this deck
54
List the potential rational zeros of the polynomial function. Do not find the zeros.
A)
B) ,
C)
D)
A)
B) ,
C)
D)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 96 في هذه المجموعة.
فتح الحزمة
k this deck
55
Solve the problem.
Which of the following polynomial functions might have the graph shown in the illustration below?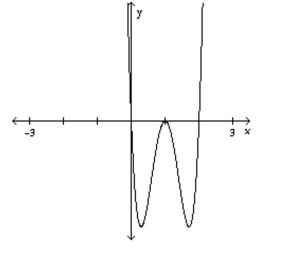
A)
B)
C)
D)
Which of the following polynomial functions might have the graph shown in the illustration below?

A)
B)
C)
D)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 96 في هذه المجموعة.
فتح الحزمة
k this deck
56
Use Descartes' Rule of Signs and the Rational Zeros Theorem to find all the real zeros of the polynomial function. Use the
zeros to factor f over the real numbers.
A)
B)
C)
D)
zeros to factor f over the real numbers.
A)
B)
C)
D)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 96 في هذه المجموعة.
فتح الحزمة
k this deck
57
List the potential rational zeros of the polynomial function. Do not find the zeros.
A)
B)
C)
D)
A)
B)
C)
D)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 96 في هذه المجموعة.
فتح الحزمة
k this deck
58
List the potential rational zeros of the polynomial function. Do not find the zeros.
A)
B)
C)
D)
A)
B)
C)
D)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 96 في هذه المجموعة.
فتح الحزمة
k this deck
59
Use Descartes' Rule of Signs and the Rational Zeros Theorem to find all the real zeros of the polynomial function. Use the
zeros to factor f over the real numbers.
A)
B) no real roots;
C) 3 , multiplicity
D) , multiplicity
zeros to factor f over the real numbers.
A)
B) no real roots;
C) 3 , multiplicity
D) , multiplicity

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 96 في هذه المجموعة.
فتح الحزمة
k this deck
60
Use Descartes' Rule of Signs and the Rational Zeros Theorem to find all the real zeros of the polynomial function. Use the
zeros to factor f over the real numbers.
A)
B)
C)
D)
zeros to factor f over the real numbers.
A)
B)
C)
D)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 96 في هذه المجموعة.
فتح الحزمة
k this deck
61
The equation has a solution r in the interval indicated. Approximate this solution correct to two decimal places.
x3 - 8x - 3 = 0; -1 ≤ r ≤ 0
x3 - 8x - 3 = 0; -1 ≤ r ≤ 0

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 96 في هذه المجموعة.
فتح الحزمة
k this deck
62
Find the x- and y-intercepts of f.
f(x) = 6x - x3
A) x-intercepts: 0, -6; y-intercept: 0
B) x-intercepts: 0, 6, -6; y-intercept: 0
C) x-intercepts: 0, 6, - 6; y-intercept: 6
D) x-intercepts: 0, -6; y-intercept: 6
f(x) = 6x - x3
A) x-intercepts: 0, -6; y-intercept: 0
B) x-intercepts: 0, 6, -6; y-intercept: 0
C) x-intercepts: 0, 6, - 6; y-intercept: 6
D) x-intercepts: 0, -6; y-intercept: 6

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 96 في هذه المجموعة.
فتح الحزمة
k this deck
63
Find the x- and y-intercepts of f.
f(x) = (x - 2)2(x2 - 9)
A) x-intercepts: -3, 2, 3; y-intercept: 36
B) x-intercepts: -2, -9; y-intercept: 18
C) x-intercepts: 2, 9; y-intercept: 18
D) x-intercepts: -3, 2, 3; y-intercept: -36
f(x) = (x - 2)2(x2 - 9)
A) x-intercepts: -3, 2, 3; y-intercept: 36
B) x-intercepts: -2, -9; y-intercept: 18
C) x-intercepts: 2, 9; y-intercept: 18
D) x-intercepts: -3, 2, 3; y-intercept: -36

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 96 في هذه المجموعة.
فتح الحزمة
k this deck
64
Solve the equation in the real number system.
A)
B)
C)
D)
A)
B)
C)
D)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 96 في هذه المجموعة.
فتح الحزمة
k this deck
65
Solve the equation in the real number system.
A) {-4, -2}
B) {2, 4}
C) {-3, 2, 4}
D) {-4, -2, 3}
A) {-4, -2}
B) {2, 4}
C) {-3, 2, 4}
D) {-4, -2, 3}

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 96 في هذه المجموعة.
فتح الحزمة
k this deck
66
Find the x- and y-intercepts of f.
f(x) = -x2 (x + 7)(x2 + 1)
A) x-intercepts: -7, 0; y-intercept: 0
B) x-intercepts: -7, -1, 0; y-intercept: 7
C) x-intercepts: -7, -1, 0, 1; y-intercept: 0
D) x-intercepts: -7, -1, 0; y-intercept: -7
f(x) = -x2 (x + 7)(x2 + 1)
A) x-intercepts: -7, 0; y-intercept: 0
B) x-intercepts: -7, -1, 0; y-intercept: 7
C) x-intercepts: -7, -1, 0, 1; y-intercept: 0
D) x-intercepts: -7, -1, 0; y-intercept: -7

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 96 في هذه المجموعة.
فتح الحزمة
k this deck
67
Solve the equation in the real number system.
A) {1}
B) {10}
C) {-10, 10}
D) {-10}
A) {1}
B) {10}
C) {-10, 10}
D) {-10}

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 96 في هذه المجموعة.
فتح الحزمة
k this deck
68
The equation has a solution r in the interval indicated. Approximate this solution correct to two decimal places.
x3 - 8x - 3 = 0; -3 ≤ r ≤-2
x3 - 8x - 3 = 0; -3 ≤ r ≤-2

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 96 في هذه المجموعة.
فتح الحزمة
k this deck
69
Solve the equation in the real number system.
A)
B)
C)
D)
A)
B)
C)
D)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 96 في هذه المجموعة.
فتح الحزمة
k this deck
70
Solve the equation in the real number system.
A)
B)
C)
D)
A)
B)
C)
D)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 96 في هذه المجموعة.
فتح الحزمة
k this deck
71
Solve the equation in the real number system.
A) {-8, 8}
B) {-4, 4}
C) {-2, 2}
D) {-4, -2, 2, 4}
A) {-8, 8}
B) {-4, 4}
C) {-2, 2}
D) {-4, -2, 2, 4}

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 96 في هذه المجموعة.
فتح الحزمة
k this deck
72
Find the x- and y-intercepts of f.
f(x) = x2 (x - 1)(x - 3)
A) x-intercepts: 0, -1, -3; y-intercept: 3
B) x-intercepts: 0, 1, 3; y-intercept: 3
C) x-intercepts: 0, 1, 3; y-intercept: 0
D) x-intercepts: 0, -1, -3; y-intercept: 0
f(x) = x2 (x - 1)(x - 3)
A) x-intercepts: 0, -1, -3; y-intercept: 3
B) x-intercepts: 0, 1, 3; y-intercept: 3
C) x-intercepts: 0, 1, 3; y-intercept: 0
D) x-intercepts: 0, -1, -3; y-intercept: 0

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 96 في هذه المجموعة.
فتح الحزمة
k this deck
73
Solve the equation in the real number system.
A) {-1, 2}
B) {-1, -2}
C) {1, 2}
D) {-2, 1}
A) {-1, 2}
B) {-1, -2}
C) {1, 2}
D) {-2, 1}

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 96 في هذه المجموعة.
فتح الحزمة
k this deck
74
The equation has a solution r in the interval indicated. Approximate this solution correct to two decimal places.
x4 - x3 - 7x2 + 5x + 10 = 0; -3 ≤ r ≤-2
x4 - x3 - 7x2 + 5x + 10 = 0; -3 ≤ r ≤-2

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 96 في هذه المجموعة.
فتح الحزمة
k this deck
75
Find the x- and y-intercepts of f.
f(x) = (x + 1)(x - 4)(x - 1)2
A) x-intercepts: -1, 1, -4; y-intercept: -4
B) x-intercepts: -1, 1, 4; y-intercept: 4
C) x-intercepts: -1, 1, 4; y-intercept: -4
D) x-intercepts: -1, 1, -4; y-intercept: 4
f(x) = (x + 1)(x - 4)(x - 1)2
A) x-intercepts: -1, 1, -4; y-intercept: -4
B) x-intercepts: -1, 1, 4; y-intercept: 4
C) x-intercepts: -1, 1, 4; y-intercept: -4
D) x-intercepts: -1, 1, -4; y-intercept: 4

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 96 في هذه المجموعة.
فتح الحزمة
k this deck
76
Find the x- and y-intercepts of f.
f(x) = -x2 (x + 2)(x2 - 1)
A) x-intercepts: -2, 0, 1; y-intercept: -2
B) x-intercepts: -2, -1, 0, 1; y-intercept: -2
C) x-intercepts: -1, 0, 1, 2; y-intercept: 0
D) x-intercepts: -2, -1, 0, 1; y-intercept: 0
f(x) = -x2 (x + 2)(x2 - 1)
A) x-intercepts: -2, 0, 1; y-intercept: -2
B) x-intercepts: -2, -1, 0, 1; y-intercept: -2
C) x-intercepts: -1, 0, 1, 2; y-intercept: 0
D) x-intercepts: -2, -1, 0, 1; y-intercept: 0

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 96 في هذه المجموعة.
فتح الحزمة
k this deck
77
Find the x- and y-intercepts of f.
f(x) = (x - 2)(x - 5)
A) x-intercepts: -2, -5; y-intercept: 10
B) x-intercepts: 2, 5; y-intercept: -7
C) x-intercepts: 2, 5; y-intercept: 10
D) x-intercepts: -2, -5; y-intercept: -7
f(x) = (x - 2)(x - 5)
A) x-intercepts: -2, -5; y-intercept: 10
B) x-intercepts: 2, 5; y-intercept: -7
C) x-intercepts: 2, 5; y-intercept: 10
D) x-intercepts: -2, -5; y-intercept: -7

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 96 في هذه المجموعة.
فتح الحزمة
k this deck
78
Solve the equation in the real number system.
A)
B)
C)
D)
A)
B)
C)
D)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 96 في هذه المجموعة.
فتح الحزمة
k this deck
79
Solve the equation in the real number system.
A) {-1, 4}
B) {-1, 1}
C) {-4, 4}
D) {-4, 1}
A) {-1, 4}
B) {-1, 1}
C) {-4, 4}
D) {-4, 1}

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 96 في هذه المجموعة.
فتح الحزمة
k this deck
80
Solve the equation in the real number system.
A)
B)
C)
D)
A)
B)
C)
D)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 96 في هذه المجموعة.
فتح الحزمة
k this deck








