Deck 4: Continuous Random Variables and Probability Distributions
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال

فتح الحزمة
قم بالتسجيل لفتح البطاقات في هذه المجموعة!
Unlock Deck
Unlock Deck
1/17
العب
ملء الشاشة (f)
Deck 4: Continuous Random Variables and Probability Distributions
1
The distribution of resistance for resistors of a certain type is known to be normal, with 10% of all resistors having a resistance exceeding 10.634 ohms, and 5% having a resistance smaller than 9.7565 ohms. What are the mean value and standard deviation of the resistance distribution?
Since 1.28 is the 90th z percentile 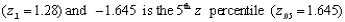 , the given information implies that
, the given information implies that 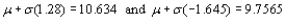 , from which
, from which 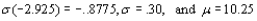
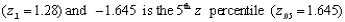 , the given information implies that
, the given information implies that 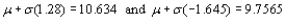 , from which
, from which 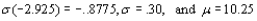
2
Let X = hourly median power (in decibels) of received radio signals transmitted between two cities. It is believed that the lognormal distribution provides a reasonable probability model for X. If the parameter values are 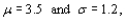 calculate the following:
calculate the following:
a. The mean value and standard deviation of received power
b. The probability that received power is between 50 and 250 dB
c. The probability that X is less than its mean value. Why is this probability not .5?
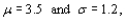 calculate the following:
calculate the following: a. The mean value and standard deviation of received power
b. The probability that received power is between 50 and 250 dB
c. The probability that X is less than its mean value. Why is this probability not .5?
a. 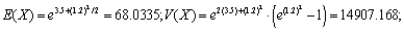 and
and 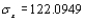 b.
b. 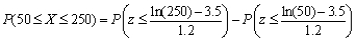
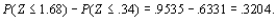 c.
c. 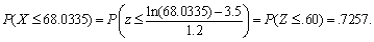 The lognormal distribution is not a symmetric distribution.
The lognormal distribution is not a symmetric distribution.
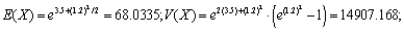 and
and 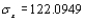 b.
b. 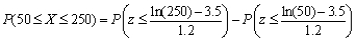
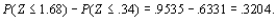 c.
c. 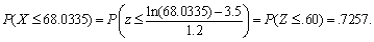 The lognormal distribution is not a symmetric distribution.
The lognormal distribution is not a symmetric distribution. 3
Suppose that when a transistor of a certain type is subjected to an accelerated life test, the lifetime X (in weeks) has a gamma distribution with mean of 40 and variance of 320.
a. What is the probability that a transistor will last between 1 and 40 weeks?
b. What is the probability that a transistor will last at most 40 weeks? Is the median of the lifetime distribution less than 40? Why or why not?
a. What is the probability that a transistor will last between 1 and 40 weeks?
b. What is the probability that a transistor will last at most 40 weeks? Is the median of the lifetime distribution less than 40? Why or why not?
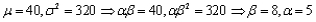 a.
a. 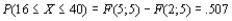 b.
b. 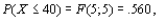 so while the mean is 40, the median is less than 40.
so while the mean is 40, the median is less than 40. 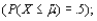 This is the result of the positive skew of the gamma distribution.
This is the result of the positive skew of the gamma distribution. 4
Let X = the time between two successive arrivals at the drive-up window of a local bank. If X has an exponential distribution with 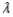 = 1 (which is identical to a standard gamma distribution with
= 1 (which is identical to a standard gamma distribution with 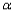 =1), compute the following:
=1), compute the following:
a. The expected time between two successive arrivals.
b. The standard deviation of the time between two successive arrivals.
c.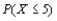
.
d.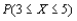
.
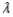 = 1 (which is identical to a standard gamma distribution with
= 1 (which is identical to a standard gamma distribution with 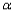 =1), compute the following:
=1), compute the following: a. The expected time between two successive arrivals.
b. The standard deviation of the time between two successive arrivals.
c.
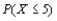
.
d.
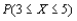
.

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 17 في هذه المجموعة.
فتح الحزمة
k this deck
5
The time X (minutes) for a lab assistant to prepare the equipment for a certain experiment is believed to have a uniform distribution with A = 20 and B = 30.
a. Write the pdf of X and sketch its graph.
b. What is the probability that preparation time exceeds 27 minutes?
c. Find the preparation mean time, then calculate the probability that preparation is within 2 minutes of the mean time?
d. For any a such that 20 < a < a + 2 < 30, what is the probability that preparation time is between a and a + 2 minutes?
a. Write the pdf of X and sketch its graph.
b. What is the probability that preparation time exceeds 27 minutes?
c. Find the preparation mean time, then calculate the probability that preparation is within 2 minutes of the mean time?
d. For any a such that 20 < a < a + 2 < 30, what is the probability that preparation time is between a and a + 2 minutes?

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 17 في هذه المجموعة.
فتح الحزمة
k this deck
6
Let X have a uniform distribution on the interval [a, b].
a. Obtain an expression for the (100p) th percentile.
b. Compute E(X), V(X), and![Let X have a uniform distribution on the interval [a, b]. a. Obtain an expression for the (100p) th percentile. b. Compute E(X), V(X), and . c. For n a positive integer, compute .](https://d2lvgg3v3hfg70.cloudfront.net/TB3498/11eb0e05_3e36_18a0_9431_3189cf507616_TB3498_11.jpg)
.
c. For n a positive integer, compute![Let X have a uniform distribution on the interval [a, b]. a. Obtain an expression for the (100p) th percentile. b. Compute E(X), V(X), and . c. For n a positive integer, compute .](https://d2lvgg3v3hfg70.cloudfront.net/TB3498/11eb0e05_3e36_18a1_9431_15fee2edef9b_TB3498_11.jpg)
.
a. Obtain an expression for the (100p) th percentile.
b. Compute E(X), V(X), and
![Let X have a uniform distribution on the interval [a, b]. a. Obtain an expression for the (100p) th percentile. b. Compute E(X), V(X), and . c. For n a positive integer, compute .](https://d2lvgg3v3hfg70.cloudfront.net/TB3498/11eb0e05_3e36_18a0_9431_3189cf507616_TB3498_11.jpg)
.
c. For n a positive integer, compute
![Let X have a uniform distribution on the interval [a, b]. a. Obtain an expression for the (100p) th percentile. b. Compute E(X), V(X), and . c. For n a positive integer, compute .](https://d2lvgg3v3hfg70.cloudfront.net/TB3498/11eb0e05_3e36_18a1_9431_15fee2edef9b_TB3498_11.jpg)
.

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 17 في هذه المجموعة.
فتح الحزمة
k this deck
7
Let X be the temperature in 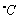 at which a certain chemical reaction takes place, and let Y be the temperature in
at which a certain chemical reaction takes place, and let Y be the temperature in 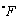 (so Y = 1.8X + 32).
(so Y = 1.8X + 32).
a. If the median of the X distribution is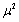
, show that 1.8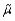
+ 32 is the median of the Y distribution.
b. How is the 90th percentile of the Y distribution related to the 90th percentile of the X distribution? Verify your conjecture.
c. More generally, if Y = aX + b, how is any particular percentile of the Y distribution related to the corresponding percentile of the X distribution?
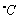 at which a certain chemical reaction takes place, and let Y be the temperature in
at which a certain chemical reaction takes place, and let Y be the temperature in 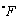 (so Y = 1.8X + 32).
(so Y = 1.8X + 32). a. If the median of the X distribution is
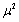
, show that 1.8
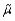
+ 32 is the median of the Y distribution.
b. How is the 90th percentile of the Y distribution related to the 90th percentile of the X distribution? Verify your conjecture.
c. More generally, if Y = aX + b, how is any particular percentile of the Y distribution related to the corresponding percentile of the X distribution?

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 17 في هذه المجموعة.
فتح الحزمة
k this deck
8
Let X denote the amount of time for which a book on 2-hour reserve at a college-library is checked out by a randomly selected student and suppose that X 
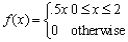 Calculate the following probabilities:
Calculate the following probabilities:
a.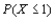
b.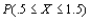

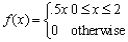 Calculate the following probabilities:
Calculate the following probabilities: a.
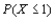
b.
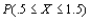

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 17 في هذه المجموعة.
فتح الحزمة
k this deck
9
"Time headway" in traffic flow is the elapsed time between the time that one car finishes passing a fixed point and the instant that the next car begins to pass that point. Let X = the time headway for two randomly chosen consecutive cars on a freeway during a period of heavy flow. The following pdf of X is 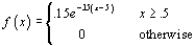 What is the probability that the time headway is
What is the probability that the time headway is
a. At most 6 seconds?
b. At least 6 seconds?
c. At most 5 seconds?
d. Between 5 and 6 seconds?
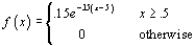 What is the probability that the time headway is
What is the probability that the time headway is a. At most 6 seconds?
b. At least 6 seconds?
c. At most 5 seconds?
d. Between 5 and 6 seconds?

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 17 في هذه المجموعة.
فتح الحزمة
k this deck
10
Suppose only 40% of all drivers in Florida regularly wear a seatbelt. A random sample of 500 drivers is selected. What is the probability that
a. Between 170 and 220 (inclusive) of the drivers in the sample regularly wear a seatbelt?
b. Fewer than 175 of those in the sample regularly wear a seatbelt?
a. Between 170 and 220 (inclusive) of the drivers in the sample regularly wear a seatbelt?
b. Fewer than 175 of those in the sample regularly wear a seatbelt?

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 17 في هذه المجموعة.
فتح الحزمة
k this deck
11
Let X have a binomial distribution with parameters n = 25 and p. Calculate each of the following probabilities using the normal approximation (with the continuity correction) for the cases p = .5 and .6, and compare to the exact probabilities calculated from the cumulative binomial probabilities table available in your text.
a.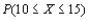
b.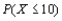
c.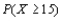
a.
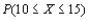
b.
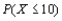
c.
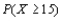

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 17 في هذه المجموعة.
فتح الحزمة
k this deck
12
The cdf of checkout duration X for a book on a 2-hour reserve at a college library is given by: 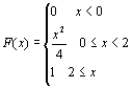 Use this cdf to compute the following:
Use this cdf to compute the following:
a.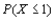
b.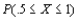
c.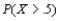
d. The median checkout duration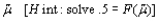
e.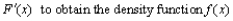
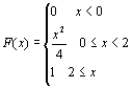 Use this cdf to compute the following:
Use this cdf to compute the following: a.
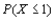
b.
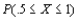
c.
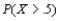
d. The median checkout duration
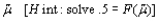
e.
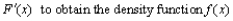

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 17 في هذه المجموعة.
فتح الحزمة
k this deck
13
Suppose the proportion X of surface area in a randomly selected quadrat that is covered by a certain plant has a standard beta distribution with 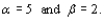
a. Compute E(X) and V(X).
b. Compute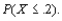
c. Compute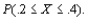
d. What is the expected proportion of the sampling region not covered by the plant?
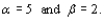
a. Compute E(X) and V(X).
b. Compute
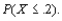
c. Compute
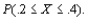
d. What is the expected proportion of the sampling region not covered by the plant?

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 17 في هذه المجموعة.
فتح الحزمة
k this deck
14
The lifetime X (in hundreds of hours) of a certain type of vacuum tube has a Weibull distribution with parameters 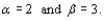 Compute the following:
Compute the following:
a. E(X) and V(X)
b.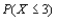
c.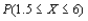
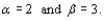 Compute the following:
Compute the following: a. E(X) and V(X)
b.
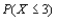
c.
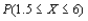

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 17 في هذه المجموعة.
فتح الحزمة
k this deck
15
A college professor always finishes his lectures within 2 minutes after the bell rings to end the period and the end of the lecture. Let X = the time that elapses between the bell and the end of the lecture and suppose the pdf of X is ![A college professor always finishes his lectures within 2 minutes after the bell rings to end the period and the end of the lecture. Let X = the time that elapses between the bell and the end of the lecture and suppose the pdf of X is a. Find the value of k. [Hint: Total area under the graph of f(x) is 1.] b. What is the probability that the lecture ends within 1minutes of the bell ringing? c. What is the probability that the lecture continues beyond the bell for between 60 and 90 seconds? d. What is the probability that the lecture continues for at least 90 seconds beyond the bell?](https://d2lvgg3v3hfg70.cloudfront.net/TB3498/11eb0e05_3e34_6ad2_9431_3df6b4c5fe4b_TB3498_11.jpg)
![A college professor always finishes his lectures within 2 minutes after the bell rings to end the period and the end of the lecture. Let X = the time that elapses between the bell and the end of the lecture and suppose the pdf of X is a. Find the value of k. [Hint: Total area under the graph of f(x) is 1.] b. What is the probability that the lecture ends within 1minutes of the bell ringing? c. What is the probability that the lecture continues beyond the bell for between 60 and 90 seconds? d. What is the probability that the lecture continues for at least 90 seconds beyond the bell?](https://d2lvgg3v3hfg70.cloudfront.net/TB3498/11eb0e05_3e34_6ad3_9431_637d31f2793a_TB3498_11.jpg)
a. Find the value of k. [Hint: Total area under the graph of f(x) is 1.]
b. What is the probability that the lecture ends within 1minutes of the bell ringing?
c. What is the probability that the lecture continues beyond the bell for between 60 and 90 seconds?
d. What is the probability that the lecture continues for at least 90 seconds beyond the bell?
![A college professor always finishes his lectures within 2 minutes after the bell rings to end the period and the end of the lecture. Let X = the time that elapses between the bell and the end of the lecture and suppose the pdf of X is a. Find the value of k. [Hint: Total area under the graph of f(x) is 1.] b. What is the probability that the lecture ends within 1minutes of the bell ringing? c. What is the probability that the lecture continues beyond the bell for between 60 and 90 seconds? d. What is the probability that the lecture continues for at least 90 seconds beyond the bell?](https://d2lvgg3v3hfg70.cloudfront.net/TB3498/11eb0e05_3e34_6ad2_9431_3df6b4c5fe4b_TB3498_11.jpg)
![A college professor always finishes his lectures within 2 minutes after the bell rings to end the period and the end of the lecture. Let X = the time that elapses between the bell and the end of the lecture and suppose the pdf of X is a. Find the value of k. [Hint: Total area under the graph of f(x) is 1.] b. What is the probability that the lecture ends within 1minutes of the bell ringing? c. What is the probability that the lecture continues beyond the bell for between 60 and 90 seconds? d. What is the probability that the lecture continues for at least 90 seconds beyond the bell?](https://d2lvgg3v3hfg70.cloudfront.net/TB3498/11eb0e05_3e34_6ad3_9431_637d31f2793a_TB3498_11.jpg)
a. Find the value of k. [Hint: Total area under the graph of f(x) is 1.]
b. What is the probability that the lecture ends within 1minutes of the bell ringing?
c. What is the probability that the lecture continues beyond the bell for between 60 and 90 seconds?
d. What is the probability that the lecture continues for at least 90 seconds beyond the bell?

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 17 في هذه المجموعة.
فتح الحزمة
k this deck
16
Stress is applied to a 20-in. steel bar that is clamped in a fixed position at each end. Let X = distance from the left end at which the bar snaps. Suppose Y/20 has a standard beta distribution with E(Y) = 10 and V(Y) = 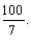
a. What are the parameters of the relevant standard beta distribution?
b. Compute
c. Compute the probability that the bar snaps more than 2 in. from where you expect it to.
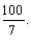
a. What are the parameters of the relevant standard beta distribution?
b. Compute

c. Compute the probability that the bar snaps more than 2 in. from where you expect it to.

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 17 في هذه المجموعة.
فتح الحزمة
k this deck
17
Construct a normal probability plot for the following sample of observations on coating thickness for low-viscosity paint. Would you feel comfortable estimating population mean thickness using a method that assumed a normal population distribution? 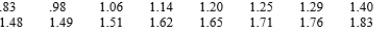
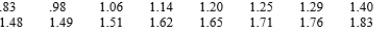

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 17 في هذه المجموعة.
فتح الحزمة
k this deck








