Deck 4: Systems of Linear Equations and Matrices
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال
سؤال

فتح الحزمة
قم بالتسجيل لفتح البطاقات في هذه المجموعة!
Unlock Deck
Unlock Deck
1/111
العب
ملء الشاشة (f)
Deck 4: Systems of Linear Equations and Matrices
1
The traffic through downtown East Podunk flows through the one-way system shown below: 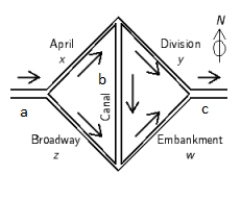
, ,
Traffic counters find that 400 vehicles enter town from the west each hour and 400 leave town toward the east each hour. Also, 50 cars drive down Canal Street each hour.
Write down the general solution of the associated system of linear equations. Is it possible to determine the number of vehicles on each street per hour
A)
B)
C)
D)
E)

, ,
Traffic counters find that 400 vehicles enter town from the west each hour and 400 leave town toward the east each hour. Also, 50 cars drive down Canal Street each hour.
Write down the general solution of the associated system of linear equations. Is it possible to determine the number of vehicles on each street per hour
A)
B)
C)
D)
E)
2
Big Red Bookstore wants to ship books from its warehouses in Brooklyn and Queens to its stores, one on Long Island and one in Manhattan. Its warehouse in Brooklyn has 700 books and its warehouse in Queens has 2,300. Each store orders 1,500 books. It costs $1 to ship each book from Brooklyn to Manhattan and $2 to ship each book from Queens to Manhattan. It costs $5 to ship each book from Brooklyn to Long Island and $4 to ship each book from Queens to Long Island.
If Big Red has a transportation budget of $8,900 and is willing to spend all of it, how many books should Big Red ship from each warehouse to each store in order to fill all the orders
A)Brooklyn to Manhattan: 1,200
Queens to Manhattan: 300
Brooklyn to Long Island: 400
Queens to Long Island: 1,100
B) Brooklyn to Manhattan: 400
Queens to Manhattan: 1,100
Brooklyn to Long Island: 400
Queens to Long Island: 1,100
C) Brooklyn to Manhattan: 400
Queens to Manhattan: 1,100
Brooklyn to Long Island: 300
Queens to Long Island: 1,200
D) Brooklyn to Manhattan: 500
Queens to Manhattan: 1,000
Brooklyn to Long Island: 300
Queens to Long Island: 1,200
E) Brooklyn to Manhattan: 500
Queens to Manhattan: 1,000
Brooklyn to Long Island: 400
Queens to Long Island: 1,100
If Big Red has a transportation budget of $8,900 and is willing to spend all of it, how many books should Big Red ship from each warehouse to each store in order to fill all the orders
A)Brooklyn to Manhattan: 1,200
Queens to Manhattan: 300
Brooklyn to Long Island: 400
Queens to Long Island: 1,100
B) Brooklyn to Manhattan: 400
Queens to Manhattan: 1,100
Brooklyn to Long Island: 400
Queens to Long Island: 1,100
C) Brooklyn to Manhattan: 400
Queens to Manhattan: 1,100
Brooklyn to Long Island: 300
Queens to Long Island: 1,200
D) Brooklyn to Manhattan: 500
Queens to Manhattan: 1,000
Brooklyn to Long Island: 300
Queens to Long Island: 1,200
E) Brooklyn to Manhattan: 500
Queens to Manhattan: 1,000
Brooklyn to Long Island: 400
Queens to Long Island: 1,100
Brooklyn to Manhattan: 400
Queens to Manhattan: 1,100
Brooklyn to Long Island: 300
Queens to Long Island: 1,200
Queens to Manhattan: 1,100
Brooklyn to Long Island: 300
Queens to Long Island: 1,200
3
The Tubular Ride Boogie Board Company has manufacturing plants in Tucson, AZ and Toronto, Ontario. You have been given the job of coordinating distribution of the latest model, the Gladiator, to outlets in Honolulu and Venice Beach. The Tucson plant, when operating at full capacity, can manufacture 620 Gladiator boards per week, while the Toronto plant, beset by labor disputes, can produce only 410 boards per week. The outlet in Honolulu orders 460 Gladiator boards per week, while Venice Beach orders 570 boards per week. Transportation costs are as follows: Tucson to Honolulu: $10 per board; Tucson to Venice Beach: $5 per board;
Toronto to Honolulu: $20 per board; Toronto to Venice Beach: $10 per board.
If Tubular Ride Boogie Board Company has a transportation budget of $10,300 and is willing to assure full capacity production at both plants, how many Gladiator boards should be shipped from each manufacturing plant to each distribution outlet
A)Tucson to Honolulu: 290
Tucson to Venice Beach: 330
Toronto to Honolulu: 170
Toronto to Venice Beach: 240
B) Tucson to Honolulu: 300
Tucson to Venice Beach: 320
Toronto to Honolulu: 170
Toronto to Venice Beach: 240
C) Tucson to Honolulu: 300
Tucson to Venice Beach: 320
Toronto to Honolulu: 160
Toronto to Venice Beach: 250
D) Tucson to Honolulu: 290
Tucson to Venice Beach: 330
Toronto to Honolulu: 160
Toronto to Venice Beach: 250
E) Tucson to Honolulu: 250
Tucson to Venice Beach: 160
Toronto to Honolulu: 300
Toronto to Venice Beach: 320
Toronto to Honolulu: $20 per board; Toronto to Venice Beach: $10 per board.
If Tubular Ride Boogie Board Company has a transportation budget of $10,300 and is willing to assure full capacity production at both plants, how many Gladiator boards should be shipped from each manufacturing plant to each distribution outlet
A)Tucson to Honolulu: 290
Tucson to Venice Beach: 330
Toronto to Honolulu: 170
Toronto to Venice Beach: 240
B) Tucson to Honolulu: 300
Tucson to Venice Beach: 320
Toronto to Honolulu: 170
Toronto to Venice Beach: 240
C) Tucson to Honolulu: 300
Tucson to Venice Beach: 320
Toronto to Honolulu: 160
Toronto to Venice Beach: 250
D) Tucson to Honolulu: 290
Tucson to Venice Beach: 330
Toronto to Honolulu: 160
Toronto to Venice Beach: 250
E) Tucson to Honolulu: 250
Tucson to Venice Beach: 160
Toronto to Honolulu: 300
Toronto to Venice Beach: 320
Tucson to Honolulu: 300
Tucson to Venice Beach: 320
Toronto to Honolulu: 160
Toronto to Venice Beach: 250
Tucson to Venice Beach: 320
Toronto to Honolulu: 160
Toronto to Venice Beach: 250
4
Use the table and the given X-ray absorption diagrams to identify the composition of each of the regions marked by a letter. The number in each region shows its absorption, and the number on each X-ray beam shows the total absorption for that beam. 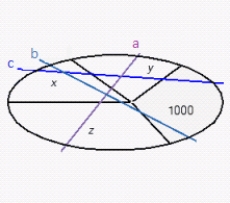 , ,
, ,
A)x = Blood
y = Bone
Z = Blood
B) x = Blood
Y = Gray matter
Z = Tumor
C) x = Bone
Y = Blood
Z = Bone
D) x = Gray matter
Y = Tumor
Z = Blood
E) x = Tumor
Y = Blood
Z = Gray matter
 , ,
, , A)x = Blood
y = Bone
Z = Blood
B) x = Blood
Y = Gray matter
Z = Tumor
C) x = Bone
Y = Blood
Z = Bone
D) x = Gray matter
Y = Tumor
Z = Blood
E) x = Tumor
Y = Blood
Z = Gray matter

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 111 في هذه المجموعة.
فتح الحزمة
k this deck
5
The chart below shows the amount spent by three U.S. airlines to fly one available seat one mile in the fourth quarter of 2000. Suppose that, on a 3000-mile New York-Los Angeles flight, United, American and SouthWest flew a total of 190 empty seats, costing them a total of $60,900. If United had three times as many empty seats as American, how many empty seats did each of these three airlines carry on its flight
A)United: 130
American: 40
SouthWest: 30
B) United: 120
American: 30
SouthWest: 40
C) United: 30
American: 40
SouthWest: 120
D) United: 120
American: 40
SouthWest: 30
E) United: 120
American: 30
SouthWest: 30
A)United: 130
American: 40
SouthWest: 30
B) United: 120
American: 30
SouthWest: 40
C) United: 30
American: 40
SouthWest: 120
D) United: 120
American: 40
SouthWest: 30
E) United: 120
American: 30
SouthWest: 30

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 111 في هذه المجموعة.
فتح الحزمة
k this deck
6
Conan the Great has boasted to his hordes of followers that many a notorious villain has fallen to his awesome sword: His total of 570 victims consists of evil sorcerers, warriors, and orcs. These he has slain with a total of 630 mighty thrusts of his sword--evil sorcerers and warriors each requiring 2 thrusts (to the chest) and orcs each requiring 1 thrust (to the neck).When asked about the number of warriors he has slain, he replies, "The number of warriors I, the mighty Conan, have slain is four times the number of evil sorcerers that have fallen to my sword!" How many of each type of villain has he slain ?
A)Evil sorcerers: 23 Warriors: 48
Orcs: 488
B) Evil sorcerers: 12
Warriors: 48
Orcs: 510
C) Evil sorcerers: 48
Warriors: 12
Orcs: 510
D) Evil sorcerers: 12
Warriors: 37
Orcs: 488
E) Evil sorcerers: 12
Warriors: 48
Orcs: 488
A)Evil sorcerers: 23 Warriors: 48
Orcs: 488
B) Evil sorcerers: 12
Warriors: 48
Orcs: 510
C) Evil sorcerers: 48
Warriors: 12
Orcs: 510
D) Evil sorcerers: 12
Warriors: 37
Orcs: 488
E) Evil sorcerers: 12
Warriors: 48
Orcs: 488

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 111 في هذه المجموعة.
فتح الحزمة
k this deck
7
The Fancy French Perfume Company recently had its secret formula divulged. It turned out that it was using, as the three ingredients, rose oil, oil of fermented prunes, and alcohol. Moreover, each 20-ounce econo-size bottle contained 3 more ounces of alcohol than oil of fermented prunes, while the amount of alcohol was equal to the combined volume of the other two ingredients. How much of each ingredient did it use in an econo-size bottle ?
A)Rose oil: 3 Oil of fermented prunes: 7
Alcohol: 10
B) Rose oil: 3
Oil of fermented prunes: 7
Alcohol: 11
C) Rose oil: 10
Oil of fermented prunes: 7
Alcohol: 3
D) Rose oil: 10
Oil of fermented prunes: 3
Alcohol: 7
E) Rose oil: 4
Oil of fermented prunes: 7
Alcohol: 11
A)Rose oil: 3 Oil of fermented prunes: 7
Alcohol: 10
B) Rose oil: 3
Oil of fermented prunes: 7
Alcohol: 11
C) Rose oil: 10
Oil of fermented prunes: 7
Alcohol: 3
D) Rose oil: 10
Oil of fermented prunes: 3
Alcohol: 7
E) Rose oil: 4
Oil of fermented prunes: 7
Alcohol: 11

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 111 في هذه المجموعة.
فتح الحزمة
k this deck
8
You invested a total of $6,800 in three funds at the beginning of 2003, including an equal amount in PNF and CMBFX. You earned a total of $407 in interest for the year. How much did you invest in each of the three funds Use the data given in the figure below.
A)PNF (Pimco N. Y.): $2,500 CMBFX (Columbia Ore): $2,500
SFCOX : $2,200
B) PNF (Pimco N. Y.): $2,500
CMBFX (Columbia Ore): $2,500
SFCOX : $1,800
C) PNF (Pimco N. Y.): $2,200
CMBFX (Columbia Ore): $2,200
SFCOX : $2,400
D) PNF (Pimco N. Y.): $2,300
CMBFX (Columbia Ore): $2,300
SFCOX : $2,200
E) PNF (Pimco N. Y.): $2,300
CMBFX (Columbia Ore): $2,300
SFCOX : $1,800
A)PNF (Pimco N. Y.): $2,500 CMBFX (Columbia Ore): $2,500
SFCOX : $2,200
B) PNF (Pimco N. Y.): $2,500
CMBFX (Columbia Ore): $2,500
SFCOX : $1,800
C) PNF (Pimco N. Y.): $2,200
CMBFX (Columbia Ore): $2,200
SFCOX : $2,400
D) PNF (Pimco N. Y.): $2,300
CMBFX (Columbia Ore): $2,300
SFCOX : $2,200
E) PNF (Pimco N. Y.): $2,300
CMBFX (Columbia Ore): $2,300
SFCOX : $1,800

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 111 في هذه المجموعة.
فتح الحزمة
k this deck
9
This exercise is based on the following simplified model of the determination of the money stock.
, Where M - money stock
C - currency in circulation
R - bank reserves
D - deposits of the public
H - high-powered money
If the money stock were $192 billion, what would bank reserves have to be
A)$11 billion
B) $18 billion
C) $17 billion
D) $12 billion
E) $16 billion
, Where M - money stock
C - currency in circulation
R - bank reserves
D - deposits of the public
H - high-powered money
If the money stock were $192 billion, what would bank reserves have to be
A)$11 billion
B) $18 billion
C) $17 billion
D) $12 billion
E) $16 billion

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 111 في هذه المجموعة.
فتح الحزمة
k this deck
10
In November, 2003, the four organizations ranking 5 through 8 in home Internet users in the US were eBay, the U.S. Government, Amazon, and Lycos, with a combined audience of 111 million users. Taking x to be the eBay audience in millions, y the U.S. Government audience in millions, z the Amazon audience in millions, and u the Lycos audience in millions, it was observed that
How large was the audience of each of the four companies in November, 2003 (All amounts in millions.)
A)eBay: 35
The U.S. Government: 25
Amazon: 25
Lycos: 24
B) eBay: 36
The U.S. Government: 26
Amazon: 25
Lycos: 24
C) eBay: 35
The U.S. Government: 26
Amazon: 25
Lycos: 23
D) eBay: 35
The U.S. Government: 26
Amazon: 24
Lycos: 24
E) eBay: 35
The U.S. Government: 26
Amazon: 25
Lycos: 24
How large was the audience of each of the four companies in November, 2003 (All amounts in millions.)
A)eBay: 35
The U.S. Government: 25
Amazon: 25
Lycos: 24
B) eBay: 36
The U.S. Government: 26
Amazon: 25
Lycos: 24
C) eBay: 35
The U.S. Government: 26
Amazon: 25
Lycos: 23
D) eBay: 35
The U.S. Government: 26
Amazon: 24
Lycos: 24
E) eBay: 35
The U.S. Government: 26
Amazon: 25
Lycos: 24

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 111 في هذه المجموعة.
فتح الحزمة
k this deck
11
Significant numbers of tourists traveled from North America and Asia to Australia and South Africa. In any year a total of 2,223,240 of these tourists visited Australia, and 393,860 of them visited South Africa. Also, 639,210 of these tourists came from North America, and a total of 2,617,100 tourists traveled from these two regions to these two destinations. (Assume that no single tourist visited both destinations or traveled from both North America and Asia.) The given information is not sufficient to determine the number of tourists from each region to each destination. If you were given the additional information that 190,000 tourists visited South Africa from Asia, would you now be able to determine the number of tourists from each region to each destination If so, what are these numbers ?
A)from North America to Australia: 435,390 from Asia to Australia: 1,787,850
From North America to South Africa: 203,820
From Asia to South Africa: 190,040
B) from North America to Australia: 203,860
From Asia to Australia: 435,350
From North America to South Africa: 190,000
From Asia to South Africa: 1,787,890
C) from North America to Australia: 435,350
From Asia to Australia: 1,787,890
From North America to South Africa: 203,860
From Asia to South Africa: 190,000
D) from North America to Australia: 203,820
From Asia to Australia: 435,390
From North America to South Africa: 190,040
From Asia to South Africa: 1,787,850
E) This information is still not sufficient to determine the number of tourists from each region to each destination.
A)from North America to Australia: 435,390 from Asia to Australia: 1,787,850
From North America to South Africa: 203,820
From Asia to South Africa: 190,040
B) from North America to Australia: 203,860
From Asia to Australia: 435,350
From North America to South Africa: 190,000
From Asia to South Africa: 1,787,890
C) from North America to Australia: 435,350
From Asia to Australia: 1,787,890
From North America to South Africa: 203,860
From Asia to South Africa: 190,000
D) from North America to Australia: 203,820
From Asia to Australia: 435,390
From North America to South Africa: 190,040
From Asia to South Africa: 1,787,850
E) This information is still not sufficient to determine the number of tourists from each region to each destination.

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 111 في هذه المجموعة.
فتح الحزمة
k this deck
12
In November 2003, the four companies with the largest number of home Internet users in the US were Microsoft, Time Warner, Yahoo, and Google, with a combined audience of 274 million users. Taking to be the Microsoft audience in millions, the Time Warner audience in millions, the Yahoo audience in millions, and the Google audience in millions, it was observed that
How large was the audience of each of the four companies in November, 2003 (All amounts in millions.)
A)Microsoft: 88
Time Warner: 77
Yahoo: 73
Google: 35
B) Microsoft: 88
Time Warner: 77
Yahoo: 74
Google: 34
C) Microsoft: 88
Time Warner: 77
Yahoo: 74
Google: 35
D) Microsoft: 88
Time Warner: 78
Yahoo: 74
Google: 35
E) Microsoft: 89
Time Warner: 77
Yahoo: 74
Google: 35
How large was the audience of each of the four companies in November, 2003 (All amounts in millions.)
A)Microsoft: 88
Time Warner: 77
Yahoo: 73
Google: 35
B) Microsoft: 88
Time Warner: 77
Yahoo: 74
Google: 34
C) Microsoft: 88
Time Warner: 77
Yahoo: 74
Google: 35
D) Microsoft: 88
Time Warner: 78
Yahoo: 74
Google: 35
E) Microsoft: 89
Time Warner: 77
Yahoo: 74
Google: 35

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 111 في هذه المجموعة.
فتح الحزمة
k this deck
13
Boeing 747s seat 400 passengers and are priced at $200 million each, Boeing 777s seat 300 passengers and are priced at $160 million, and European Airbus A321s seat 200 passengers and are priced at $60 million. You are the purchasing manager of an airline company and have a $8,640 million budget to purchase new aircraft to seat a total of 20,800 passengers. Your company has a policy of supporting U.S. industries, and you have been required to spend twice as much on U.S.- manufactured aircraft as on foreign aircraft. Given the selection of three aircraft, how many of each should you order ?
A)Number of Boeing 747s: 18 Number of Boeing 777s: 14
Number of European Airbus A321s: 45
B) Number of Boeing 747s: 18
Number of Boeing 777s: 16
Number of European Airbus A321s: 45
C) Number of Boeing 747s: 48
Number of Boeing 777s: 16
Number of European Airbus A321s: 16
D) Number of Boeing 747s: 16
Number of Boeing 777s: 16
Number of European Airbus A321s: 48
E) Number of Boeing 747s: 16
Number of Boeing 777s: 16
Number of European Airbus A321s: 45
A)Number of Boeing 747s: 18 Number of Boeing 777s: 14
Number of European Airbus A321s: 45
B) Number of Boeing 747s: 18
Number of Boeing 777s: 16
Number of European Airbus A321s: 45
C) Number of Boeing 747s: 48
Number of Boeing 777s: 16
Number of European Airbus A321s: 16
D) Number of Boeing 747s: 16
Number of Boeing 777s: 16
Number of European Airbus A321s: 48
E) Number of Boeing 747s: 16
Number of Boeing 777s: 16
Number of European Airbus A321s: 45

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 111 في هذه المجموعة.
فتح الحزمة
k this deck
14
Urban Community College is planning to offer courses in Finite Math, Applied Calculus, and Computer Methods. Each section of Finite Math holds 40 students and earns the college $1,300 in revenue per student. Each section of Applied Calculus holds 45 students and earns the college $1,600 per student, and each section of Computer Methods holds 15 students and earns the college $1,900 per student. Assuming that the college can offer a total of seven sections, wishes to accommodate 240 students, and wishes to bring in $357,000 in revenues, how many sections of each course should it offer ?
A)Offer 2 sections of Finite Math, 2 sections of Applied Calculus, and 1 section of Computer Methods
B) Offer 2 sections of Finite Math, 3 sections of Applied Calculus, and 2 sections of Computer Methods
C) Offer 3 sections of Finite Math, 2 sections of Applied Calculus, and 2 sections of Computer Methods
D) Offer 5 sections of Finite Math, 1 section of Applied Calculus, and 1 section of Computer Methods
E) Offer 1 section of Finite Math, 5 sections of Applied Calculus, and 1 section of Computer Methods
A)Offer 2 sections of Finite Math, 2 sections of Applied Calculus, and 1 section of Computer Methods
B) Offer 2 sections of Finite Math, 3 sections of Applied Calculus, and 2 sections of Computer Methods
C) Offer 3 sections of Finite Math, 2 sections of Applied Calculus, and 2 sections of Computer Methods
D) Offer 5 sections of Finite Math, 1 section of Applied Calculus, and 1 section of Computer Methods
E) Offer 1 section of Finite Math, 5 sections of Applied Calculus, and 1 section of Computer Methods

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 111 في هذه المجموعة.
فتح الحزمة
k this deck
15
Given that the number of U.S. 10th graders who were alcohol-free was 50% more than the number who had used alcohol, find the missing data.
A)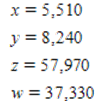
B)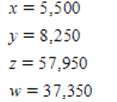
C)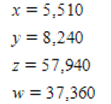
D)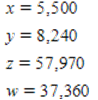
E)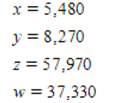
A)
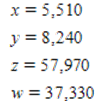
B)
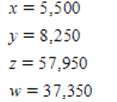
C)
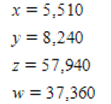
D)
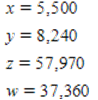
E)
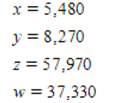

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 111 في هذه المجموعة.
فتح الحزمة
k this deck
16
You own a hamburger franchise and are planning to shut down operations for the day, but you are left with 12 bread rolls, 17 defrosted beef patties, and 13 opened cheese slices. Rather than throw them out, you decide to use them to make burgers that you will sell at a discount. Plain burgers each require 1 beef patty and 1 bread roll, double cheeseburgers each require 2 beef patties, 1 bread roll, and 2 slices of cheese, and regular cheeseburgers each require 1 beef patty, 1 bread roll, and 1 slice of cheese. How many of each should you make ?
A)Plain burgers: 4 Double cheeseburgers: 5
Regular cheeseburgers: 3
B) Plain burgers: 5
Double cheeseburgers: 3
Regular cheeseburgers: 4
C) Plain burgers: 5
Double cheeseburgers: 2
Regular cheeseburgers: 4
D) Plain burgers: 3
Double cheeseburgers: 6
Regular cheeseburgers: 2
E) ?Plain burgers: 6
Double cheeseburgers: 2
Regular cheeseburgers: 3
A)Plain burgers: 4 Double cheeseburgers: 5
Regular cheeseburgers: 3
B) Plain burgers: 5
Double cheeseburgers: 3
Regular cheeseburgers: 4
C) Plain burgers: 5
Double cheeseburgers: 2
Regular cheeseburgers: 4
D) Plain burgers: 3
Double cheeseburgers: 6
Regular cheeseburgers: 2
E) ?Plain burgers: 6
Double cheeseburgers: 2
Regular cheeseburgers: 3

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 111 في هذه المجموعة.
فتح الحزمة
k this deck
17
Electric current measures (in amperes, or amps) the flow of electrons through wires. Like traffic flow, the current entering an intersection of wires must equal the current leaving it. Here is an electrical circuit known as a Wheatstone bridge: 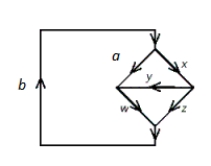 A, A
A, A
If the currents in two of the wires are 20 amps and 5 amps as shown, determine the currents in the unlabeled wires in terms of suitable parameters.
A)
B)
C)
D)
E)
 A, A
A, AIf the currents in two of the wires are 20 amps and 5 amps as shown, determine the currents in the unlabeled wires in terms of suitable parameters.
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 111 في هذه المجموعة.
فتح الحزمة
k this deck
18
The chart below shows the amount spent by three U.S. airlines to fly one available seat one mile in the fourth quarter of 2000.
Suppose that, on a 2000-mile Miami-Memphis flight, Continental, SkyWest, and SouthWest flew a total of 170 empty seats, costing them a total of $48,520. If SkyWest had twice as many empty seats as SouthWest, how many empty seats did each of these three airlines carry on its flight
A)Continental: 50
SkyWest: 70
SouthWest: 40
B) Continental: 50
SkyWest: 70
SouthWest: 50
C) Continental: 50
SkyWest: 80
SouthWest: 40
D) Continental: 60
SkyWest: 70
SouthWest: 50
E) Continental: 60
SkyWest: 80
SouthWest: 40
Suppose that, on a 2000-mile Miami-Memphis flight, Continental, SkyWest, and SouthWest flew a total of 170 empty seats, costing them a total of $48,520. If SkyWest had twice as many empty seats as SouthWest, how many empty seats did each of these three airlines carry on its flight
A)Continental: 50
SkyWest: 70
SouthWest: 40
B) Continental: 50
SkyWest: 70
SouthWest: 50
C) Continental: 50
SkyWest: 80
SouthWest: 40
D) Continental: 60
SkyWest: 70
SouthWest: 50
E) Continental: 60
SkyWest: 80
SouthWest: 40

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 111 في هذه المجموعة.
فتح الحزمة
k this deck
19
In 2000, total revenues from sales of recorded rock, rap, and classical music amounted to $12.0 billion. Rock music brought in twice as much revenue as rap music and 600% the revenue of classical music. How much revenues were earned in each of the three categories of recorded music ?
A)Revenues from sales of recorded rock: 7.2 Revenues from sales of recorded rap: 3.7
Revenues from sales of recorded classical music: 1.2
B) Revenues from sales of recorded rock: 7.2
Revenues from sales of recorded rap: 3.6
Revenues from sales of recorded classical music: 1.2
C) Revenues from sales of recorded rock: 1.2
Revenues from sales of recorded rap: 7.2
Revenues from sales of recorded classical music: 3.6
D) Revenues from sales of recorded rock: 7.1
Revenues from sales of recorded rap: 3.7
Revenues from sales of recorded classical music: 1.2
E) Revenues from sales of recorded rock: 3.6
Revenues from sales of recorded rap: 7.2
Revenues from sales of recorded classical music: 1.2
A)Revenues from sales of recorded rock: 7.2 Revenues from sales of recorded rap: 3.7
Revenues from sales of recorded classical music: 1.2
B) Revenues from sales of recorded rock: 7.2
Revenues from sales of recorded rap: 3.6
Revenues from sales of recorded classical music: 1.2
C) Revenues from sales of recorded rock: 1.2
Revenues from sales of recorded rap: 7.2
Revenues from sales of recorded classical music: 3.6
D) Revenues from sales of recorded rock: 7.1
Revenues from sales of recorded rap: 3.7
Revenues from sales of recorded classical music: 1.2
E) Revenues from sales of recorded rock: 3.6
Revenues from sales of recorded rap: 7.2
Revenues from sales of recorded classical music: 1.2

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 111 في هذه المجموعة.
فتح الحزمة
k this deck
20
Use the table and the given X-ray absorption diagrams to identify the composition of each of the regions marked by a letter. The number in each region shows its absorption, and the number on each X-ray beam shows the total absorption for that beam. 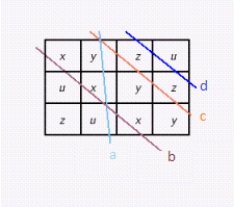 , , ,
, , ,
A)
B)
C)
D)
E)
 , , ,
, , , A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 111 في هذه المجموعة.
فتح الحزمة
k this deck
21
The chart below shows the amount spent by three U.S. airlines to fly one available seat one mile in the fourth quarter of 2000. ?
Suppose that, on a 3000-mile New York-Los Angeles flight, United, American and SouthWest flew a total of 150 empty seats, costing them a total of $47,430. If United had three times as many empty seats as American, how many empty seats did each of these three airlines carry on its flight
United __________
American __________
SouthWest __________
Suppose that, on a 3000-mile New York-Los Angeles flight, United, American and SouthWest flew a total of 150 empty seats, costing them a total of $47,430. If United had three times as many empty seats as American, how many empty seats did each of these three airlines carry on its flight
United __________
American __________
SouthWest __________

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 111 في هذه المجموعة.
فتح الحزمة
k this deck
22
Boeing 747s seat 400 passengers and are priced at $200 million each, Boeing 777s seat 300 passengers and are priced at $160 million, and European Airbus A321s seat 200 passengers and are priced at $60 million. You are the purchasing manager of an airline company and have a $7,920 million budget to purchase new aircraft to seat a total of 19,000 passengers. Your company has a policy of supporting U.S. industries, and you have been required to spend twice as much on U.S.- manufactured aircraft as on foreign aircraft. Given the selection of three aircraft, how many of each should you order
Number of Boeing 747s
__________
Number of Boeing 777s
__________
Number of European Airbus A321s
__________
Number of Boeing 747s
__________
Number of Boeing 777s
__________
Number of European Airbus A321s
__________

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 111 في هذه المجموعة.
فتح الحزمة
k this deck
23
This exercise is based on the following simplified model of the determination of the money stock.
,
where M - money stock
C - currency in circulation
R - bank reserves
D - deposits of the public
H - high-powered money
If the money stock were $108 billion, what would bank reserves have to be
$____________billion
,
where M - money stock
C - currency in circulation
R - bank reserves
D - deposits of the public
H - high-powered money
If the money stock were $108 billion, what would bank reserves have to be
$____________billion

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 111 في هذه المجموعة.
فتح الحزمة
k this deck
24
Use Gauss-Jordan row reduction to solve the system of equations.
A)
B)
C)
D) Redundant
E) Inconsistent
A)
B)
C)
D) Redundant
E) Inconsistent

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 111 في هذه المجموعة.
فتح الحزمة
k this deck
25
Urban Community College is planning to offer courses in Finite Math, Applied Calculus, and Computer Methods. Each section of Finite Math holds 50 students and earns the college $1,000 in revenue per student. Each section of Applied Calculus holds 45 students and earns the college $1,500 per student, and each section of Computer Methods holds 10 students and earns the college $2,200 per student. Assuming that the college can offer a total of seven sections, wishes to accommodate 305 students, and wishes to bring in $339,500 in revenues, how many sections of each course should it offer
Number of sections of Finite Math:
__________
Number of sections of Applied Calculus:
__________
Number of sections of Computer Methods:
__________
Number of sections of Finite Math:
__________
Number of sections of Applied Calculus:
__________
Number of sections of Computer Methods:
__________

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 111 في هذه المجموعة.
فتح الحزمة
k this deck
26
You invested a total of $6,400 in three funds at the beginning of 2003, including an equal amount in PNF and CMBFX. You earned a total of $391 in interest for the year. How much did you invest in each of the three funds Use the data given in the figure below.
PNF (Pimco N. Y.)
$__________
CMBFX (Columbia Ore)
$__________
SFCOX
$__________
PNF (Pimco N. Y.)
$__________
CMBFX (Columbia Ore)
$__________
SFCOX
$__________

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 111 في هذه المجموعة.
فتح الحزمة
k this deck
27
In November 2003, the four companies with the largest number of home Internet users in the US were Microsoft, Time Warner, Yahoo, and Google, with a combined audience of 282 million users. Taking x to be the Microsoft audience in millions, ?y the Time Warner audience in millions, z the Yahoo audience in millions, and u the Google audience in millions, it was observed that
How large was the audience of each of the four companies in November, 2003
Microsoft
__________ millions
Time Warner
__________ millions
Yahoo
__________ millions
Google
__________ millions
How large was the audience of each of the four companies in November, 2003
Microsoft
__________ millions
Time Warner
__________ millions
Yahoo
__________ millions
__________ millions

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 111 في هذه المجموعة.
فتح الحزمة
k this deck
28
Use the table and the given X-ray absorption diagrams to identify the composition of each of the regions marked by a letter. The number in each region shows its absorption, and the number on each X-ray beam shows the total absorption for that beam. 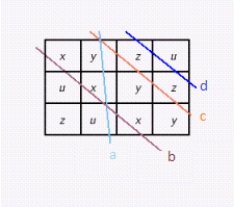 , , ,
, , ,
x = __________
y = __________
z = __________
u = _________
 , , ,
, , , x = __________
y = __________
z = __________
u = _________

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 111 في هذه المجموعة.
فتح الحزمة
k this deck
29
Big Red Bookstore wants to ship books from its warehouses in Brooklyn and Queens to its stores, one on Long Island and one in Manhattan. Its warehouse in Brooklyn has 1,000 books and its warehouse in Queens has 2,000. Each store orders 1,500 books. It costs $1 to ship each book from Brooklyn to Manhattan and $2 to ship each book from Queens to Manhattan. It costs $5 to ship each book from Brooklyn to Long Island and $4 to ship each book from Queens to Long Island.
a. If Big Red has a transportation budget of $9,200 and is willing to spend all of it, how many books should Big Red ship from each warehouse to each store in order to fill all the orders
Brooklyn to Manhattan __________
Queens to Manhattan __________
Brooklyn to Long Island __________
Queens to Long Island __________
b. How many books should Big Red ship from each warehouse to each store in order to fill all the orders for the minimum amount of money
Brooklyn to Manhattan __________
Queens to Manhattan __________
Brooklyn to Long Island __________
Queens to Long Island __________
a. If Big Red has a transportation budget of $9,200 and is willing to spend all of it, how many books should Big Red ship from each warehouse to each store in order to fill all the orders
Brooklyn to Manhattan __________
Queens to Manhattan __________
Brooklyn to Long Island __________
Queens to Long Island __________
b. How many books should Big Red ship from each warehouse to each store in order to fill all the orders for the minimum amount of money
Brooklyn to Manhattan __________
Queens to Manhattan __________
Brooklyn to Long Island __________
Queens to Long Island __________

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 111 في هذه المجموعة.
فتح الحزمة
k this deck
30
In the matrix of a system of linear equations, if one row of a matrix is a multiple of another row, then during the process of row reduction, one of those rows will eventually become all ones. Is statement

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 111 في هذه المجموعة.
فتح الحزمة
k this deck
31
Use the table and the given X-ray absorption diagrams to identify the composition of each of the regions marked by a letter. The number in each region shows its absorption, and the number on each X-ray beam shows the total absorption for that beam. 
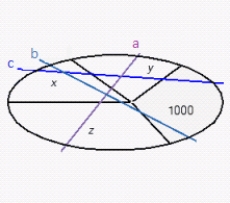
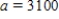 ,
, 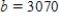 ,
, 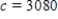
x =
__________
y =
__________
z =
__________


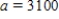 ,
, 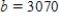 ,
, 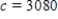
x =
__________
y =
__________
z =
__________

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 111 في هذه المجموعة.
فتح الحزمة
k this deck
32
In 2000, total revenues from sales of recorded rock, rap, and classical music amounted to $14.5 billion. Rock music brought in twice as much revenue as rap music and 900% the revenue of classical music. How much revenues were earned in each of the three categories of recorded music (Round your answers to one decimal place.)
Rock
$__________ billion
Rap
$__________ billion
Classical music
$__________ billion
Rock
$__________ billion
Rap
$__________ billion
Classical music
$__________ billion

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 111 في هذه المجموعة.
فتح الحزمة
k this deck
33
In November, 2003, the four organizations ranking 5 through 8 in home Internet users in the US were eBay, the U.S. Government, Amazon, and Lycos, with a combined audience of 115 million users. Taking x to be the eBay audience in millions, y the U.S. Government audience in millions, z the Amazon audience in millions, and u the Lycos audience in millions, it was observed that
How large was the audience of each of the four companies in November, 2003
eBay
__________ millions
The U.S. Government
__________ millions
Amazon
__________ millions
Lycos
__________ millions
How large was the audience of each of the four companies in November, 2003
eBay
__________ millions
The U.S. Government
__________ millions
Amazon
__________ millions
Lycos
__________ millions

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 111 في هذه المجموعة.
فتح الحزمة
k this deck
34
Conan the Great has boasted to his hordes of followers that many a notorious villain has fallen to his awesome sword: His total of 545 victims consists of evil sorcerers, warriors, and orcs. These he has slain with a total of 580 mighty thrusts of his sword--evil sorcerers and warriors each requiring 2 thrusts (to the chest) and orcs each requiring 1 thrust (to the neck).When asked about the number of warriors he has slain, he replies, "The number of warriors I, the mighty Conan, have slain is four times the number of evil sorcerers that have fallen to my sword!" How many of each type of villain has he slain
Evil sorcerers:
__________
Warriors:
__________
Orcs:
__________
Evil sorcerers:
__________
Warriors:
__________
Orcs:
__________

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 111 في هذه المجموعة.
فتح الحزمة
k this deck
35
You own a hamburger franchise and are planning to shut down operations for the day, but you are left with 10 bread rolls, 15 defrosted beef patties, and 13 opened cheese slices. Rather than throw them out, you decide to use them to make burgers that you will sell at a discount. Plain burgers each require 1 beef patty and 1 bread roll, double cheeseburgers each require 2 beef patties, 1 bread roll, and 2 slices of cheese, and regular cheeseburgers each require 1 beef patty, 1 bread roll, and 1 slice of cheese. How many of each should you make
Plain burgers:
__________
Double cheeseburgers:
__________
Regular cheeseburgers:
__________
Plain burgers:
__________
Double cheeseburgers:
__________
Regular cheeseburgers:
__________

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 111 في هذه المجموعة.
فتح الحزمة
k this deck
36
Your friend Frans tells you that the system of linear equations you are solving cannot have a unique solution because the reduced matrix has a row of zeros. Is Frans' statement

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 111 في هذه المجموعة.
فتح الحزمة
k this deck
37
Use Gauss-Jordan row reduction to solve the system.
A)
B)
C)
D)
E) inconsistent
A)
B)
C)
D)
E) inconsistent

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 111 في هذه المجموعة.
فتح الحزمة
k this deck
38
The chart below shows the amount spent by three U.S. airlines to fly one available seat one mile in the fourth quarter of 2000. ?
Suppose that, on a 2000-mile Miami-Memphis flight, Continental, SkyWest, and SouthWest flew a total of 180 empty seats, costing them a total of $54,280. If SkyWest had twice as many empty seats as SouthWest, how many empty seats did each of these three airlines carry on its flight
Continental __________
SkyWest __________
SouthWest __________
Suppose that, on a 2000-mile Miami-Memphis flight, Continental, SkyWest, and SouthWest flew a total of 180 empty seats, costing them a total of $54,280. If SkyWest had twice as many empty seats as SouthWest, how many empty seats did each of these three airlines carry on its flight
Continental __________
SkyWest __________
SouthWest __________

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 111 في هذه المجموعة.
فتح الحزمة
k this deck
39
The Fancy French Perfume Company recently had its secret formula divulged. It turned out that it was using, as the three ingredients, rose oil, oil of fermented prunes, and alcohol. Moreover, each 18-ounce econo-size bottle contained 3 more ounces of alcohol than oil of fermented prunes, while the amount of alcohol was equal to the combined volume of the other two ingredients. How much of each ingredient did it use in an econo-size bottle
Rose oil: __________
Oil of fermented prunes: __________
Alcohol: __________
Rose oil: __________
Oil of fermented prunes: __________
Alcohol: __________

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 111 في هذه المجموعة.
فتح الحزمة
k this deck
40
The Tubular Ride Boogie Board Company has manufacturing plants in Tucson, AZ and Toronto, Ontario. You have been given the job of coordinating distribution of the latest model, the Gladiator, to outlets in Honolulu and Venice Beach. The Tucson plant, when operating at full capacity, can manufacture 620 Gladiator boards per week, while the Toronto plant, beset by labor disputes, can produce only 410 boards per week. The outlet in Honolulu orders 540 Gladiator boards per week, while Venice Beach orders 490 boards per week. Transportation costs are as follows:
Tucson to Honolulu: $10 per board; Tucson to Venice Beach: $5 per board;
Toronto to Honolulu: $20 per board; Toronto to Venice Beach: $10 per board.
a. If Tubular Ride Boogie Board Company has a transportation budget of $10,750 and is willing to assure full capacity production at both plants, how many Gladiator boards should be shipped from each manufacturing plant to each distribution outlet
Tucson to Honolulu
__________
Tucson to Venice Beach
__________
Toronto to Honolulu
__________
Toronto to Venice Beach
__________
b. How many Gladiator boards should be shipped from each manufacturing plant to each distribution outlet to assure full capacity production at both plants for less money
Tucson to Honolulu
__________
Tucson to Venice Beach
__________
Toronto to Honolulu
__________
Toronto to Venice Beach
__________
Tucson to Honolulu: $10 per board; Tucson to Venice Beach: $5 per board;
Toronto to Honolulu: $20 per board; Toronto to Venice Beach: $10 per board.
a. If Tubular Ride Boogie Board Company has a transportation budget of $10,750 and is willing to assure full capacity production at both plants, how many Gladiator boards should be shipped from each manufacturing plant to each distribution outlet
Tucson to Honolulu
__________
Tucson to Venice Beach
__________
Toronto to Honolulu
__________
Toronto to Venice Beach
__________
b. How many Gladiator boards should be shipped from each manufacturing plant to each distribution outlet to assure full capacity production at both plants for less money
Tucson to Honolulu
__________
Tucson to Venice Beach
__________
Toronto to Honolulu
__________
Toronto to Venice Beach
__________

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 111 في هذه المجموعة.
فتح الحزمة
k this deck
41
Use Gauss-Jordan row reduction to solve the given system of equations.
A) , z, u arbitrary
B) , z, u arbitrary
C) , z, u arbitrary
D) , z, u arbitrary
E) , z, u arbitrary
A) , z, u arbitrary
B) , z, u arbitrary
C) , z, u arbitrary
D) , z, u arbitrary
E) , z, u arbitrary

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 111 في هذه المجموعة.
فتح الحزمة
k this deck
42
Use Gauss-Jordan row reduction to solve the given system of equations.
A) , z, w arbitrary
B) , z, w arbitrary
C) , z, w arbitrary
D) , z, w arbitrary
E) , z, w arbitrary
A) , z, w arbitrary
B) , z, w arbitrary
C) , z, w arbitrary
D) , z, w arbitrary
E) , z, w arbitrary

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 111 في هذه المجموعة.
فتح الحزمة
k this deck
43
Use Gauss-Jordan row reduction to solve the given system of equations.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 111 في هذه المجموعة.
فتح الحزمة
k this deck
44
Use Gauss-Jordan row reduction to solve the system of equations.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 111 في هذه المجموعة.
فتح الحزمة
k this deck
45
Use technology to solve the system of equations.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 111 في هذه المجموعة.
فتح الحزمة
k this deck
46
Use technology to solve the system of equations. Express all solutions as decimals, rounded to one decimal place.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 111 في هذه المجموعة.
فتح الحزمة
k this deck
47
Use Gauss-Jordan row reduction to solve the system of equations.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 111 في هذه المجموعة.
فتح الحزمة
k this deck
48
Use Gauss-Jordan row reduction to solve the given systems of equation.
A)
B)
C)
D) inconsistent
E) dependent
A)
B)
C)
D) inconsistent
E) dependent

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 111 في هذه المجموعة.
فتح الحزمة
k this deck
49
Use Gauss-Jordan row reduction to solve the system of equations.
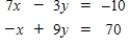
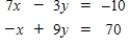

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 111 في هذه المجموعة.
فتح الحزمة
k this deck
50
Use Gauss-Jordan reduction to solve the system of equations.
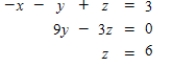
If the system is dependent or inconsistent, indicate this.
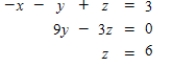
If the system is dependent or inconsistent, indicate this.

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 111 في هذه المجموعة.
فتح الحزمة
k this deck
51
Use Gauss-Jordan row reduction to solve the given system of equations.
A) , z arbitrary
B) , z arbitrary
C)
D) , z arbitrary
E) inconsistent
A) , z arbitrary
B) , z arbitrary
C)
D) , z arbitrary
E) inconsistent

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 111 في هذه المجموعة.
فتح الحزمة
k this deck
52
Use Gauss-Jordan row reduction to solve the system of equations.
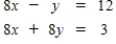
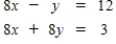

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 111 في هذه المجموعة.
فتح الحزمة
k this deck
53
Use Gauss-Jordan row reduction to solve the given systems of equation.
A)
B)
C)
D) inconsistent
E) dependent
A)
B)
C)
D) inconsistent
E) dependent

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 111 في هذه المجموعة.
فتح الحزمة
k this deck
54
Use Gauss-Jordan row reduction to solve the given system of equations.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 111 في هذه المجموعة.
فتح الحزمة
k this deck
55
Use Gauss-Jordan row reduction to solve the given system of equations.
A) , z, u, v arbitrary
B) , z, u, v arbitrary
C) , z, u, v arbitrary
D) , z, u, v arbitrary
E) , z, u, v arbitrary
A) , z, u, v arbitrary
B) , z, u, v arbitrary
C) , z, u, v arbitrary
D) , z, u, v arbitrary
E) , z, u, v arbitrary

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 111 في هذه المجموعة.
فتح الحزمة
k this deck
56
Use Gauss-Jordan row reduction to solve the given system of equations.
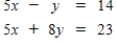
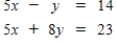

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 111 في هذه المجموعة.
فتح الحزمة
k this deck
57
Use Gauss-Jordan reduction to solve the system of equations.
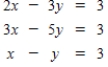
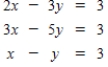

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 111 في هذه المجموعة.
فتح الحزمة
k this deck
58
Solve the system of equations using Gauss-Jordan row reduction.
A)
B)
C)
D) inconsistent
E) dependent
A)
B)
C)
D) inconsistent
E) dependent

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 111 في هذه المجموعة.
فتح الحزمة
k this deck
59
Solve the system of equations using Gauss-Jordan row reduction.
A)
B)
C)
D) inconsistent
E) dependent
A)
B)
C)
D) inconsistent
E) dependent

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 111 في هذه المجموعة.
فتح الحزمة
k this deck
60
Use Gauss-Jordan reduction to solve the system of equations.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 111 في هذه المجموعة.
فتح الحزمة
k this deck
61
Use technology to solve the system of equations. Express all solutions as fractions.
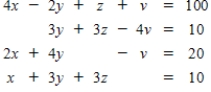
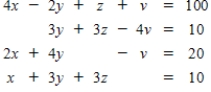

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 111 في هذه المجموعة.
فتح الحزمة
k this deck
62
Use technology to solve the system of equations. Express all solutions as decimals, rounded to one decimal place.
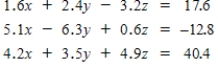
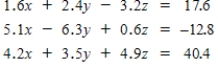

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 111 في هذه المجموعة.
فتح الحزمة
k this deck
63
If adding two linear equations gives and subtracting them gives , then the graphs of those equations are perpendicular.

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 111 في هذه المجموعة.
فتح الحزمة
k this deck
64
Use Gauss-Jordan row reduction to solve the given system of equations.
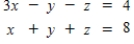
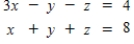

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 111 في هذه المجموعة.
فتح الحزمة
k this deck
65
Use Gauss-Jordan row reduction to solve the system.
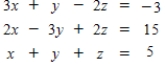
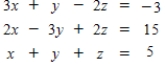

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 111 في هذه المجموعة.
فتح الحزمة
k this deck
66
If the addition or subtraction of two linear equations results in the equation , then the graphs of those equations intersect in a single point.

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 111 في هذه المجموعة.
فتح الحزمة
k this deck
67
If adding two linear equations gives and subtracting them gives , then the graphs of those equations are:
A)perpendicular
B) equal
C) parallel
D) not parallel
E) none of these
A)perpendicular
B) equal
C) parallel
D) not parallel
E) none of these

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 111 في هذه المجموعة.
فتح الحزمة
k this deck
68
Use Gauss-Jordan row reduction to solve the system of equations.
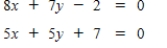
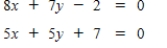

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 111 في هذه المجموعة.
فتح الحزمة
k this deck
69
Use Gauss-Jordan row reduction to solve the given system of equations.
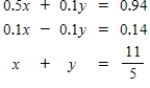
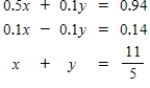

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 111 في هذه المجموعة.
فتح الحزمة
k this deck
70
If the addition or subtraction of two linear equations results in the equation , then the graphs of those equations are _______.
A)equal
B) parallel
C) not parallel
D) perpendicular
E) none of these
A)equal
B) parallel
C) not parallel
D) perpendicular
E) none of these

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 111 في هذه المجموعة.
فتح الحزمة
k this deck
71
If the addition or subtraction of two linear equations results in the equation , then the graphs of those equations are ______.
A)equal
B) not parallel
C) perpendicular
D) parallel
E) none of the above
A)equal
B) not parallel
C) perpendicular
D) parallel
E) none of the above

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 111 في هذه المجموعة.
فتح الحزمة
k this deck
72
If the addition or subtraction of two linear equations results in the equation , then the graphs of those equations are parallel.

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 111 في هذه المجموعة.
فتح الحزمة
k this deck
73
Use Gauss-Jordan row reduction to solve the given system of equations.
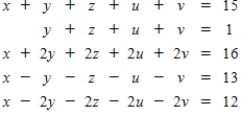
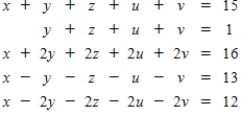

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 111 في هذه المجموعة.
فتح الحزمة
k this deck
74
Find the solution to the system of equations.
A)
B)
C)
D)
E)
A)
B)
C)
D)
E)

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 111 في هذه المجموعة.
فتح الحزمة
k this deck
75
Use Gauss-Jordan row reduction to solve the given system of equations.

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 111 في هذه المجموعة.
فتح الحزمة
k this deck
76
Use Gauss-Jordan row reduction to solve the given system of equations.
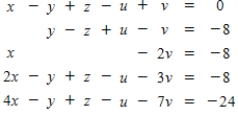
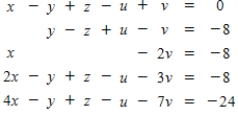

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 111 في هذه المجموعة.
فتح الحزمة
k this deck
77
If the addition or subtraction of two linear equations results in the equation , then the graphs of those equations are perpendicular.

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 111 في هذه المجموعة.
فتح الحزمة
k this deck
78
Use Gauss-Jordan row reduction to solve the given system of equations.
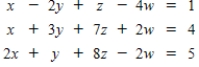
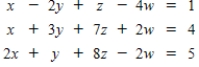

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 111 في هذه المجموعة.
فتح الحزمة
k this deck
79
Use Gauss-Jordan row reduction to solve the given system of equations.
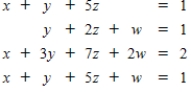
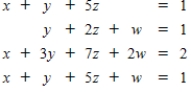

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 111 في هذه المجموعة.
فتح الحزمة
k this deck
80
Use Gauss-Jordan reduction to solve the system of equations.
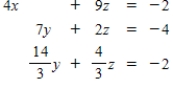
If the system is dependent or inconsistent, indicate this.
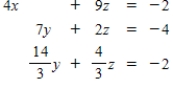
If the system is dependent or inconsistent, indicate this.

فتح الحزمة
افتح القفل للوصول البطاقات البالغ عددها 111 في هذه المجموعة.
فتح الحزمة
k this deck








